On the Adequacy of Some Low-Order Moments Method to Simulate Certain Particle Removal Processes
Abstract
:1. Introduction
2. Theoretical Analysis
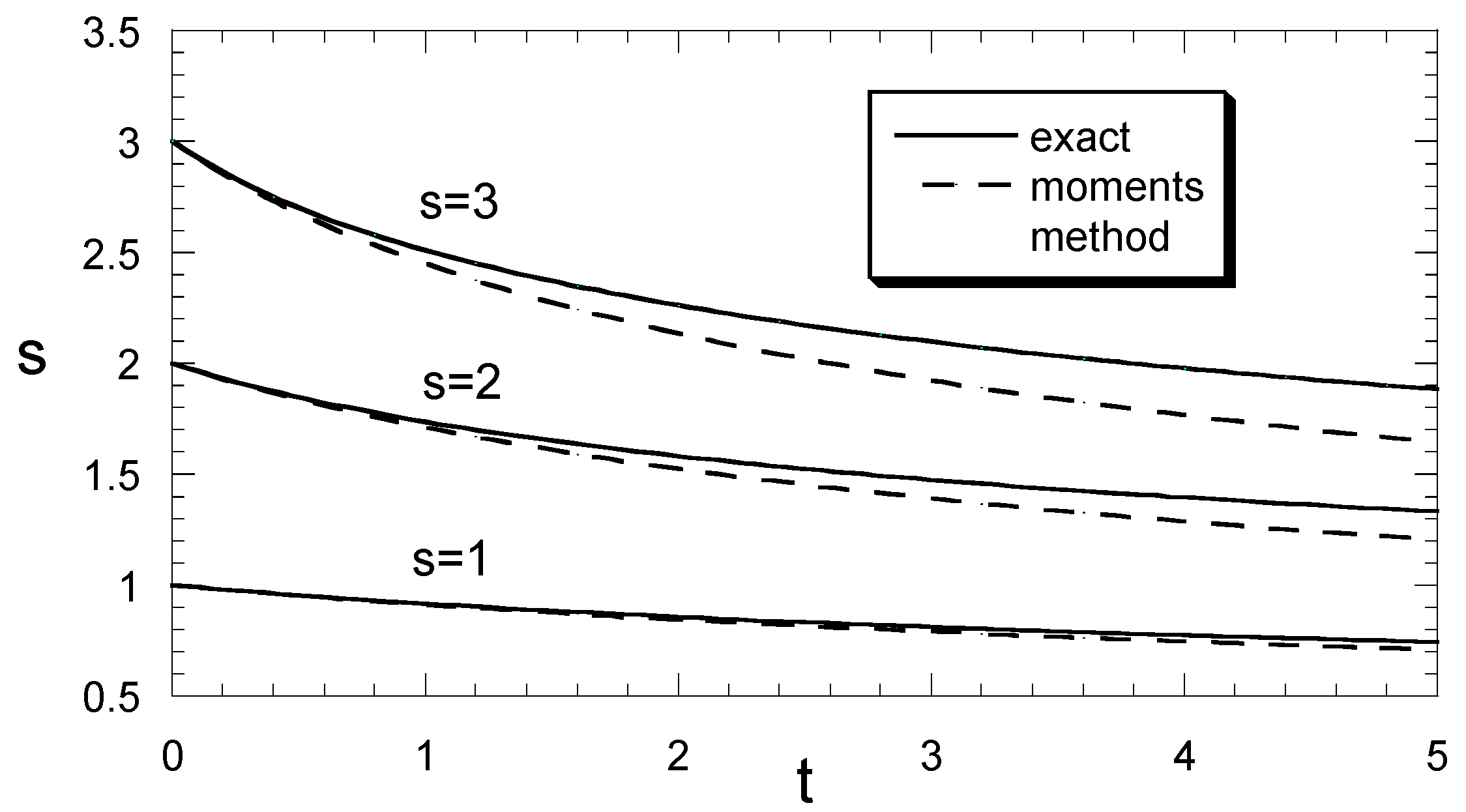
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Kumar, S.; Ramkrishna, D. On the solution of population balance equations by discretization-III. Nucleation, growth and aggregation of particles. Chem. Eng. Sci. 1997, 52, 4659–4679. [Google Scholar] [CrossRef]
- Jurcik, B.J.; Brock, J.R. Numerical simulation of particle formation and growth in rapidly expanding axisymmetric flows. J. Phys. Chem. 1993, 97, 323–331. [Google Scholar] [CrossRef]
- McGraw, R.; Nemesure, S.; Schwartz, S.E. Properties and evolution of aerosol with size distributions having identical moments. J. Aerosol Sci. 1998, 29, 761–772. [Google Scholar] [CrossRef]
- Barret, J.C.; Jheeta, J.S. Improving the accuracy of the moments method for solving the aerosol general dynamic equation. J. Aerosol Sci. 1996, 27, 1135–1142. [Google Scholar] [CrossRef]
- McGraw, R. Description of atmospheric aerosol dynamics by the quadrature method of moments. Aerosol Sci. Technol. 1997, 27, 255–265. [Google Scholar] [CrossRef]
- Yu, M.; Liu, Y.; Lin, J.; Seipenbusch, M. Generalized TEMOM scheme for solving the population balance equation. Aerosol Sci. Technol. 2015, 49, 1021–1036. [Google Scholar] [CrossRef] [Green Version]
- Falola, A.; Borissova, A.; Wang, X.Z. Extended method of moment for general population balance models including size dependent growth rate, aggregation and breakage kernels. Comp. Chem. Eng. 2013, 56, 1–11. [Google Scholar] [CrossRef] [Green Version]
- Kielkiewicz, M. Accuracy of the moments method. Ann. Nucl. Energy 1994, 21, 189–193. [Google Scholar] [CrossRef]
- Williams, M.M.R.; Loyalka, S.K. Aerosol Science: Theory and Practice; Pergamon Press: New York, NY, USA, 1991. [Google Scholar]
- Press, W.; Flannery, B.; Teukolski, S.; Vetterling, W. Numerical Recipes; Cambridge University Press: New York, NY, USA, 1986. [Google Scholar]
- Kostoglou, M.; Karabelas, A.J. On modeling incipient crystallization of sparingly soluble salts in frontal membrane filtration. J. Colloid Interface Sci. 2011, 362, 202–214. [Google Scholar] [CrossRef] [PubMed]
- Kostoglou, M.; Karapantsios, T.D. Population balance modeling of flotation pulp: The route from process frequency functions to spatially distributed models. Comp. Chem. Eng. 2021, 155, 107506. [Google Scholar] [CrossRef]
- Friedlander, S.K. Smoke, Dust and Haze; Wiley Interscience: New York, NY, USA, 1977. [Google Scholar]
- Williams, M.M.R. The time-dependent behavior of aerosols with growth and deposition I. Without coagulation. J. Colloid Interface Sci. 1983, 93, 252–263. [Google Scholar] [CrossRef]
- Jain, S.; Kodas, T.T. Asymptotic widths of size distributions resulting from collisional growth assuming log-normally distributed fractal aggregates. J. Aerosol Sci. 1998, 29, 259–261. [Google Scholar] [CrossRef]
- Williams, M.M.R. On the modified Gamma distribution for representing the size spectra of coagulating aerosol particles. J. Colloid Interface Sci. 1985, 103, 516–527. [Google Scholar] [CrossRef]
- Tsang, T.H.; Rao, A. Comparison of different numerical schemes for condensational growth of aerosols. Aerosol Sci. Technol. 1988, 9, 271–277. [Google Scholar] [CrossRef]
- Kostoglou, M.; Karabelas, A.J. Comprehensive modeling of precipitation and fouling in turbulent pipe flow. Ind. Eng. Res. Chem. 1998, 37, 1536–1550. [Google Scholar] [CrossRef]
- Kostoglou, M.; Karabelas, A.J. Evaluation of numerical methods for simulating an evolving particle size distribution in growth processes. Chem. Eng. Comm. 1995, 136, 177–199. [Google Scholar] [CrossRef]
- Kostoglou, M. Extended cell average technique for the solution of coagulation equation. J. Colloid Interface Sci. 2007, 306, 72–81. [Google Scholar] [CrossRef] [PubMed]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kostoglou, M.; Karapantsios, T.D. On the Adequacy of Some Low-Order Moments Method to Simulate Certain Particle Removal Processes. Colloids Interfaces 2021, 5, 46. https://doi.org/10.3390/colloids5040046
Kostoglou M, Karapantsios TD. On the Adequacy of Some Low-Order Moments Method to Simulate Certain Particle Removal Processes. Colloids and Interfaces. 2021; 5(4):46. https://doi.org/10.3390/colloids5040046
Chicago/Turabian StyleKostoglou, Margaritis, and Thodoris D. Karapantsios. 2021. "On the Adequacy of Some Low-Order Moments Method to Simulate Certain Particle Removal Processes" Colloids and Interfaces 5, no. 4: 46. https://doi.org/10.3390/colloids5040046
APA StyleKostoglou, M., & Karapantsios, T. D. (2021). On the Adequacy of Some Low-Order Moments Method to Simulate Certain Particle Removal Processes. Colloids and Interfaces, 5(4), 46. https://doi.org/10.3390/colloids5040046






