Physicochemical Parameters for Brea Gum Exudate from Cercidium praecox Tree
Abstract
1. Introduction
2. Materials and Methods
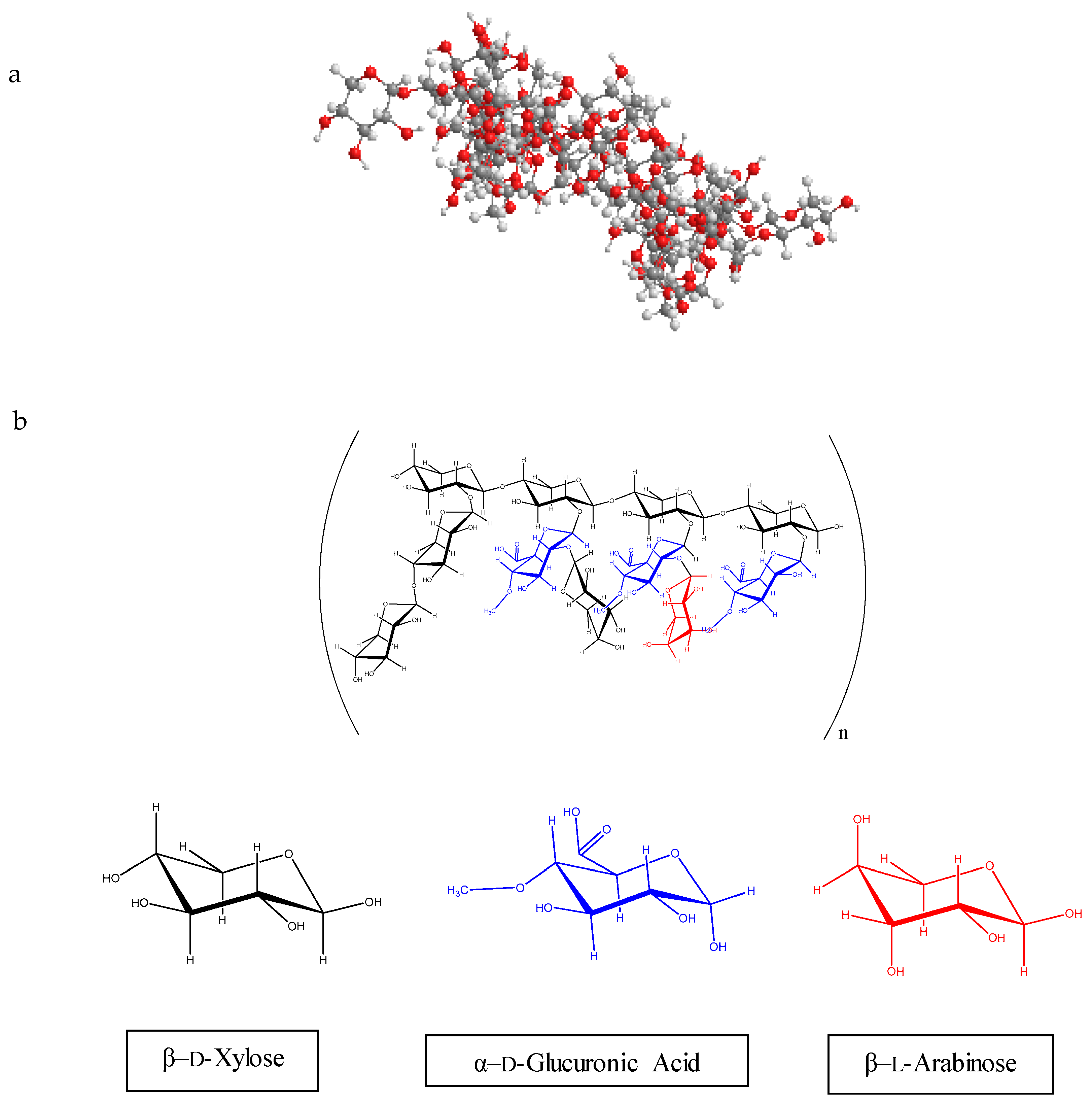
2.1. Brea Gum Extraction, Purification and Hydrolysis
2.2. Size-Exclusion Chromatography (SEC)
2.3. Dynamic Light Scattering
2.4. Intrinsic Viscosity Measurement
3. Results and Discussion
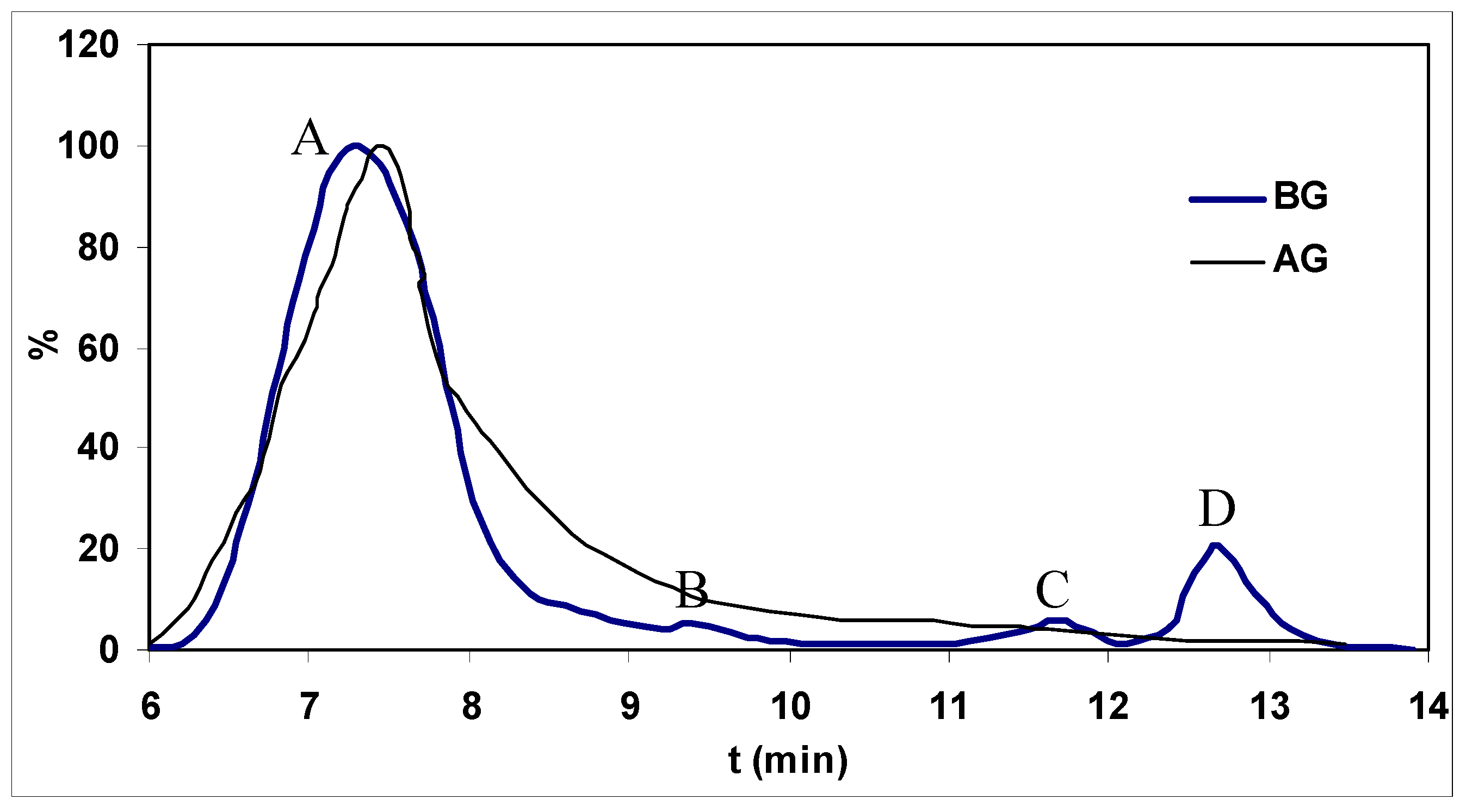
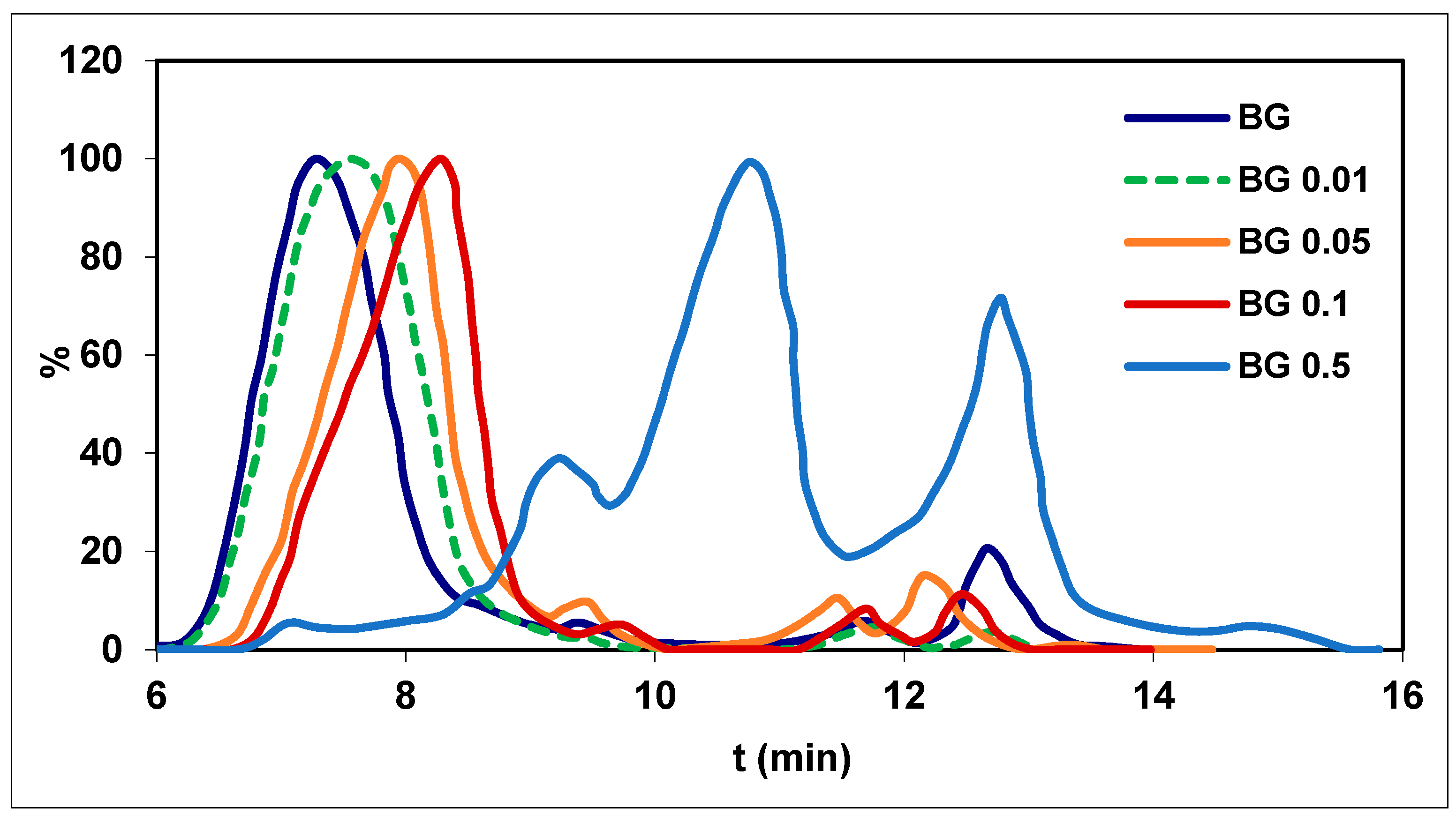
3.1. SEC Determination
3.2. Intrinsic Viscosity and Density
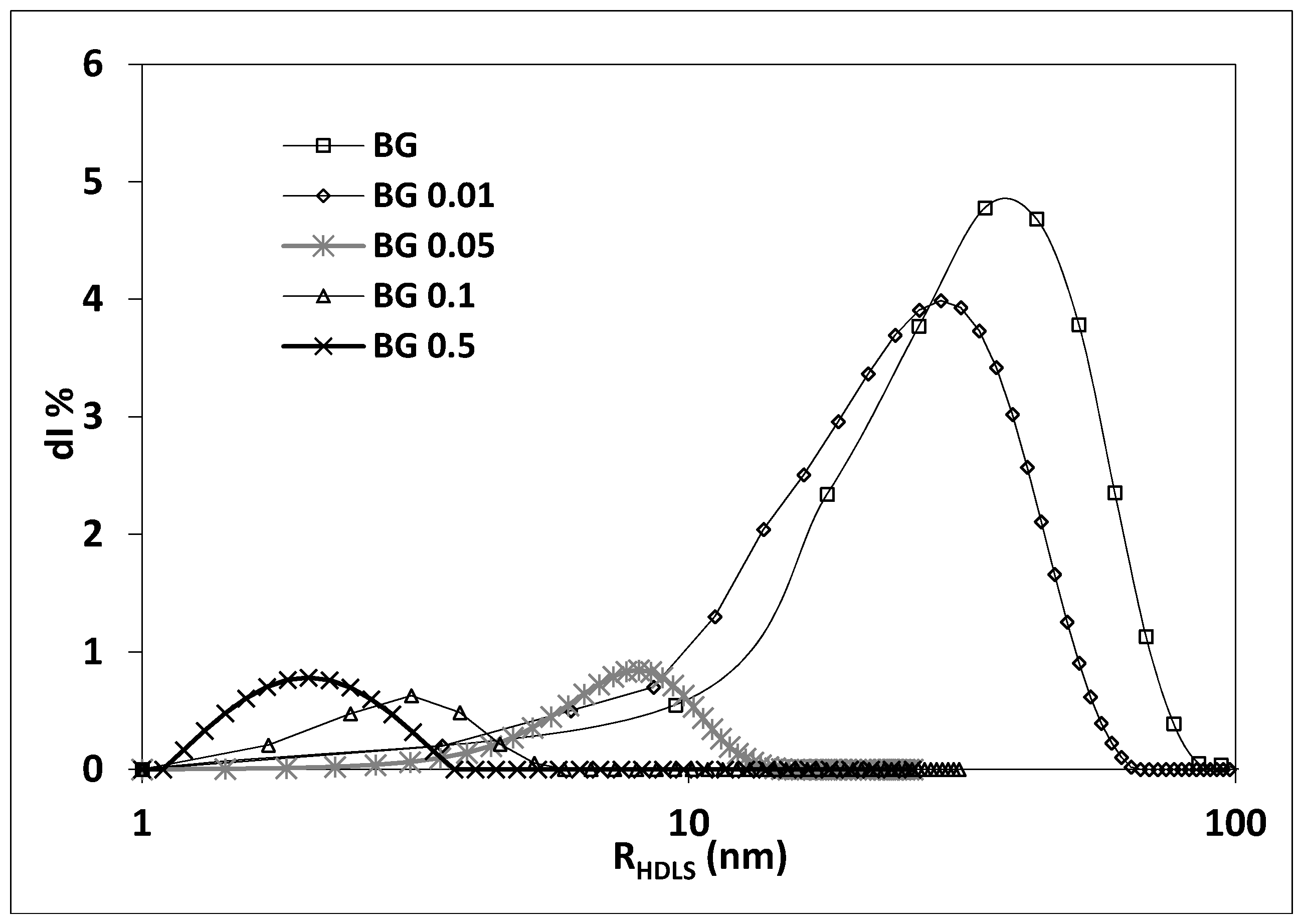
3.3. Dynamic Light Scattering Determination
4. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Wareing, M.V. Exudates gums. In Thickening and Gelling Agents for Food; Imeson, A.P., Ed.; Springer Science & Business Media: Hong Kong, China, 1999; pp. 86–118. [Google Scholar]
- Yaseen, E.I.; Herald, T.J.; Aramouni, F.M.; Alavi, S. Rheological properties of selected gum solutions. Food Res. Int. 2005, 38, 111–119. [Google Scholar] [CrossRef]
- Verbeken, D.; Dierckx, S.; Dewettinck, K. Exudate gums: Occurrence, production, and applications. Appl. Microbiol. Biotechnol. 2003, 63, 10–21. [Google Scholar] [CrossRef] [PubMed]
- Bertuzzi, M.A.; Slavutsky, A.M.; Armada, M. Physicochemical characterisation of the hydrocolloid from Brea tree (Cercidium praecox). Int. J. Food Sci. Technol. 2012, 47, 776–782. [Google Scholar] [CrossRef]
- von Müller, A.R.; López, C.B.; Eynard, A.R.; Guzmán, C.A. Subchronic toxicological evaluation of brea gum (Parkinsonia praecox) as a food additive in BALB/c mice. Drug Chem. Toxicol. 2009, 32, 307–311. [Google Scholar] [CrossRef]
- Cerezo, A.S.; Stacey, M.; Webber, J.M. Some Structural Studies of Brea Gum (an Exudate from Cercidium Australe Johnst.). Carbohydr. Res. 1969, 9, 505–517. [Google Scholar] [CrossRef]
- Ministerio de Agricultura, Ganadería y Pesca. Goma Brea: Un Producto con Potencial para el Desarrollo de la Economía Regional; Subsecretaría de Desarrollo de Economías Regionales, Universidad Nacional de Salta: Salta, Argentina, 2010. [Google Scholar]
- De Pinto, G.; Rodriguez, O.; Martinez, M.; Rivas, C. Composition of cercidium praecox gum exudates. Biochem. Syst. Ecol. 1993, 21, 297–300. [Google Scholar] [CrossRef]
- De Pinto, G.L.; Martínez, M.; Rivas, C. Chemical and spectroscopic studies of cercidium praecox gum exudate. Carbohydr. Res. 1994, 260, 17–25. [Google Scholar] [CrossRef]
- Tischer, C. The free reducing oligosaccharides of gum arabic: Aids for structural assignments in the polysaccharide. Carbohydr. Polym. 2002, 47, 151–158. [Google Scholar] [CrossRef]
- Wang, Q.; Burchard, W.; Cui, S.W.; Huang, X.; Phillips, G.O. Solution properties of conventional gum arabic and a matured gum arabic (acacia (sen) super gum). Biomacromolecules 2008, 9, 1163–1169. [Google Scholar] [CrossRef] [PubMed]
- Castel, V.; Zivanovic, S.; Jurat-Fuentes, J.L.; Santiago, L.G.; Rubiolo, A.C.; Carrara, C.R.; Harte, F.M. Chromatographic fractionation and molecular mass characterization ofcercidium praecox(brea) gum. J. Sci. Food Agric. 2016, 96, 4345–4350. [Google Scholar] [CrossRef] [PubMed]
- Bertuzzi, M.A.; Slavutsky, A.M. Formulation and Characterization of Film Based on Gum Exudates from Brea Tree (Cercidium praecox). J. Food Sci. Eng. 2013, 3, 113–122. [Google Scholar]
- Slavutsky, A.M.; Bertuzzi, M.A.; Armada, M.; García, M.G.; Ochoa, N.A. Preparation and characterization of montmorillonite/brea gum nanocomposites films. Food Hydrocolloids 2014, 35, 270–278. [Google Scholar] [CrossRef]
- Slavutsky, A.M.; Bertuzzi, M.A. Thermodynamic study of water sorption and water barrier properties of nanocomposite films based on brea gum. Appl. Clay Sci. 2015, 108, 144–148. [Google Scholar] [CrossRef]
- Spotti, M.L.; Cecchini, J.P.; Spotti, M.J.; Carrara, C.R. Brea gum (from cercidium praecox) as a structural support for emulsion-based edible films. LWT-Food Sci. Technol. 2016, 68, 127–134. [Google Scholar] [CrossRef]
- Mellinas, C.; Valdés, A.; Ramos, M.; Burgos, N.; del Carmen Garrigós, M.; Jiménez, A. Active edible films: Current state and future trends. J. Appl. Polym. Sci. 2016, 133. [Google Scholar] [CrossRef]
- López, E.P.; Jiménez, P.L. Effect of different proportions of brea gum in the functional characteristics of wheat flour starch: Impact on the physical quality of bread. Food Sci. Technol. (Campinas) 2016, 36, 83–89. [Google Scholar] [CrossRef]
- Castel, V.; Rubiolo, A.C.; Carrara, C.R. Droplet size distribution, rheological behavior and stability of corn oil emulsions stabilized by a novel hydrocolloid (brea gum) compared with gum arabic. Food Hydrocolloids 2017, 63, 170–177. [Google Scholar] [CrossRef]
- López, E.P.; Goldner, M.C. Influence of storage time for the acceptability of bread formulated with lupine protein isolate and added brea gum. LWT-Food Sci. Technol. 2015, 64, 1171–1178. [Google Scholar] [CrossRef]
- López, E.P.; Pérez, G.T.; Jiménez de Erramouspe, P.L.; Cuevas, C.M. Effect of Brea Gum on the characteristics of wheat bread at different storage times. Food Sci. Technol. (Campinas) 2013, 33, 745–752. [Google Scholar] [CrossRef]
- Heineck, M.E.; Cardoso, M.B.; Giacomelli, F.C.; da Silveira, N.P. Evidences of amylose coil-to-helix transition in stored dilute solutions. Polymer 2008, 49, 4386–4392. [Google Scholar] [CrossRef]
- Rojas, R.; Giacomelli, C.E. Effect of structure and bonding on the interfacial properties and the reactivity of layered double hydroxides and zn hydroxide salts. Colloids Surf. A: Physicochem. Eng. Asp. 2013, 419, 166–173. [Google Scholar] [CrossRef]
- dos Santos, M.C.; Kroetz, T.; Dora, C.L.; Giacomelli, F.C.; Frizon, T.E.A.; Pich, C.T.; da Silva Pinto, L.; Soares, A.S.; Rodembusch, F.S.; Lima, V.R.; et al. Elucidating Bauhinia variegata lectin/phosphatidylcholine interactions in lectin-containing liposomes. J. colloid Interface Sci. 2018, 519, 232–241. [Google Scholar] [CrossRef]
- Masuelli, M.A. Hydrodynamic Parameters of Strelitzia Gum. Colloids Interfaces 2018, 2, 45. [Google Scholar] [CrossRef]
- Matsuoka, S.; Cowman, M.K. Equation of state for polymer solution. Polymer 2002, 43, 3447–3453. [Google Scholar] [CrossRef]
- Sun, S.F. Physical Chemistry of Macromolecules: Basic Principles and Issues, 2nd ed.; Wiley: Hoboken, NJ, USA, 2004; pp. 165–184. [Google Scholar]
- Masuelli, M.A. Viscometric study of pectin. Effect of temperature on the hydrodynamic properties. Int. J. Biological Macromol. 2011, 48, 286–291. [Google Scholar] [CrossRef] [PubMed]
- Harding, S.E.; Varum, K.; Stoke, B.; Smidsrod, O. Molecular weight determination of polysaccharides. Adv. Carbohydr. Anal. 1991, 1, 63–144. [Google Scholar]
- Harding, S.E. The Viscosity Intrinsic of Biological Macromolecules. Progress in Measurement, Interpretation and Application to Structure in Dilute Solution. Progr. Biophys. Mol. Biol. 1997, 68, 207–262. [Google Scholar] [CrossRef]
- Masuelli, M.A. Study of Bovine Serum Albumin Solubility in Aqueous Solutions by Intrinsic Viscosity Measurements. Adv. Phys. Chem. 2013, 2013. [Google Scholar] [CrossRef]
- Garcia de la Torre, J.; Carrasco, B. Universal size-independent quantities for the conformational characterization of rigid and flexible macromolecules. Progr. Colloid Polym. Sci. 1999, 113, 81–86. [Google Scholar]
- Ortega, A.; García de la Torre, J. Equivalent radii and ratios of radii from solution properties as indicators of macromolecular conformation, shape, and flexibility. Biomacromolecules 2007, 8, 2464–2475. [Google Scholar] [CrossRef] [PubMed]
- Masuelli, M.A. Hydrodynamic Properties of Whole Arabic Gum. Am. J. Food Sci. Technol. 2013, 1, 60–66. [Google Scholar]
- Morris, G.A.; Patel, T.R.; Picout, D.R.; Ross-Murphy, S.B.; Ortega, A.; Garcia de la Torre, J.; Harding, S.E. Global hydrodynamic analysis of the molecular flexibility of galactomannans. Carbohydr. Polym. 2008, 72, 356–360. [Google Scholar] [CrossRef]
- Lewandowska, K.; Staszewska, D.U.; Bohdanecký, M. The huggins viscosity coefficient of aqueous solution of poly(vinyl alcohol). Eur. Polym. J. 2001, 37, 25–32. [Google Scholar] [CrossRef]
- Curvale, R.; Masuelli, M.; Padilla, A.P. Intrinsic viscosity of bovine serum albumin conformers. Int. J. Biological Macromol. 2008, 42, 133–137. [Google Scholar] [CrossRef] [PubMed]
- Chenlo, F.; Moreira, R.; Pereira, G.; Silva, C. Rheological modelling of binary and ternary systems of tragacanth, guar gum and methylcellulose in dilute range of concentration at different temperatures. LWT-Food Sci. Technol. 2009, 42, 519–524. [Google Scholar] [CrossRef]
- Lapasin, R.; Pricl, S. Rheology of polysaccharide systems. In Rheology of Industrial Polysaccharides: Theory and Applications; Glasgow: Blackie, UK, 1995; pp. 250–494. [Google Scholar]
- Anderson, D.M.W.; Rahman, S. Studies on uronic acid materials: Part XX. The viscosity–molecular weight relationship for acacia gums. Carbohydr. Res. 1967, 4, 298–304. [Google Scholar] [CrossRef]
- Idris, O.H.M.; Williams, P.A.; Phillips, G.O. Characterisation of gum from acacia senegal trees of different age and location using multidetection gel permeation chromatography. Food Hydrocolloids 1998, 12, 379–388. [Google Scholar] [CrossRef]
- Assaf, S.; Phillips, G.; Williams, P. Studies on acacia exudate gums. Part I: The molecular weight of gum exudate. Food Hydrocolloids 2005, 19, 647–660. [Google Scholar] [CrossRef]
- Al-Assaf, S.; Phillips, G.O.; Williams, P.A. Studies on acacia exudate gums: Part ii. Molecular weight comparison of the Vulgares and Gummiferae series of Acacia gums. Food Hydrocolloids 2005, 19, 661–667. [Google Scholar] [CrossRef]
- Al-Assaf, S.; Hassan, E.A.; Phillips, G.O.; Williams, P.A. Studies on Acacia gums: Part III molecular weight characteristics of Acacia seyal var. seyal and Acacia seyal var fistula. Food Hydrocolloids 2005, 19, 669–677. [Google Scholar]
- Savary, G.; Hucher, N.; Bernadi, E.; Grisel, M.; Malhiac, C. Relationship between the emulsifying properties of acacia gums and the retention and diffusion of aroma compounds. Food Hydrocolloids 2010, 24, 178–183. [Google Scholar] [CrossRef]
- Sanchez, C.; Renard, D.; Robert, P.; Schmitt, C.; Lefebvre, J. Structure and rheological properties of acacia gum dispersions. Food Hydrocolloids 2002, 16, 257–267. [Google Scholar] [CrossRef]
- Sanchez, C.; Schmitt, C.; Kolodziejczyk, E.; Lapp, A.; Gaillard, C.; Renard, D. The acacia gum arabinogalactan fraction is a thin oblate ellipsoid: A new model based on small-angle neutron scattering and ab initio calculation. Biophys. J. 2008, 94, 629–639. [Google Scholar] [CrossRef] [PubMed]
- Guner, A. Unperturbed dimensions and theta temperature of dextran in aqueous solutions. J. Appl. Polym. Sci. 1999, 72, 871–876. [Google Scholar] [CrossRef]
- Güner, A.; Kibarer, G. The important role of thermodynamic interaction parameter in the determination of theta temperature, dextran/water system. Eur. Polym. J. 2001, 37, 619–622. [Google Scholar] [CrossRef]
- Lee, K.Y.; Bouhadir, K.H.; Mooney, D.J. Evaluation of chain stiffness of partially oxidized polyguluronate. Biomacromolecules 2002, 3, 1129–1134. [Google Scholar] [CrossRef] [PubMed]
| c (g cm−3) | t (s) | ρ (g cm−3) | ηr |
|---|---|---|---|
| 0.0010 | 46.44 | 0.9988 | 1.052451 |
| 0.0025 | 49.79 | 0.9992 | 1.128822 |
| 0.0050 | 55.11 | 1.0002 | 1.250686 |
| 0.0075 | 60.15 | 1.0011 | 1.366294 |
| 0.0100 | 64.92 | 1.0020 | 1.475969 |
| Huggins | Kraemer | Schulz-Blaschke | Martin | |
|---|---|---|---|---|
| [η] (cm3/g) | 52.91 | 51.99 | 53.02 | 52.96 |
| RE% | - | 1.7388 | 0.2079 | 0.0908 |
| (cm3/g) | RH (nm) | δ (g/g) |
|---|---|---|
| 0.6424 | 32.49 | 20.5483 |
| vsp (cm3/g) | va-b | p |
| 21.1640 | 2.5031 | 1.0524 |
| BG Hydrolyzed | ts (s) | ηr | ηsp | [η] (cm3/g) | RH (nm) |
|---|---|---|---|---|---|
| BG 0.01 | 53.09 | 1.2416 | 0.24158 | 44.8958 | 25.12 |
| BG 0.05 | 45.79 | 1.0709 | 0.07086 | 13.8506 | 6.58 |
| BG 0.10 | 44.69 | 1.0451 | 0.04513 | 8.8948 | 4.00 |
| BG 0.50 | 42.96 | 1.0047 | 0.00467 | 1.9340 | 0.70 |
| Sample | RHDLS(max) (nm) | RHDLS(mean) (nm) | MD (g mol−1) | Mw (g mol−1) | Mv (g mol−1) |
|---|---|---|---|---|---|
| BG | 34.89 | 40.43 | 2,134,000 | 2,320,000 | 1,890,000 |
| BG 0.01 | 25.14 | 30.21 | 1,245,000 | 1,249,000 | 1,268,000 |
| BG 0.05 | 8.21 | 8.08 | 109,000 | 72,600 | 73,500 |
| BG 0.10 | 3.11 | 3.16 | 19,200 | 25,500 | 26,000 |
| BG 0.50 | 2.02 | 2.23 | 1000 | 700 | 622 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Masuelli, M.A.; Slatvustky, A.; Ochoa, A.; Bertuzzi, M.A. Physicochemical Parameters for Brea Gum Exudate from Cercidium praecox Tree. Colloids Interfaces 2018, 2, 72. https://doi.org/10.3390/colloids2040072
Masuelli MA, Slatvustky A, Ochoa A, Bertuzzi MA. Physicochemical Parameters for Brea Gum Exudate from Cercidium praecox Tree. Colloids and Interfaces. 2018; 2(4):72. https://doi.org/10.3390/colloids2040072
Chicago/Turabian StyleMasuelli, Martin A., Aníbal Slatvustky, Ariel Ochoa, and M. Alejandra Bertuzzi. 2018. "Physicochemical Parameters for Brea Gum Exudate from Cercidium praecox Tree" Colloids and Interfaces 2, no. 4: 72. https://doi.org/10.3390/colloids2040072
APA StyleMasuelli, M. A., Slatvustky, A., Ochoa, A., & Bertuzzi, M. A. (2018). Physicochemical Parameters for Brea Gum Exudate from Cercidium praecox Tree. Colloids and Interfaces, 2(4), 72. https://doi.org/10.3390/colloids2040072






