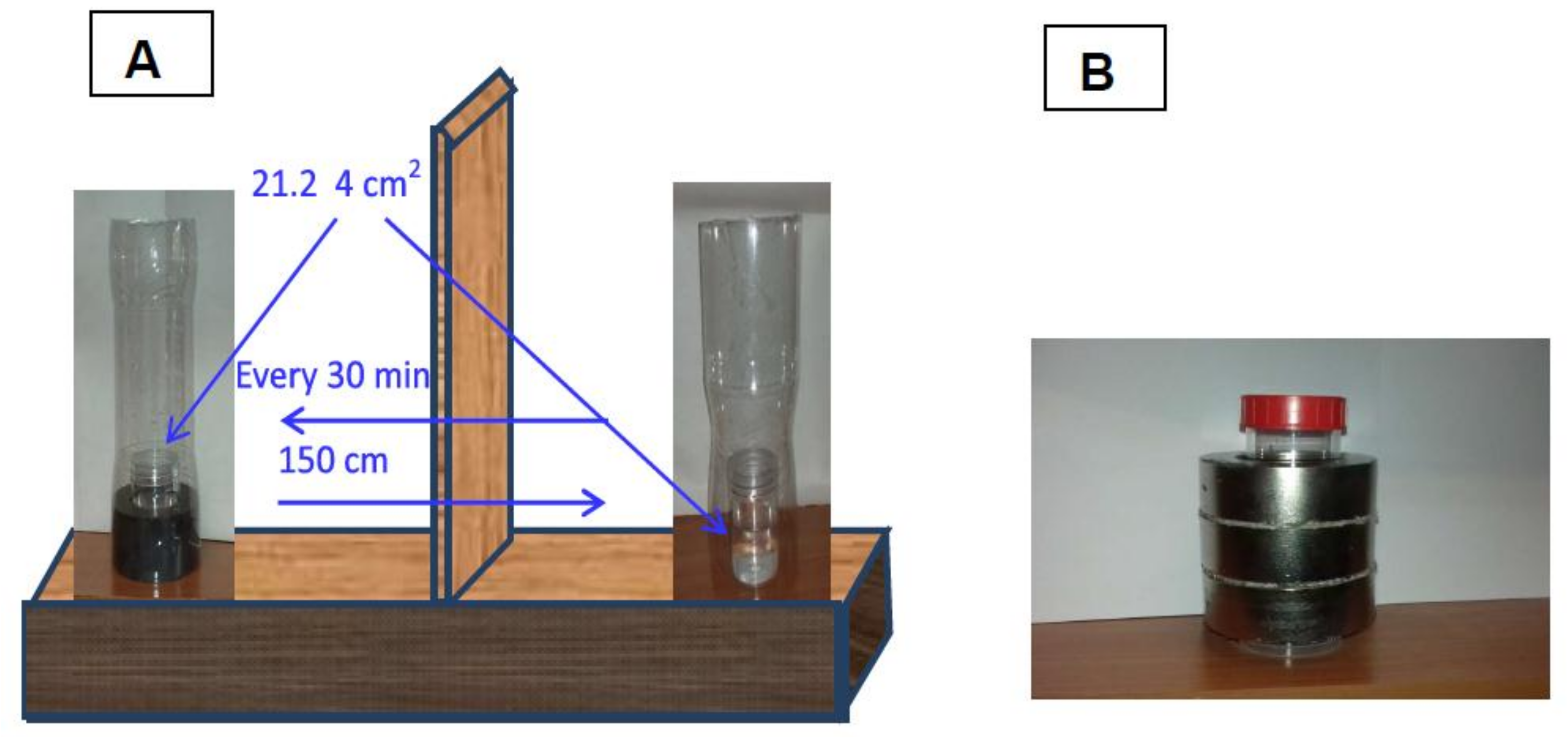
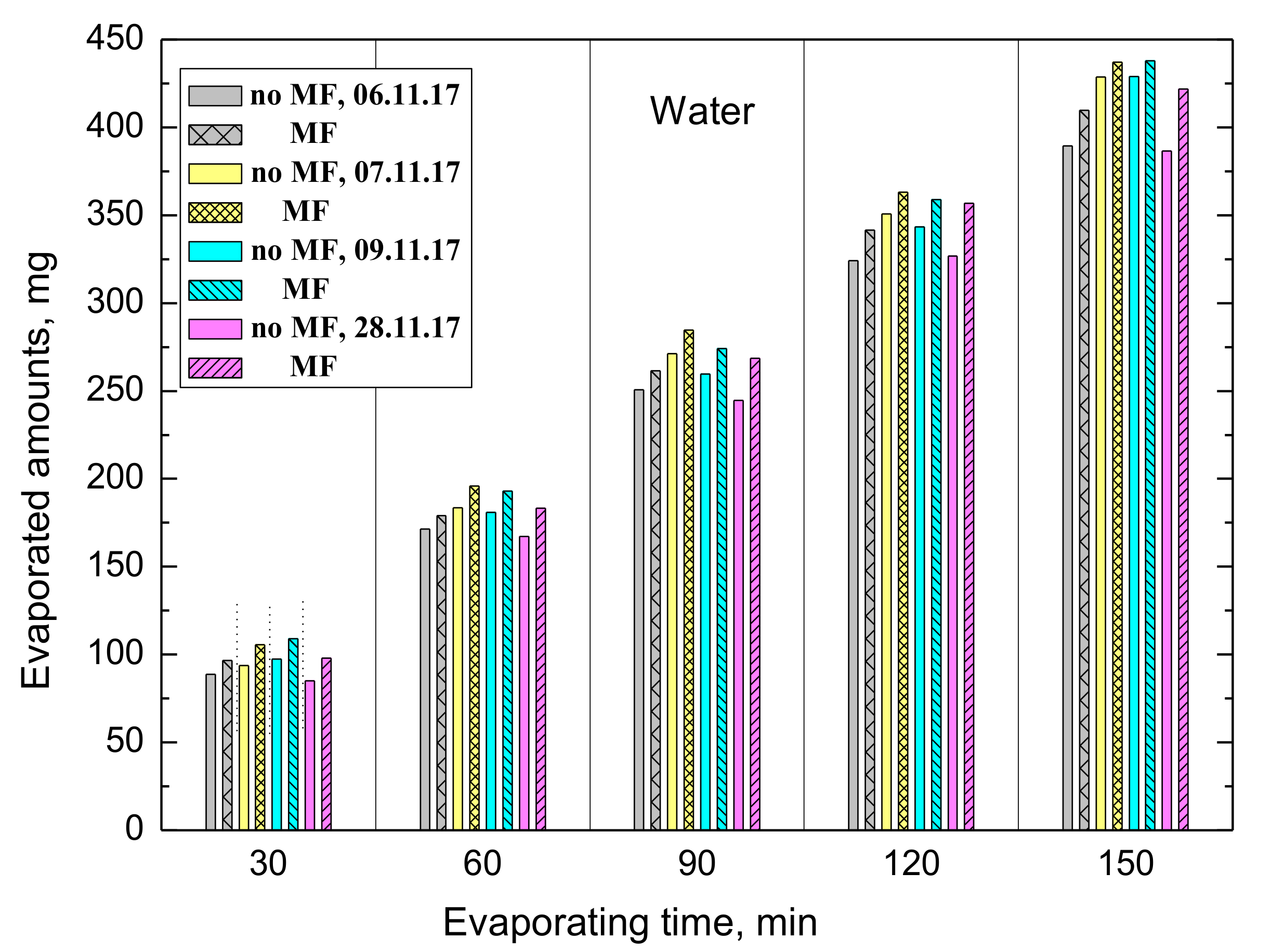
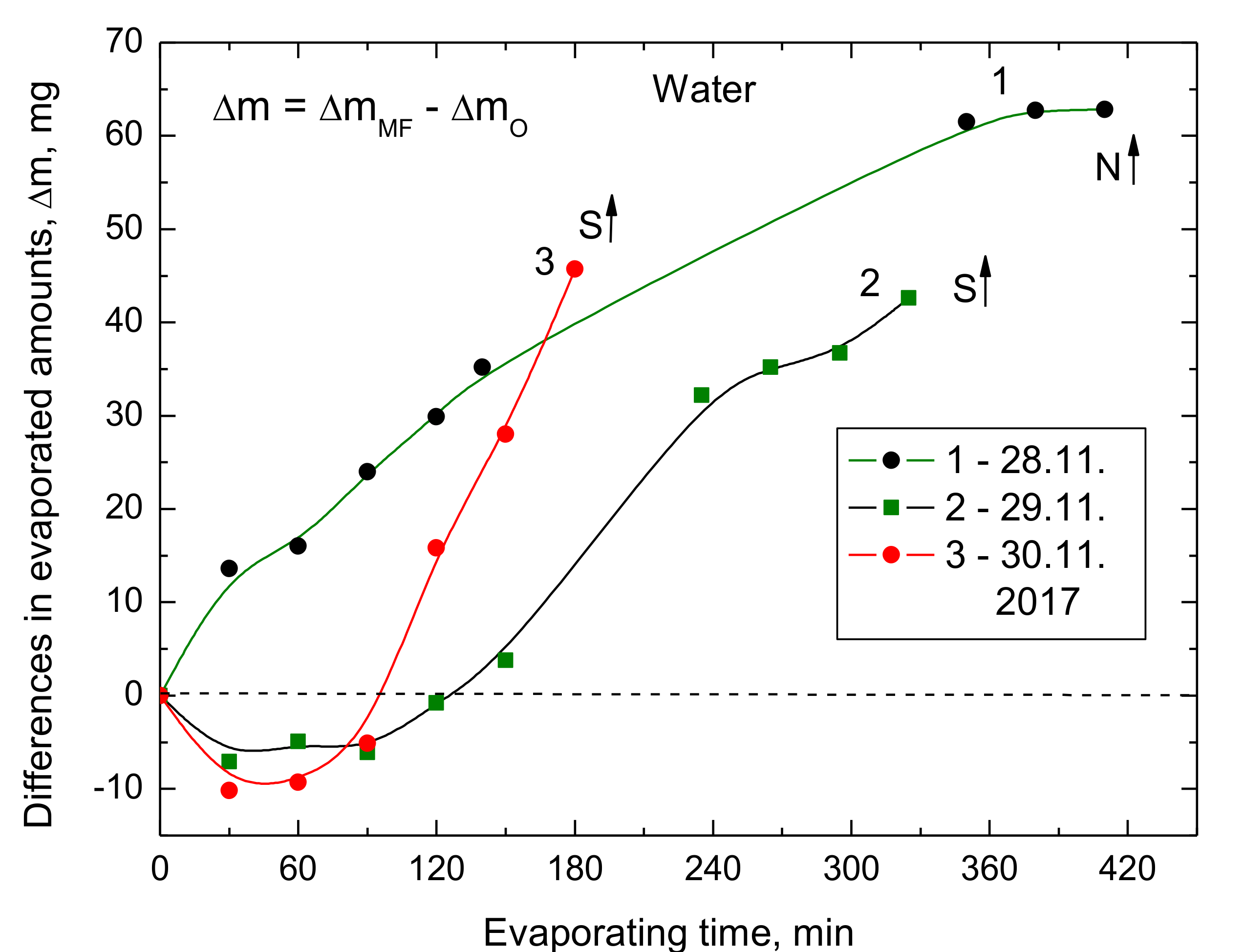
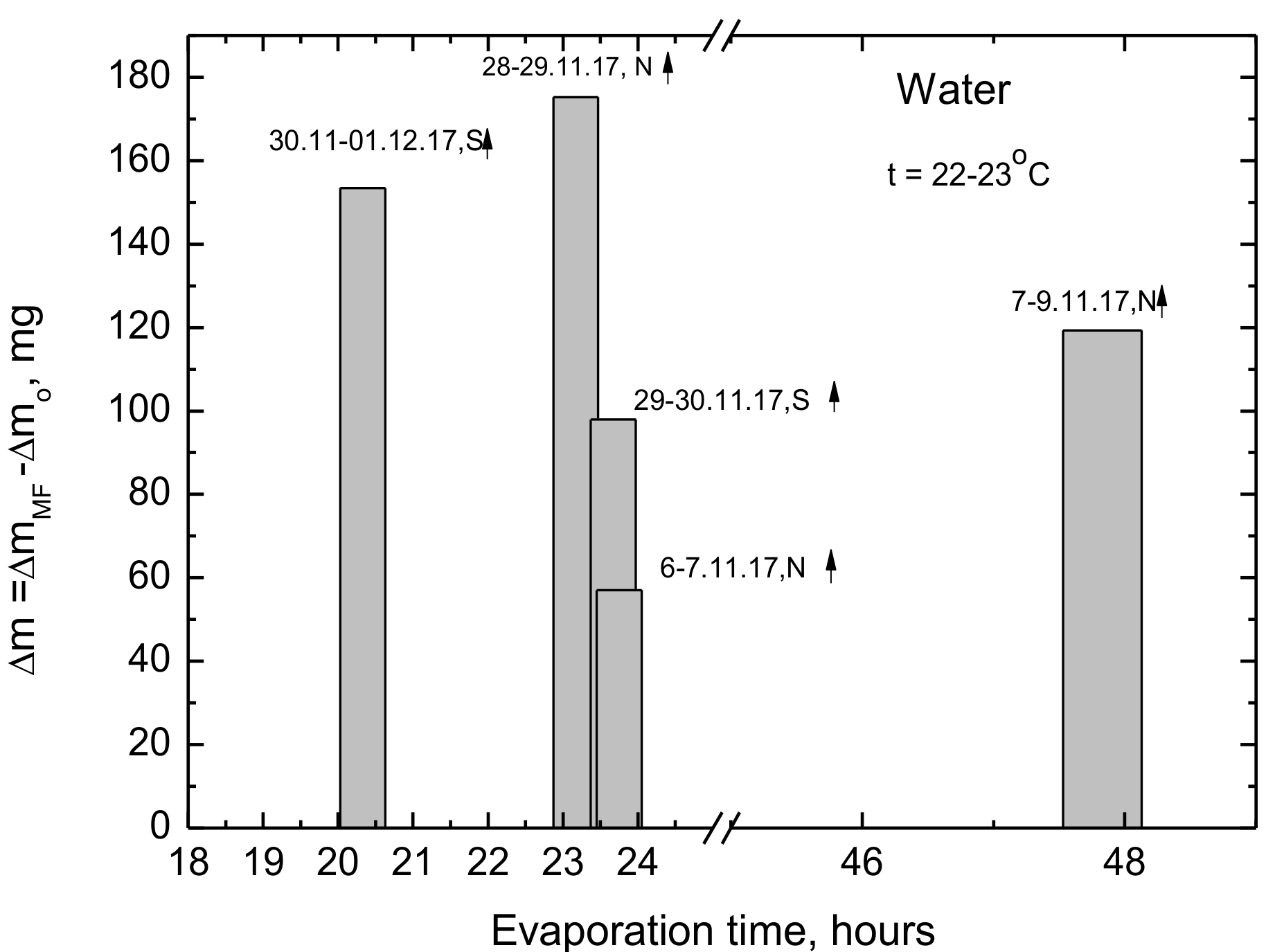
1. Introduction
The effects of magnetic field (MF) on water treatment have been studied over 50 years in various aspects, and they still attract many researchers. Originally, the studies were focused on the protection of industrial installations or home-heating systems against hard scale formation at elevated temperatures; later and recently, the MF effects in the liquid phase have been studied in various fundamental aspects as well as possible practical applications. A broad scope of such MF effects can be found in several review articles published lately [
1,
2,
3,
4,
5,
6], including an older one published by Baker and Judd [
1] in 1996.
Among various investigated MF effects, several papers deal with pure water [
7,
8,
9,
10,
11,
12,
13,
14], where changes in the evaporation rate, surface tension, viscosity and other parameters were observed [
15,
16]. The authors reported an increase in water evaporation rate caused by MF which depended also on the experiment setup. Moreover, it was found [
7] that the effect depended more on the product of MF strength and its gradient than on the field itself (B·dB/dx). Also, the direction of air or oxygen flow relative to the MF gradient affected the evaporation rate. Larger influence was observed in pure oxygen flow than in air because of its larger-volume magnetic susceptibility χ (= M/H, where M is the magnetization of the material and H is the MF strength, which are in A/m, χ
ox >> χ
water). The flow could cause a susceptibility gradient in the vertical direction during the water vaporization and the vaporization from the surface parallel to the field gradient was enhanced. These experiments were conducted in a strong 8 T superconducting solenoid MF which was confirmed later by Guo et al. [
12] who also used the superconducting magnet and simulated gravity. These authors also mentioned the importance of Lorentz and magnetization forces for the observed effect as well as that hydrogen bonds must be broken and van der Waals forces weakened during increased water evaporation [
13,
17].
A 6% increase in water evaporation treated in 0.5 T MF was reported by Rashid et al. [
11] but only if the origin of the field was placed at the water/air interface level and no effect was observed if the MF was positioned in the middle or bottom of the sample. However, the evaporated amount calculated from the Fick’s law for diffusion appeared to be much bigger than the experimental amount obtained not only for the MF-treated but also the untreated samples.
Holysz et al. [
9] found that the evaporated amount of water from the aqueous solutions of inorganic electrolytes (0.1 M NaCl, KCl, Na
3PO
4, and CaCl
2) was smaller after a weak MF (magnetic stack B = 15 mT) treatment than from pure water. Also, changes in electric conductivity occurred which were related to the thermodynamic hydration functions of these ions. Hence the authors concluded that the hydration shell structures around the ions were changed by the MF. This was proved by molecular simulations made by Chang and Weng [
18]. At a relatively low NaCl concentration (1M) and strong MF (1–10T) the coefficient of water self-diffusion decreases if the field increases while at a high concentration the change is opposite. However, the mobility of Na
+ and Cl
− ions increases regardless of their concentration and the hydrogen bonds damage takes place.
Seyfi et al. [
13] interpreted the increased evaporation of water based on the kinetic energy of water molecules and the Lorentz force acting on the charged molecules. It might cause weakening or even breaking of the hydrogen bonding. The experiments were conducted using the ring magnets and the samples were placed inside the ring. The water surface level was at the middle of the magnet height whose north pole was directed up and the MF field was perpendicular to the water surface. After 80 min ca. 19% more water evaporated from MF-treated (75 mT, three ring magnets) than from the untreated sample. The difference increased linearly with the experiment duration. However, there was no difference in the evaporation rate of the samples if the MF was tangentially directed to the water surface (two N-N poles connected magnets). The calculations have shown that under the experimental conditions [
13] the difference between the volume magnetic force and the gravity force per unit air volume was too small to influence convection of water molecules if caused by the MF action. Therefore, to explain the effect which was observed only perpendicular but not tangential to the water surface MF field, the authors [
13] considered the Lorentz force acting randomly on the bouncing dipoles. The literature reports the data dealing with the presence of the electrostatic potential across the water/vapor interface [
19,
20]. Thus, the Lorentz force normal to the water surface can influence the momenta of hydrogen bonds in the clusters, weaken or break them, leading to an increased evaporation rate is observed [
13].
Recently Amor et al. [
14] published a statistically significant MF effect on the rate of evaporation of irrigation water at different temperatures using different commercial magnets (0.33 T, 0.29 T, 0.5 T, and an electromagnet 0.09 T) mounted on a pipe. They found as large as 42% increase in the evaporation at 80 °C during 1 h treatment. Moreover, 24% reduction in the surface tension accompanying the effect of evaporation was measured. The authors have not suggested any mechanism of the observed effects.
Lately Wang et al. [
16] reported changes in the evaporated amounts, a decrease in the specific heat and boiling point of tap water after treatment in the 100–400 mT MF composed of 26 magnets for 5 min and at the water flow rate 0.8 m/s.
Finally, it should be mentioned that Otsuka and Ozeki [
21] claimed that in pure water (distilled in vacuum with no gas dissolved) MF did not change its properties but it did if oxygen or air was dissolved.
The above brief review of the papers dealing with the MF effects on water properties indicates that still there is a lack of coherent view on the mechanisms of effects, although some interesting explanations have already been put forward. To sum up, the papers published on water evaporation are listed in
Table 1. As can be seen some of the experiments are not well specified and it would be difficult to reproduce them. In the light of the above it still seemed interesting for us to carry out more studies on the static MF influence on the evaporation rate of water and its surface tension. We were especially interested whether the experiments conducted in typical environments at room temperature and humidity for several days or even weeks are reproducible and meaningful. If so, such results would have potentially practical importance for faster water evaporation.
4. Discussion
The presented results show clearly that MF causes changes in the structure of water which appears in the changes of the rate of evaporation and surface tension which was already reported in the earlier published papers [
7,
8,
9,
10,
11,
12,
13,
14,
15,
16]. However, some new features of the effects were found. A possible role of dissolved air (oxygen) in the tested water samples should be kept in mind. Both in the case of evaporation and surface tension experiments the water samples contacted with the atmosphere during the deionization (MilliporeQ System) and distillation processes. Otsuka and Ozeki [
21] claimed that in the ultrapure water distilled in a vacuum no MF effects occurred even in the 6 T magnetic field. Moreover, no effects were observed in 10 mM NaCl, KCl, CaCl
2 if no gas was dissolved. However, if water was saturated with oxygen (700 Torr) and in the presence of air atmosphere, the MF effect appeared. The presence of MF effect was evaluated via the contact angle measurements on a platinum surface which after the MF treatment decreased from 65° to 56°, and by 10° on the copper surface. However, there is no doubt that the contact angle is a sufficiently sensitive parameter to study the MF effects. Based on the analysis of changed Raman bonds and electrolytic potential of water, as well as precipitation of aragonite instead of calcite in the magnetized water, the authors [
21] concluded that the observed effects can be assigned to “formation of clathrate-like hydrate of O
2 and promotion of hydrogen bonded network”. As the dispersion interactions between two bodies depend on the magnetic susceptibility of the medium, the interactions between the clusters can be affected by MF in the paramagnetic medium [
21]. Considering this model, the question arises whether it reflected in the increased evaporation of MF-treated water and a small decrease in its surface tension. Both these effects involve changes in the water structure, at least in its surface layer. Water evaporation relates to breaking of the hydrogen bonding, therefore the above model of “promotion of hydrogen bonded network” should lead to reduced water evaporation instead of increased which was stated in many papers [
7,
8,
9,
10,
11,
12,
13,
14,
15,
16]. Nakagawa et al. [
7] found that the evaporation rate increased when the vessel was placed off the field center and concluded that rather the MF field gradient
B·dB/dx is more important than the field itself. They suggested that oxygen (and air) can cause a susceptibility gradient in normal direction to the evaporating water surface and enhance magnetic convection and hence a decrease in the water vapor density. Volume susceptibility at 293 K of oxygen and air amounts to: χ
ox = +1.8028 × 10
−6; χ
air = +0.3736 × 10
−6, while that for nitrogen and water to only −0.0063 × 10
−6, and −0.0068 × 10
−6, respectively.
where
=
. The susceptibility χ indicates how given material behaves in MF (attracts into or repels out), as well as it informs about the material structure, energy and bonding. If χ > 0 (the magnetization is higher than that of empty space) the substance is paramagnetic and if χ < 0 the material is diamagnetic. Magnetic susceptibility is the ratio; χ = M/H, where M is the magnetization (magnetic moment per unit volume) and H is the applied magnetizing field intensity. Both M and H have the same unit A/m and hence the volume susceptibility is a dimensionless quantity. The
μ is the measure of a material ability to support to form magnetic field in its bulk, i.e., the material magnetization due to the applied MF and μ
o is the vacuum permeability, (also called as permeability of free space or permeability of vacuum) which is the magnetic constant. It is a measure of resistance appearing when a MF is formed in classical vacuum. The
µ0 = 4π × 10
−7 H·m
−1 ≈ 12.57 × 10
−7 H·m
−1 [Vs/Am]. The volume magnetic susceptibility χ
v and magnetic permeability μ are related by the formula: μ = μ
o (1 + χ
v), where the term in bracket is the relative permeability of the material and it is dimensionless.
In the MF 8 T and
B·dB/dx = 320 T
2/m at 60 mm from the force center, the bulk magnetic force difference ∆F
m was equal to 2.2 N/m
3 (1.7 N/kg), which is the difference between the force in the wet air near the water surface and the force in the bulk dry air, corresponds to ca. 17% of the gravitational force acting on the air. The authors [
7] compared this force effect to the thermal convection effect if the temperature increased by 50 K from 293 K. Moreover, no MF effect on water vaporization was observed in nitrogen contrary to that in oxygen because χ
ox>> χ
water. They finally concluded that for the magnetic convection to occur a susceptibility gradient must be present perpendicularly to the MF gradient.
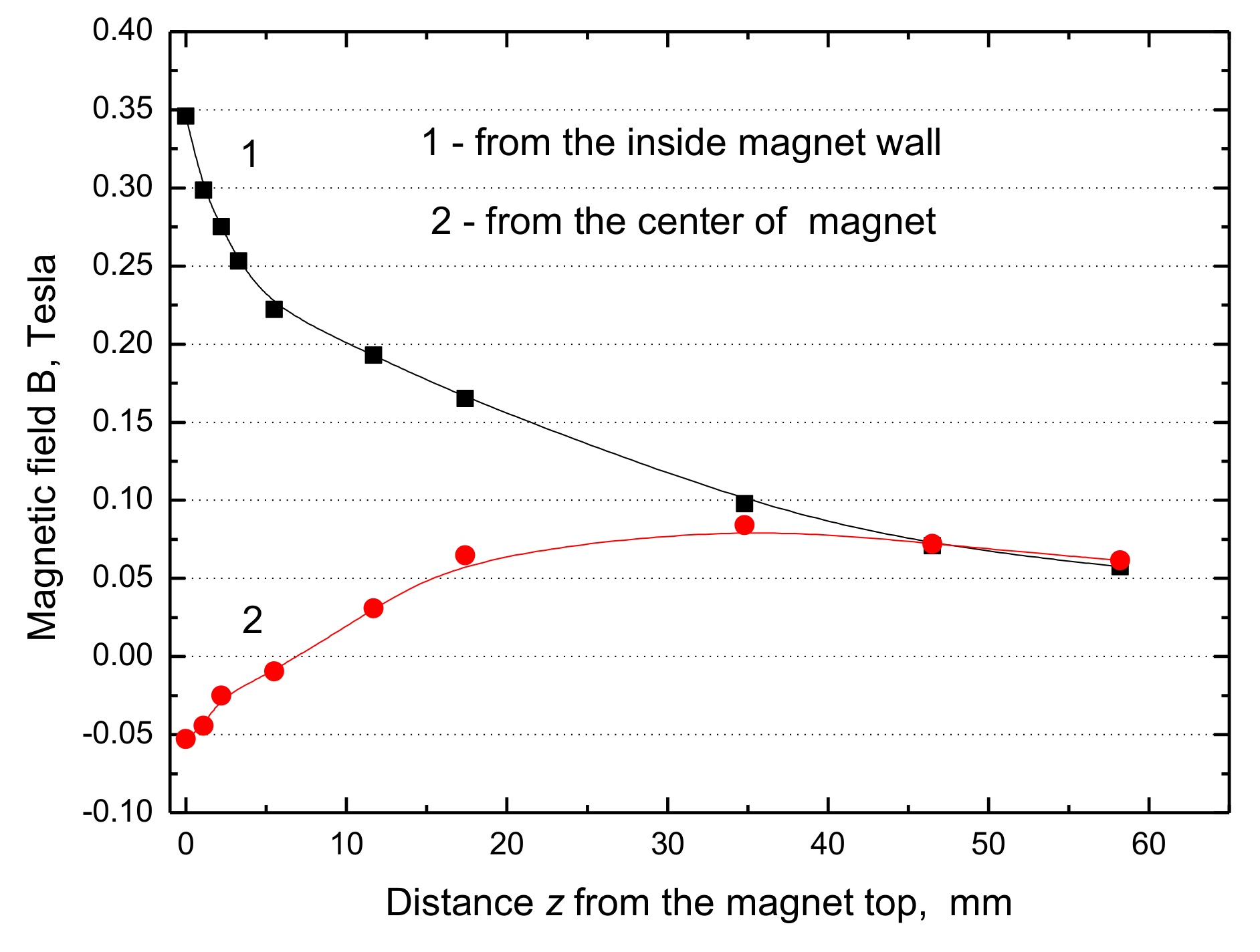
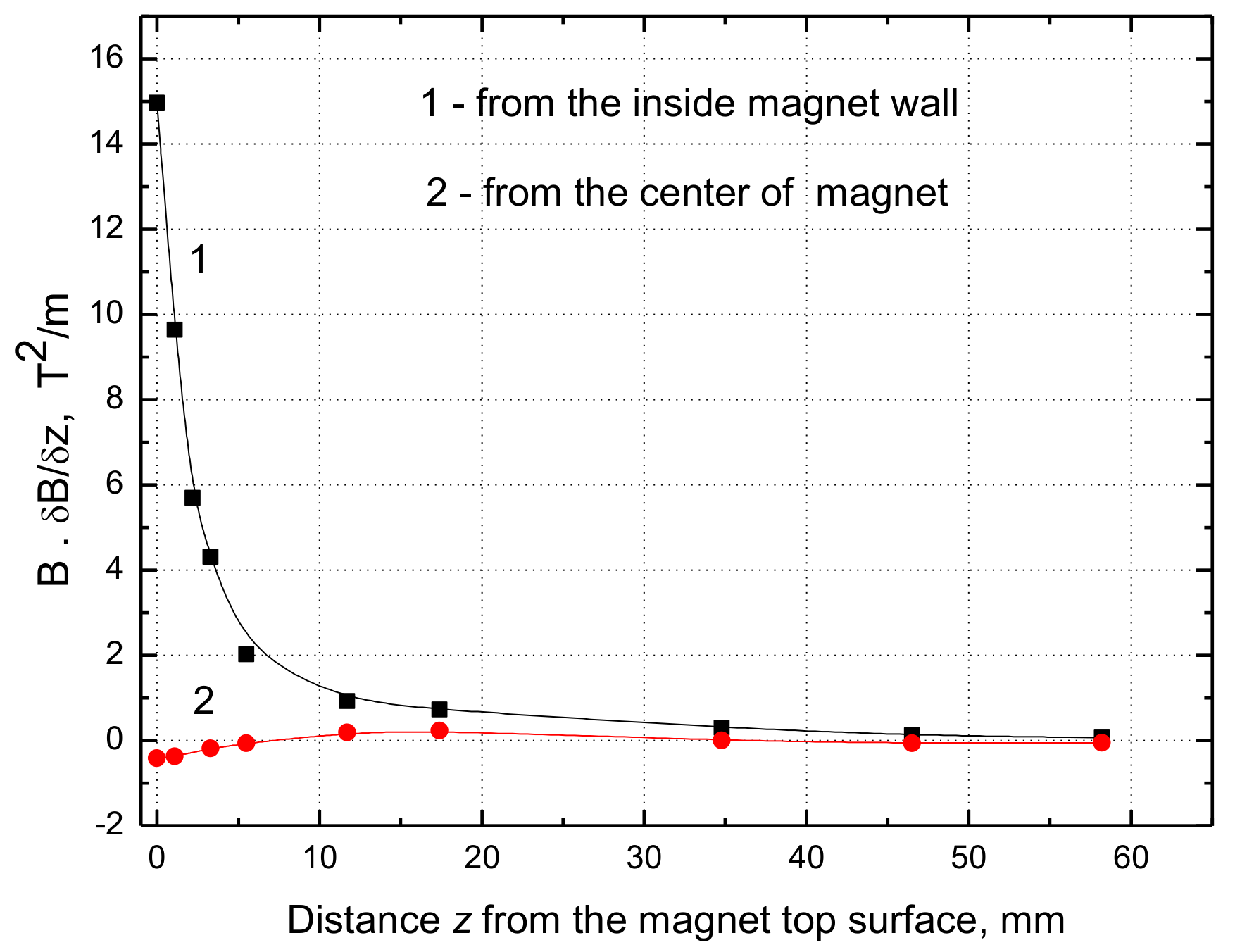
From our results presented in
Figure 8 and
Figure 9 it can be seen that the maximum value of
B·dB/dz = 15 T
2/m at the water surface close to the inner magnet wall and only 0.42 T
2/m at the magnet center. Hence the maximum force difference ∆F
m (Equation (1)) amounts to 0.089 N/kg, which is only 0.91% of the gravitational force and its contribution in the magnetic convection is minimal, whereas it was 17% if the
B·dB/dx = 320 T
2/m [
7]. Thus, the MF enhancement of the water evaporation is situated close to the inner wall of the ring magnet. Therefore, some other reasons must be considered to explain the observed increase in the evaporation rates (
Figure 3,
Figure 4,
Figure 5,
Figure 6 and
Figure 7).
Guo et al. [
12] used a superconducting magnet which allowed generation of high gradient (from −1500 T
2/m to 1313 T
2/m) and simulated gravity (~0 g–~2 g, where g
o = 9.80665 m/s
2). Placing the water sample at different positions the amount of evaporated water was determined at different field strengths, its gradient and simulated gravity. The simulated gravity g
m (simulated acceleration) in a gradient MF is described following [
12]:
where
B′ is the field gradient and ρ is the water density.
They considered four reasons influencing enhancement of water vaporization, i.e., effect of MF itself, combined effect of MF and its gradient, changes in the water surface area due to MF, MF effect on the convection. Moreover, also the effect of MF on the hydrogen bonds was discussed. They showed that at the same gravity the homogeneous MF (16.12 T) increased water evaporation. The largest evaporation was at simulated 0 gravity. However, the samples were extremely small 60 μL and the difference in the evaporated water after 24 h experiment was ca. 0.2 mg (Figure 1 in ref. [
12]). They concluded that the amount of evaporated water, among others, depended on the surface area of water/gas interface which changed at the “non-center position” and depended on three investigated positions of the sample in the magnet (32.08; 34.65; 44.65 in mm
2). An important role of Lorentz force, as well as breaking of hydrogen bonds and weakening of van der Waals interactions [
17] were suggested [
12]. However, because the published MF effects on the hydrogen bonds are contested [
22,
23,
24] they did not discuss them.
Seyfi et al. [
13], who also used ring magnets, the increased evaporation from the MF-treated samples was considered first in the light of kinetic energy of water molecules and Lorentz force which acts on the charged molecules moving at the water/air interface. The water surface was on the same level as the magnet upper height. Having the MF (75 mT) from the north pole directed normally to the water surface, the maximum increase in the evaporated amount during 80 min amounts to ca. 19% at 31 ± 1 °C. However, no effect was observed if the field was parallel to the surface. Similarly to Nakagawa et al. [
7], taking into account the volume magnetic and Lorentz forces, they calculated the difference between the gravity and the volume magnetic force and found that it was too small to increase convection of water molecules that would be caused by the MF applied. To explain the observed increase in the evaporation rate, occurring only in the case of MF perpendicular to the water surface, the authors [
13] put forward the hypothesis that the Lorentz force affects the kinetic energy of the random motion of the dipoles. This is possible because it is believed that across the water/air interface there exists an electrostatic potential resulting from the bouncing dipoles [
19,
20]. As a result, changed momenta can occur in the clusters along the hydrogen bonds. This can result in possible weakening or even breaking the hydrogen bonds and escaping of the freed water molecules. Seyfi et al. [
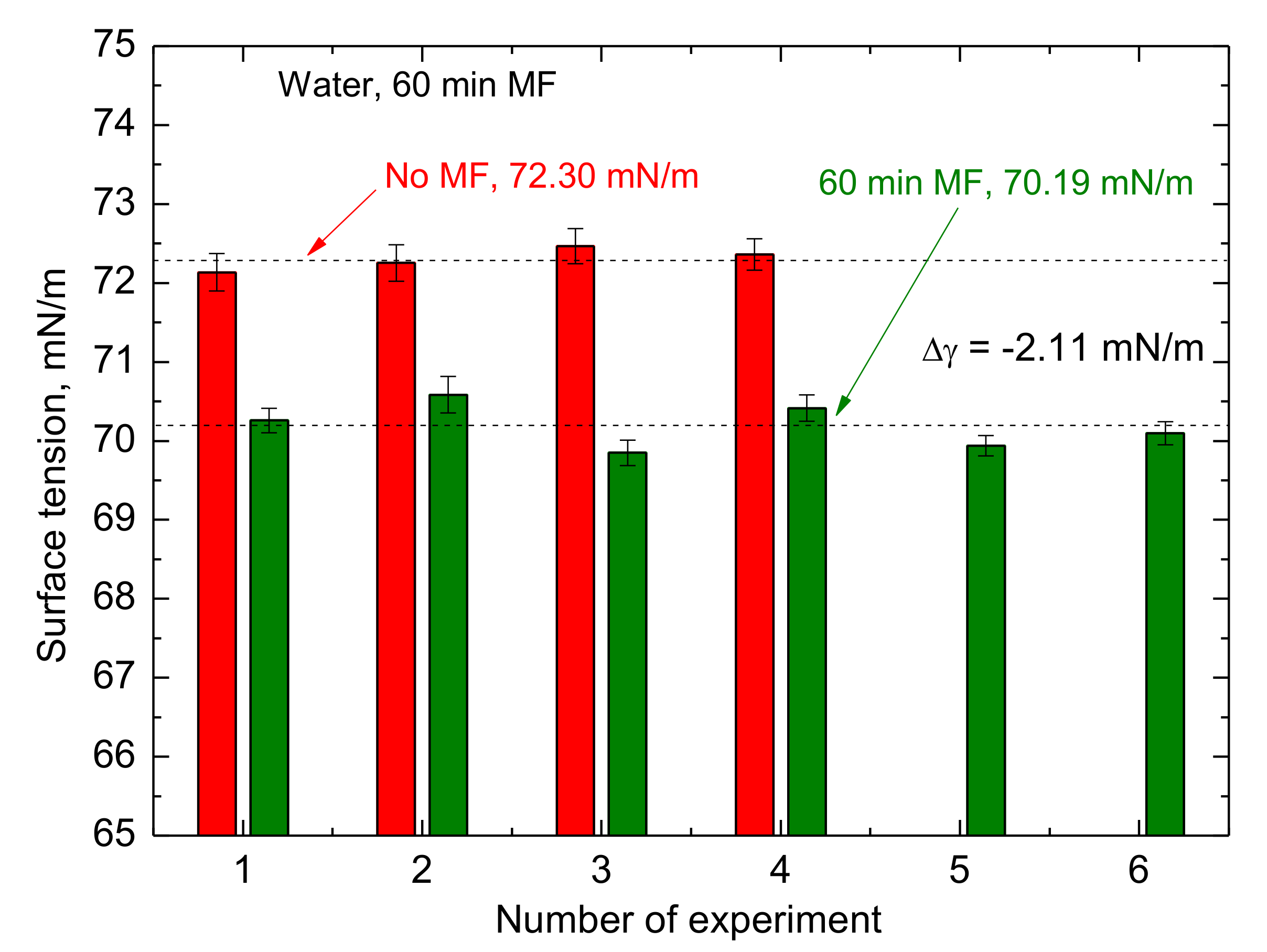
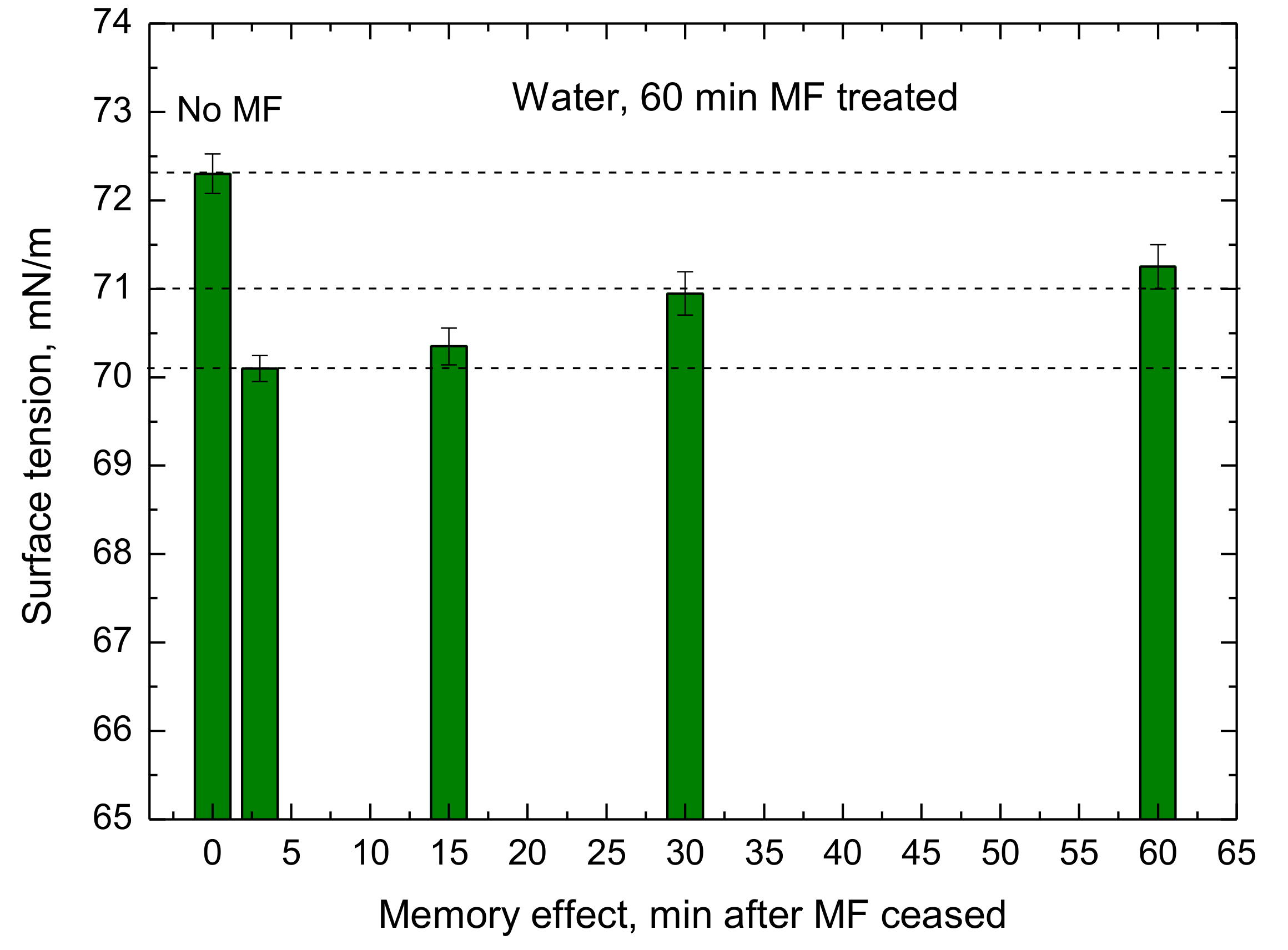
13] also suggested that if the water structure is changed due to the MF action, i.e., more monomer water molecules or weakened water clusters are present for a period of time, this would be the origin of the memory effect observed up to 40 min. We are in favor of this hypothesis because it explains our results, both the increased evaporation rate (
Figure 3,
Figure 4,
Figure 5,
Figure 6 and
Figure 7) and the slightly decreased surface tension (
Figure 10), as well as the memory effect (
Figure 11).
Also, the theoretical simulations of Toledo et al. [
15] help to explain of our results. They applied the GAUSSIAN98 program package and the properties of water were calculated at the Density Functional Theory level using the density functional Becke’s three-parameters exchange functional and the gradient corrected functional [
25,
26]. Different geometries of the water clusters were considered in the simulated apparent MF (up to 17 T). They considered water clusters of up to 15 molecules that had the same charge. This was due to many possible geometries of larger clusters; hence there might be several minima on the shallow potential/energy surface. This would cause difficulties in determination of the true minimum for each cluster. Anyway, using some literature data the authors [
15] calculated the total binding energy of intra- and inter-clusters. However, the energy of inter-clusters is very small in comparison to that of the intra clusters. Thus, calculated value for magnetically untreated water was close to the experimental value (−12.293 and −11.30 kcal/mol, respectively). Moreover, from the calculations it resulted that the clusters of a higher symmetry have lower energy. Although at low MF strengths the changes of the intra cluster binding energy are small but generally the field affects the energy. MF (especially strong one) decreases stability of the clusters consisting of 2, 7, 11, 14 and 15 molecules and stabilizes the clusters with 3, 4, 8 and 12 molecules. Generally, MF can weaken the intra cluster hydrogen bonds thus reducing their average number between water molecules [
15]. Also, Zhou et al. [
22] concluded from the Monte Carlo simulation that the intra clusters hydrogen bonds are weakened by MF, and the second neighbor solvation shell is affected too, causing increased number of the neighbors. Thus, the final suggestion of Toledo et al. [
15] was that MF weakens stronger intra cluster hydrogen bonds; breaks the larger clusters and thus forms smaller clusters which characterize the stronger inter-cluster hydrogen bonds. This can contribute to the paramagnetic term of water. We approve of the conclusions of these simulations because they help to understand the MF effects presented in this paper. Even the observed initial decreased evaporation rate, if the south pole S↑ is up (
Figure 5) can result from the competition between the inter and intra clusters whose relative amounts change during the MF treatment time [
15].