High Strength and High Electrical Conductivity Al Nanocomposites for DC Transmission Cable Applications
Abstract
:1. Introduction
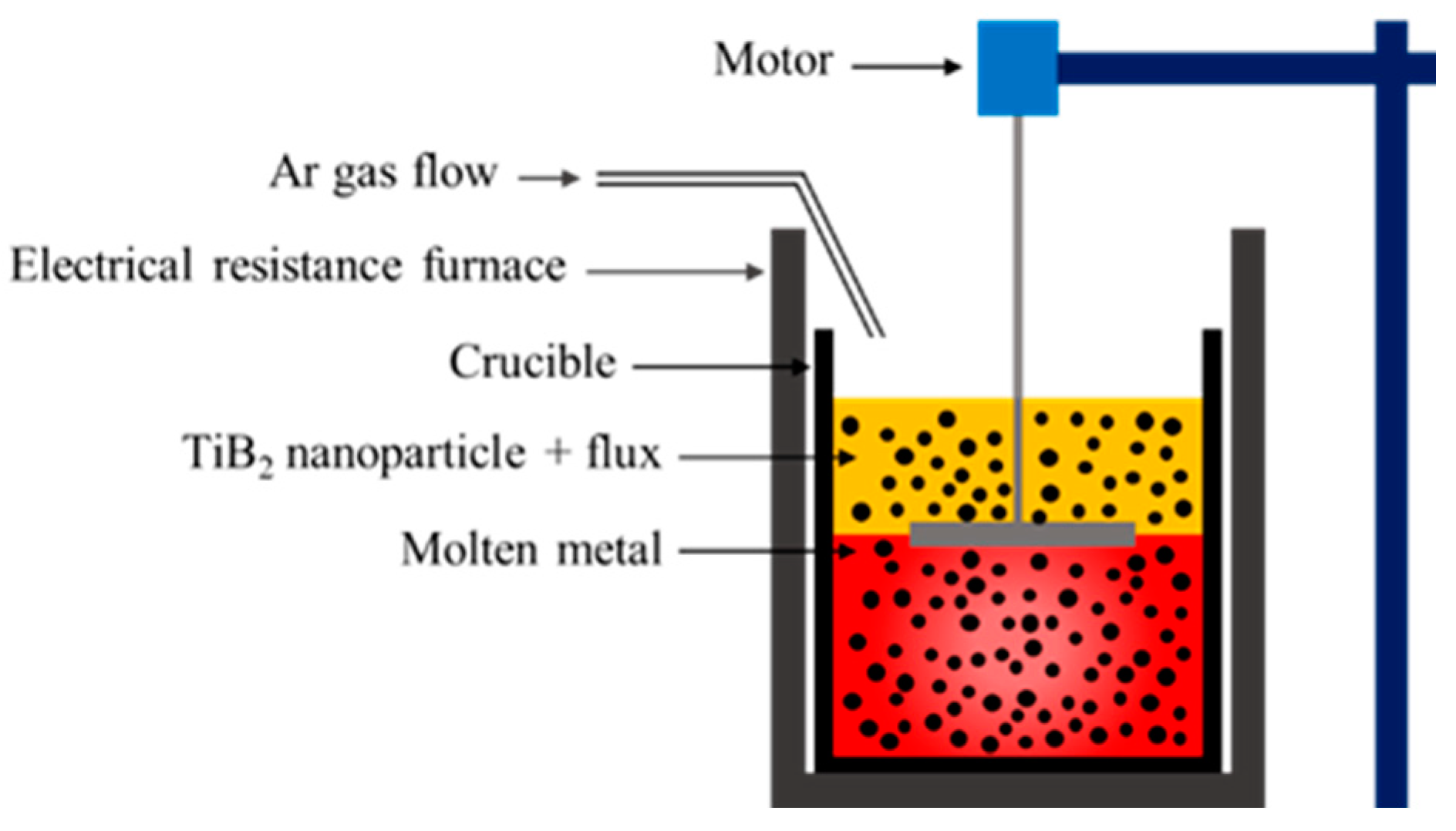
2. Materials and Methods
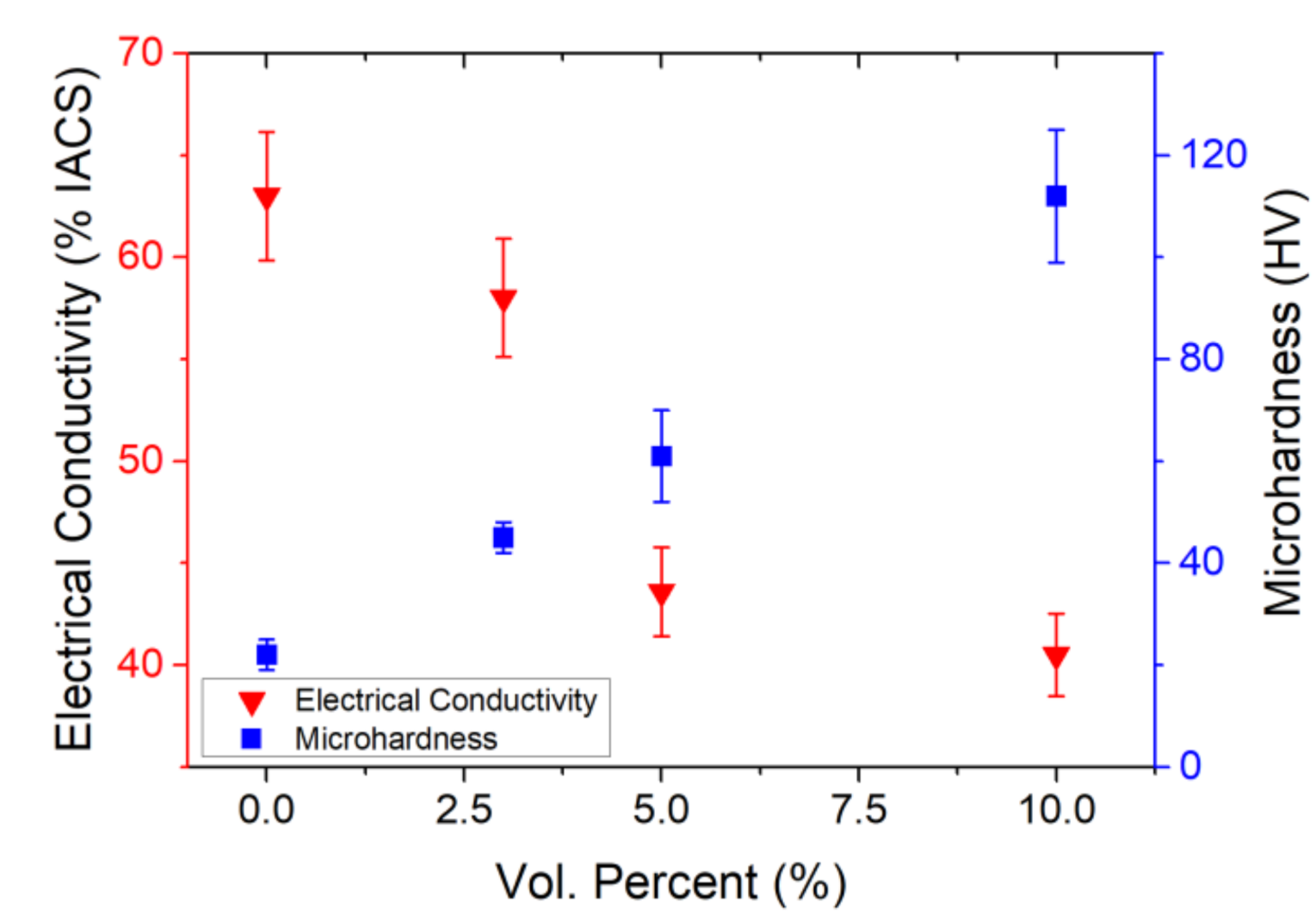
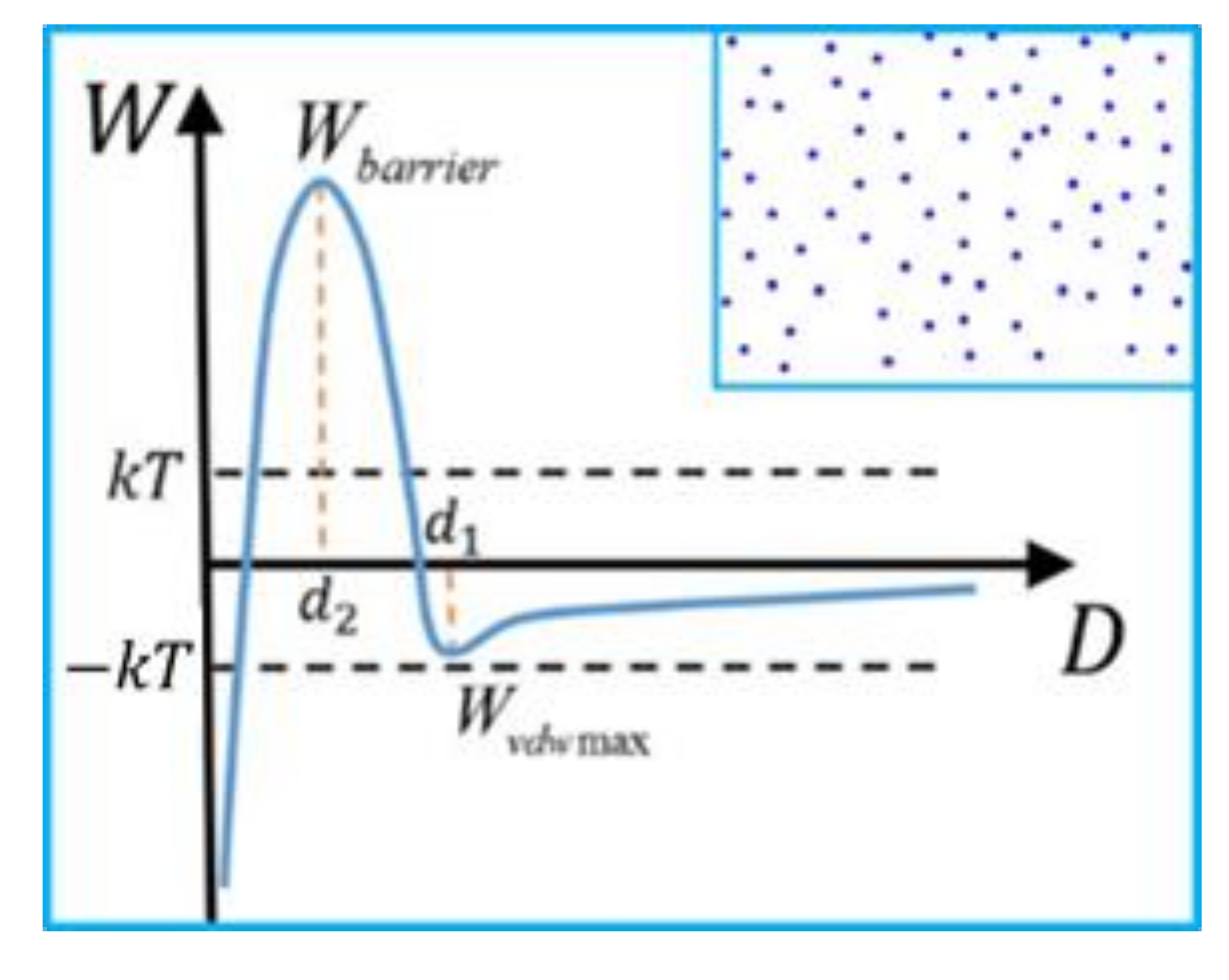
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Karabay, S. Modification of AA-6201 alloy for manufacturing of high conductivity and extra high conductivity wires with property of high tensile stress after artificial aging heat treatment for all-aluminium alloy conductors. Mater. Des. 2006, 27, 821–832. [Google Scholar] [CrossRef]
- Karabay, S. Influence of AlB2 compound on elimination of incoherent precipitation in artificial aging of wires drawn from redraw rod extruded from billets cast of alloy AA-6101 by vertical direct chill casting. Mater. Des. 2008, 29, 1364–1375. [Google Scholar] [CrossRef]
- Sauvage, X.; Bobruk, E.V.; Murashkin, M.Y.; Nasedkina, Y.; Enikeev, N.A.; Valiev, R.Z. Optimization of electrical conductivity and strength combination by structure design at the nanoscale in Al–Mg–Si alloys. Acta Mater. 2015, 98, 355–366. [Google Scholar] [CrossRef]
- Valiev, R.Z.; Murashkin, M.Y.; Sabirov, I. A nanostructural design to produce high-strength Al alloys with enhanced electrical conductivity. Scr. Mater. 2014, 76, 13–16. [Google Scholar] [CrossRef]
- Tian, L.; Anderson, I.; Riedemann, T.; Russell, A.; Kim, H. Prospects for novel deformation processed Al/Ca composite conductors for overhead high voltage direct current (HVDC) power transmission. Electr. Power Syst. Res. 2013, 105, 105–114. [Google Scholar] [CrossRef]
- Hou, J.P.; Wang, Q.; Yang, H.J.; Wu, X.M.; Li, C.H.; Li, X.W.; Zhang, Z.F. Microstructure evolution and strengthening mechanisms of cold-drawn commercially pure aluminum wire. Mater. Sci. Eng. A 2015, 639, 103–106. [Google Scholar] [CrossRef]
- Rhee, H.; Whittington, W.R.; Oppedal, A.L.; Sherif, A.R.; King, R.L.; Kim, H.J.; Lee, C. Mechanical properties of novel aluminum metal matrix metallic composites: Application to overhead conductors. Mater. Des. 2015, 88, 16–21. [Google Scholar] [CrossRef]
- How Much Electricity Is Lost in Transmission and Distribution in the United States? Available online: https://www.eia.gov/tools/faqs/faq.php?id=105&t=3 (accessed on 4 November 2017).
- Cabibbo, M. Microstructure strengthening mechanisms in different equal channel angular pressed aluminum alloys. Mater. Sci. Eng. A 2013, 560, 413–432. [Google Scholar] [CrossRef]
- Ding, S.X.; Lee, W.T.; Chang, C.P.; Chang, L.W.; Kao, P.W. Improvement of strength of magnesium alloy processed by equal channel angular extrusion. Scr. Mater. 2008, 59, 1006–1009. [Google Scholar] [CrossRef]
- Kim, W.J.; Wang, J.Y. Microstructure of the post-ECAP aging processed 6061 Al alloys. Mater. Sci. Eng. A 2007, 464, 23–27. [Google Scholar] [CrossRef]
- Chinh, N.Q.; Gubicza, J.; Langdon, T.G. Characteristics of face-centered cubic metals processed by equal-channel angular pressing. J. Mater. Sci. 2007, 42, 1594–1605. [Google Scholar] [CrossRef] [Green Version]
- Ito, Y.; Horita, Z. Microstructural evolution in pure aluminum processed by high-pressure torsion. Mater. Sci. Eng. A 2009, 503, 32–36. [Google Scholar] [CrossRef]
- Zhilyaev, A.P.; Langdon, T.G. Using high-pressure torsion for metal processing: Fundamentals and applications. Prog. Mater. Sci. 2008, 53, 893–979. [Google Scholar] [CrossRef]
- Sakai, G.; Horita, Z.; Langdon, T.G. Grain refinement and superplasticity in an aluminum alloy processed by high-pressure torsion. Mater. Sci. Eng. A 2005, 393, 344–351. [Google Scholar] [CrossRef]
- Kamikawa, N.; Huang, X.; Tsuji, N.; Hansen, N. Strengthening mechanisms in nanostructured high-purity aluminium deformed to high strain and annealed. Acta Mater. 2009, 57, 4198–4208. [Google Scholar] [CrossRef]
- Russell, A.M.; Chumbley, L.S.; Tian, Y. Deformation processed metal–metal composites. Adv. Eng. Mater. 2000, 2, 11–22. [Google Scholar] [CrossRef]
- Saito, Y.; Utsunomiya, H.; Tsuji, N.; Sakai, T. Novel ultra-high straining process for bulk materials—development of the accumulative roll-bonding (ARB) process. Acta Mater. 1999, 47, 579–583. [Google Scholar] [CrossRef]
- Pan, S.; Yao, G.; Yuan, J.; Sokoluk, M.; Li, X. Manufacturing of Bulk Al-12Zn-3.7 Mg-1Cu Alloy with TiC Nanoparticles. Procedia Manuf. 2020, 48, 325–331. [Google Scholar] [CrossRef]
- Yuan, J.; Zuo, M.; Sokoluk, M.; Yao, G.; Pan, S.; Li, X. Nanotreating High-Zinc Al–Zn–Mg–Cu Alloy by TiC Nanoparticles. In Light Metals; Springer: Cham, Switzerland, 2020; pp. 318–323. [Google Scholar]
- Fribourg, G.; Bréchet, Y.; Deschamps, A.; Simar, A. Microstructure-based modelling of isotropic and kinematic strain hardening in a precipitation-hardened aluminium alloy. Acta Mater. 2011, 59, 3621–3635. [Google Scholar] [CrossRef]
- Gupta, A.K.; Lloyd, D.J.; Court, S.A. Precipitation hardening in Al–Mg–Si alloys with and without excess Si. Mater. Sci. Eng. A 2001, 316, 11–17. [Google Scholar] [CrossRef]
- Carlton, C.E.; Ferreira, P.J. What is behind the inverse Hall–Petch effect in nanocrystalline materials? Acta Mater. 2007, 55, 3749–3756. [Google Scholar] [CrossRef]
- Pan, S.; Sokoluk, M.; Cao, C.; Guan, Z.; Li, X. Facile fabrication and enhanced properties of Cu-40 wt% Zn/WC nanocomposite. J. Alloys Compd. 2019, 784, 237–243. [Google Scholar] [CrossRef]
- Seidman, D.N.; Marquis, E.A.; Dunand, D.C. Precipitation strengthening at ambient and elevated temperatures of heat-treatable Al (Sc) alloys. Acta Mater. 2002, 50, 4021–4035. [Google Scholar] [CrossRef]
- Thieme, C.L.H.; Pourrahimi, S.; Foner, S. High strength Al metal-matrix microcomposite wire with 20 vol% Nb and ultimate tensile strengths up to 1030 MPa. Scr. Metall. Mater. 1993, 28, 913–918. [Google Scholar] [CrossRef]
- Russell, A.M.; Lund, T.; Chumbley, L.S.; Laabs, F.A.; Keehner, L.L.; Harringa, J.L. A high-strength, high-conductivity Al–Ti deformation processed metal metal matrix composite. Compos. Part A Appl. Sci. Manuf. 1999, 30, 239–247. [Google Scholar] [CrossRef]
- Xu, K.; Russell, A.M.; Chumbley, L.S.; Laabs, F.C.; Gantovnik, V.B.; Tian, Y. Characterization of strength and microstructure in deformation processed Al-Mg composites. J. Mater. Sci. 1999, 34, 5955–5959. [Google Scholar] [CrossRef]
- Hernandez, O.; Gonzalez, G. Microstructural and mechanical behavior of highly deformed Al–Sn alloys. Mater. Charact. 2008, 59, 534–541. [Google Scholar] [CrossRef]
- Chen, L.Y.; Xu, J.Q.; Choi, H.; Pozuelo, M.; Ma, X.; Bhowmick, S.; Yang, J.M.; Mathaudhu, S.; Li, X.C. Processing and properties of magnesium containing a dense uniform dispersion of nanoparticles. Nature 2015, 528, 539–543. [Google Scholar] [CrossRef] [PubMed]
- Pan, S.; Yuan, J.; Zheng, T.; She, Z.; Li, X. Interfacial thermal conductance of in situ aluminum-matrix nanocomposites. J. Mater. Sci. 2021, 56, 13646–13658. [Google Scholar] [CrossRef]
- Pan, S.; Guan, Z.; Li, X. Unusual thermal performance in Cu-60Ag by WC nanoparticles. Mater. Sci. Eng. B 2021, 265, 115010. [Google Scholar] [CrossRef]
- Yuan, J.; Yao, G.; Pan, S.; Murali, N.; Li, X. Size Control of In Situ Synthesized TiB 2 Particles in Molten Aluminum. Metall. Mater. Trans. A 2021, 52, 2657–2666. [Google Scholar] [CrossRef]
- Drude, P. The Theory of Optics; Courier Dover Publications: New York, NY, USA, 1901. [Google Scholar]
- Gao, W.; Sammes, N.M. An Introduction to Electronic and Ionic Materials; World Scientific Publishin Co. Inc.: Singapore, 1999. [Google Scholar]
- Andrews, P.V.; West, M.B.; Robeson, C.R. The effect of grain boundaries on the electrical resistivity of polycrystalline copper and aluminium. Philos. Mag. 1969, 19, 887–898. [Google Scholar] [CrossRef]
- Miyajima, Y.; Komatsu, S.Y.; Mitsuhara, M.; Hata, S.; Nakashima, H.; Tsuji, N. Change in electrical resistivity of commercial purity aluminium severely plastic deformed. Philos. Mag. 2010, 90, 4475–4488. [Google Scholar] [CrossRef]
- Friedel, J. On some electrical and magnetic properties of metallic solid solutions. Can. J. Phys. 1956, 34, 1190–1211. [Google Scholar] [CrossRef]
- Nordheim, V. Zur theorie der Elektronen in Metallen. Ann. Phys. 1931, 5, 642. [Google Scholar]
- Kittel, C.; Kroemer, H. Thermal Physics, 2nd ed.; AAPT: Sydney, Australia, 1998. [Google Scholar]
- Pan, S.; Yuan, J.; Zhang, P.; Sokoluk, M.; Yao, G.; Li, X. Effect of electron concentration on electrical conductivity in in situ Al-TiB2 nanocomposites. Appl. Phys. Lett. 2020, 116, 014102. [Google Scholar] [CrossRef]
- Pan, S.; Guan, Z.; Yao, G.; Cao, C.; Li, X. Study on electrical behaviour of copper and its alloys containing dispersed nanoparticles. Curr. Appl. Phys. 2019, 19, 452–457. [Google Scholar] [CrossRef]
- Pan, S.; Yao, G.; Yuan, J.; Li, X. Electrical Performance of Bulk Al–ZrB 2 Nanocomposites from 2 K to 300 K. In Nanocomposites VI: Nanoscience and Nanotechnology in Advanced Composites; Springer: Cham, Switzerland, 2019; pp. 63–70. [Google Scholar]
- Israelachvili, J.N. Intermolecular and Surface Forces; Academic Press: Cambridge, MA, USA, 2011. [Google Scholar]
- Hamaker, H. The London—van der Waals attraction between spherical particles. Physica 1937, 4, 1058–1072. [Google Scholar] [CrossRef]
- Rhee, S.K. Wetting of AlN and TiC by liquid Ag and liquid Cu. J. Am. Ceram. Soc. 1970, 53, 639–641. [Google Scholar] [CrossRef]
- Cook, B.A.; Russell, A.M.; Peters, J.; Harringa, J.L. Estimation of surface energy and bonding between AlMgB14 and TiB2. J. Phys. Chem. Solids 2010, 71, 824–826. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Javadi, A.; Pan, S.; Cao, C.; Li, X. High Strength and High Electrical Conductivity Al Nanocomposites for DC Transmission Cable Applications. J. Compos. Sci. 2021, 5, 172. https://doi.org/10.3390/jcs5070172
Javadi A, Pan S, Cao C, Li X. High Strength and High Electrical Conductivity Al Nanocomposites for DC Transmission Cable Applications. Journal of Composites Science. 2021; 5(7):172. https://doi.org/10.3390/jcs5070172
Chicago/Turabian StyleJavadi, Abdolreza, Shuaihang Pan, Chezheng Cao, and Xiaochun Li. 2021. "High Strength and High Electrical Conductivity Al Nanocomposites for DC Transmission Cable Applications" Journal of Composites Science 5, no. 7: 172. https://doi.org/10.3390/jcs5070172
APA StyleJavadi, A., Pan, S., Cao, C., & Li, X. (2021). High Strength and High Electrical Conductivity Al Nanocomposites for DC Transmission Cable Applications. Journal of Composites Science, 5(7), 172. https://doi.org/10.3390/jcs5070172






