Integrated CAD/CAM Approach for Parametric Design and High-Precision Microfabrication of Planar Functional Structures Comprising Radially Oriented V-Grooves
Abstract
1. Introduction
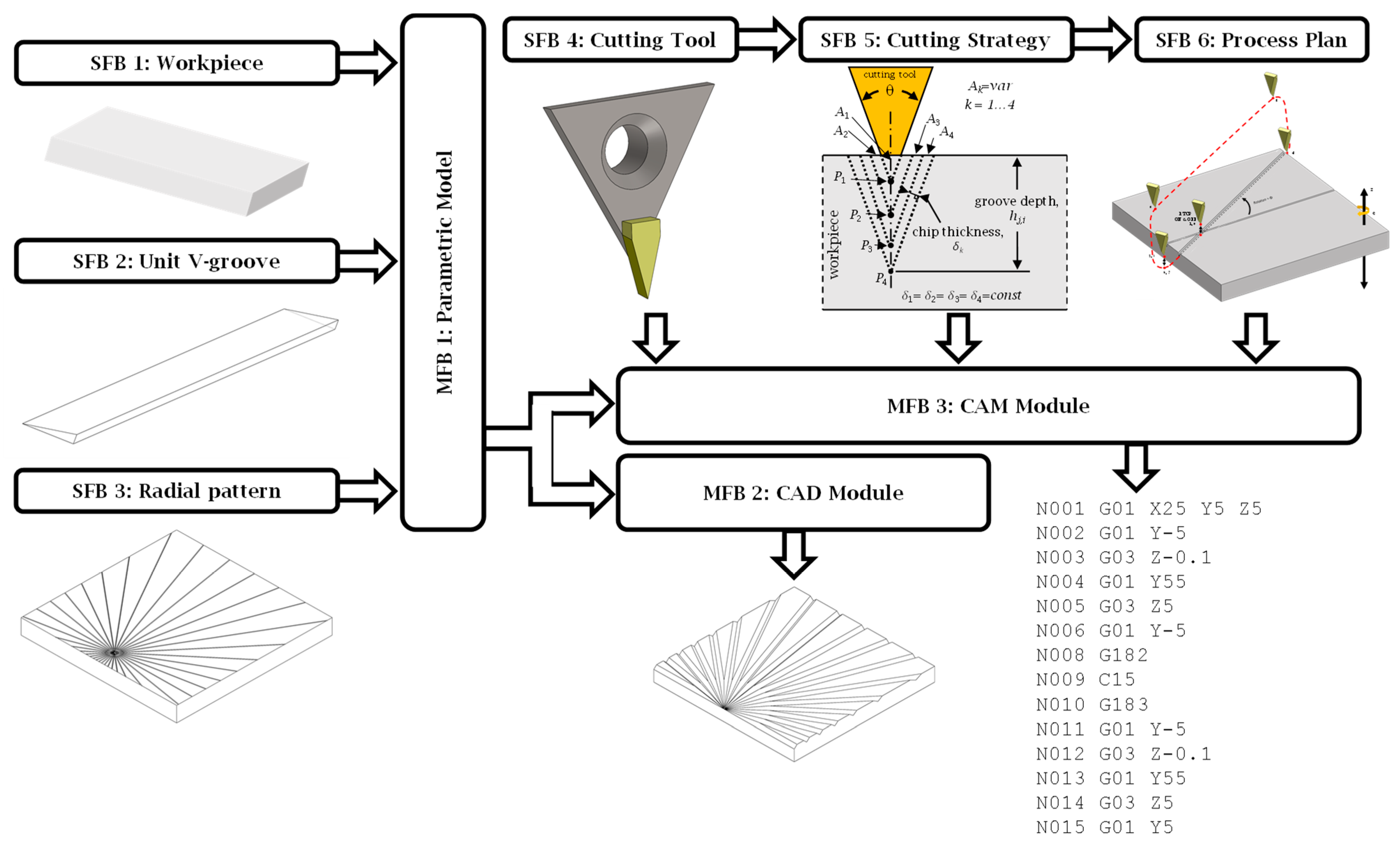
2. Integrated CAD/CAM Approach
3. Parametric Model
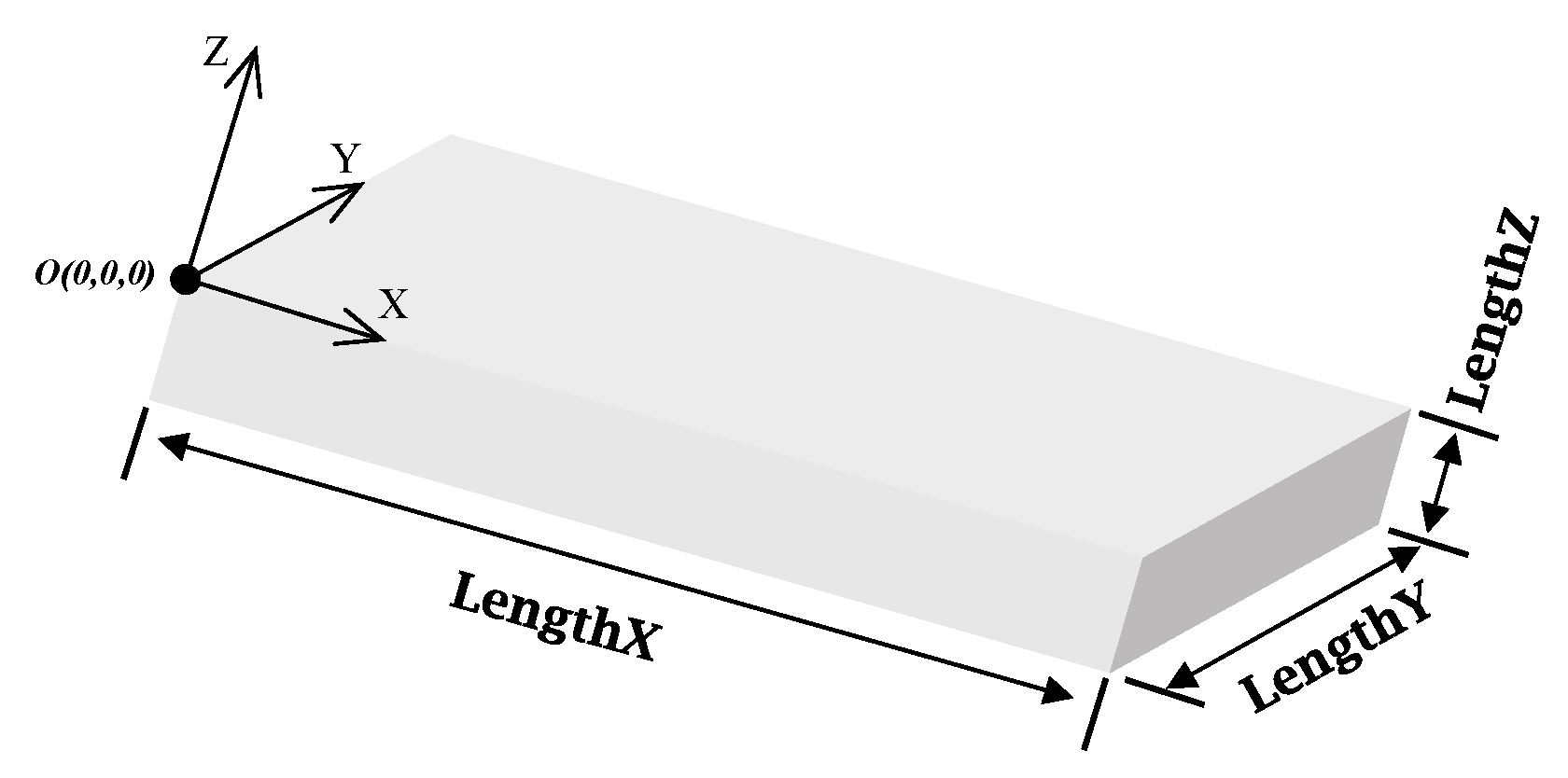
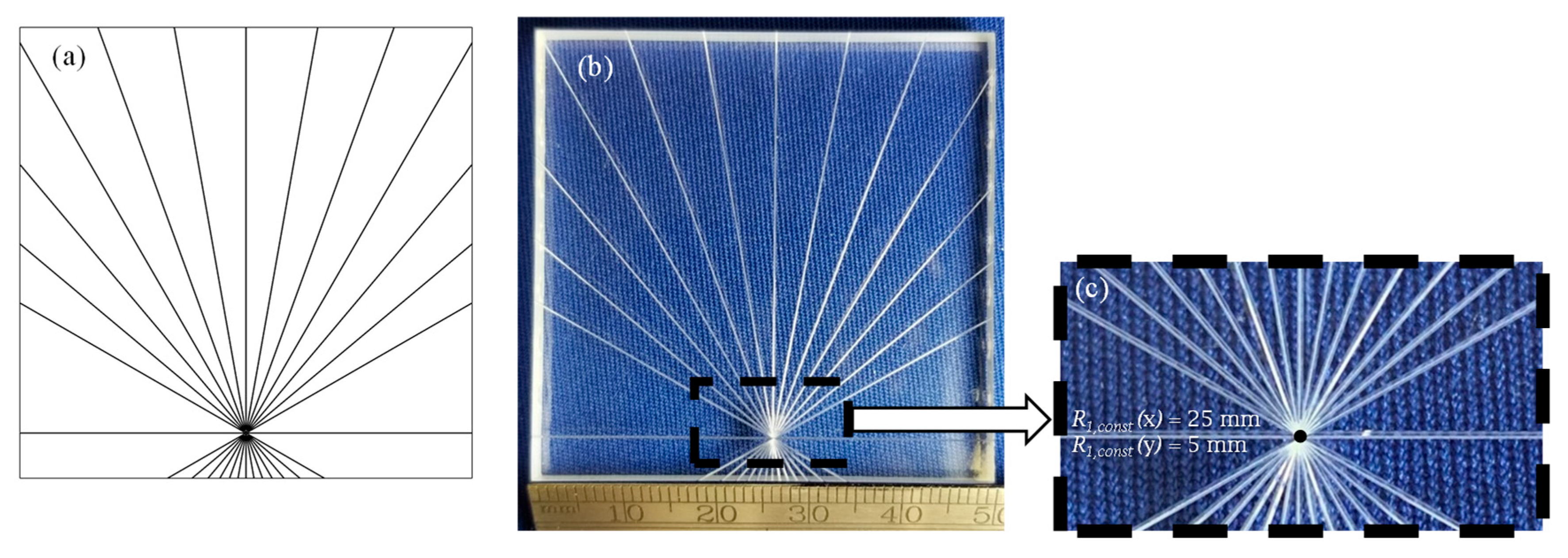
3.1. Workpiece Geometry
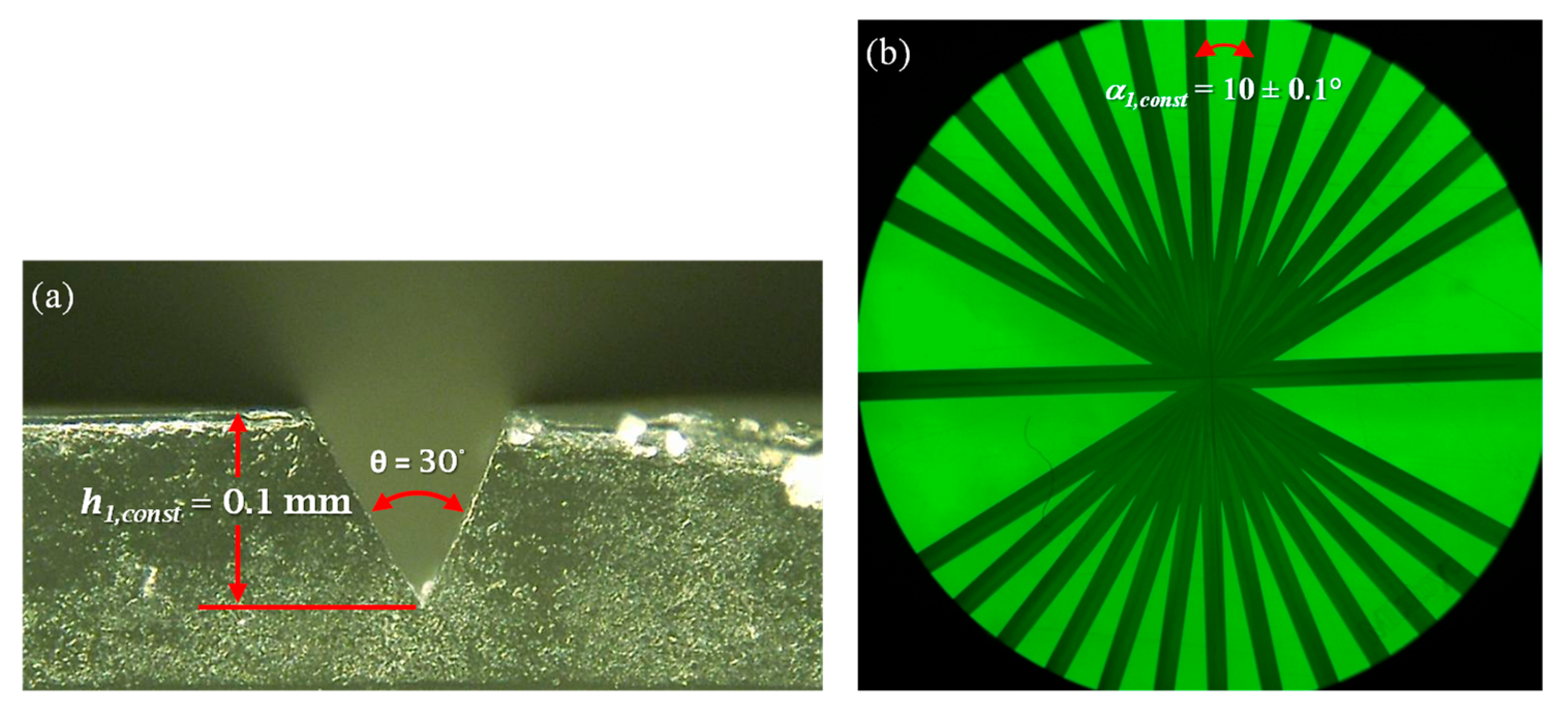
3.2. Unit V-Groove Geometry
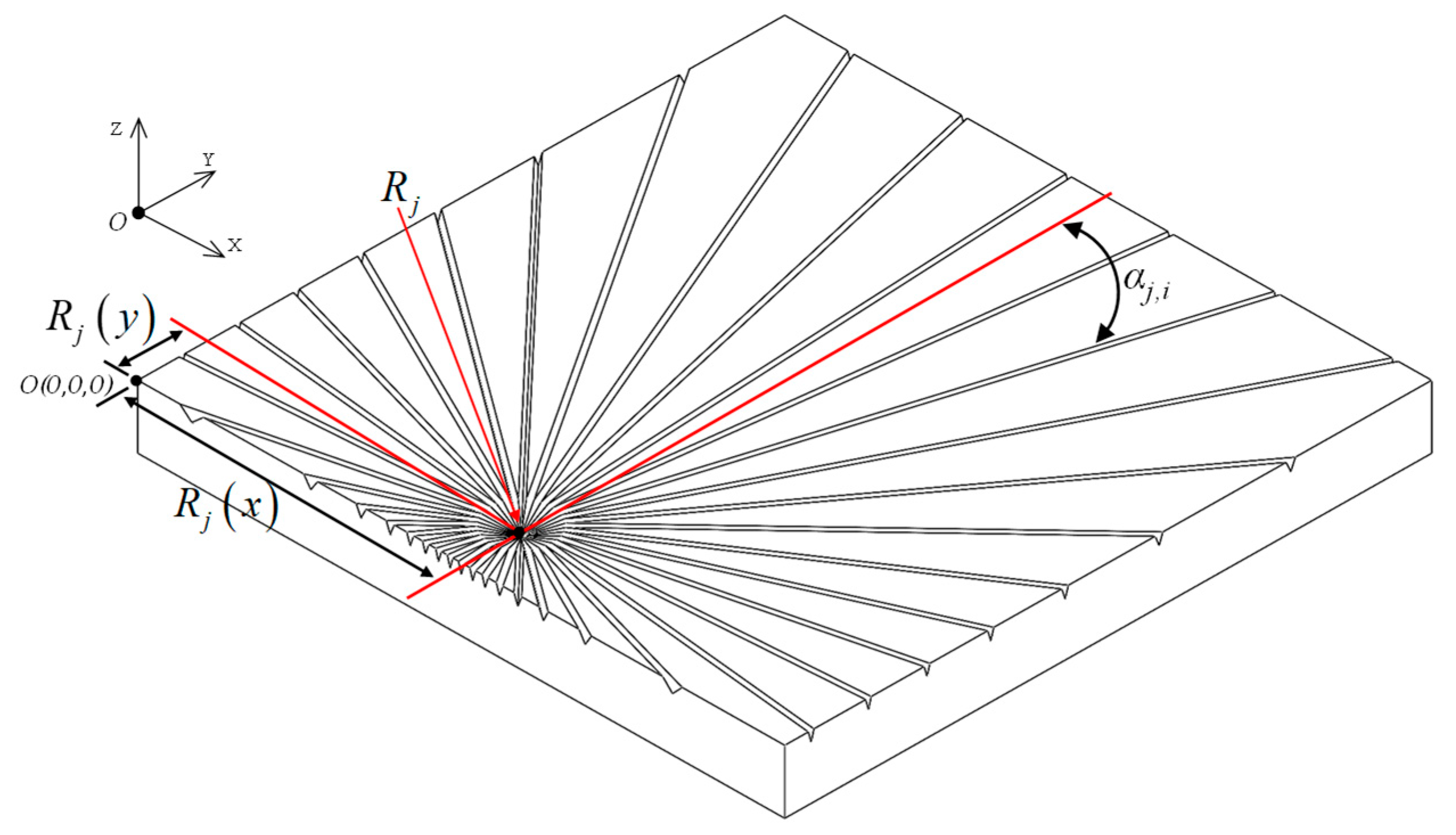
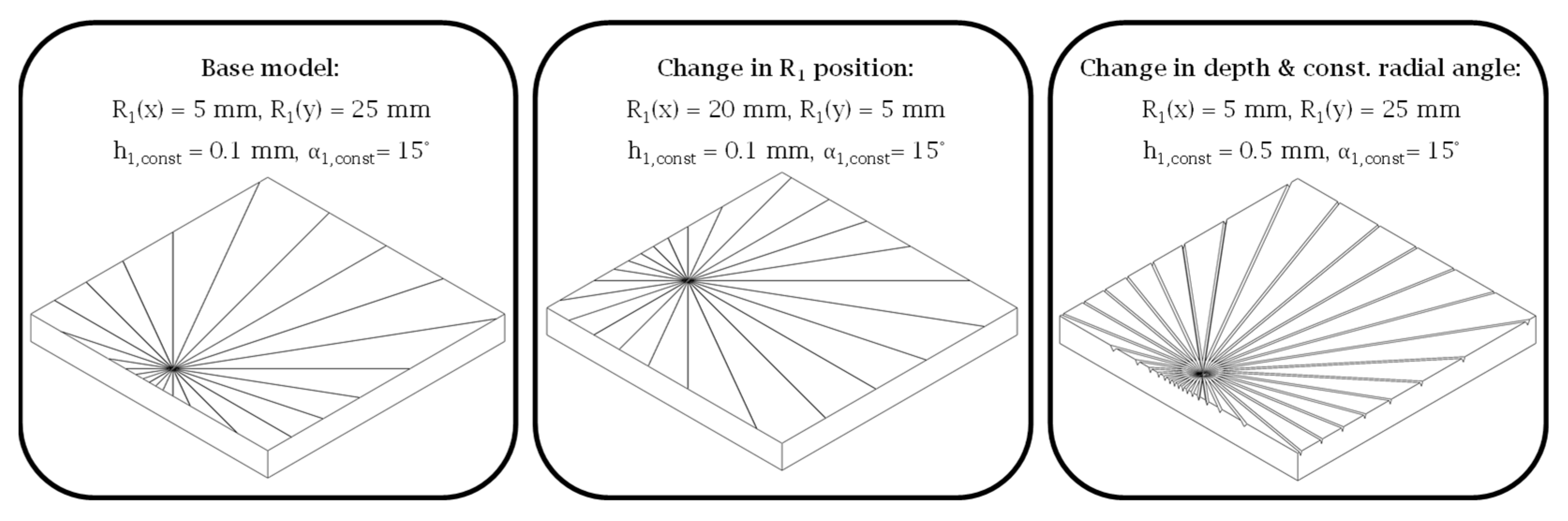
3.3. Radial Pattern
4. CAD Module
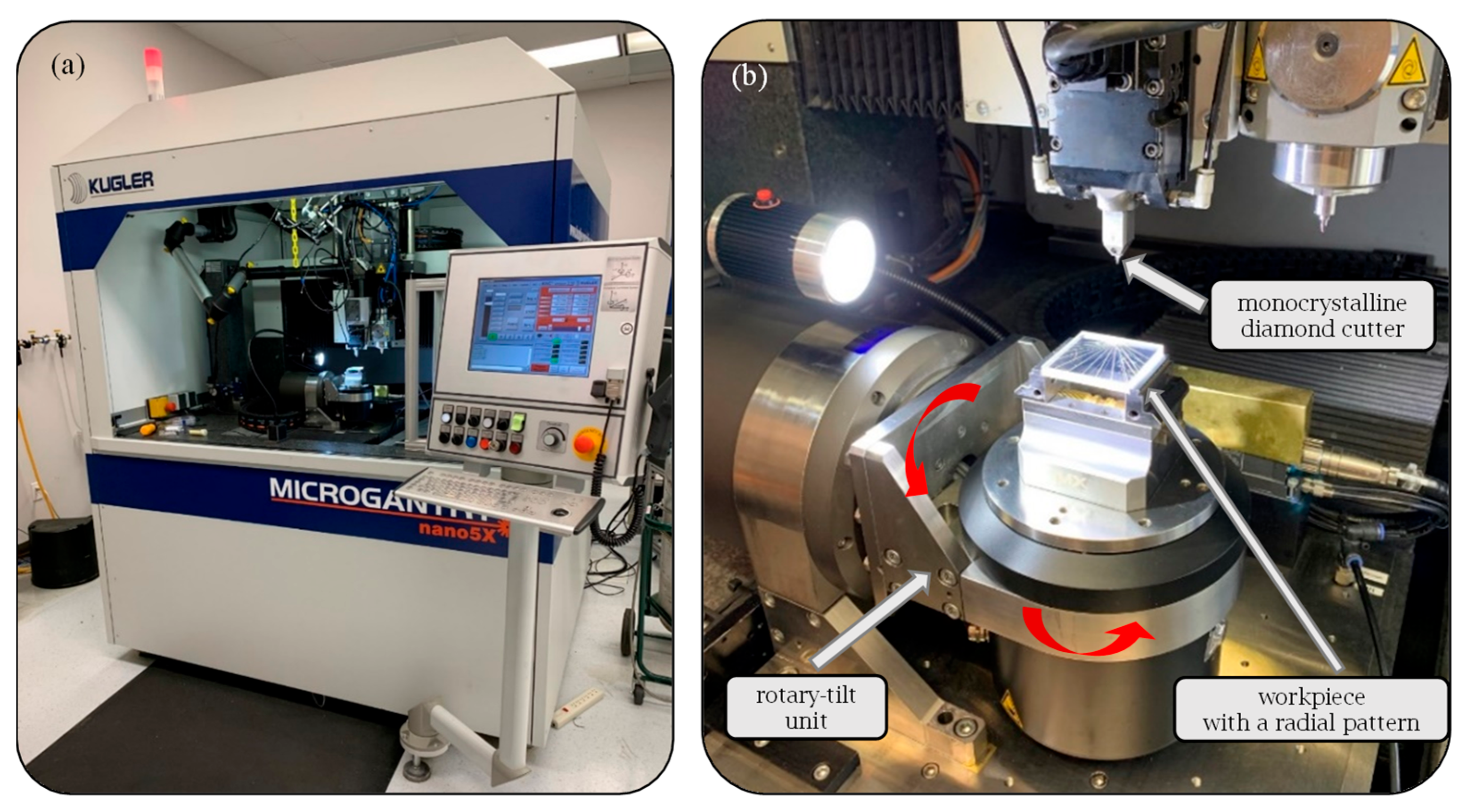
5. CAM Module
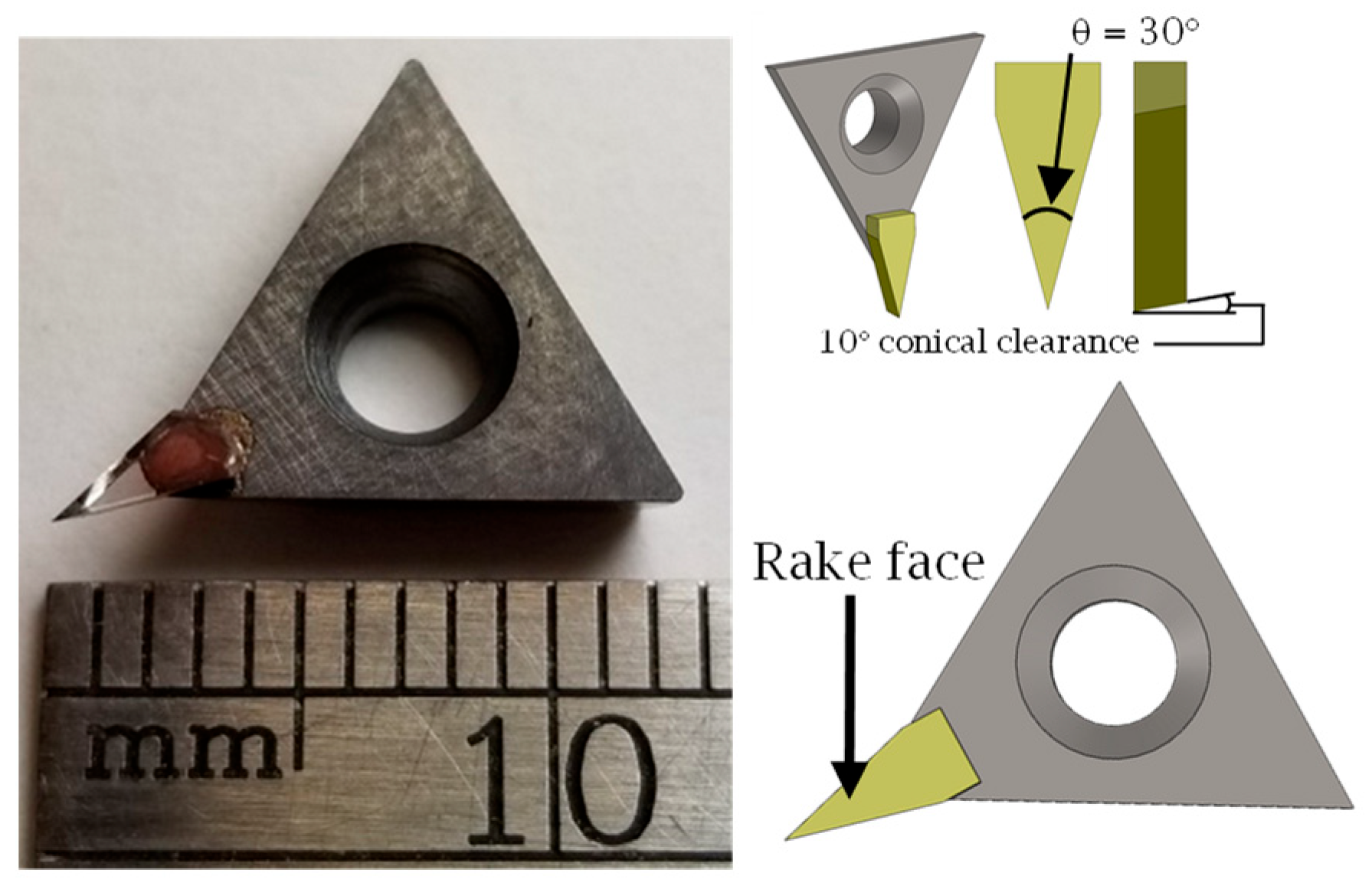
5.1. Tool Geometry
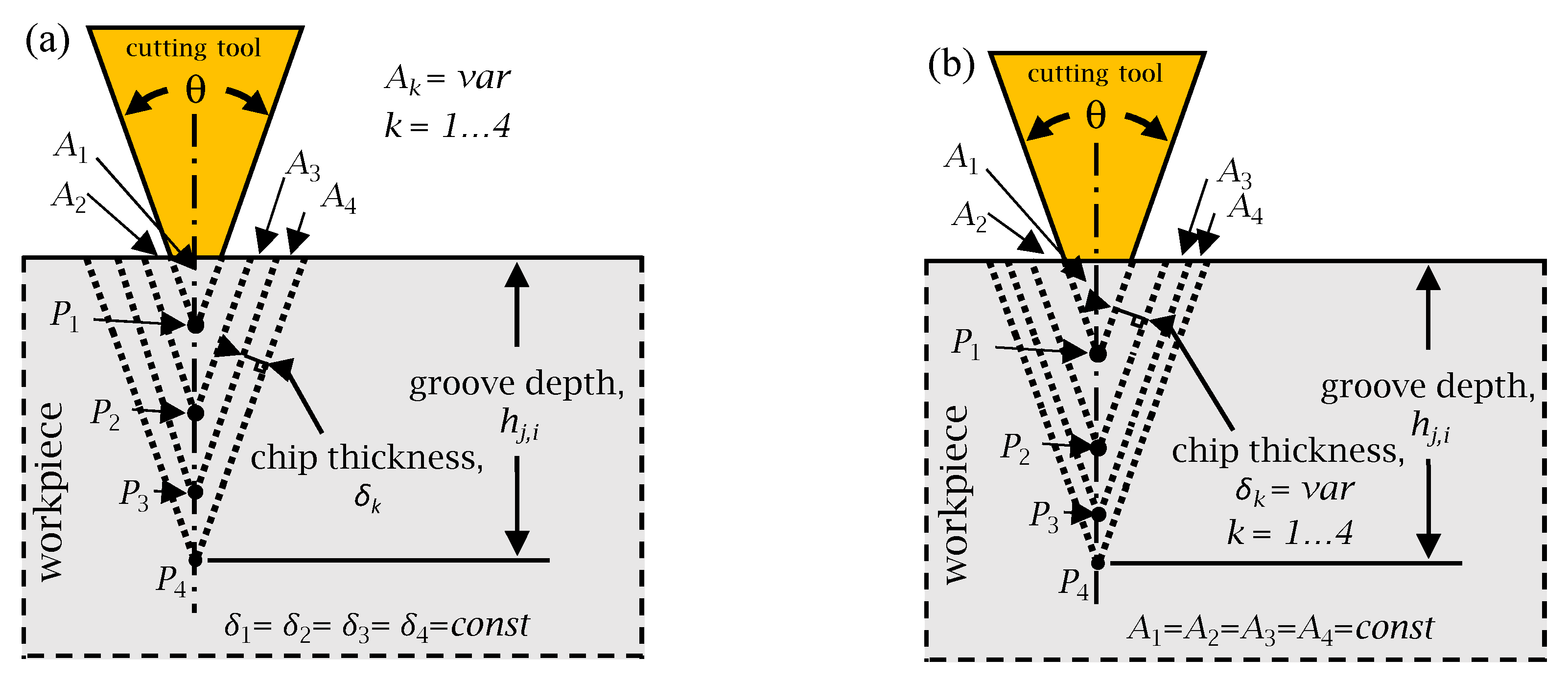
5.2. Cutting Strategy
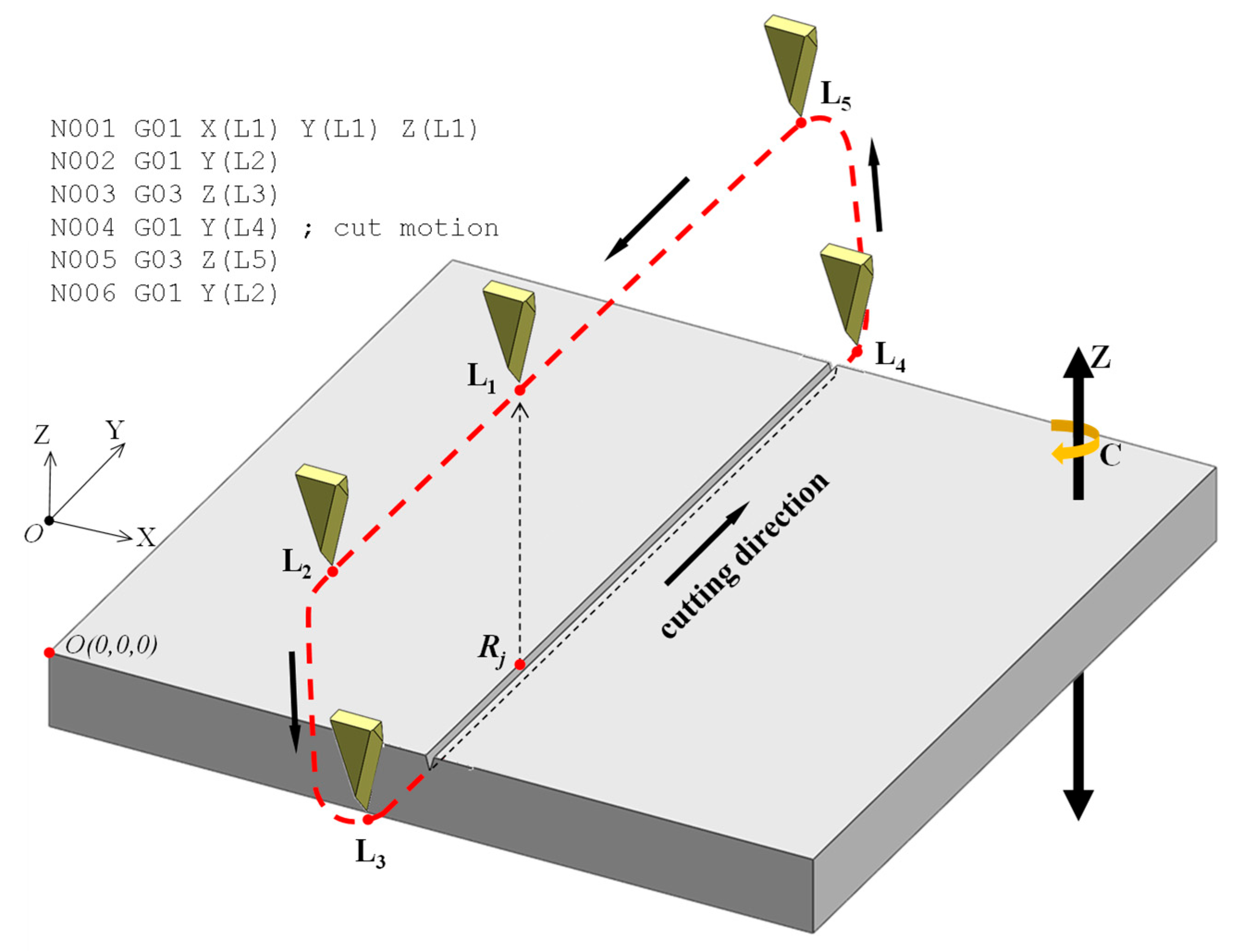
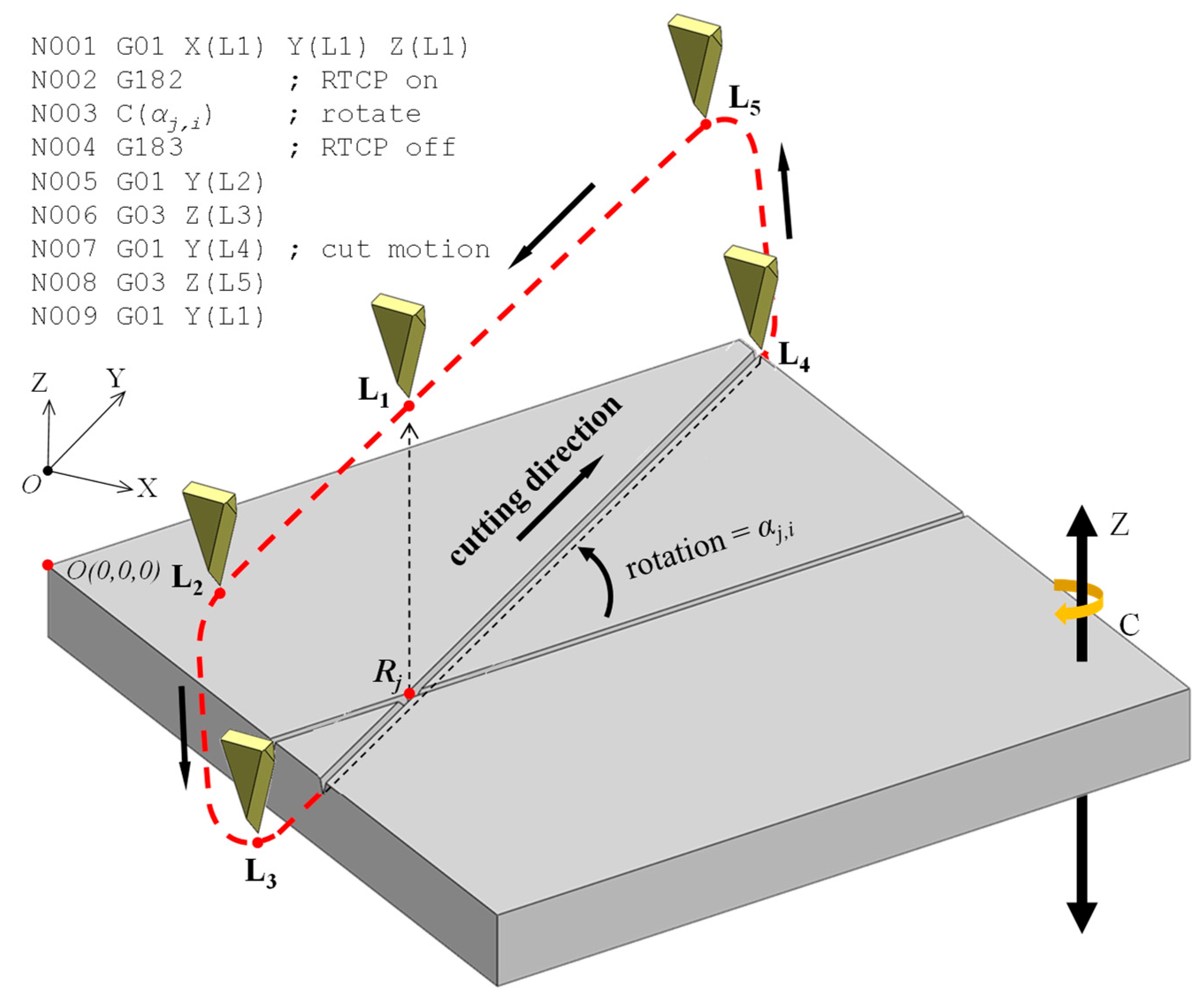
5.3. Cutting Process Plan
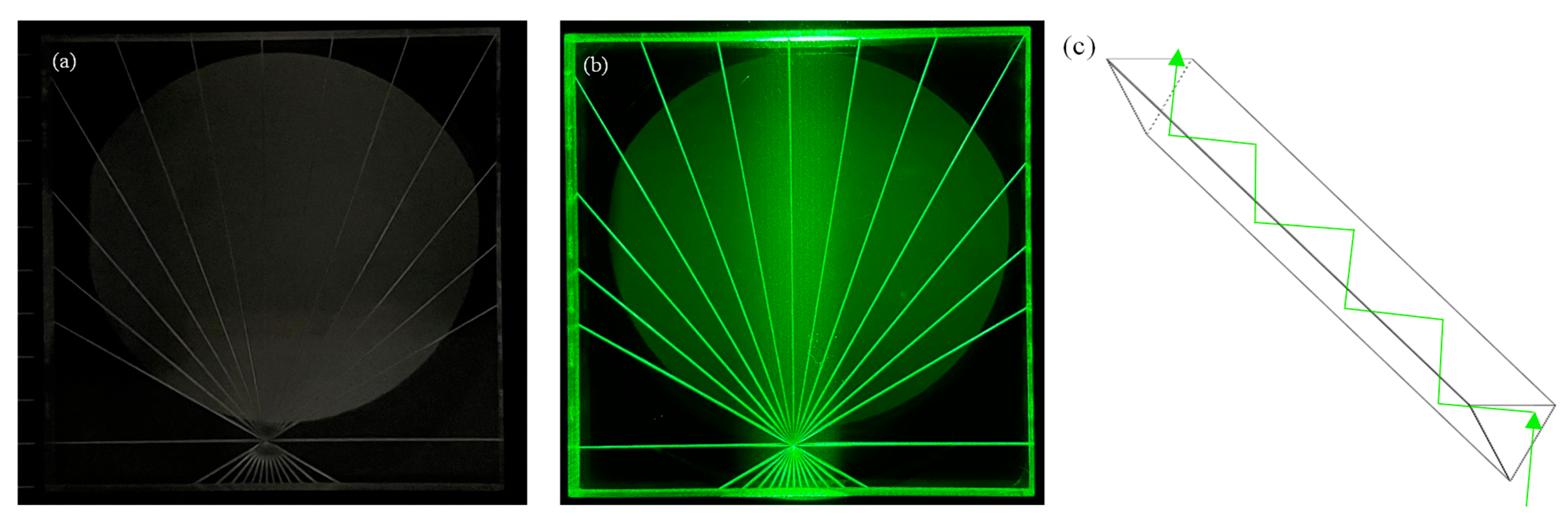
6. Application and Implementation of the Proposed Approach
7. Summary and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Guo, Q.; Liu, Z.; Yang, Z.; Jiang, Y.; Sun, Y.; Xu, J.; Zhao, W.; Wang, W.; Wang, W.; Ren, Q. Development, challenges and future trends on the fabrication of micro-textured surfaces using milling technology. J. Manuf. Process. 2024, 126, 285–331. [Google Scholar] [CrossRef]
- Chen, E.; Guo, J.; Jiang, Z.; Shen, Q.; Ye, Y.; Xu, S.; Sun, J.; Yan, Q.; Guo, T. Edge/direct-lit hybrid mini-LED backlight with U-grooved light guiding plates for local dimming. Opt. Express 2021, 29, 12179–12194. [Google Scholar] [CrossRef]
- Chen, C.-F.; Kuo, S.-H. A highly directional light guide plate based on V-groove microstructure cell. J. Disp. Technol. 2014, 10, 1030–1035. [Google Scholar] [CrossRef]
- Tian, J.F.; Kannangara, D.; Li, X.; Shen, W. Capillary driven low-cost V-groove microfluidic device with high sample transport efficiency. Lab Chip 2010, 10, 2258–2264. [Google Scholar] [CrossRef] [PubMed]
- Steinhoff, K.; Rasp, W.; Pawelski, O. Development of deterministic-stochastic surface structures to improve the tribological conditions of sheet forming processes. J. Mater. Process Tech. 1996, 60, 355–361. [Google Scholar] [CrossRef]
- Pan, A.; Si, J.; Chen, T.; Ma, Y.; Chen, F.; Hou, X. Fabrication of high-aspect-ratio grooves in silicon using femtosecond laser irradiation and oxygen-dependent acid etching. Opt. Express 2013, 21, 16657–16662. [Google Scholar] [CrossRef]
- Abdulbari, H.A.; Mahammed, H.D.; Hassan, Z.B. Bio-inspired passive drag reduction techniques: A review. ChemBioEng Rev. 2015, 2, 185–203. [Google Scholar] [CrossRef]
- Miyazaki, M.; Hirai, Y.; Moriya, H.; Shimomura, M.; Miyauchi, A.; Liu, H. Biomimetic Riblets Inspired by Sharkskin Denticles: Digitizing, Modeling and Flow Simulation. J. Bionic Eng. 2018, 15, 999–1011. [Google Scholar] [CrossRef]
- Wang, S.; To, S.; Chen, X.; Wang, H.; Xia, H. A study of the fabrication of v-groove structure in ultra-precision milling. Int. J. Comput. Integr. Manuf. 2014, 27, 986–996. [Google Scholar] [CrossRef]
- Domel, A.G.; Saadat, M.; Weaver, J.C.; Haj-Hariri, H.; Bertoldi, K.; Lauder, G.V. Shark skin-inspired designs that improve aerodynamic performance. J. R. Soc. Interface 2018, 15, 20170828. [Google Scholar] [CrossRef]
- Chen, D.; Liu, Y.; Chen, H.; Zhang, D. Bio-inspired drag reduction surface from sharkskin. Biosurface Biotribology 2018, 4, 39–45. [Google Scholar] [CrossRef]
- Kawtharani, F.; Serio, B.; Guida, G.; Twardowski, P.; Hammoud, M. Solar Energy Management Using a V-Groove: An Approach Based on a Multiple Optical Path Algorithm. Technologies 2022, 10, 101. [Google Scholar] [CrossRef]
- Cho, C.; Kim, H.; Jeong, S.; Baek, S.-W.; Seo, J.-W.; Han, D.; Kim, K.; Park, Y.; Yoo, S.; Lee, J.-Y. Random and V-groove texturing for efficient light trapping in organic photovoltaic cells. Sol. Energy Mater. Sol. Cells 2013, 115, 36–41. [Google Scholar] [CrossRef]
- Kim, B.K.; Kang, K.-H.; Gao, M.-Y.; Kim, J.; Kim, D.-E. Effect of v-groove surface pattern on the tribological properties of epoxy. Tribol. Trans. 2021, 64, 302–312. [Google Scholar] [CrossRef]
- Yang, S.; Guo, C.; Ren, W. Research on optimization of milling performance of V-groove micro-texture ball-end milling cutter. J. Mech. Sci. Technol. 2022, 36, 2849–2860. [Google Scholar] [CrossRef]
- Xue, B.; Yan, Y.; Ma, G.; Hu, Z. Study on the machining process of micro V-shaped groove by using a revolving tip. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2018, 232, 1523–1537. [Google Scholar] [CrossRef]
- Moriya, T.; Nakamoto, K.; Ishida, T.; Takeuchi, Y. Creation of V-shaped microgrooves with flat-ends by 6-axis control ultraprecision machining. Cirp Ann-Manuf. Technol. 2010, 59, 61–66. [Google Scholar] [CrossRef]
- Brinksmeier, E.; Gläbe, R.; Schönemann, L. Review on diamond-machining processes for the generation of functional surface structures. CIRP J. Manuf. Sci. Technol. 2012, 5, 1–7. [Google Scholar] [CrossRef]
- Joao, D.; Milliken, N.; Tutunea-Fatan, O.R.; Bordatchev, E.V. Axial strategies for ultraprecise single point cutting of V-grooves. Precis. Eng. 2020, 66, 10–20. [Google Scholar] [CrossRef]
- Joao, D.; Rangel, O.; Milliken, N.; Tutunea-Fatan, O.R.; Bordatchev, E. Single-flank machining strategy for ultraprecise single-point cutting of V-grooves. Int. J. Adv. Manuf. Tech. 2022, 120, 4505–4523. [Google Scholar] [CrossRef]
- Li, H.; Xu, Z.; Ji, Z.; Pi, J. Quantitative characterization of Poisson burrs on the V-shaped texture based on the volume method. J. Mater. Res. Technol. 2020, 9, 16280–16288. [Google Scholar] [CrossRef]
- Rangel, O.F.; Tutunea-Fatan, O.R.; Bordatchev, E.V. Single point diamond cutting of high-aspect ratio V-grooves: Alternative flank cutting strategy and preliminary burr formation analysis. In Proceedings of the 36th Annual Meeting of the American Society for Precision Engineering, ASPE 2021, Minneapolis, MN, USA, 1–5 November 2021. [Google Scholar]
- Sawada, K.; Kawai, T.; Takeuchi, Y.; Sata, T. Development of ultraprecision micro grooving: Manufacture of V-shaped groove. JSME Int. J. Ser. C Mech. Syst. Mach. Elem. Manuf. 2000, 43, 170–176. [Google Scholar] [CrossRef]
- Bordatchev, E.; Tauhiduzzaman, M.; Kugler, T.; Katz, A.; Bohr, R. Demonstration of advanced capabilities of 5-axis micromilling: Geometries with high-aspect ratio and/or optical surface quality. In Proceedings of the 8th International Conference on Micromanufacturing, Victoria, BC, Canada, 25–28 March 2013; pp. 357–362. [Google Scholar]
- Park, S.; Shin, Y. Light guide plate with curved V-groove patterns in edge-lit backlight. Opt. Eng. 2016, 55, 015103. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Churchill-Baird, J.T.; Tutunea-Fatan, O.R.; Bordatchev, E.V. Integrated CAD/CAM Approach for Parametric Design and High-Precision Microfabrication of Planar Functional Structures Comprising Radially Oriented V-Grooves. J. Manuf. Mater. Process. 2025, 9, 100. https://doi.org/10.3390/jmmp9030100
Churchill-Baird JT, Tutunea-Fatan OR, Bordatchev EV. Integrated CAD/CAM Approach for Parametric Design and High-Precision Microfabrication of Planar Functional Structures Comprising Radially Oriented V-Grooves. Journal of Manufacturing and Materials Processing. 2025; 9(3):100. https://doi.org/10.3390/jmmp9030100
Chicago/Turabian StyleChurchill-Baird, Jonas T., O. Remus Tutunea-Fatan, and Evgueni V. Bordatchev. 2025. "Integrated CAD/CAM Approach for Parametric Design and High-Precision Microfabrication of Planar Functional Structures Comprising Radially Oriented V-Grooves" Journal of Manufacturing and Materials Processing 9, no. 3: 100. https://doi.org/10.3390/jmmp9030100
APA StyleChurchill-Baird, J. T., Tutunea-Fatan, O. R., & Bordatchev, E. V. (2025). Integrated CAD/CAM Approach for Parametric Design and High-Precision Microfabrication of Planar Functional Structures Comprising Radially Oriented V-Grooves. Journal of Manufacturing and Materials Processing, 9(3), 100. https://doi.org/10.3390/jmmp9030100







