Accuracy and Sheet Thinning Improvement of Deep Titanium Alloy Part with Warm Incremental Sheet-Forming Process
Abstract
:1. Introduction
- Single-Point Incremental Forming (SPIF) is done using a single tool and no need for tailored tools and dies [4,5,6]. The major parameters in SPIF have been identified, and process capabilities are being expanded [7,8]. An incremental sheet-forming process has been successfully employed at room temperature for many sheet metals especially those with good formability such as aluminum alloy [9], stainless steel [10] and magnesium sheets [11].
- Two-Point Incremental Forming (TPIF) is similar to SPIF, but on the other side of the sheet is a local supported partially by a die to ensure a better and more precise shape of the final part [3].
- Göttmann et al. [17] proposed a new machine setup that projects an elliptical laser onto Ti-A16V4 sheets in order to attempt a heating of the forming zone at 400 °C. Other solutions were proposed to improve the titanium formability titanium alloy.
- Xu et al. [18] combined high-speed rotation of the punch tool with an electric static heating to reach a high-level temperature (400 °C) in SPIF that is enough to affect the material behavior.
- Honarpisheh et al. [19] investigate experimentally and numerically electric hot incremental forming and study the effect of process parameters (wall angle, step size, and tool diameter) on the forming force and thickness distribution of the final part.
- Vahdani et al. investigated using resistance as an electric current to generate a heat SPIF setup for the contact zone between the forming tool and the sheet [20]. The obtained results show that the formability of the Ti-6Al-4V alloy sheet strongly depends on the lubrication condition.
- Liu [21] presented a state-of-the-art review of heat-assisted incremental sheet forming. The author groups together the works carried out, in particular those used for heating titanium alloys, laser heat [17], friction heat [22], electric heat [20], induction heat [23], and combined heat friction [24].
- Jin et al. present several warm SPIF (WSPIF) methods to improve the formability and overcome the low geometrical accuracy [25].
2. Materials and Methods
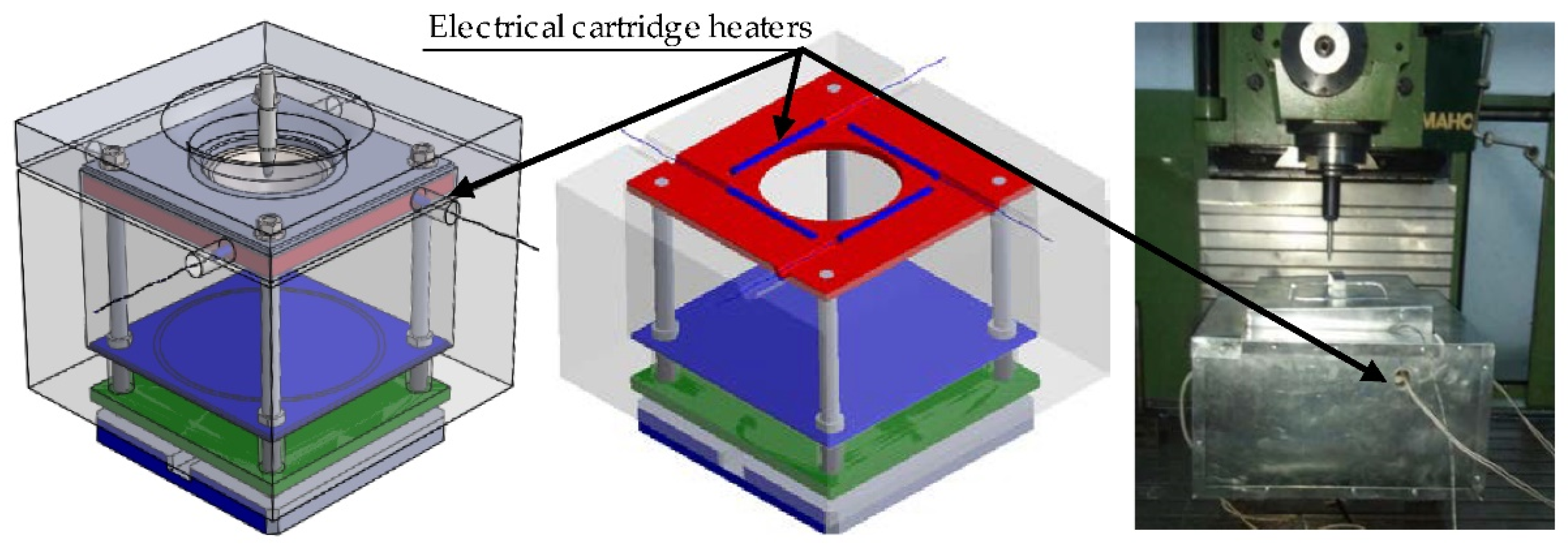
2.1. Experimental Warm SPIF Setup
- (a)
- A multiaxial load cell force sensor FN7325-M6 provides monitoring and measurement of the forces. This sensor measures forces up to 5000 N and moments up to 200 Nm on the X and Y axes, and along the Z axis, forces up to 250 kN and moments up to 7000 Nm. The working temperature range is between −20 and 80 °C, and for the reliability of the measurements, the sensor is calibrated.
- (b)
- The incremental forming process is carried out with a 3-axis CNC vertical milling machine MAHO.
- (c)
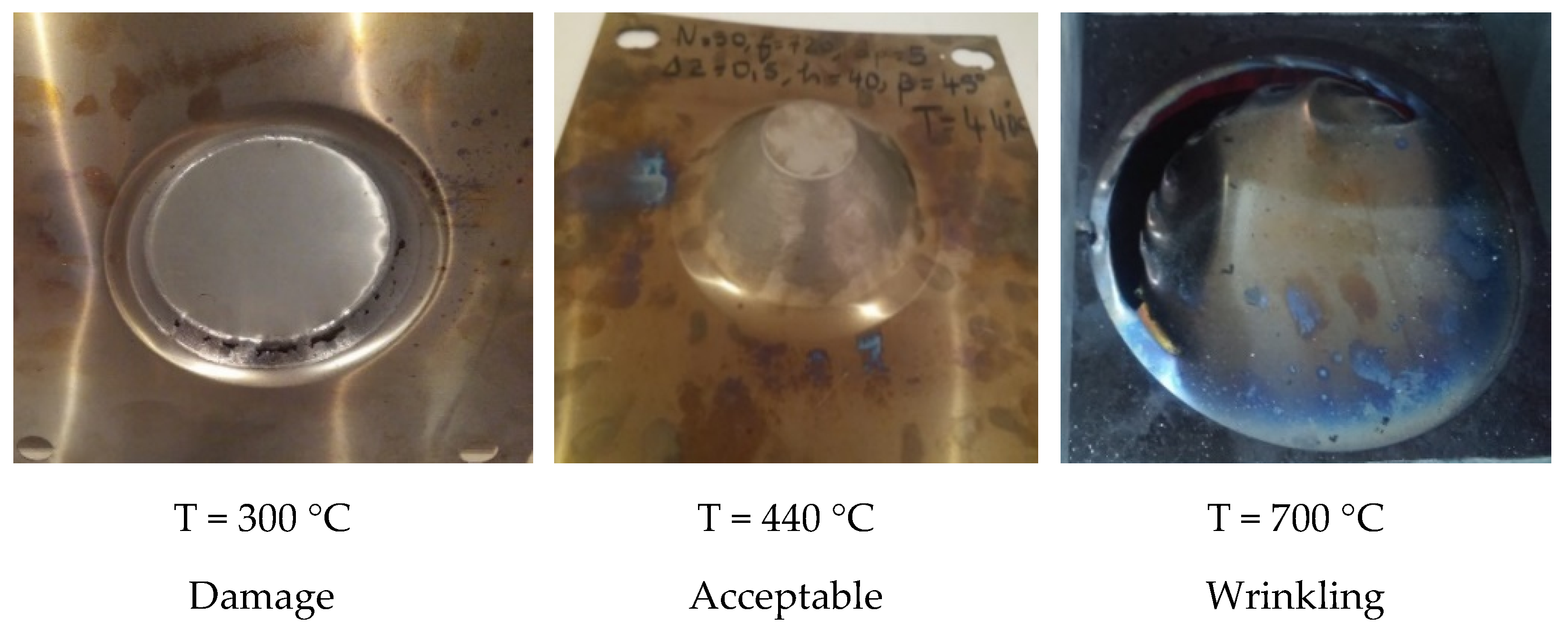
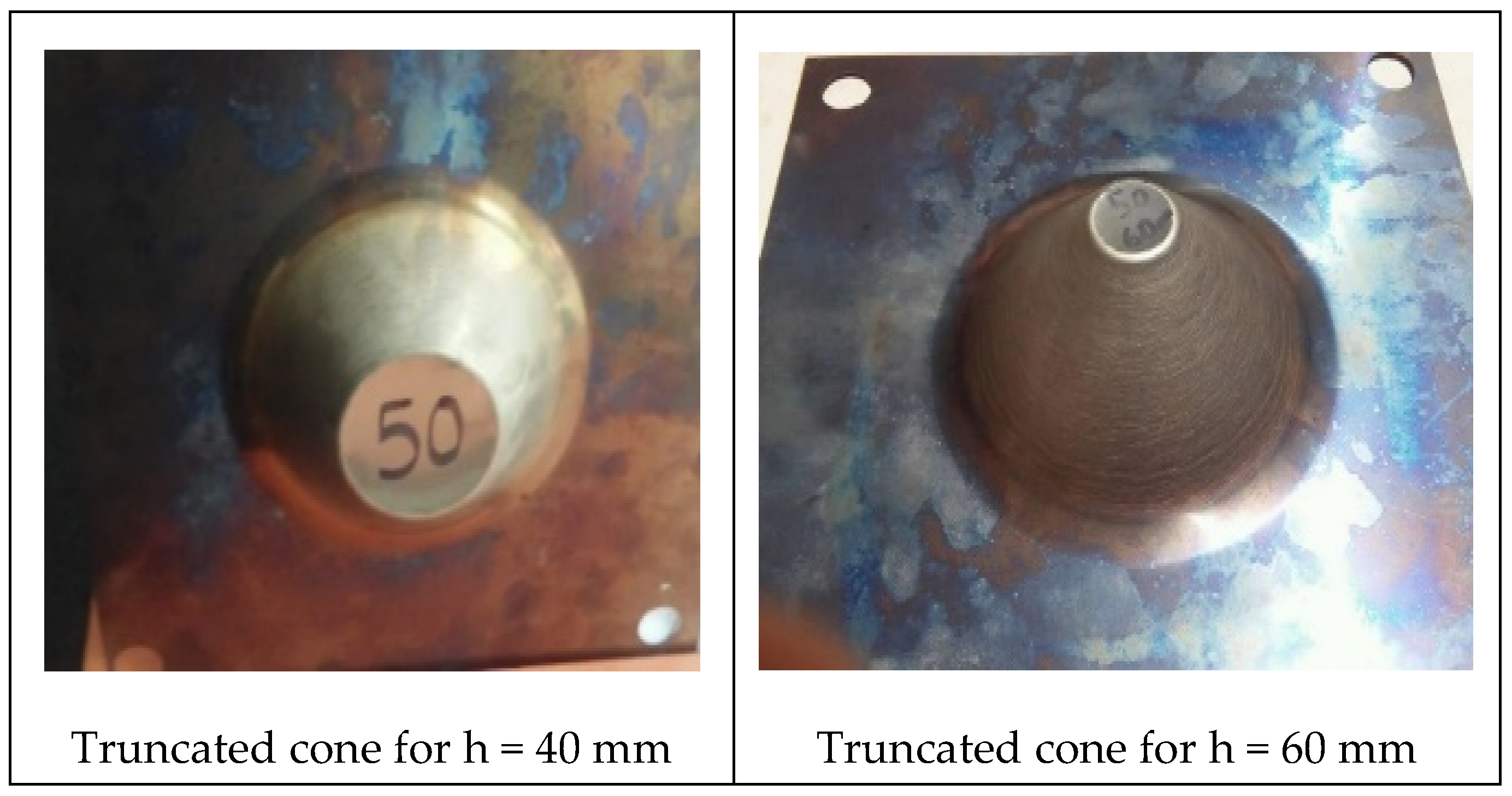
- The Ti-6Al-4V alloy sheet size (300 × 300 mm) with initial thickness ti = 0.5 mm and the effective working area was (70 × 70 mm) is formed with spherical punch controlled by computer machine. The diameter of the punch of dp = 5 mm is made of X160CrMoV12 steel, which has undergone a heat treatment (55HRC Hardness). The punch speed S = 50 rpm and Feed rate f = 600 mm/min are chosen in order to reduce the effect on the heat generation due to the friction with the sheet (see Figure 3). The incremental forming of Ti-6Al-4V alloy, the mechanical characteristics of which are given in Table 1, is carried out for a temperature in the range of 400 to 600 °C and a step down Δz of 0.5 mm.
- (d)
- The real-time temperature monitoring is provided by an IRISYS 4000 type infrared camera.
- (e)
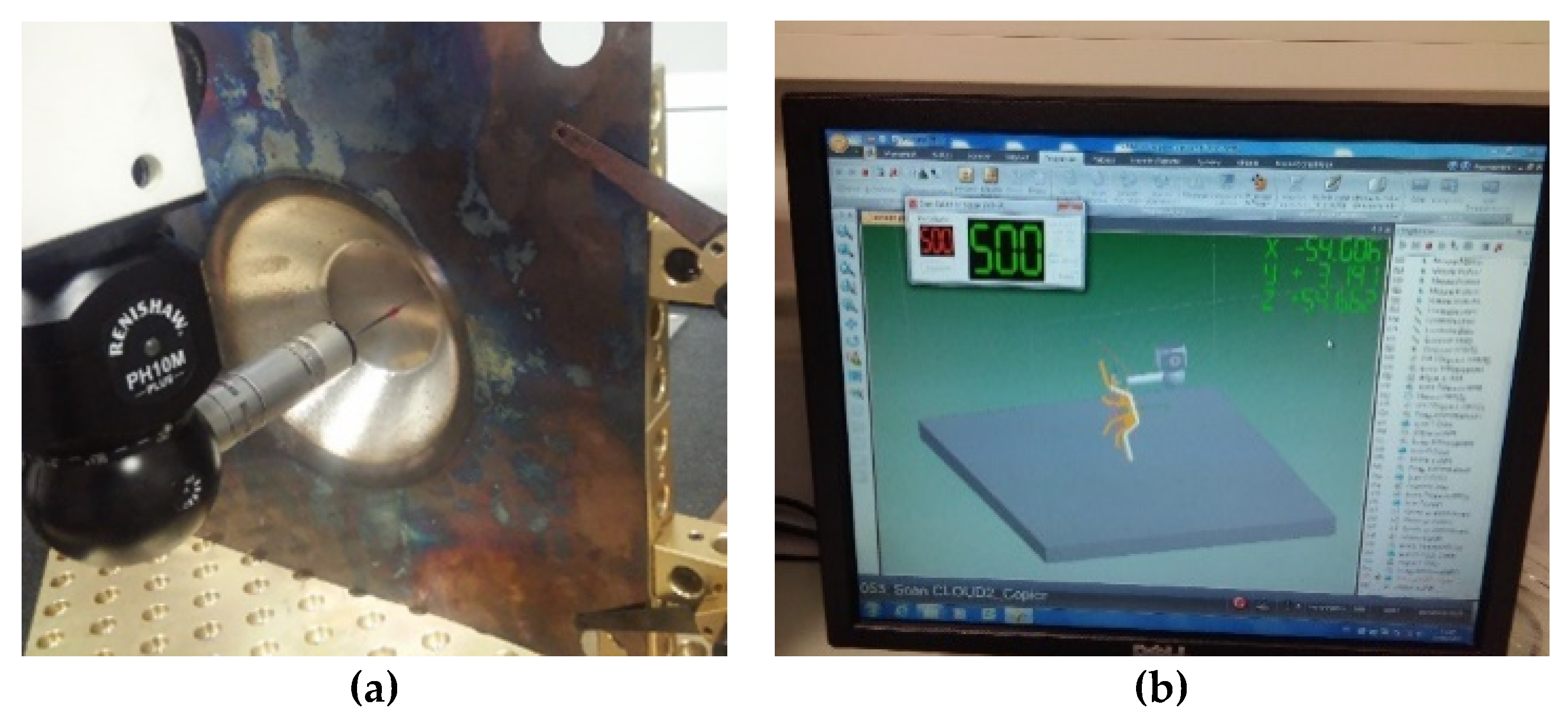
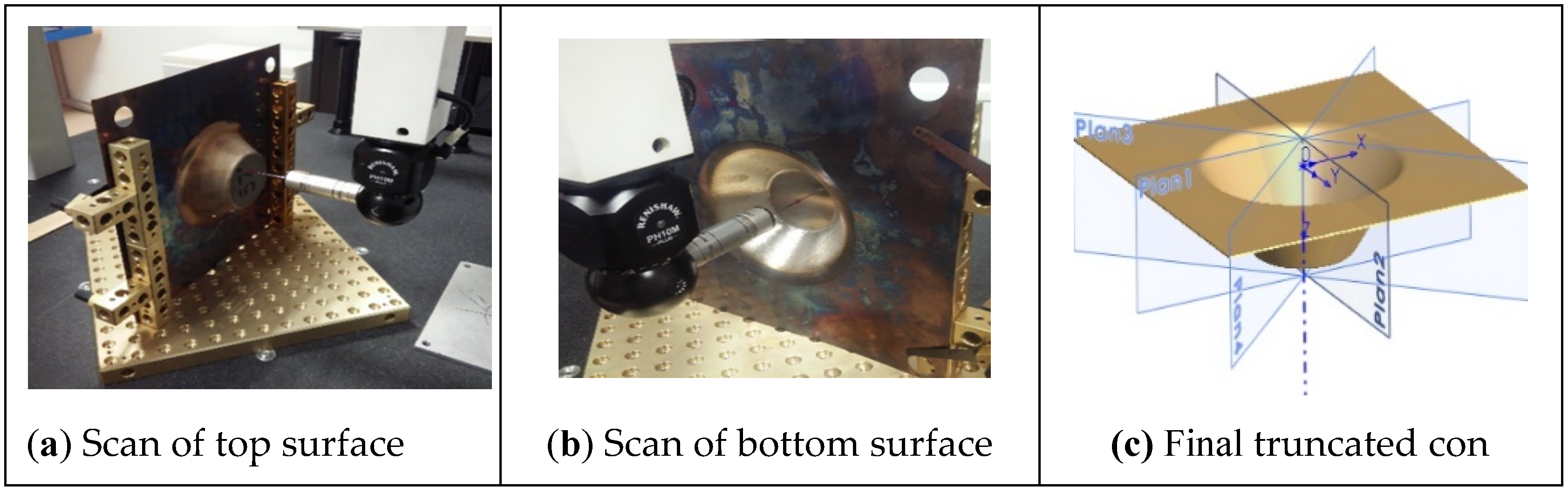
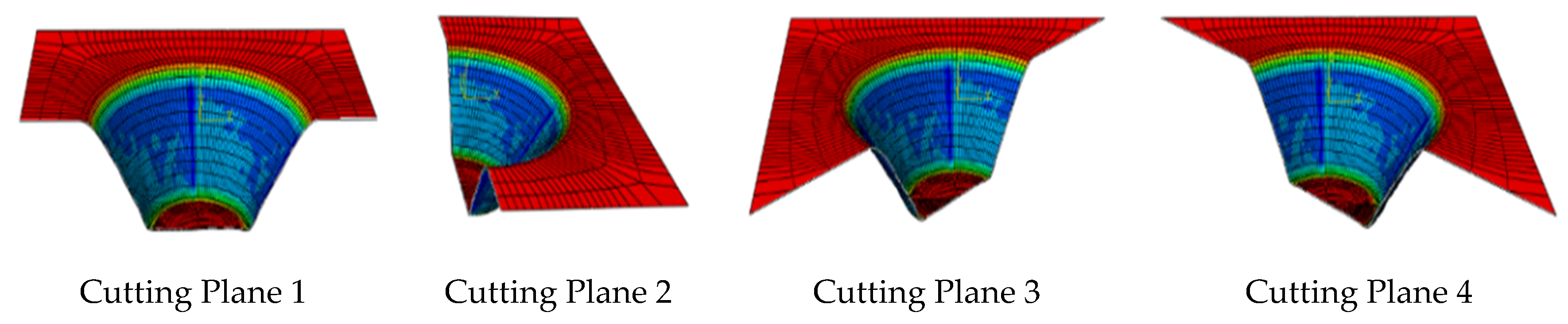
- The reverse engineering approach for the surface reconstruction of CAD models starting from 3D mesh data is performed to analyze the formability of a deformed truncated cone with a wall angle α of 50°, using three-dimensional Coordinate-Measuring Machine.
2.2. Experimental Test Conditions
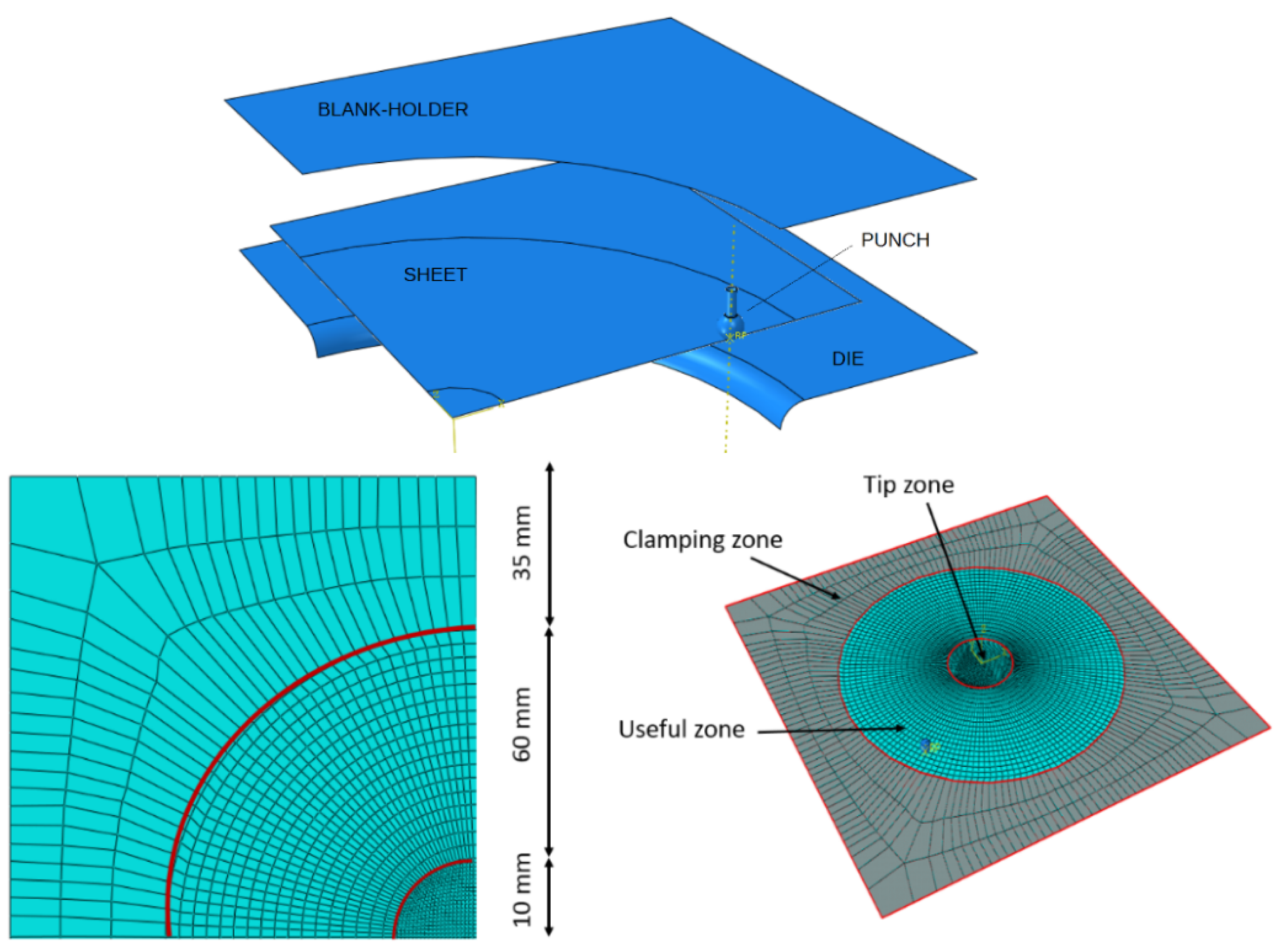
2.3. Finite Elements Modeling of Warm SPIF
- A coarse mesh (480 finite elements with a size of 10 mm × 10 mm) in the large clamping zone 1.
- A circular fine mesh (1600 finite elements) in a useful zone 2 of 60 mm diameter (3 mm × 3 mm).
- Fine mesh (1200 finite elements) in the fine tip zone 3 (0.5 mm × 0.5 mm).
3. Results and Discussions
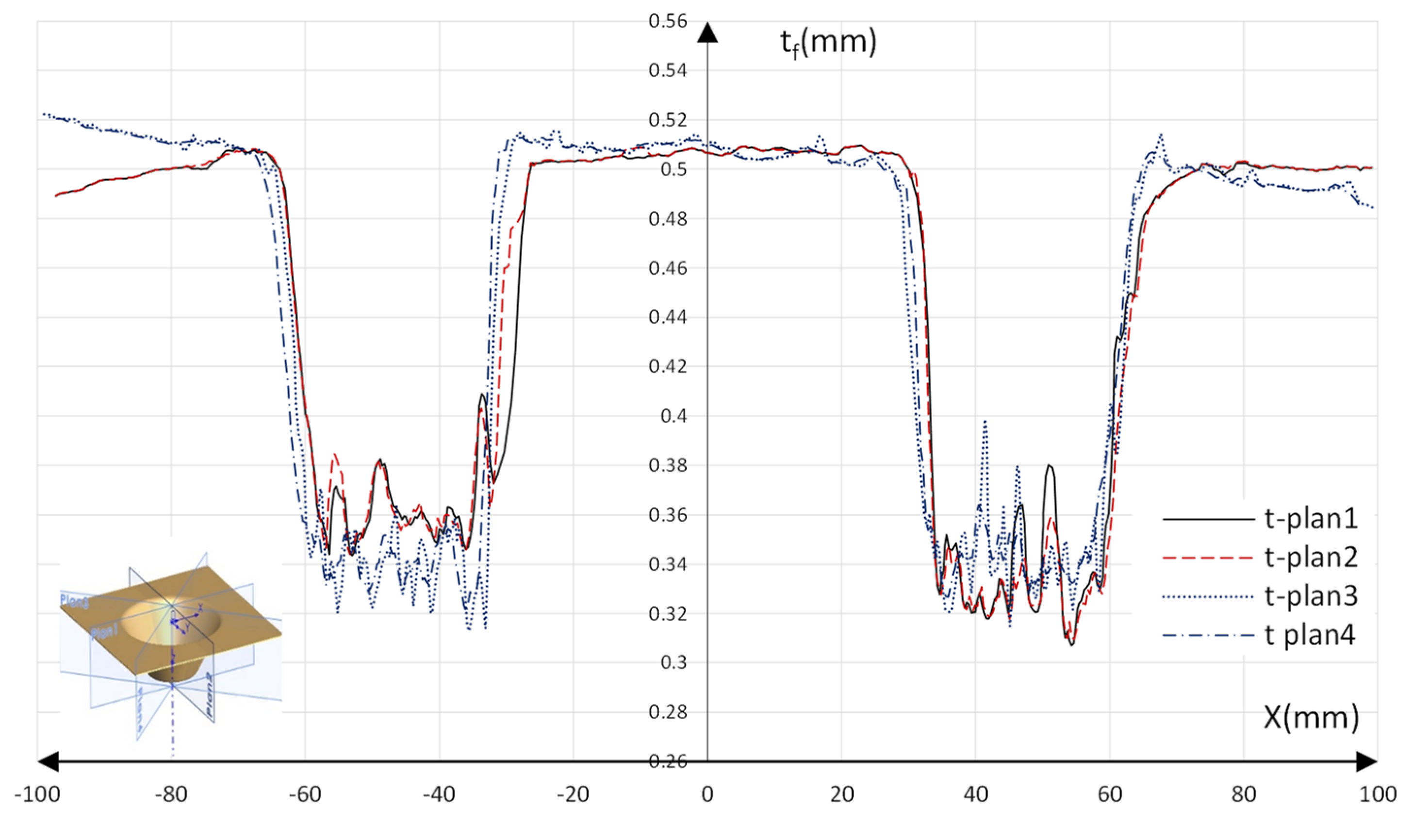
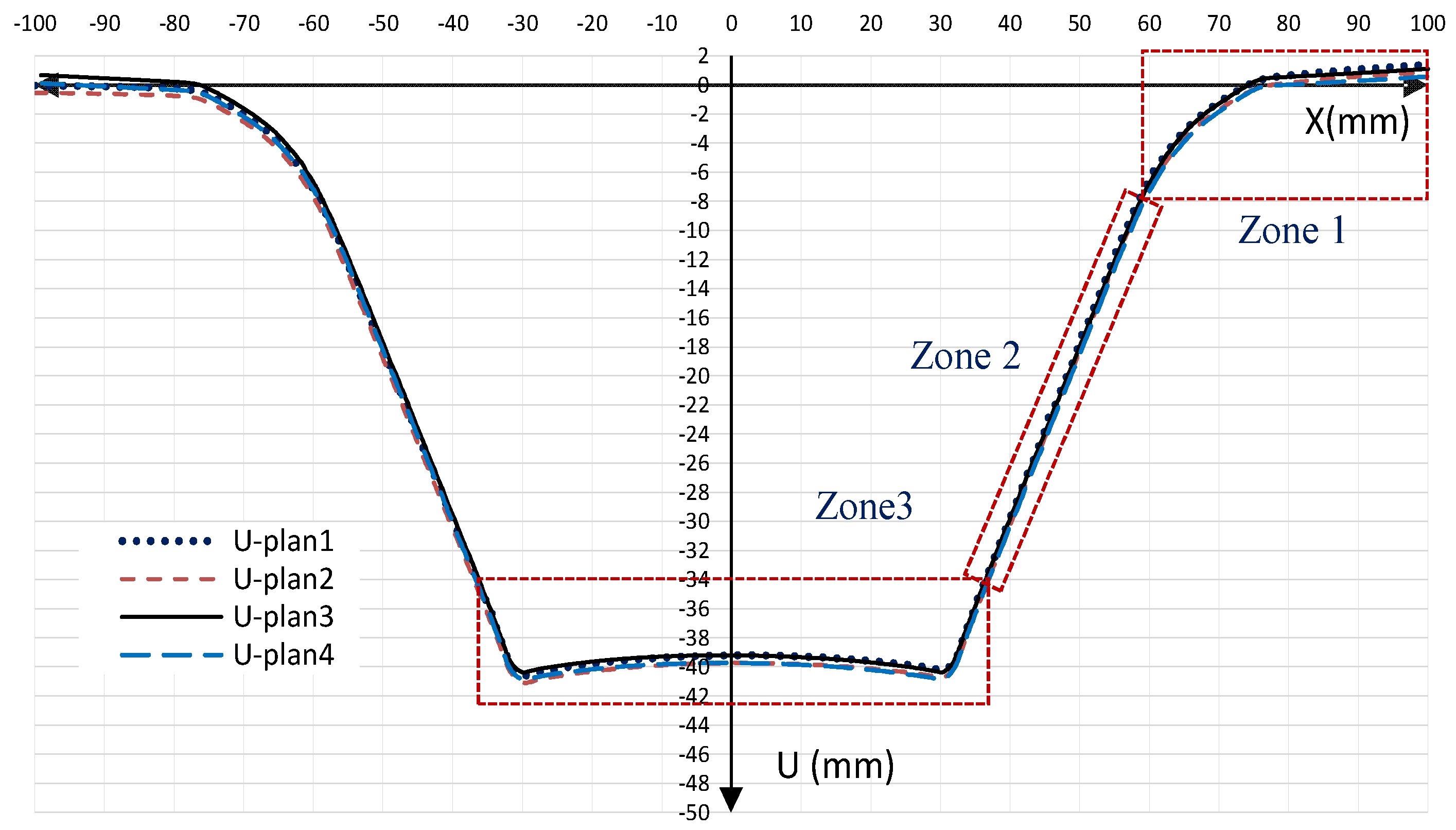
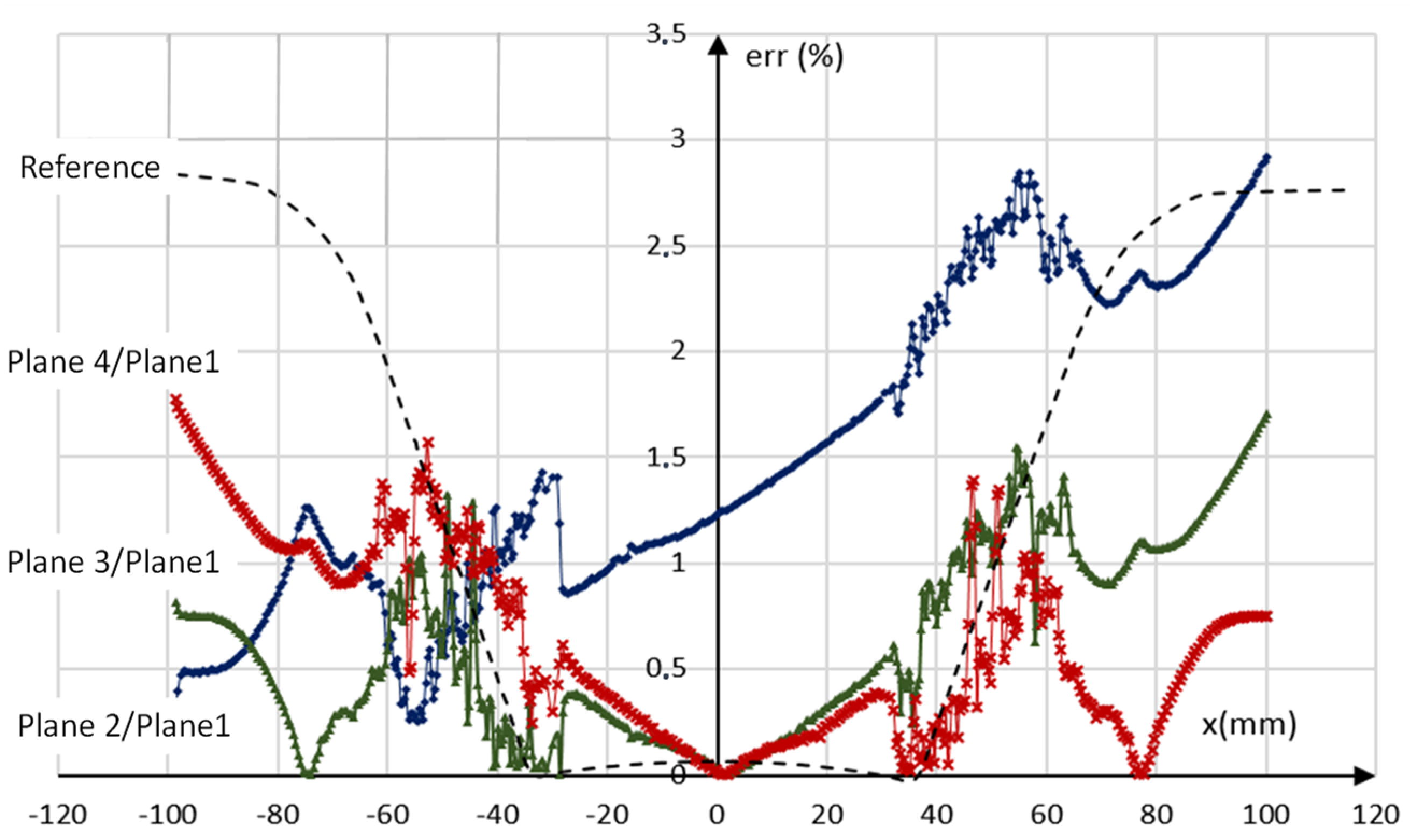
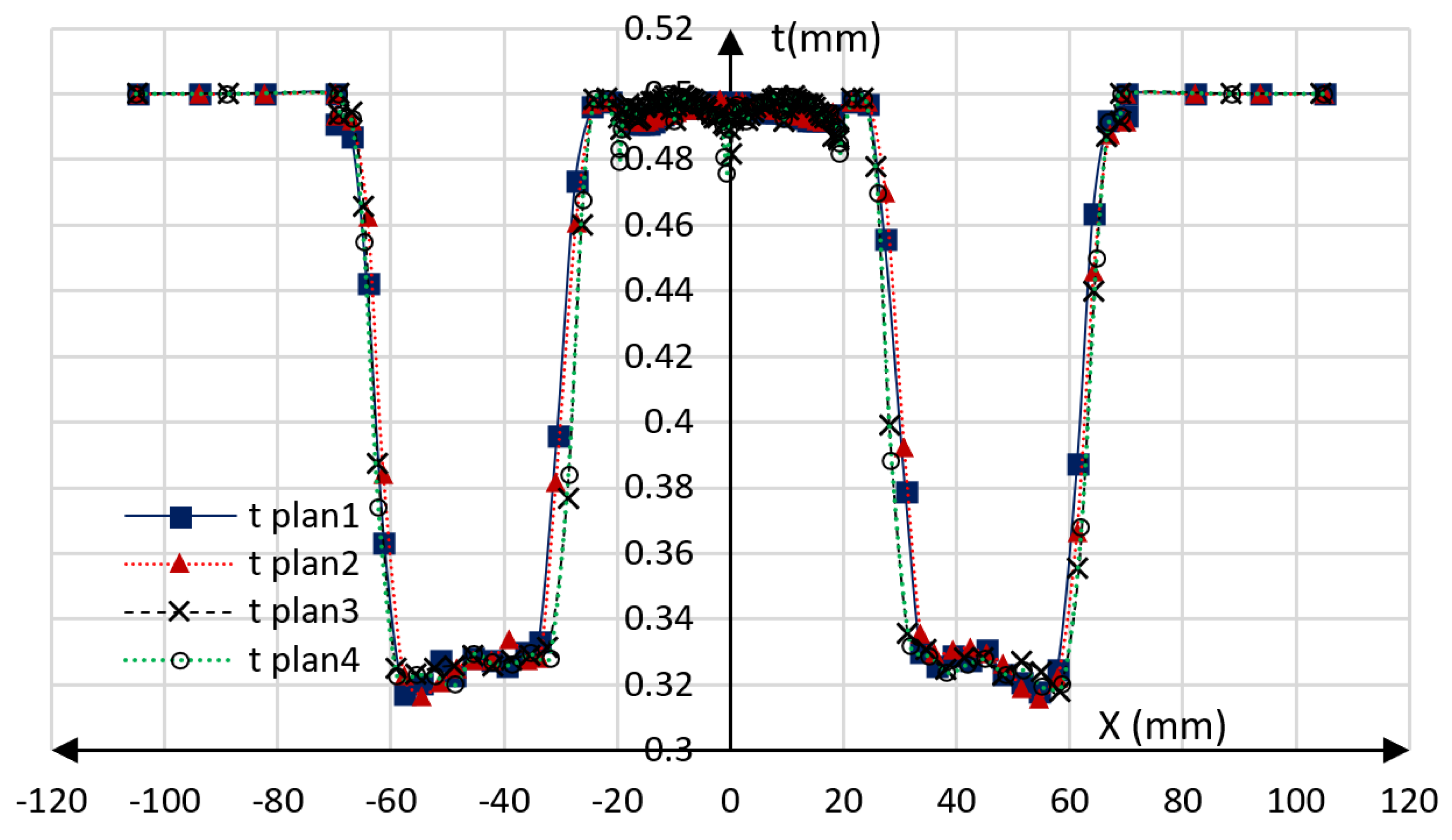
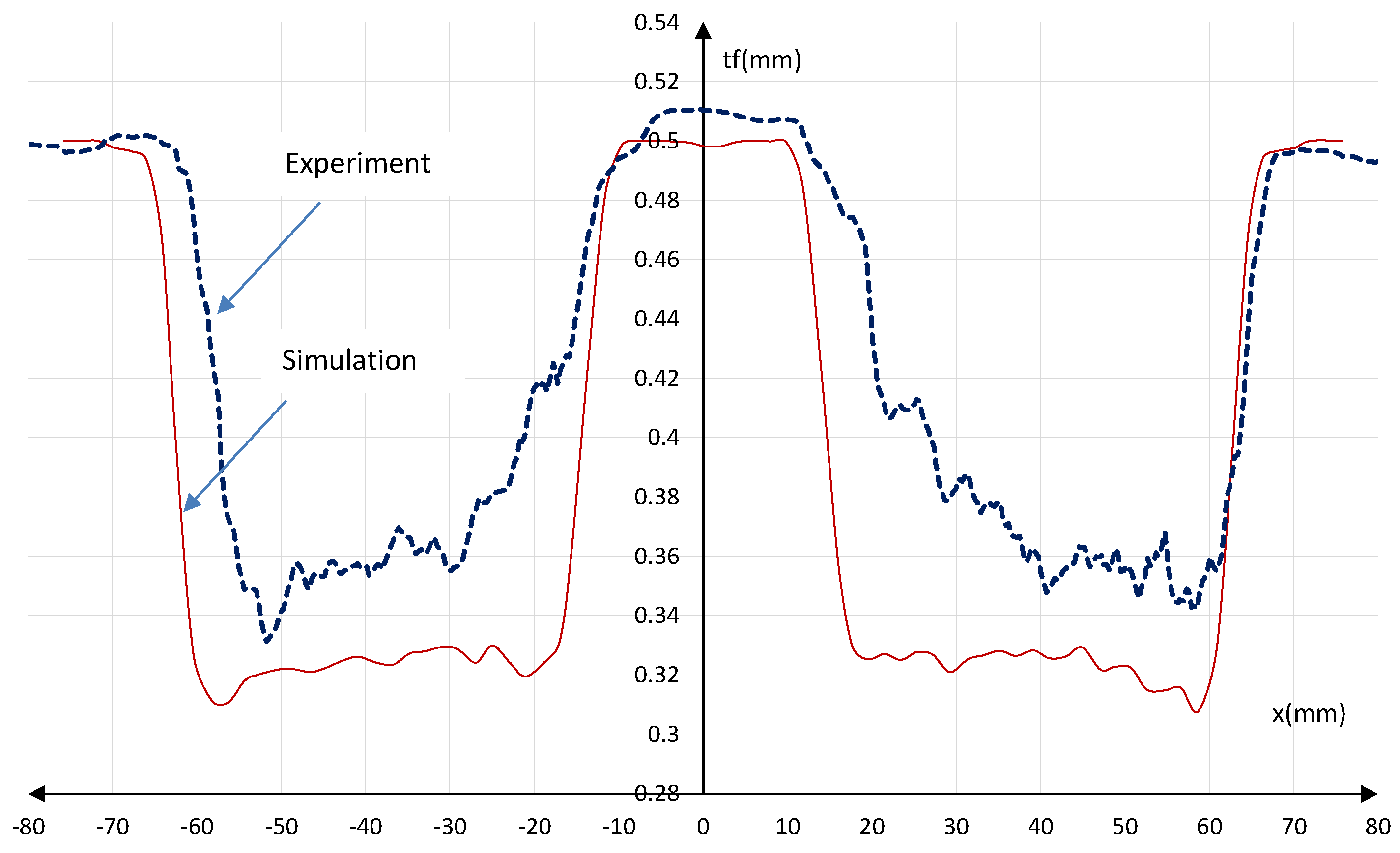
3.1. Measurement of Deformed and Thickness Profile of the Deformed Truncated Cone
3.2. Predicted Profile Shape and Thickness Distribution of the Deformed Truncated Cone
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kim, Y.H.; Park, J.J. Effect of process parameters on formability in incremental forming of sheet metal. J. Mater. Process. Technol. 2002, 130–131, 42–46. [Google Scholar] [CrossRef]
- Möllensiep, D.; Kulessa, P.; Thyssen, L.; Kuhlenkötter, B. Regression-based compensation of part inaccuracies in incremental sheet forming at elevated temperatures. Int. J. Adv. Manuf. Technol. 2020, 109, 1917–1928. [Google Scholar] [CrossRef]
- Li, Y.; Chen, X.; Liu, Z.; Sun, J.; Li, F.; Li, J.; Zhao, G. A review on the recent development of incremental sheet-forming process. Int. J. Adv. Manuf. Technol. 2017, 92, 2439–2462. [Google Scholar] [CrossRef]
- Barimani-Varandi, A.; Nasrabadi, M.K.; Ravan, B.A.; Javadi, M. Rapid prototyping of aircraft canopy based on the incremental forming process. J. Braz. Soc. Mech. Sci. Eng. 2021, 43, 59. [Google Scholar] [CrossRef]
- Azhiri, R.B.; Rahimidehgolan, F.; Javidpour, F.; Tekiyeh, R.M.; Moussavifard, S.M.; Bideskan, A.S. Optimization of Single Point Incremental Forming Process Using Ball Nose Tool. Exp. Tech. 2020, 44, 75–84. [Google Scholar] [CrossRef]
- Kilani, L.; Mabrouki, T.; Ayadi, M.; Chermiti, H.; Belhadi, S. Effects of rolling ball tool parameters on roughness, sheet thinning, and forming force generated during SPIF process. Int. J. Adv. Manuf. Technol. 2020, 106, 4123–4142. [Google Scholar] [CrossRef]
- Gatea, S.; Ou, H.; McCartney, G. Review on the influence of process parameters in incremental sheet forming. Int. J. Adv. Manuf. Technol. 2016, 87, 479–499. [Google Scholar] [CrossRef] [Green Version]
- Gheysarian, A.; Honarpisheh, M. Process Parameters Optimization of the Explosive-Welded Al/Cu Bimetal in the Incremental Sheet Metal Forming Process. Iran. J. Sci. Technol. Trans. Mech. Eng. 2019, 43, 945–956. [Google Scholar] [CrossRef]
- Kumar, N.; Singh, A.; Agrawal, A. Formability Analysis of AA1200 H14 Aluminum Alloy Using Single Point Incremental Forming Process. Trans. Indian Inst. Met. 2020, 73, 1975–1984. [Google Scholar] [CrossRef]
- Saidi, B.; Boulila, A.; Ayadi, M.; Nasri, R. Experimental force measurements in single point incremental sheet forming SPIF. Mech. Ind. 2015, 16, 4. [Google Scholar] [CrossRef]
- Khazaali, H.; Fereshteh-Saniee, F. An Inclusive Experimental Investigation on Influences of Different Process Parameters in Warm Incremental Forming of AZ31 Magnesium Sheets. Iran. J. Sci. Technol. Trans. Mech. Eng. 2019, 43, 347–358. [Google Scholar] [CrossRef]
- Fan, G.; Sun, F.; Meng, X.; Gao, L.; Tong, G. Electric hot incremental forming of Ti-6Al-4V titanium sheet. Int. J. Adv. Manuf. Technol. 2010, 49, 941–947. [Google Scholar] [CrossRef]
- Saidi, B.; Moreau, L.G.; Mhemed, S.; Cherouat, A.; Adragna, P.-A.; Nasri, R. Hot incremental forming of titanium human skull prosthesis by using cartridge heaters: A reverse engineering approach. Int. J. Adv. Manuf. Technol. 2019, 101, 873–880. [Google Scholar] [CrossRef]
- Trevisan, F.; Calignano, F.; Aversa, A.; Marchese, G.; Lombardi, M.; Biamino, S.; Ugues, D.; Manfredi, D. Additive manufacturing of titanium alloys in the biomedical field: Processes, properties and applications. J. Appl. Biomater. Funct. Mater. 2018, 16, 2, 57–67. [Google Scholar] [CrossRef]
- Sbayti, M.; Ghiotti, A.; Bahloul, R.; Belhadjsalah, H.; Bruschi, S. Finite Element Analysis of hot Single Point Incremental forming of hip prostheses. MATEC Web Conf. 2016, 80, 14006. [Google Scholar] [CrossRef] [Green Version]
- Konka, P.; Lingam, R.; Singh, U.A.; Shivaprasad, C.; Reddy, N.V. Enhancement of accuracy in double sided incremental forming by compensating tool path for machine tool errors. Int. J. Adv. Manuf. Technol. 2020, 111, 1187–1199. [Google Scholar] [CrossRef]
- Göttmann, A.; Diettrich, J.; Bergweiler, G.; Bambach, M.; Hirt, G.; Loosen, P.; Poprawe, R. Laser-assisted asymmetric incremental sheet forming of titanium sheet metal parts. Prod. Eng. 2011, 5, 263–271. [Google Scholar] [CrossRef]
- Xu, D.; Lu, B.; Cao, T.; Zhang, H.; Chen, J.; Long, H.; Cao, J. Enhancement of process capabilities in electrically-assisted double sided incremental forming. Mater. Des. 2016, 92, 268–280. [Google Scholar] [CrossRef]
- Honarpisheh, M.; Abdolhoseini, M.J.; Amini, S. Experimental and numerical investigation of the hot incremental forming of Ti-6Al-4V sheet using electrical current. Int. J. Adv. Manuf. Technol. 2016, 83, 9–12. [Google Scholar] [CrossRef]
- Vahdani, M.; Mirnia, M.J.; Gorji, H.; Bakhshi-Jooybari, M. Experimental Investigation of Formability and Surface Finish into Resistance Single-Point Incremental Forming of Ti–6Al–4V Titanium Alloy Using Taguchi Design. Trans. Indian Inst. Met. 2019, 72, 1031–1041. [Google Scholar] [CrossRef]
- Liu, Z. Heat-assisted incremental sheet forming: A state-of-the-art review. Int. J. Adv. Manuf. Technol. 2018, 98, 2987–3003. [Google Scholar] [CrossRef]
- Uheida, E.H.; Oosthuizen, G.A.; Dimitrov, D.M.; Bezuidenhout, M.B.; Hugo, P.A. Effects of the relative tool rotation direction on formability during the incremental forming of titanium sheets. Int. J. Adv. Manuf. Technol. 2018, 96, 3311–3319. [Google Scholar] [CrossRef] [Green Version]
- Ambrogio, G.; Gagliardi, F.; Chamanfar, A.; Misiolek, W.Z.; Filice, L. Induction heating and cryogenic cooling in single point incremental forming of Ti-6Al-4V: Process setup and evolution of microstructure and mechanical properties. Int. J. Adv. Manuf. Technol. 2017, 91, 803–812. [Google Scholar] [CrossRef]
- Palumbo, G.; Brandizzi, M. Experimental investigations on the single point incremental forming of a titanium alloy component combining static heating with high tool rotation speed. Mater. Des. 2012, 40, 43–51. [Google Scholar] [CrossRef]
- Jin, K.; Wang, J.; Guo, X.; Domblesky, J.; Wang, H.; Jin, X.; Ding, R. Experimental analysis of electro-assisted warm spin forming of commercial pure titanium components. Int. J. Adv. Manuf. Technol. 2019, 102, 293–304. [Google Scholar] [CrossRef]
- Saidi, B.; Giraud-Moreau, L.; Cherouat, A.; Nasri, R. Experimental and numerical study on optimization of the single point incremental forming of AINSI 304L stainless steel sheet. J. Phys. Conf. Ser. 2017, 896, 012039. [Google Scholar] [CrossRef]
- Saidi, B.; Moreau, L.G.; Cherouat, A.; Nasri, R. Experimental and numerical study on warm single-point incremental sheet forming (WSPIF) of titanium alloy Ti–6Al–4V, using cartridge heaters. J. Braz. Soc. Mech. Sci. Eng. 2020, 42, 534. [Google Scholar] [CrossRef]
- Saidi, B.; Giraud-Moreau, L.; Cherouat, A. Optimization of the single point incremental forming process for titanium sheets by using response surface. MATEC Web Conf. 2016, 80, 10011. [Google Scholar] [CrossRef]
- Khazaali, H.; Fereshteh-Saniee, F. Process Parameter Enhancement for Incremental Forming of Titanium Ti–6Al–4V Truncated Cone with Varying Wall Angle at Elevated Temperatures. Int. J. Precis. Eng. Manuf. 2019, 20, 769–776. [Google Scholar] [CrossRef]
- Johnson, G.R.; Cook, W.H. A Constitutive Model and Data for metals subjected to large strains, high strain rates, and high temperatures. In Proceedings of the 7th International Symposium on Ballistics, The Hague, The Netherlands, 19–21 April 1983; pp. 541–547. [Google Scholar]
- Chen, G.; Ren, C.; Yang, X.; Jin, X.; Guo, T. Finite element simulation of high-speed machining of titanium alloy (Ti–6Al–4V) based on ductile failure model. Int. J. Adv. Manuf. Technol. 2011, 56, 1027–1038. [Google Scholar] [CrossRef]
- Çakırcalı, M.; Kılıçaslan, C.; Güden, M.; Kıranlı, E.; Shchukin, V.Y.; Petronko, V.V. Cross wedge rolling of a Ti6Al4V (ELI) alloy: The experimental studies and the finite element simulation of the deformation and failure. Int. J. Adv. Manuf. Technol. 2013, 65, 9–12. [Google Scholar] [CrossRef] [Green Version]
- Honarpisheh, M.; Gheysarian, A. An Experimental Study on the process parameters of Incremental Forming of Explosively-Welded Al/Cu Bimetal. J. Comput. Appl. Res. Mech. Eng. JCARME 2017, 7, 73–83. [Google Scholar] [CrossRef]
- Naranjo, J.; Miguel, V.; Martínez, A.; Coello, J.; Manjabacas, M. Evaluation of the Formability and Dimensional Accuracy Improvement of Ti6Al4V in Warm SPIF Processes. Metals 2019, 9, 272. [Google Scholar] [CrossRef] [Green Version]





| No. | ti [mm] | rc [mm] | α [°] | dp [mm] | ΔZ [mm] | ØD [mm] | S [rpm] | f [mm/min] | T [°C] | h [mm] |
|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0.5 | 5 | 50 | 5 | 0.5 | 130 | 50 | 600 | 450 | 40 |
| 2 | 0.5 | 5 | 50 | 5 | 0.5 | 130 | 50 | 600 | 450 | 60 |
| T [°C] | Young’s Modulus E [MPa] | Poisson’s Ratio ν | Density ρ [kg/m3] |
|---|---|---|---|
| 21.11 | 117,210 | 0.31 | 4430 |
| 204.44 | 106,870 | 0.31 | 4430 |
| 426.67 | 95,150 | 0.31 | 4430 |
| 648.89 | 82,720 | 0.31 | 4430 |
| Temperature [°C] | Heat Conductivity α [W/m/°C] | Expansion λ [μm/m/°C] | Specific Heat Cp [J/kg/°C] |
|---|---|---|---|
| 17.78 | 6.92 | 1.13 | 387.56 |
| 93.34 | 7.44 | 1.13 | 406.93 |
| 204.44 | 8.65 | 1.13 | 426.31 |
| 426.67 | 11.94 | 1.13 | 474.76 |
| 537.78 | 13.67 | 1.13 | 517.39 |
| 958.22 | 18 | 1.13 | 697.61 |
| A [MPa] | B [MPa] | C | m | n | |||
|---|---|---|---|---|---|---|---|
| 928 | 1062 | 0.0167 | 0.75 | 0.62 | 10−3 | 1663 | 25 |
| Depth (h) | 40 mm | 60 mm |
|---|---|---|
| Minimum predicted thickness: tnum [mm] | 0.33 | 0.31 |
| FE minimum predicted thickness reduction ratio: tnum/ti [%] | 33.8% | 33.6% |
| Minimum experimental thickness: texp [mm] | 0.33 | 0.30 |
| Experimental minimum thickness reduction ratio: texp/ti [%] | 34.4% | 38% |
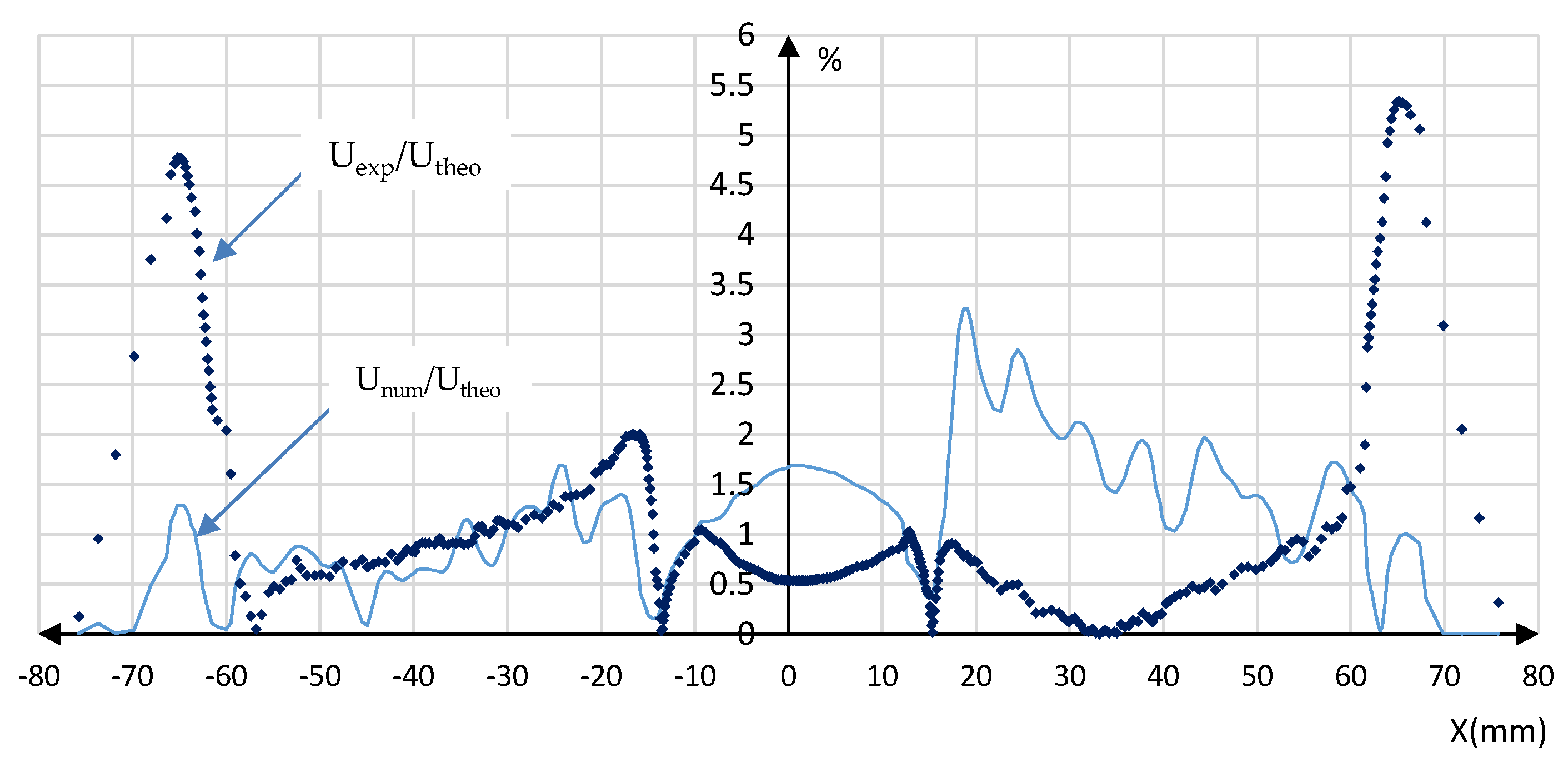
| Error between the experimental and theoretical displacement in Zone 3 (bottom): Uexp/Utheo [%] | 2.75% | 1% |
| Error between the FE simulation and theoretical displacement Zone 3 (bottom): Unum/Utheo [%] | 3% | 3% |
| Error between the experimental and theoretical displacement in Zone 1 (leave level): Uexp/Utheo [%] | 8.75% | 6% |
| Error between the FE simulation and theoretical displacement in Zone 1 (leave level): Unum/Utheo [%] | 5.5% | 2% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Saidi, B.; Giraud Moreau, L.; Cherouat, A.; Nasri, R. Accuracy and Sheet Thinning Improvement of Deep Titanium Alloy Part with Warm Incremental Sheet-Forming Process. J. Manuf. Mater. Process. 2021, 5, 122. https://doi.org/10.3390/jmmp5040122
Saidi B, Giraud Moreau L, Cherouat A, Nasri R. Accuracy and Sheet Thinning Improvement of Deep Titanium Alloy Part with Warm Incremental Sheet-Forming Process. Journal of Manufacturing and Materials Processing. 2021; 5(4):122. https://doi.org/10.3390/jmmp5040122
Chicago/Turabian StyleSaidi, Badreddine, Laurence Giraud Moreau, Abel Cherouat, and Rachid Nasri. 2021. "Accuracy and Sheet Thinning Improvement of Deep Titanium Alloy Part with Warm Incremental Sheet-Forming Process" Journal of Manufacturing and Materials Processing 5, no. 4: 122. https://doi.org/10.3390/jmmp5040122
APA StyleSaidi, B., Giraud Moreau, L., Cherouat, A., & Nasri, R. (2021). Accuracy and Sheet Thinning Improvement of Deep Titanium Alloy Part with Warm Incremental Sheet-Forming Process. Journal of Manufacturing and Materials Processing, 5(4), 122. https://doi.org/10.3390/jmmp5040122







