Effect of the Melt Pool Boundary Network on the Anisotropic Mechanical Properties of Selective Laser Melted 304L
Abstract
:1. Introduction
2. Manufacturing and Experimental Testing
3. Results and Discussion
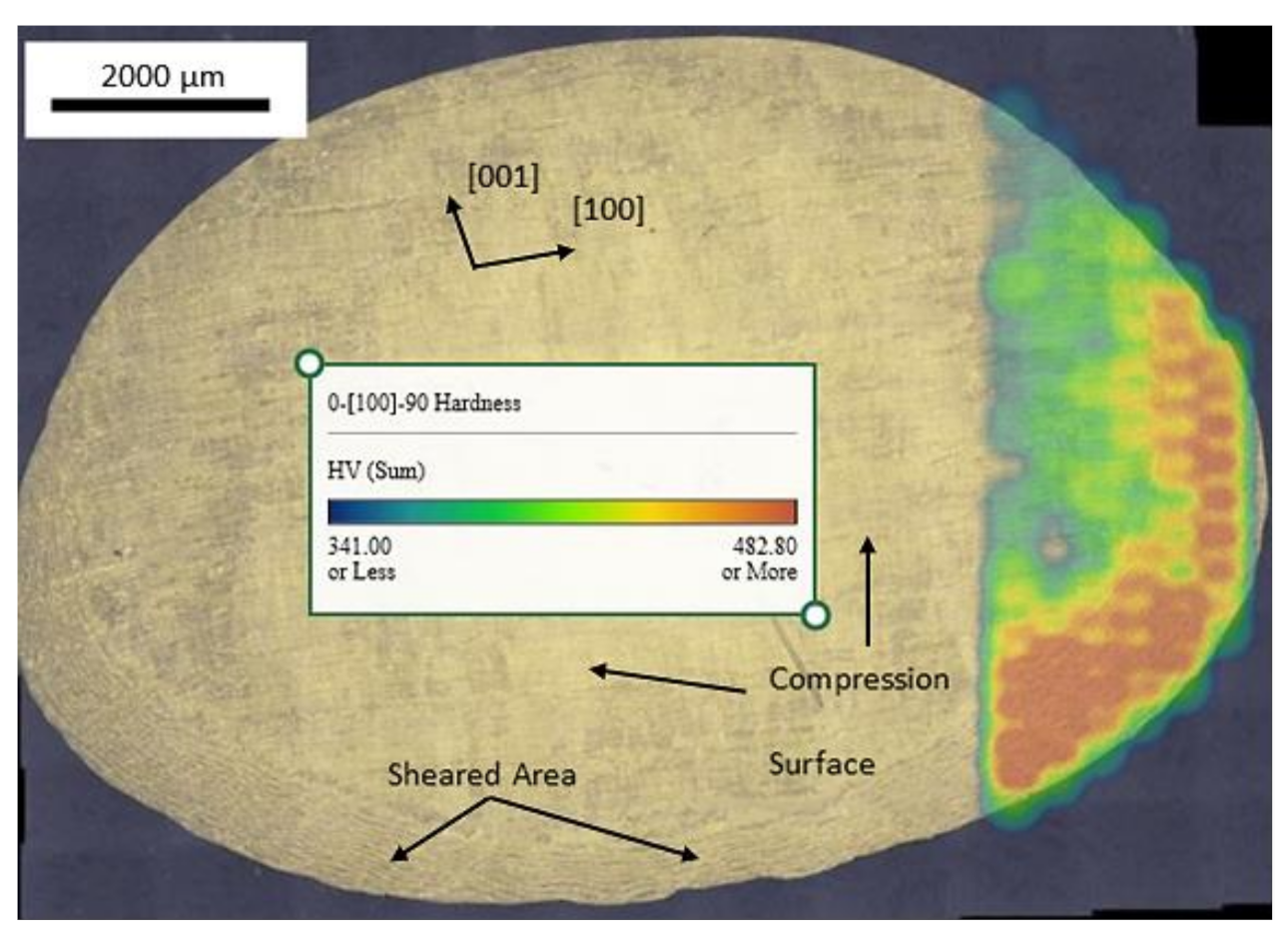
3.1. Experiments
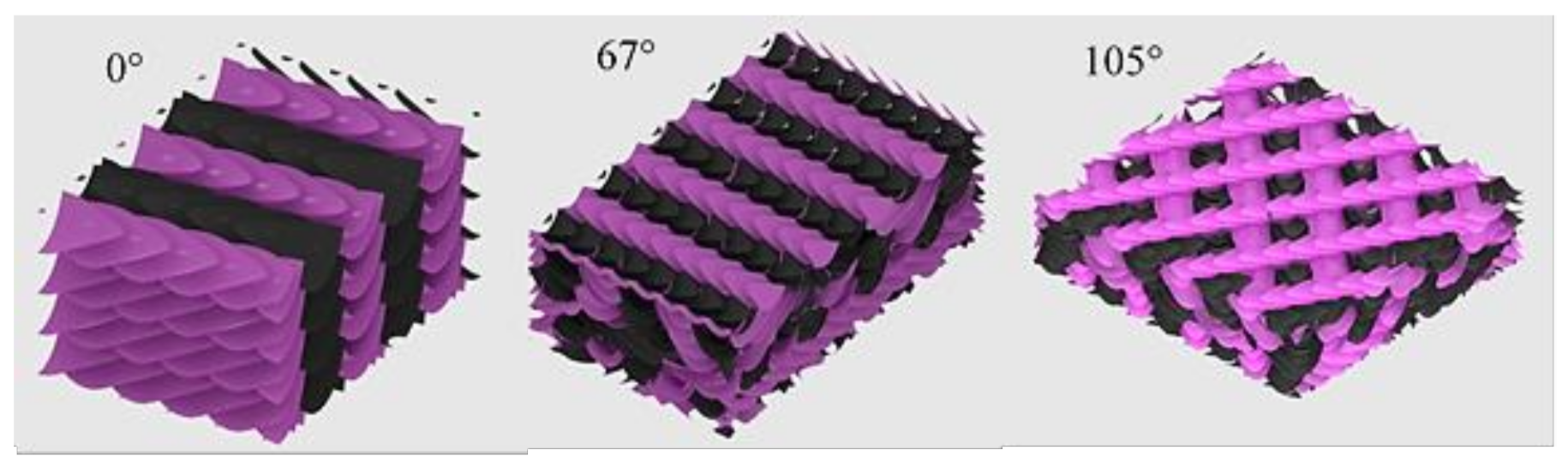
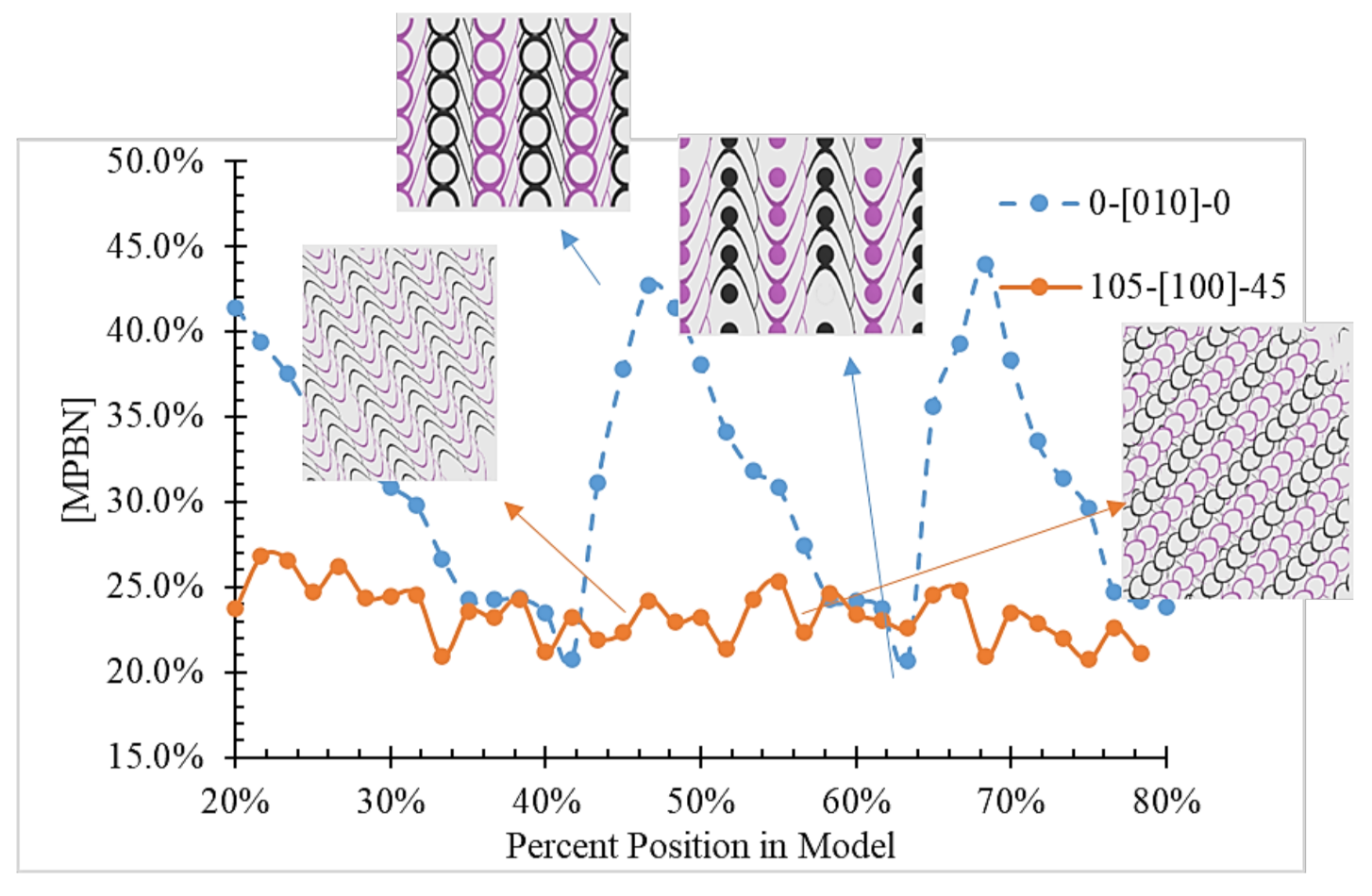
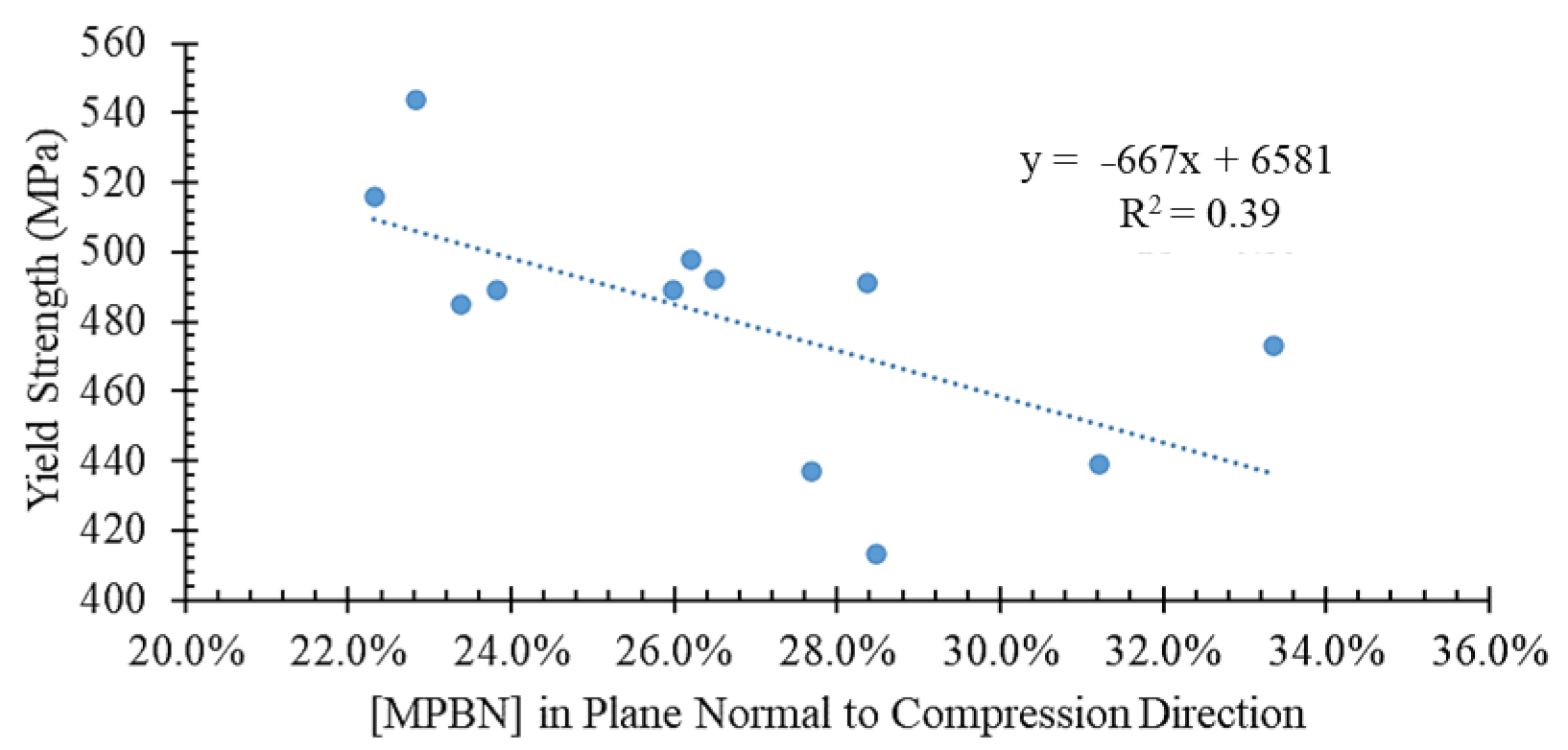
3.2. Modeling of the MPBN
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Levy, G.N.; Schindel, R.; Kruth, J.P. Rapid Manufacturing and Rapid Tooling with Layer Manufacturing (Lm) Technologies, State of the Art and Future Perspectives. CIRP Ann.-Manuf. Technol. 2003, 52, 589–609. [Google Scholar] [CrossRef]
- Huang, R.; Riddle, M.; Graziano, D.; Warren, J.; Das, S.; Nimbalkar, S.; Cresko, J.; Masanet, E. Energy and emissions saving potential of additive manufacturing: The case of lightweight aircraft components. J. Clean. Prod. 2016, 135, 1559–1570. [Google Scholar] [CrossRef] [Green Version]
- Murr, L.E.; Martinez, E.; Amato, K.; Gaytan, S.M.; Hernandez, J.; Ramirez, D.A.; Shindo, P.W.; Medina, F.; Wicker, R.B. Fabrication of Metal and Alloy Components by Additive Manufacturing: Examples of 3D Materials Science. J. Mater. Res. Technol. 2012, 1, 42–54. [Google Scholar] [CrossRef] [Green Version]
- Frazier, W.E. Metal Additive Manufacturing: A Review. J. Mater. Eng. Perform. 2014, 23, 1917–1928. [Google Scholar] [CrossRef]
- Khairallah, S.A.; Anderson, A.T.; Rubenchik, A.; King, W.E. Laser powder-bed fusion additive manufacturing: Physics of complex melt flow and formation mechanisms of pores, spatter, and denudation zones. Acta Mater. 2016, 108, 36–45. [Google Scholar] [CrossRef] [Green Version]
- Li, L.; Lough, C.; Replogle, A.; Bristow, D.; Landers, R.; Kinzel, E. Thermal Modeling of 304L Stainless Steel Selective Laser Melting. In Proceedings of the 28th Annual International Solid Freeform Fabrication Symposium—An Additive Manufacturing Conference, Austin, TX, USA, 7–9 August 2017; pp. 1068–1081. [Google Scholar]
- Pham, M.-S.; Dovgyy, B.; Hooper, P. Twinning induced plasticity in austenitic stainless steel 316L made by additive manufacturing. Mater. Sci. Eng. A 2017, 704, 102–111. [Google Scholar] [CrossRef]
- Guo, P.; Zou, B.; Huang, C.; Gao, H. Study on microstructure, mechanical properties and machinability of efficiently additive manufactured AISI 316L stainless steel by high-power direct laser deposition. J. Mater. Process. Technol. 2017, 240, 12–22. [Google Scholar] [CrossRef]
- Vilaro, T.; Colin, C.; Bartout, J.-D. As-Fabricated and Heat-Treated Microstructures of the Ti-6Al-4V Alloy Processed by Selective Laser Melting. Met. Mater. Trans. A 2011, 42, 3190–3199. [Google Scholar] [CrossRef]
- Thijs, L.; Verhaeghe, F.; Craeghs, T.; van Humbeeck, J.; Kruth, J.-P. A study of the microstructural evolution during selective laser melting of Ti–6Al–4V. Acta Mater. 2010, 58, 3303–3312. [Google Scholar] [CrossRef]
- Köhnen, P.; Haase, C.; Bültmann, J.; Ziegler, S.; Schleifenbaum, J.H.; Bleck, W. Mechanical properties and deformation behavior of additively manufactured lattice structures of stainless steel. Mater. Des. 2018, 145, 205–217. [Google Scholar] [CrossRef]
- Wen, S.F.; Li, S.; Wei, Q.S.; Yan, C.Z.; Zhang, S.; Shi, Y.S. Effect of molten pool boundaries on the mechanical properties of selective laser melting parts. J. Mater. Process. Technol. 2014, 214, 2660–2667. [Google Scholar] [CrossRef]
- Wang, D.; Song, C.; Yang, Y.; Bai, Y. Investigation of crystal growth mechanism during selective laser melting and mechanical property characterization of 316L stainless steel parts. Mater. Des. 2016, 100, 291–299. [Google Scholar] [CrossRef]
- Wang, X.; Keya, T.; Chou, K. Build Height Effect on the Inconel 718 Parts Fabricated by Selective Laser Melting. Procedia Manuf. 2016, 5, 1006–1017. [Google Scholar] [CrossRef] [Green Version]
- Kok, Y.; Tan, X.P.; Wang, P.; Nai, M.L.S.; Loh, N.H.; Liu, E.; Tor, S.B. Anisotropy and heterogeneity of microstructure and mechanical properties in metal additive manufacturing: A critical review. Mater. Des. 2018, 139, 565–586. [Google Scholar] [CrossRef]
- DebRoy, T.; Wei, H.L.; Zuback, J.S.; Mukherjee, T.; Elmer, J.W.; Milewski, J.O.; Beese, A.M.; Wilson-Heid, A.; De, A.; Zhang, W. Additive manufacturing of metallic components—Process, structure and properties. Prog. Mater. Sci. 2018, 92, 112–224. [Google Scholar] [CrossRef]
- Carroll, B.E.; Palmer, T.A.; Beese, A. Anisotropic tensile behavior of Ti–6Al–4V components fabricated with directed energy deposition additive manufacturing. Acta Mater. 2015, 87, 309–320. [Google Scholar] [CrossRef]
- Maamoun, A.H.; Elbestawi, M.; Dosbaeva, G.K.; Veldhuis, S.C. Thermal post-processing of AlSi10Mg parts produced by Selective Laser Melting using recycled powder. Addit. Manuf. 2018, 21, 234–247. [Google Scholar] [CrossRef]
- Liverani, E.; Toschi, S.; Ceschini, L.; Fortunato, A. Effect of selective laser melting (SLM) process parameters on micro-structure and mechanical properties of 316L austenitic stainless steel. J. Mater. Process. Technol. 2017, 249, 255–263. [Google Scholar] [CrossRef]
- Wang, Z.; Palmer, T.A.; Beese, A. Effect of processing parameters on microstructure and tensile properties of austenitic stainless steel 304L made by directed energy deposition additive manufacturing. Acta Mater. 2016, 110, 226–235. [Google Scholar] [CrossRef] [Green Version]
- Xiong, Z.H.; Liu, S.L.; Li, S.F.; Shi, Y.; Yang, Y.F.; Misra, R.D.K. Role of melt pool boundary condition in determining the mechanical properties of selective laser melting AlSi10Mg alloy. Mater. Sci. Eng. A 2019, 740–741, 148–156. [Google Scholar] [CrossRef]
- Wang, Y.M.; Voisin, T.; McKeown, J.; Ye, J.; Calta, N.; Li, Z.; Zeng, Z.; Zhang, Y.; Chen, W.; Roehling, T.T.; et al. Additively manufactured hierarchical stainless steels with high strength and ductility. Nat. Mater. 2018, 17, 63–71. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Mower, T.M.; Long, M.J. Mechanical behavior of additive manufactured, powder-bed laser-fused materials. Mater. Sci. Eng. A 2016, 651, 198–213. [Google Scholar] [CrossRef]
- Fashanu, O.; Buchely, M.F.; Spratt, M.; Newkirk, J.; Chandrashekhara, K.; Misak, H.; Walker, M. Effect of SLM Build Parameters on the Compressive Properties of 304L Stainless Steel. J. Manuf. Mater. Process. 2019, 3, 43. [Google Scholar] [CrossRef] [Green Version]
- ASM Aerospace Specification Metals Inc, “AISI Type 304L Stainless Steel,” ASM Material Data Sheet. Available online: http://asm.matweb.com/search/SpecificMaterial.asp?bassnum=MQ304L (accessed on 3 January 2019).









| Parameter | Value |
|---|---|
| Fill pattern | Stripes |
| Layer thickness | 50 [μm] |
| Inert gas during manufacturing | Argon |
| Laser type | 1070 nm NdYAG |
| Laser spot | 70 |
| Laser power | 200 [W] |
| Effective build volume | 248 × 248 × 280 [mm × mm × mm] |
| Hatch distance | 0.085 [mm] |
| Exposure time | 75 [μs] |
| Point distance | 60 [μm] |
| Sample | (100) | (010) | (001) | (011) |
|---|---|---|---|---|
| 0° | 0°–[010]–90° | 0°–[100]–90° | 0°–[100]–0° | 0°–[010]–45° |
| 67° | 67°–[010]–90° | 67°–[100]–90° | 67°–[100]–0° | 67°–[010]–45° |
| 105° | 105°–[010]–90° | 105°–[100]–90° | 105°–[100]–0° | 105°–[010]–45° |
| Hatch Angle–Rotation Axis–Rotation Angle (Plane Normal to Compression Direction) | Aspect Ratio | 0.2% Offset Yield Strength (MPa) |
|---|---|---|
| 0°–[010]–90° (100) | 1.22 ± 0.008 | 437 ± 4.70 |
| 0°–[100]–90° (010) | 1.53 ± 0.052 | 489 ± 9.94 |
| 0°–[100]–0° (001) | 1.65 ± 0.022 | 439 ± 7.94 |
| 0°–[010]–45° (011) | 1.07 ± 0.026 | 413 ± 9.26 |
| 67°–[010]–90° (100) | 1.18 ± 0.025 | 492 ± 2.00 |
| 67°–[100]–90° (010) | 1.10 ± 0.042 | 489 ± 5.08 |
| 67°–[100]–0° (001) | 1.04 ± 0.015 | 473 ± 11.6 |
| 67°–[010]–45° (011) | 1.05 ± 0.012 | 491 ± 9.89 |
| 105°–[010]–90° (100) | 1.04 ± 0.019 | 544 ± 10.7 |
| 105°–[100]–90° (010) | 1.03 ± 0.007 | 516 ± 14.24 |
| 105°–[100]–0° (001) | 1.06 ± 0.023 | 498 ± 3.72 |
| 105°–[010]–45° (011) | 1.10 ± 0.022 | 485 ± 2.08 |
| Hatch Angle | Direction | Percent Maximum [MPBN] | Percent Minimum [MPBN] | Difference between Maximum and Minimum |
|---|---|---|---|---|
| 0° | [100] | 68% | 9% | 59% |
| 0° | [001] | 44% | 21% | 23% |
| 0° | [010] | 31% | 18% | 13% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Spratt, M.; Newkirk, J.W.; Fashanu, O.; Chandrashekhara, K. Effect of the Melt Pool Boundary Network on the Anisotropic Mechanical Properties of Selective Laser Melted 304L. J. Manuf. Mater. Process. 2021, 5, 110. https://doi.org/10.3390/jmmp5040110
Spratt M, Newkirk JW, Fashanu O, Chandrashekhara K. Effect of the Melt Pool Boundary Network on the Anisotropic Mechanical Properties of Selective Laser Melted 304L. Journal of Manufacturing and Materials Processing. 2021; 5(4):110. https://doi.org/10.3390/jmmp5040110
Chicago/Turabian StyleSpratt, Myranda, Joseph W. Newkirk, Okanmisope Fashanu, and K. Chandrashekhara. 2021. "Effect of the Melt Pool Boundary Network on the Anisotropic Mechanical Properties of Selective Laser Melted 304L" Journal of Manufacturing and Materials Processing 5, no. 4: 110. https://doi.org/10.3390/jmmp5040110
APA StyleSpratt, M., Newkirk, J. W., Fashanu, O., & Chandrashekhara, K. (2021). Effect of the Melt Pool Boundary Network on the Anisotropic Mechanical Properties of Selective Laser Melted 304L. Journal of Manufacturing and Materials Processing, 5(4), 110. https://doi.org/10.3390/jmmp5040110






