Numerical-Experimental Plastic-Damage Characterisation of Additively Manufactured 18Ni300 Maraging Steel by Means of Multiaxial Double-Notched Specimens
Abstract
:1. Introduction
2. Materials and Methods
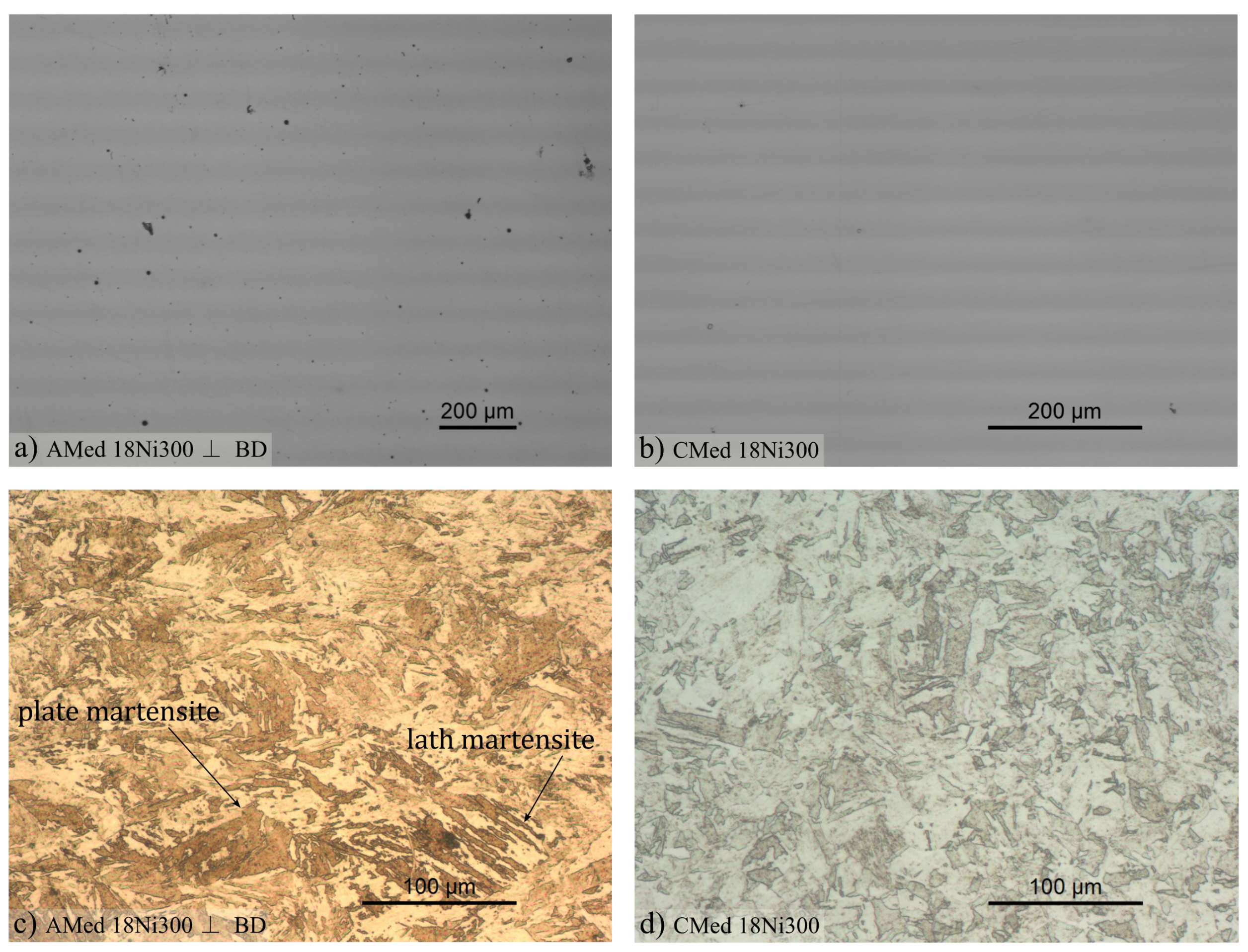
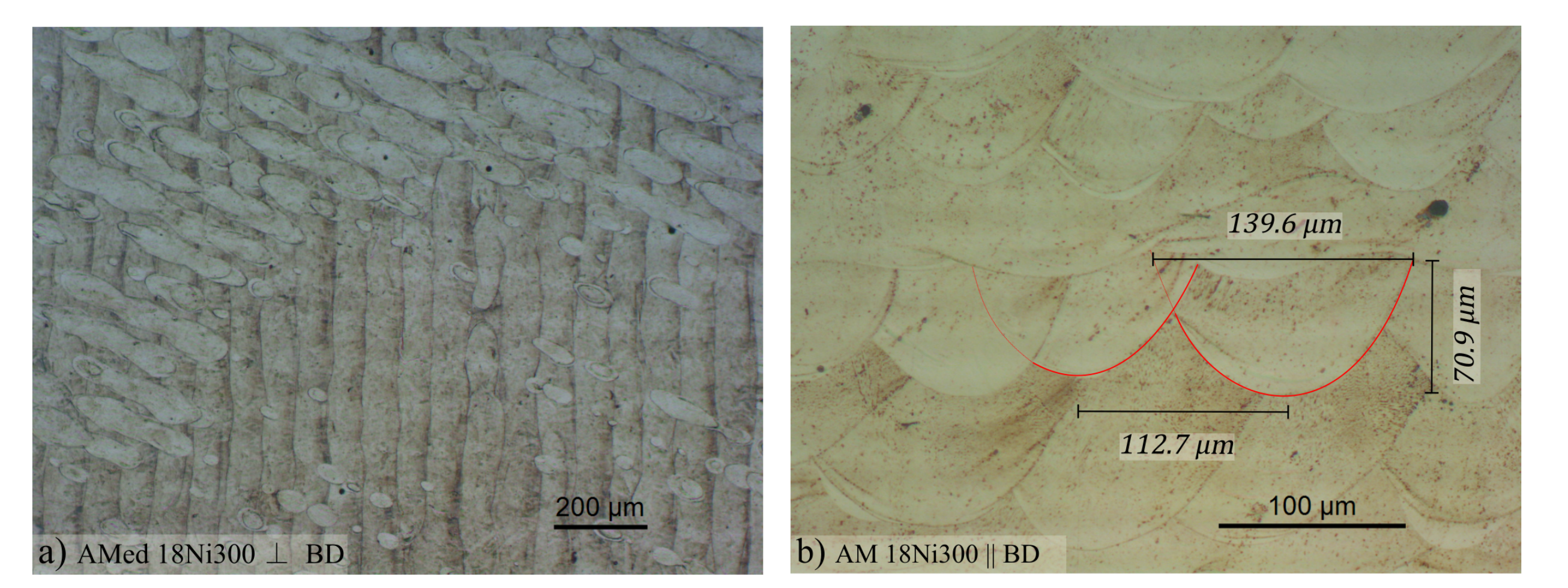
2.1. Additively Manufactured 18Ni300
2.2. Compression of Multiaxial Double-Notched Specimens
2.3. Smooth and Notched Tensile Specimens
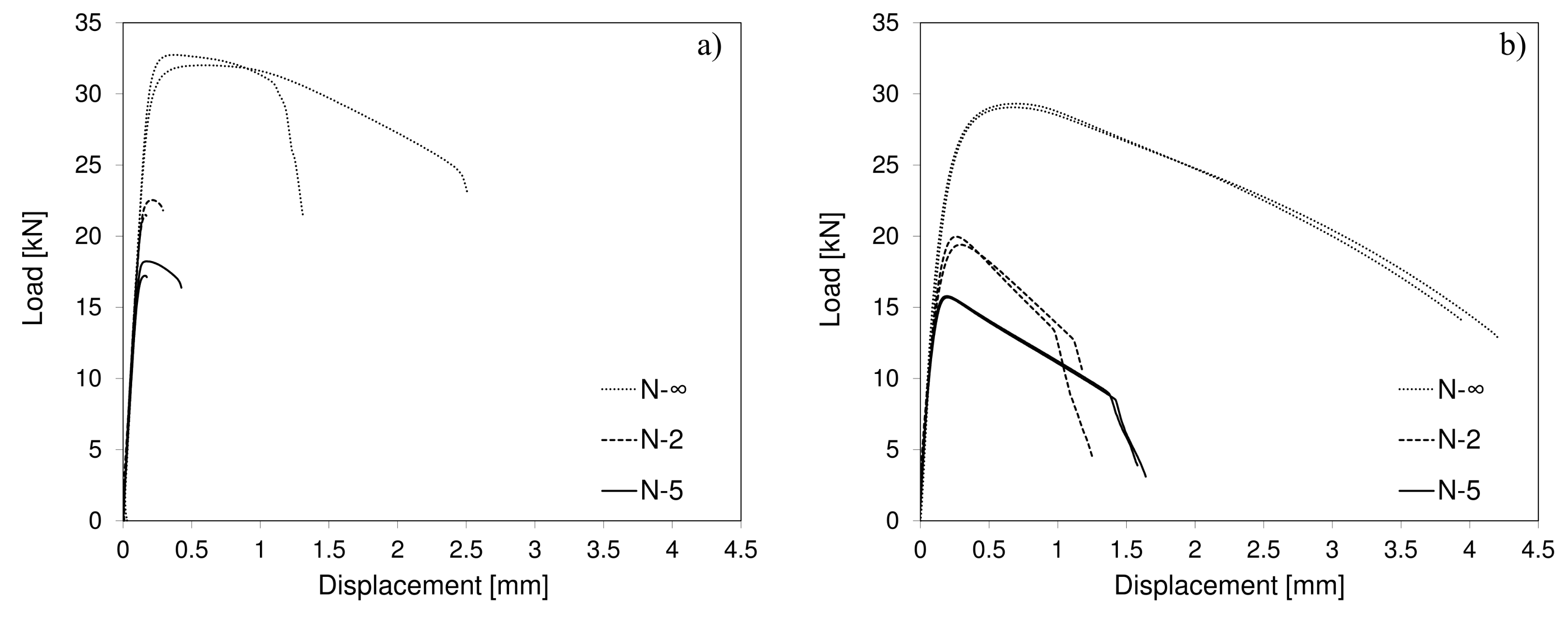
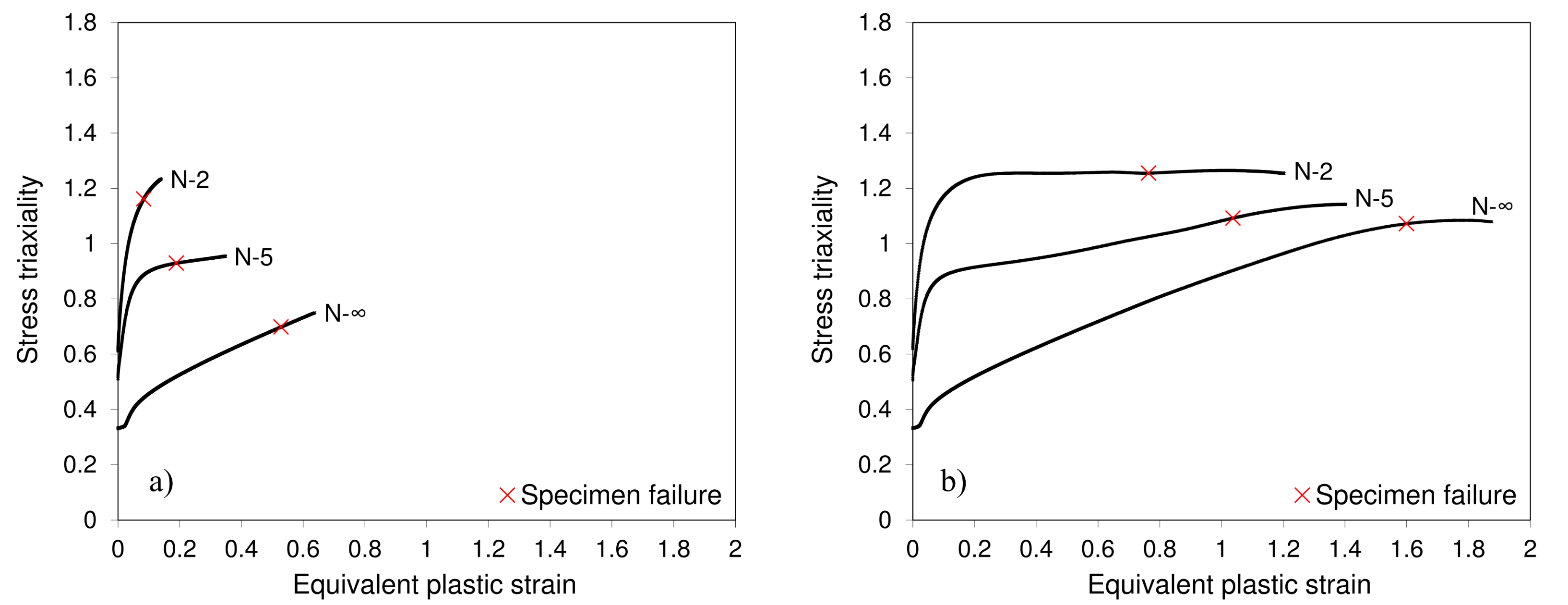
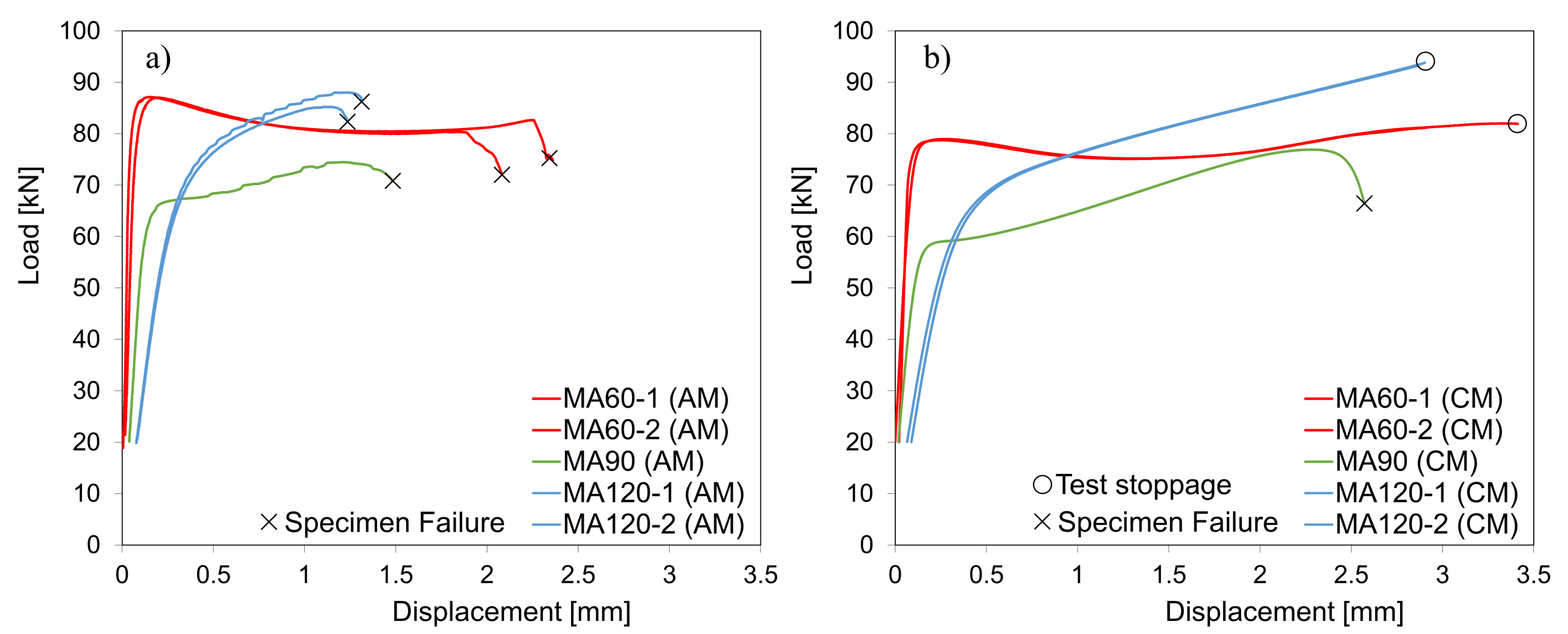
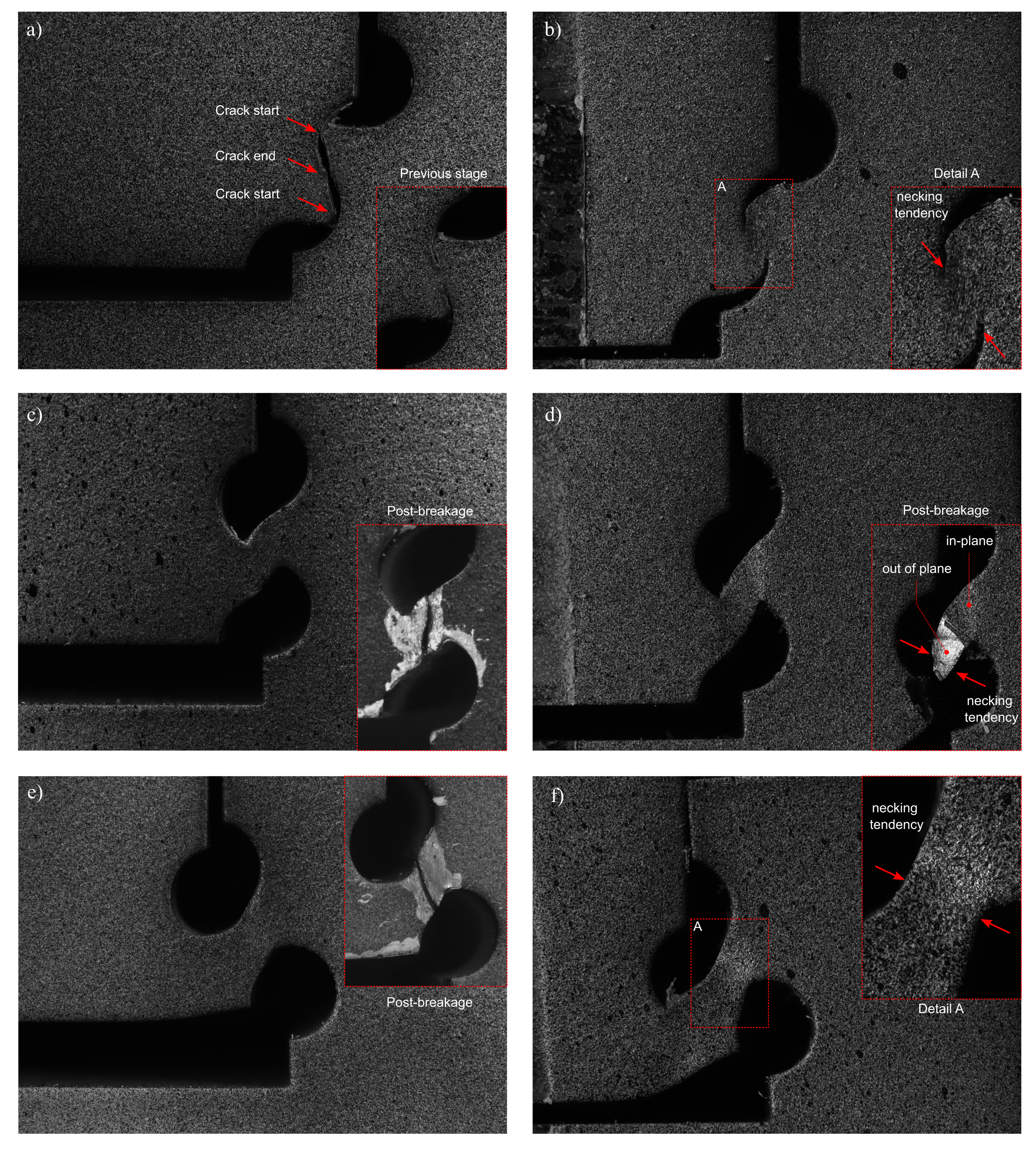
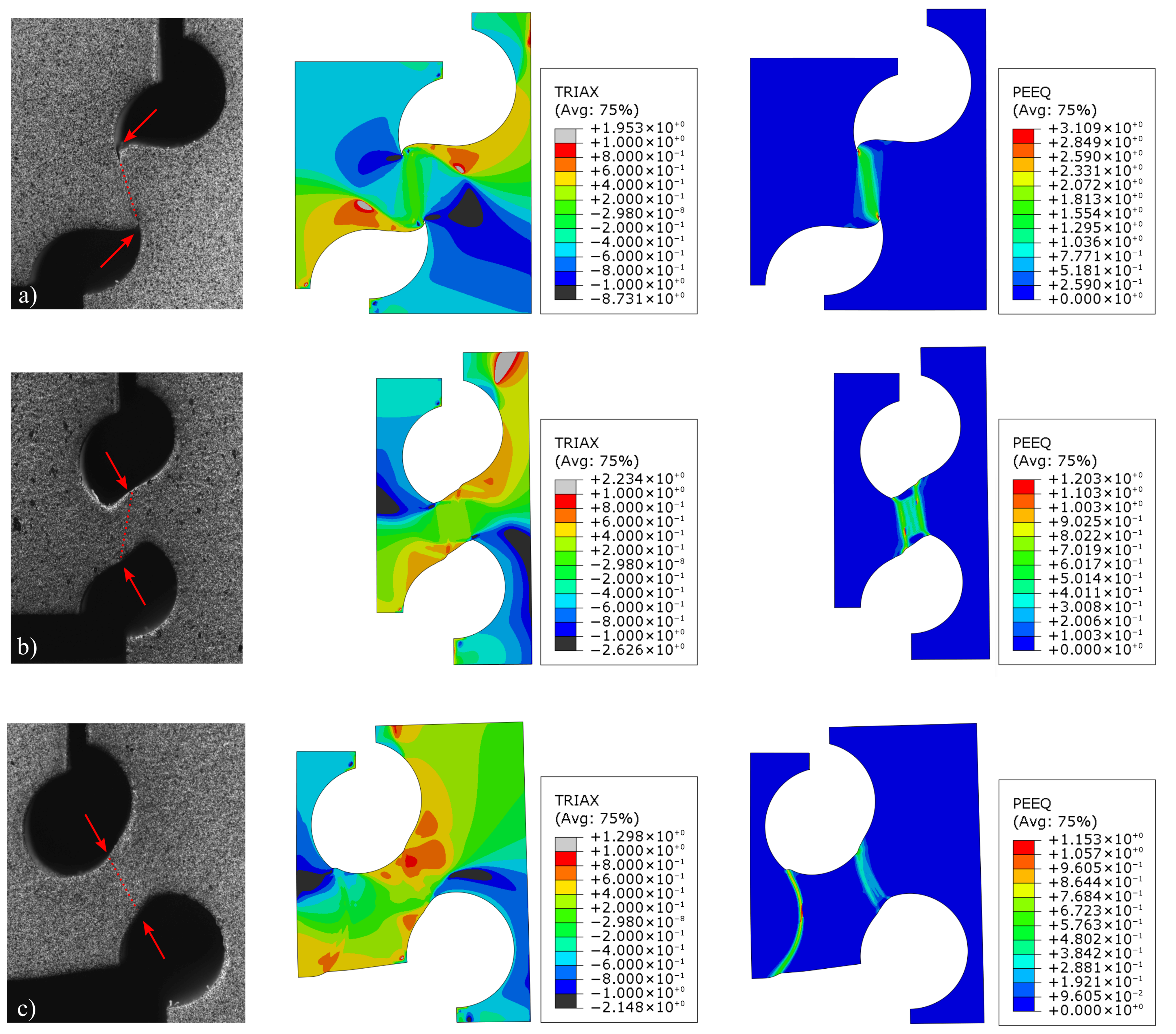
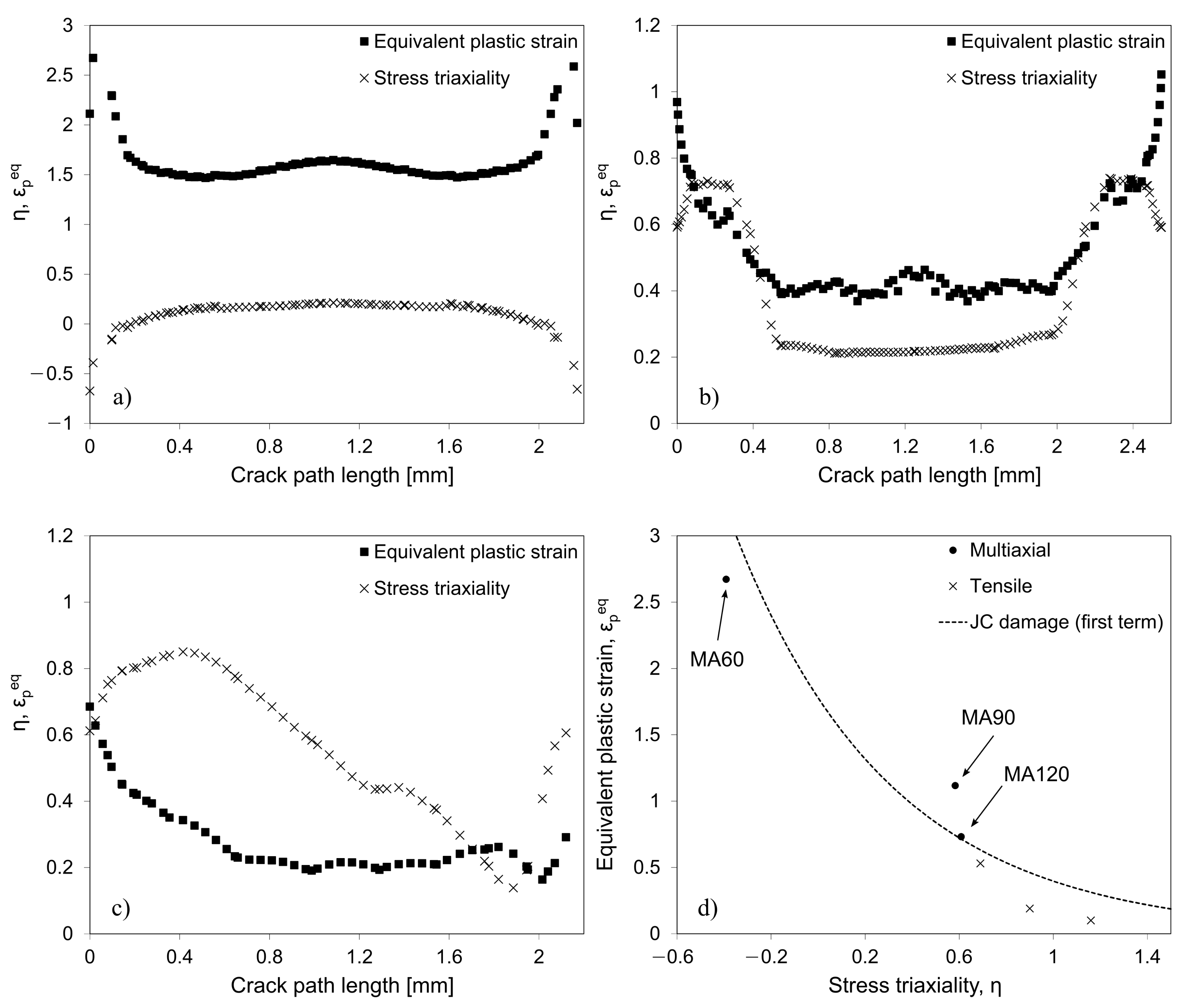
3. Results and Discussion
4. Conclusions
- -
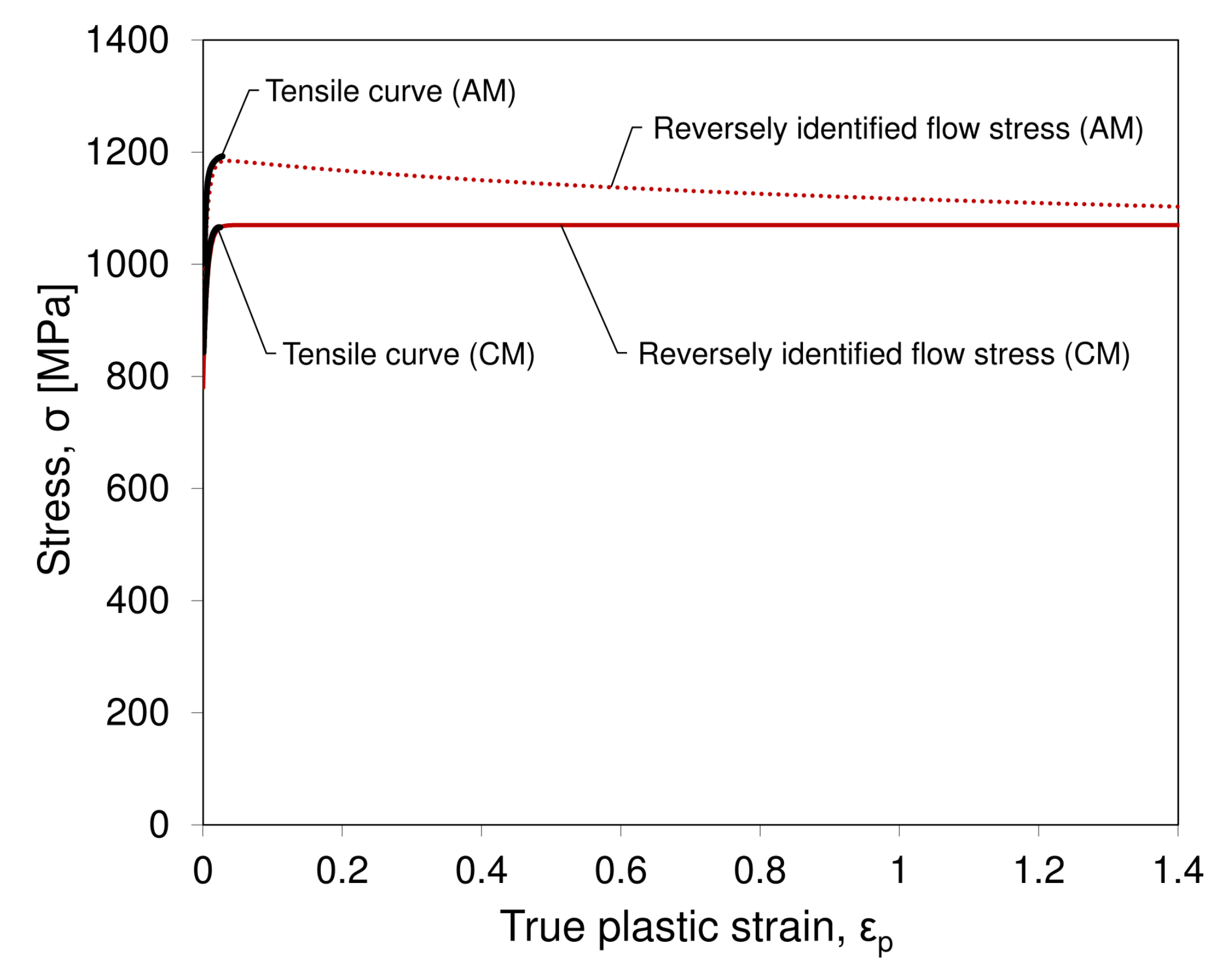
- Tensile tests showed the higher ductility of the CMed maraging steel compared to the AMed ones. A significant amount of diffuse necking seems to occur in both AMed and CMed maraging steels, which accounts for incipient geometrical softening in tensile conditions.
- -
- The multiaxial double-notched tests confirmed the higher mechanical strength of the AMed metallurgical condition as well as the increased ductility of the conventional maraging steel. In addition, the diffuse necking tendency along with the high ductility of the latter material precluded its fracture characterisation, revealing the inadequacy in selecting the same double-notched geometry towards fracture strain identification, on materials with distinct strength–ductility ratios.
- -
- Even though they are often seen as fracture specimens, the double-notched geometry provided very important insight into material’s plasticity and flow stress inverse identification, constituting a valuable alternative to the typical mechanical characterisation methodology that also presents widely known flaws (e.g., friction in compression tests and plastic instability in tensile tests).
- -
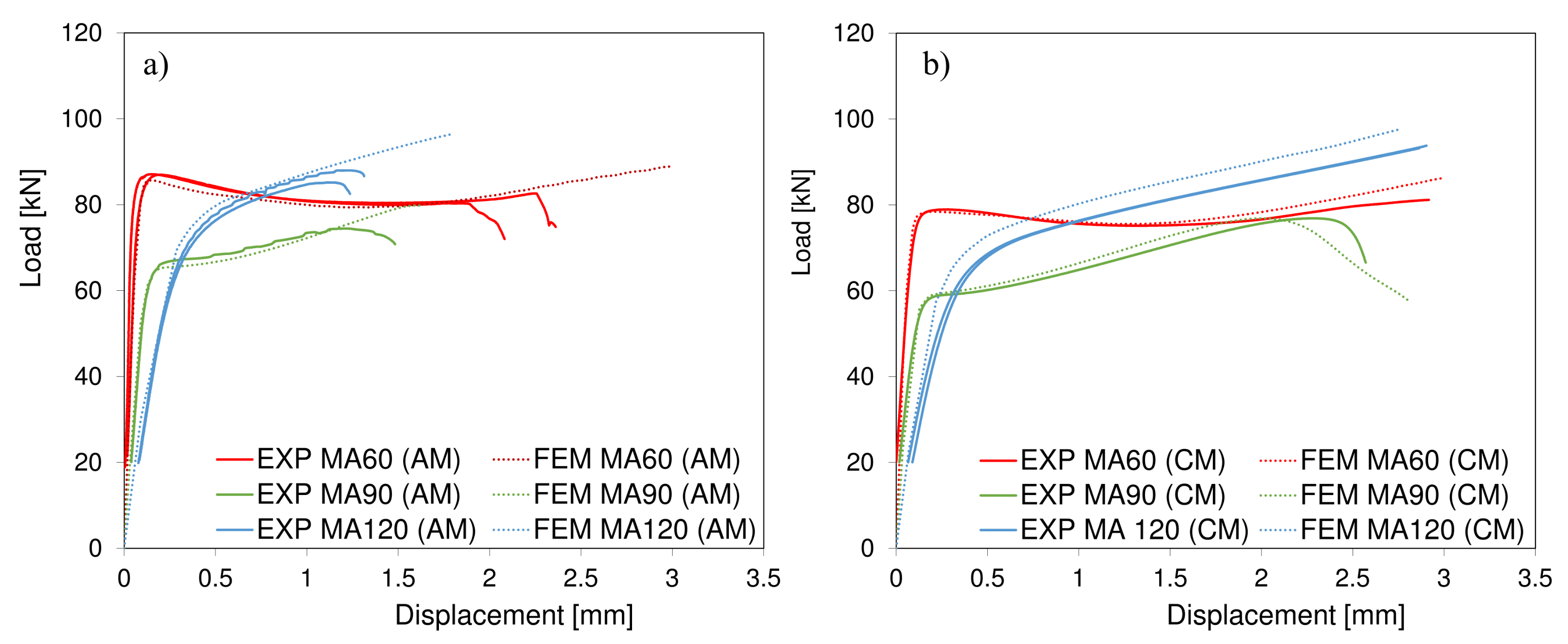
- The multiaxial double-notched tests were revealed to be very useful for calibrating the constitutive flow stress behaviour of the AMed maraging steel in plane strain and combined shear–tension/compression conditions. These tests are a useful alternative to typical characterisation approaches (such as compression and tensile tests) which show some recognised limitations (friction and limited strain, respectively). They are also valuable for damage onset definition despite requiring a proper design to avoid large deformations that could lead to unwanted stress states. The capability of the test for evaluation of fracture energies is limited since its does not correspond to a true fracture test, since a significant amount of energy will precede the crack initiation.
- -
- The employed approach using the von Mises isotropic hardening as well as inverse definition of a flow stress with slight softening and uncoupled stress triaxiality sensitive damage initiation was revealed to suitably depict the mechanical response of the AMed 18Ni300 maraging steel under distinct scenarios of stress state (mixed compression and shear, theoretically pure shear and tensile and shear).
- -
- The usage of DIC-levelling approaches, in which the FEA data are processed through the same DIC engine as the experimental DIC data, allows for the mitigation of apparent strain errors, through minimisation of inconsistencies between FEA and DIC, namely the strain calculation algorithm, spatial resolution and data filtering [48]. The fact that such procedure seems to realistically simulate experimental heterogeneous deformations at various load steps [49] may bring a new light on the DIC results. Full-field data can be valuable to allow the search for the proper constitutive model solution in the apparent multiple solution problem.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Cunha, F.; Santos, T.; Xavier, J. In Situ Monitoring of Additive Manufacturing Using Digital Image Correlation: A Review. Materials 2021, 14, 1511. [Google Scholar] [CrossRef] [PubMed]
- Jiménez, A.; Bidare, P.; Hassanin, H.; Tarlochan, F.; Dimov, S.; Essa, K. Powder-based laser hybrid additive manufacturing of metals: A review. Int. J. Adv. Manuf. Technol. 2021, 114, 63–96. [Google Scholar] [CrossRef]
- Kumar, L.J.; Nair, C.G.K. Current trends of additive manufacturing in the aerospace industry. In Advances in 3D Printing and Additive Manufacturing Technologies; Wimpenny, D., Pandey, P., Kumar, L., Eds.; Springer: Singapore, 2017; pp. 39–54. [Google Scholar]
- Meng, L.; Zhang, W.; Quan, D.; Shi, G.; Tang, L.; Hou, Y.; Breitkopf, P.; Zhu, J.; Gao, T. From Topology Optimization Design to Additive Manufacturing: Today’s Success and Tomorrow’s Roadmap. Arch. Comput. Methods Eng. 2020, 27, 805–830. [Google Scholar] [CrossRef]
- Wang, X.; Xu, S.; Zhou, S.; Xu, W.; Leary, M.; Choong, P.; Qian, M.; Brandt, M.; Xie, Y.M. Topological design and additive manufacturing of porous metals for bone scaffolds and orthopaedic implants: A review. Biomaterials 2016, 83, 127–141. [Google Scholar] [CrossRef]
- Gebhardt, A.; Schmidt, F.; Hötter, J.; Sokalla, W.; Sokalla, P. Additive manufacturing by selective laser melting the realizer desktop machine and its application for the dental industry. Phys. Procedia 2010, 5, 543–549. [Google Scholar] [CrossRef] [Green Version]
- Mazur, M.; Leary, M.; McMillan, M.; Elambasseril, J.; Brandt, M. SLM additive manufacture of H13 tool steel with conformal cooling and structural lattices. Rapid Prototyp. J. 2016, 22, 504–518. [Google Scholar] [CrossRef]
- Khairallah, S.A.; Anderson, A.T.; Rubenchik, A.; King, W.E. Laser powder-bed fusion additive manufacturing: Physics of complex melt flow and formation mechanisms of pores, spatter, and denudation zones. Acta Mater. 2016, 108, 36–45. [Google Scholar] [CrossRef] [Green Version]
- Sames, W.J.; List, F.A.; Pannala, S.; Dehoff, R.R.; Babu, S.S. The metallurgy and processing science of metal additive manufacturing. Int. Mater. Rev. 2016, 61, 315–360. [Google Scholar] [CrossRef]
- Lewandowski, J.J.; Seifi, M. Metal Additive Manufacturing: A Review of Mechanical Properties. Annu. Rev. Mater. Res. 2016, 46, 151–186. [Google Scholar] [CrossRef] [Green Version]
- Haghdadi, N.; Laleh, M.; Moyle, M.; Primig, S. Additive manufacturing of steels: A review of achievements and challenges. J. Mater. Sci. 2021, 56, 64–107. [Google Scholar] [CrossRef]
- Yasa, E.; Kempen, K.; Kruth, J.; Thijs, L.; Van Humbeeck, J. Microstructure and mechanical properties of maraging steel 300 after selective laser melting. In Proceedings of the Solid Freeform Fabrication Symposium Proceedings, Austin, Texas, USA, 9–11 August 2010; pp. 383–396. [Google Scholar]
- Tan, C.; Zhou, K.; Kuang, M.; Ma, W.; Kuang, T. Microstructural characterization and properties of selective laser melted maraging steel with different build directions. Sci. Technol. Adv. Mater. 2018, 19, 746–758. [Google Scholar] [CrossRef] [Green Version]
- Albinmousa, J.; AlSadah, J.; Hawwa, M.; Al-Qahtani, H. Estimation of Mode I Fracture of U-Notched Polycarbonate Specimens Using the Equivalent Material Concept and Strain Energy Density. Appl. Sci. 2021, 11, 3370. [Google Scholar] [CrossRef]
- Toscano, D.; Behravesh, S.B.; Shaha, S.K.; Jahed, H.; Williams, B. Characterization of closed-die forged AZ31B under pure axial and pure shear loading. Int. J. Fatigue 2020, 139, 105754. [Google Scholar] [CrossRef]
- Shekarian, A.; Varvani-Farahani, A. Ratcheting Response of SS316 Steel Samples with Different Notch Shapes under Various Loading Spectra. J. Mater. Eng. Perform. 2021, 30, 3524–3535. [Google Scholar] [CrossRef]
- Cyr, E.; Lloyd, A.; Mohammadi, M. Tension-compression asymmetry of additively manufactured Maraging steel. J. Manuf. Process. 2018, 35, 289–294. [Google Scholar] [CrossRef]
- Spitzig, W.A.; Sober, R.J.; Richmond, O. The effect of hydrostatic pressure on the deformation behavior of maraging and HY-80 steels and its implications for plasticity theory. Metall. Trans. A 1976, 7, 1703–1710. [Google Scholar] [CrossRef]
- Bai, Y.; Wierzbicki, T. A new model of metal plasticity and fracture with pressure and Lode dependence. Int. J. Plast. 2008, 24, 1071–1096. [Google Scholar] [CrossRef]
- Ferreira, I.; Jesus, A.; Machado, M.; Pereira, J.; Alves, J.L.; Xavier, J. Experimental characterisation of fused filament fabrication printed parts under tension, shear, and combined shear–tension loads via Arcan test. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2020, 234, 835–850. [Google Scholar] [CrossRef]
- Arcan, M.; Hashin, Z.; Voloshin, A. A method to produce uniform plane-stress states with applications to fiber-reinforced materials. Exp. Mech. 1978, 18, 141–146. [Google Scholar] [CrossRef]
- Xavier, J.; Oliveira, M.; Morais, J.; Pinto, J. Measurement of the shear properties of clear wood by the Arcan test. Holzforschung 2009, 63, 217–225. [Google Scholar] [CrossRef]
- Xavier, J.; Morais, J.; Pereira, F. Non-linear shear behaviour of bovine cortical bone by coupling the Arcan test with digital image correlation. Opt. Lasers Eng. 2018, 110, 462–470. [Google Scholar] [CrossRef]
- Abushawashi, Y.M. Modeling of Metal Cutting as Purposeful Fracture of Work Material. Ph.D. Thesis, Michigan State University, East Lansing, MI, USA, 2013; p. 243. [Google Scholar]
- Würzinger, P.; Rabitsch, R.; Meyer, W. Production of maraging steel grades and the influence of specified and nonspecified elements for special applications. J. Mater. Sci. 2004, 39, 7295–7302. [Google Scholar] [CrossRef]
- Stull, J.A.; Hill, M.A.; Lienert, T.J.; Tokash, J.; Bohn, K.R.; Hooks, D.E. Corrosion characteristics of laser-engineered net shaping additively-manufactured 316L stainless steel. J. Miner. Met. Mater. Soc. 2018, 70, 2677–2683. [Google Scholar] [CrossRef]
- Lang, F.H.; Kenyon, N. Welding of Maraging Steels; Welding Research Council: New York, NY, USA, 1971. [Google Scholar]
- Król, M.; Snopiński, P.; Czech, A. The phase transitions in selective laser-melted 18-NI (300-grade) maraging steel. J. Therm. Anal. Calorim. 2020, 142, 1011–1018. [Google Scholar] [CrossRef] [Green Version]
- MIL-S-46850D. Steel: Bar, Plate, Sheet, Strip, Forgings and Extrusions—18 Percent Ni Alloy Maraging 200 KSI, 250 KSI and 300 KSI; US Military Standards: Washington, DC, USA, 1986. [Google Scholar]
- Spierings, A.B.; Schneider, M.; Eggenberger, R. Comparison of density measurement techniques for additive manufactured metallic parts. Rapid Prototyp. J. 2011, 17, 380–386. [Google Scholar] [CrossRef]
- Grédiac, M.; Hild, F.; Pineau, A. Full-Field Measurements and Identification in Solid Mechanics; John Wiley and Sons: London, UK, 2012. [Google Scholar]
- Sutton, M.; Orteu, J.J.; Schreier, H. Image Correlation for Shape, Motion and Deformation Measurements: Basic Concepts, Theory and Applications; Springer: New York, NY, USA, 2009. [Google Scholar]
- Silva, A.; de Jesus, A.; Xavier, J.; Correia, J.; Fernandes, A. Combined analytical-numerical methodologies for the evaluation of mixed-mode (I+II) fatigue crack growth rates in structural steels. Eng. Fract. Mech. 2017, 185, 124–138. [Google Scholar] [CrossRef]
- Xavier, J.; de Jesus, A.; Morais, J.; Pinto, J. Stereovision measurements on evaluating the modulus of elasticity of wood by compression tests parallel to the grain. Constr. Build. Mater. 2012, 26, 207–215. [Google Scholar] [CrossRef]
- Pereira, J.; Xavier, J.; Ghiassi, B.; Lousada, J.; Morais, J. On the identification of earlywood and latewood radial elastic modulus of Pinus pinaster by digital image correlation: A parametric analysis. J. Strain Anal. Eng. Des. 2018, 53, 566–574. [Google Scholar] [CrossRef]
- Bridgman, P.W. Studies in Large Plastic Flow and Fracture; McGraw-Hill: New York, NY, USA, 1952; p. 362. [Google Scholar]
- Wierzbicki, T.; Bao, Y.; Lee, Y.W.; Bai, Y. Calibration and evaluation of seven fracture models. Int. J. Mech. Sci. 2005, 47, 719–743. [Google Scholar] [CrossRef]
- Yokota, M.J.; Lai, G.Y. Toughness of Lath Versus Plate Martensites. Metall. Trans. A 1974, 6, 1832–1835. [Google Scholar] [CrossRef]
- Kelly, P.M.; Nutting, J. The martensite transformation in carbon steels. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1961, 259, 45–58. [Google Scholar]
- Kim, F.H.; Moylan, S.P.; Phan, T.Q.; Garboczi, E.J. Investigation of the Effect of Artificial Internal Defects on the Tensile Behavior of Laser Powder Bed Fusion 17–4 Stainless Steel Samples: Simultaneous Tensile Testing and X-ray Computed Tomography. Exp. Mech. 2020, 60, 987–1004. [Google Scholar] [CrossRef]
- Leça, T.C.; Silva, T.E.F.; de Jesus, A.M.P.; Neto, R.L.; Alves, J.L.; Pereira, J.P. Influence of multiple scan fields on the processing of 316L stainless steel using laser powder bed fusion. Proc. Inst. Mech. Eng. Part L J. Mater. Des. Appl. 2020, 235, 19–41. [Google Scholar] [CrossRef]
- Semiatin, S.L.; Jonas, J.J. Formability and Workability of Metals: Plastic Instability and Flow Localization; ASM International: Novelty, OH, USA, 1984. [Google Scholar]
- Hwang, J.K. Revealing the small post-necking elongation in twinning-induced plasticity steels. J. Mater. Sci. 2020, 55, 8285–8302. [Google Scholar] [CrossRef]
- Sakaki, T.; Sugimoto, K.; Miyagawa, O. Strain Hardening and Uniform Elongation of 1700MPa Grade Maraging Steel. Trans. Iron Steel Inst. Japan 1986, 26, 219–227. [Google Scholar] [CrossRef]
- Voestalpine. Product Description: BOHLER W720 VMR. 2021. Available online: https://www.bohler-edelstahl.com/en/products/w720-vmr/ (accessed on 2 August 2021).
- Bluhm, J.I.; Morrissey, R.J. Fracture in a Tensile Specimen; Technical Report; Army Materials Research Agency: Watertown, MA, USA, 1966. [Google Scholar]
- Shamsdini, S.; Ghoncheh, M.H.; Sanjari, M.; Pirgazi, H.; Amirkhiz, B.S.; Kestens, L.; Mohammadi, M. Plastic deformation throughout strain-induced phase transformation in additively manufactured maraging steels. Mater. Des. 2021, 198, 109289. [Google Scholar] [CrossRef]
- Lava, P.; Jones, E.M.C.; Wittevrongel, L.; Pierron, F. Validation of finite-element models using full-field experimental data: Levelling finite-element analysis data through a digital image correlation engine. Strain 2020, 56, e12350. [Google Scholar] [CrossRef]
- Lava, P.; Cooreman, S.; Coppieters, S.; De Strycker, M.; Debruyne, D. Assessment of measuring errors in DIC using deformation fields generated by plastic FEA. Opt. Lasers Eng. 2009, 47, 747–753. [Google Scholar] [CrossRef]
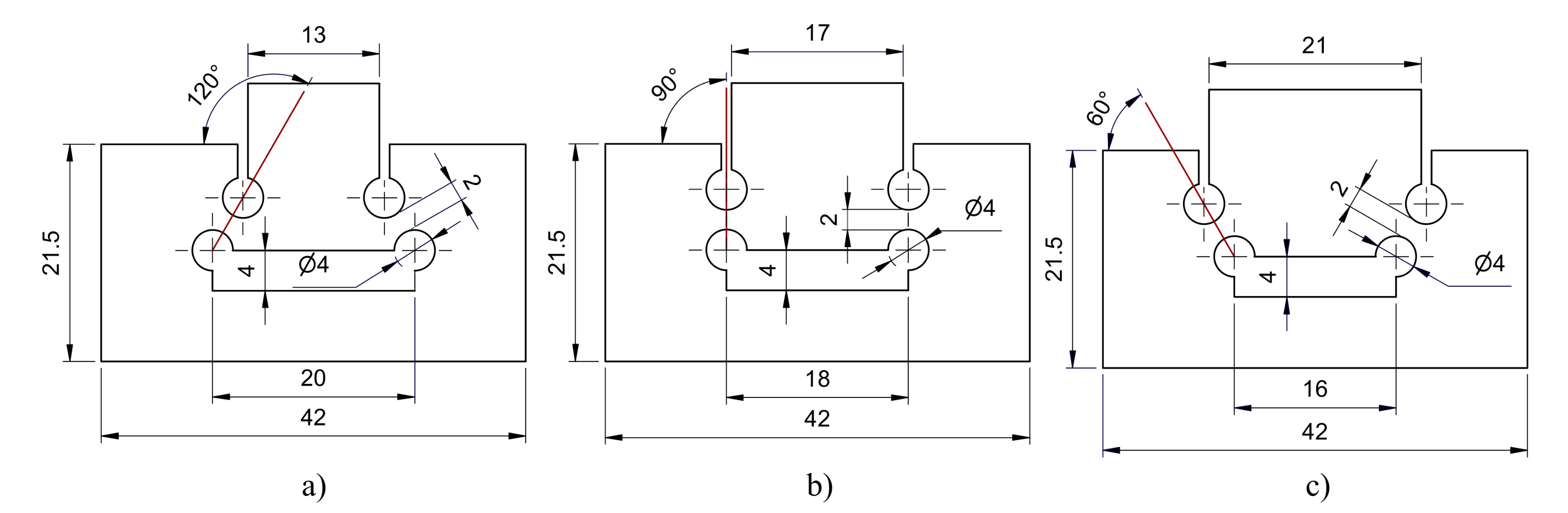

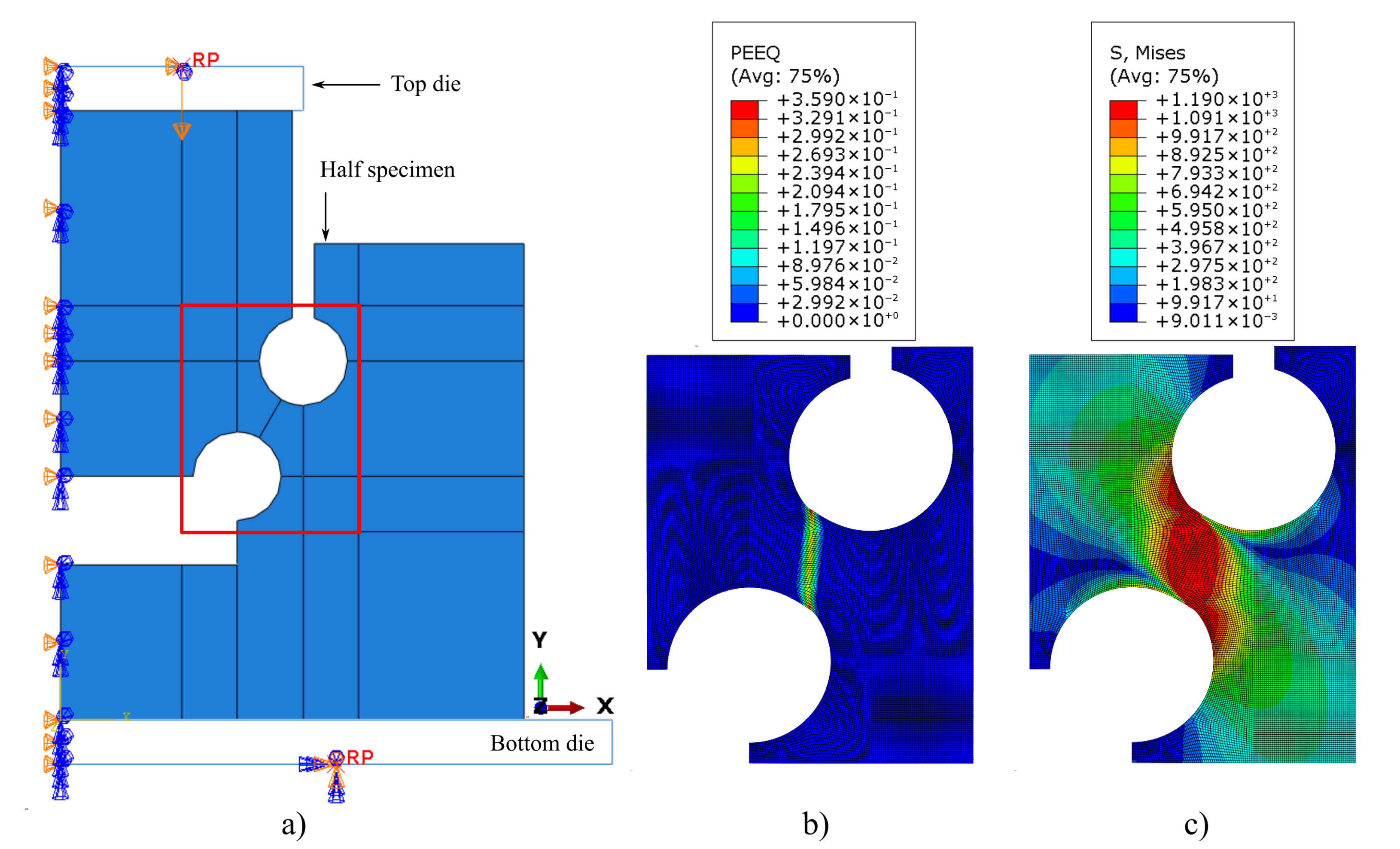
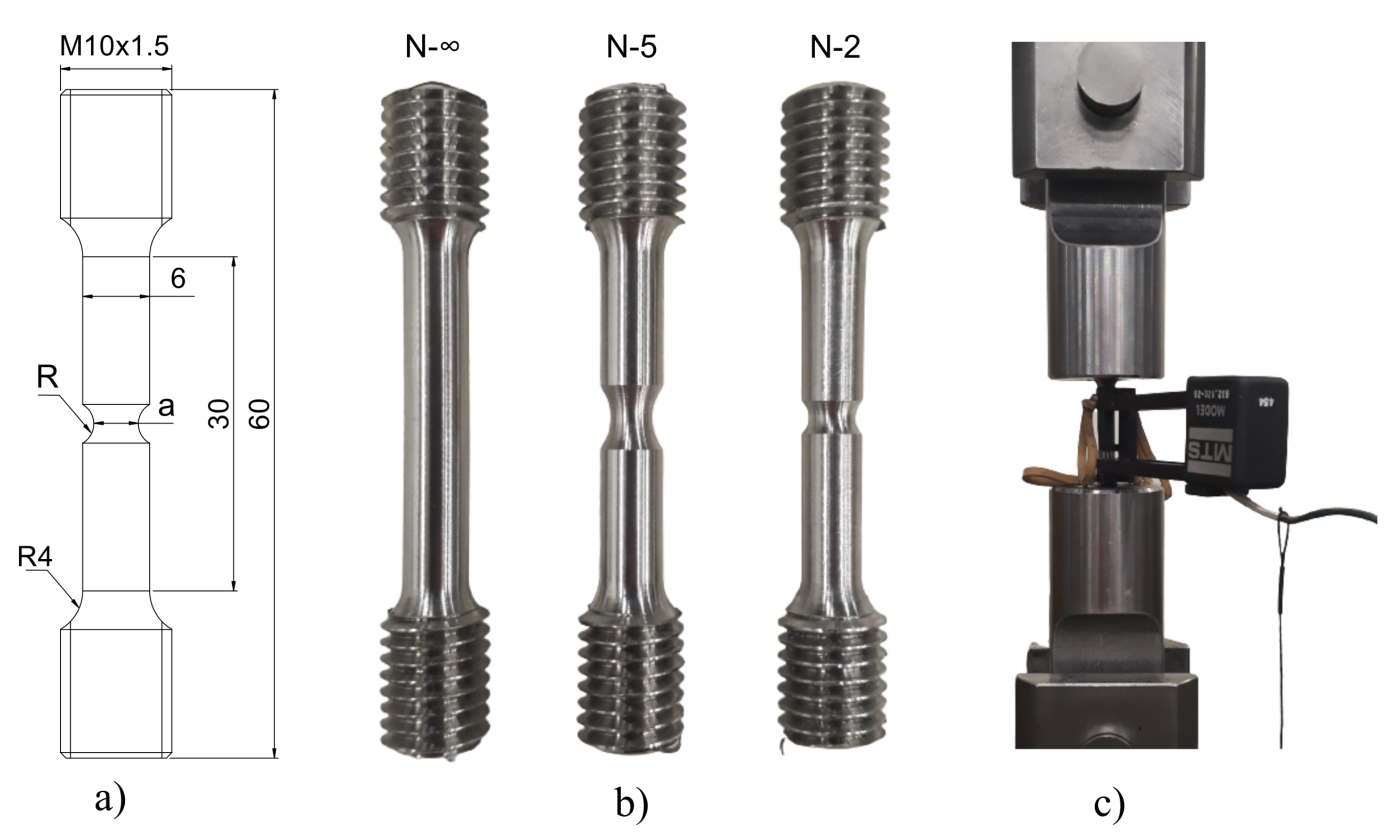
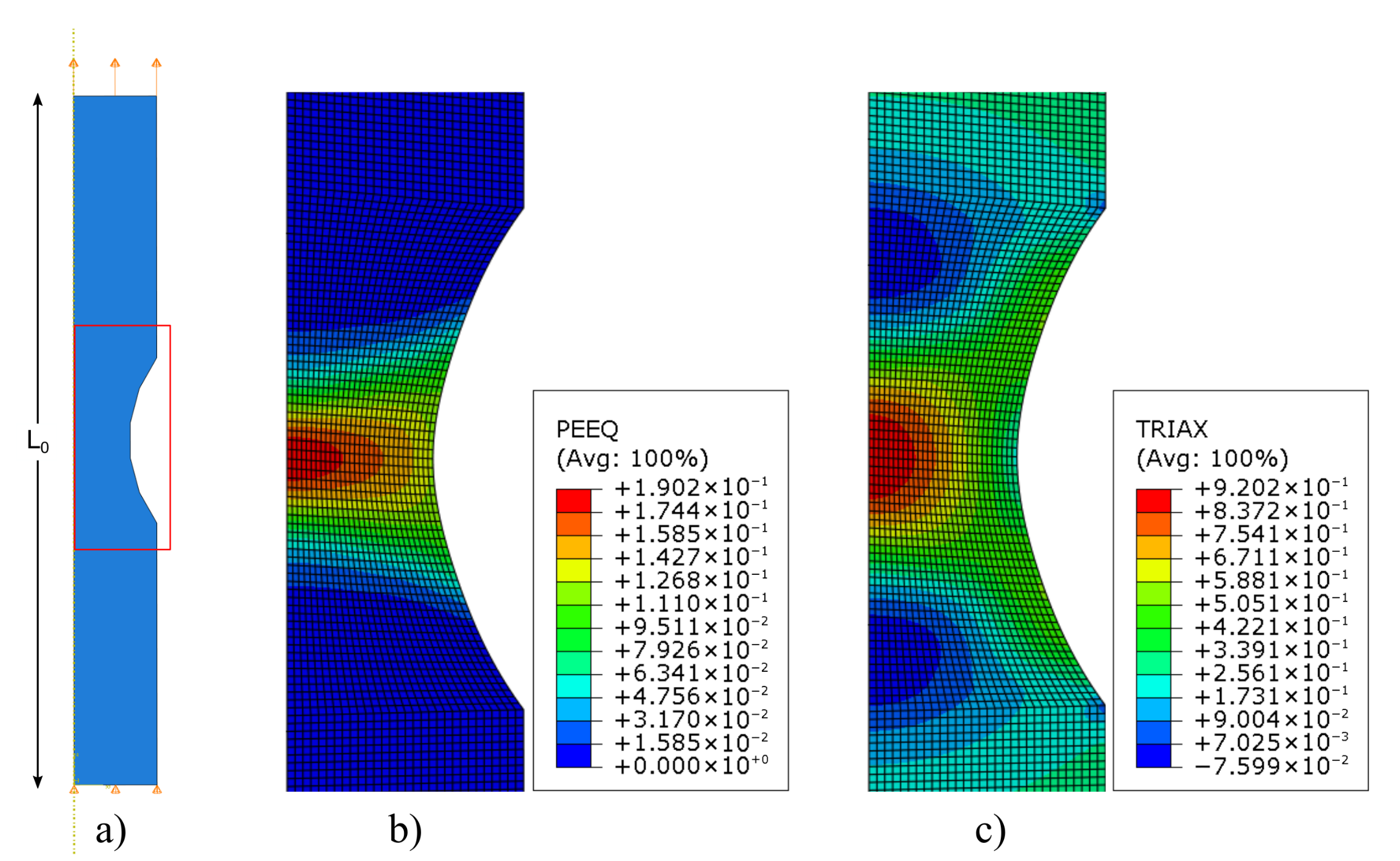



| Ni | Co | Mo | Ti | Si | Mn | C | P | S | |
|---|---|---|---|---|---|---|---|---|---|
| [29] | 18.0–19.0 | 8.5–9.5 | 4.6–5.2 | 0.5–0.8 | <0.10 | <0.10 | <0.03 | <0.01 | <0.01 |
| AM | 18.80 | 8.84 | 5.15 | 0.65 | 0.05 | 0.03 | 0.02 | <0.001 | <0.001 |
| CM | 18.93 | 8.92 | 4.88 | 0.77 | 0.02 | 0.03 | 0.01 | <0.001 | <0.001 |
| Tested Configurations | N-∞ | N-5 | N-2 |
|---|---|---|---|
| a (refer to Figure 4a) | 6 | 6 | 4 |
| R (refer to Figure 4a) | ∞ | 5 | 2 |
| 0.33 | 0.52 | 0.74 |
| Material | K [MPa] | n | [MPa] | Q [MPa] | |||
|---|---|---|---|---|---|---|---|
| AM | 950 | 1 | −0.7 | 887.5 | 362.5 | 170 | 0.2 |
| CM | 950 | 1 | 0 | 887.5 | 362.5 | 170 | 0.2 |
| Material | |||
|---|---|---|---|
| AM | −0.01 | 1.77 | −1.5 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Silva, T.; Gregório, A.; Silva, F.; Xavier, J.; Reis, A.; Rosa, P.; de Jesus, A. Numerical-Experimental Plastic-Damage Characterisation of Additively Manufactured 18Ni300 Maraging Steel by Means of Multiaxial Double-Notched Specimens. J. Manuf. Mater. Process. 2021, 5, 84. https://doi.org/10.3390/jmmp5030084
Silva T, Gregório A, Silva F, Xavier J, Reis A, Rosa P, de Jesus A. Numerical-Experimental Plastic-Damage Characterisation of Additively Manufactured 18Ni300 Maraging Steel by Means of Multiaxial Double-Notched Specimens. Journal of Manufacturing and Materials Processing. 2021; 5(3):84. https://doi.org/10.3390/jmmp5030084
Chicago/Turabian StyleSilva, Tiago, Afonso Gregório, Filipe Silva, José Xavier, Ana Reis, Pedro Rosa, and Abílio de Jesus. 2021. "Numerical-Experimental Plastic-Damage Characterisation of Additively Manufactured 18Ni300 Maraging Steel by Means of Multiaxial Double-Notched Specimens" Journal of Manufacturing and Materials Processing 5, no. 3: 84. https://doi.org/10.3390/jmmp5030084
APA StyleSilva, T., Gregório, A., Silva, F., Xavier, J., Reis, A., Rosa, P., & de Jesus, A. (2021). Numerical-Experimental Plastic-Damage Characterisation of Additively Manufactured 18Ni300 Maraging Steel by Means of Multiaxial Double-Notched Specimens. Journal of Manufacturing and Materials Processing, 5(3), 84. https://doi.org/10.3390/jmmp5030084











