Surface Integrity of AISI 52100 Bearing Steel after Robot-Based Machine Hammer Peening
Abstract
1. Introduction
1.1. Initial Situation and Motivation
1.2. Objective
1.3. Approach
2. Materials and Methods
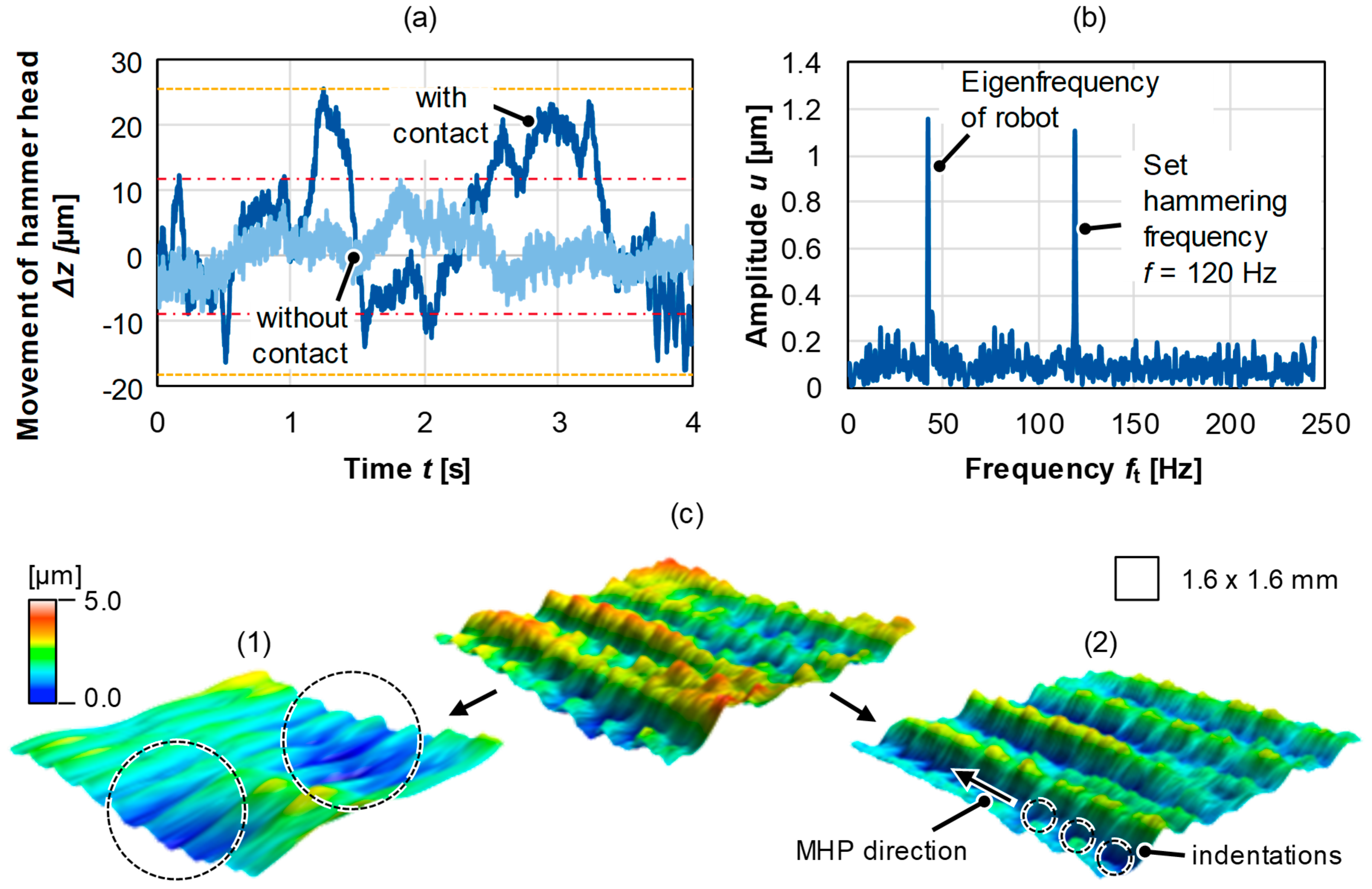
2.1. Analysis of Robot Dynamics
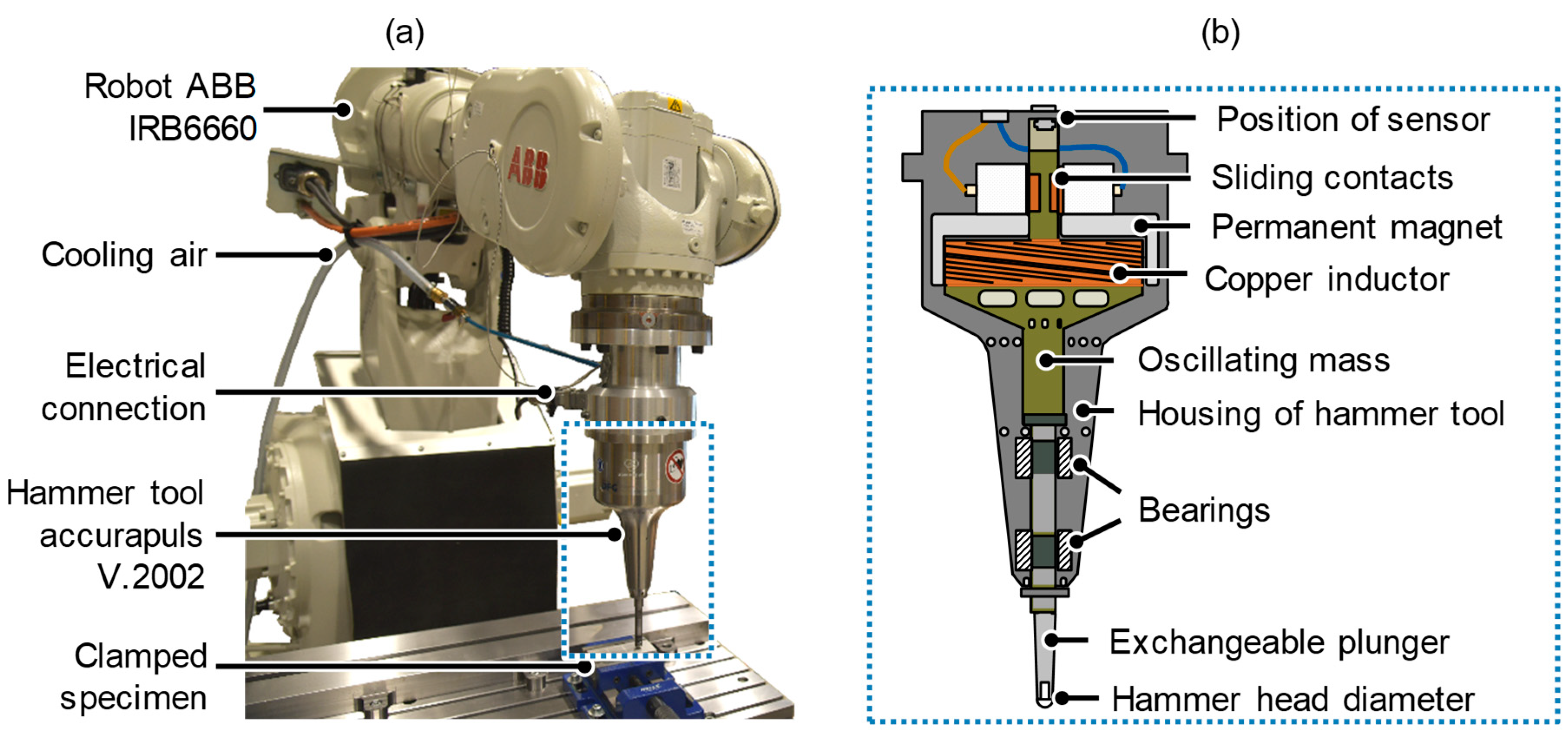
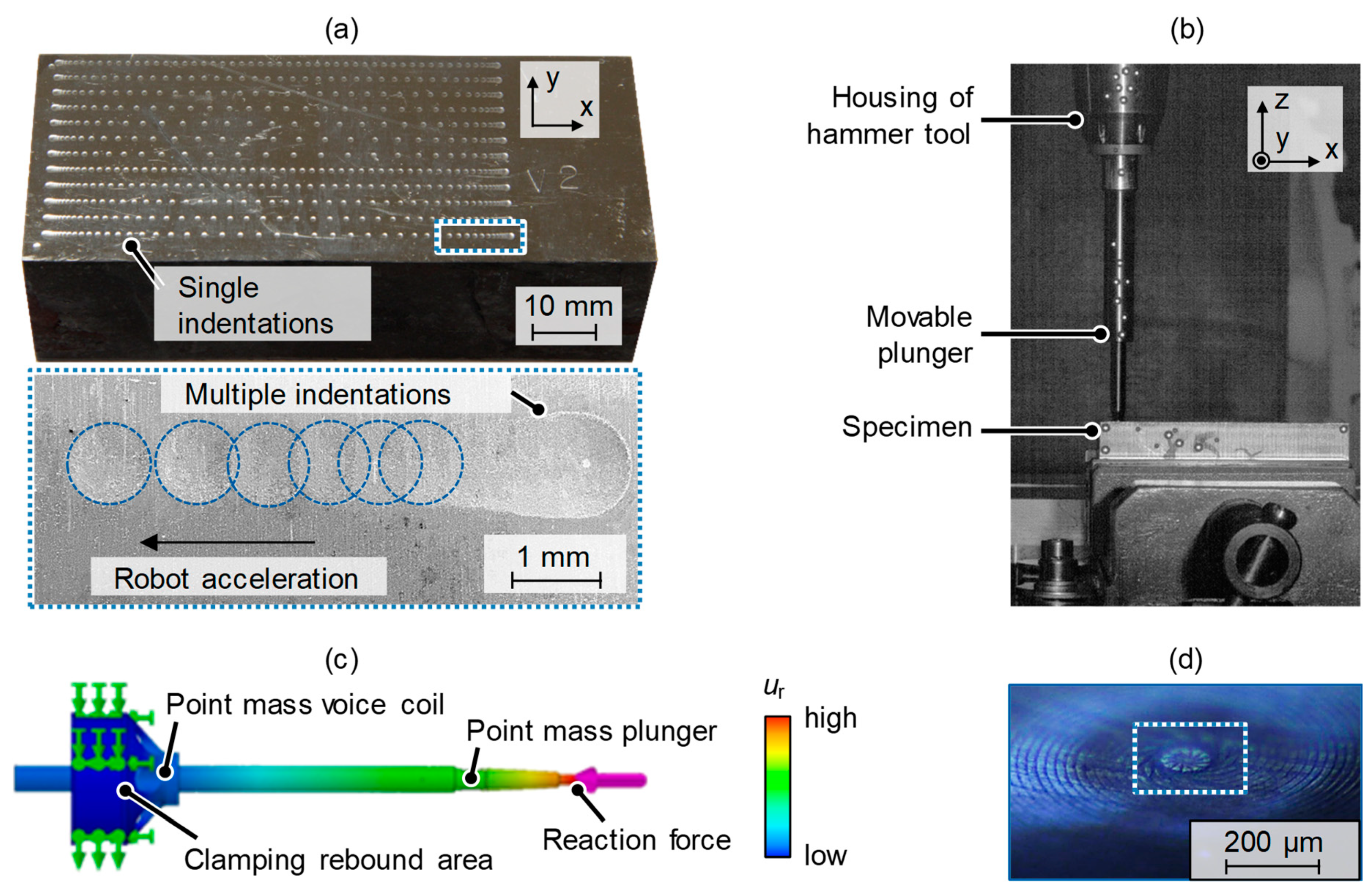
2.2. MHP Test Bench Environment and Characterisation Methods
3. Results and Discussion
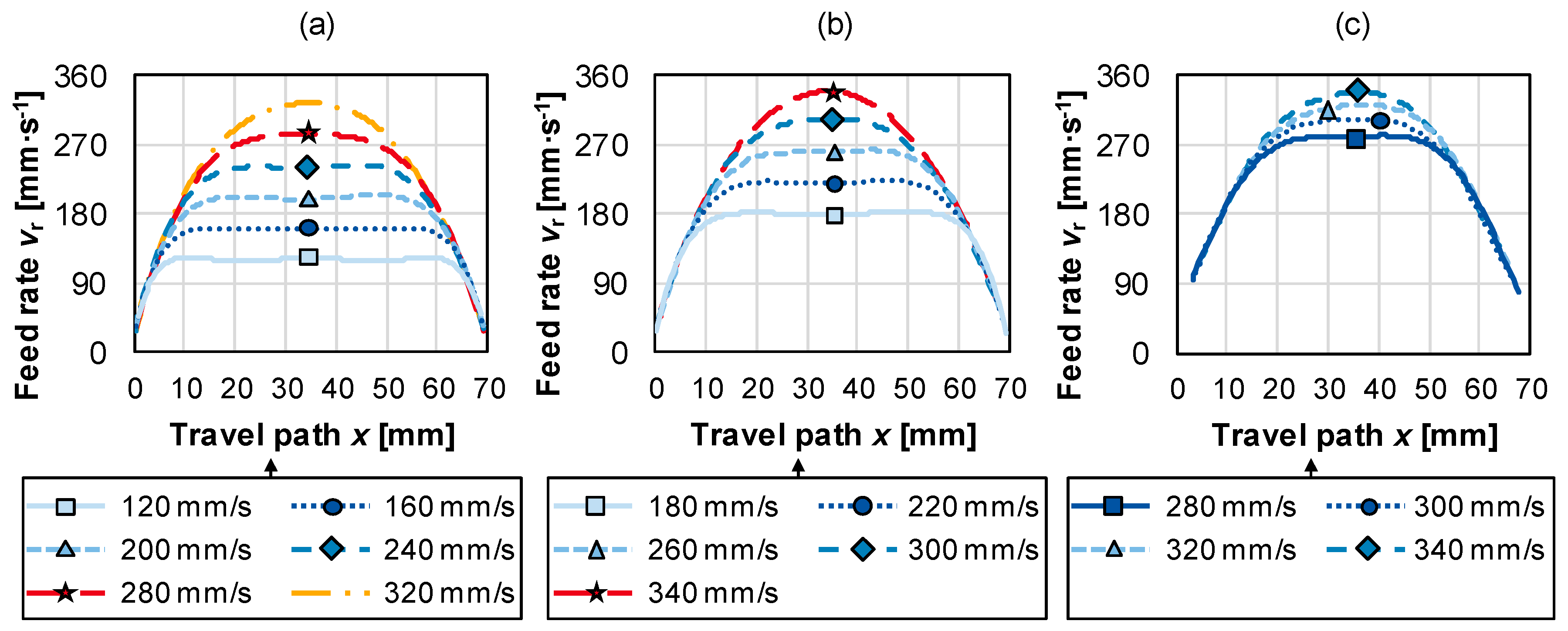
3.1. Robot Acceleration
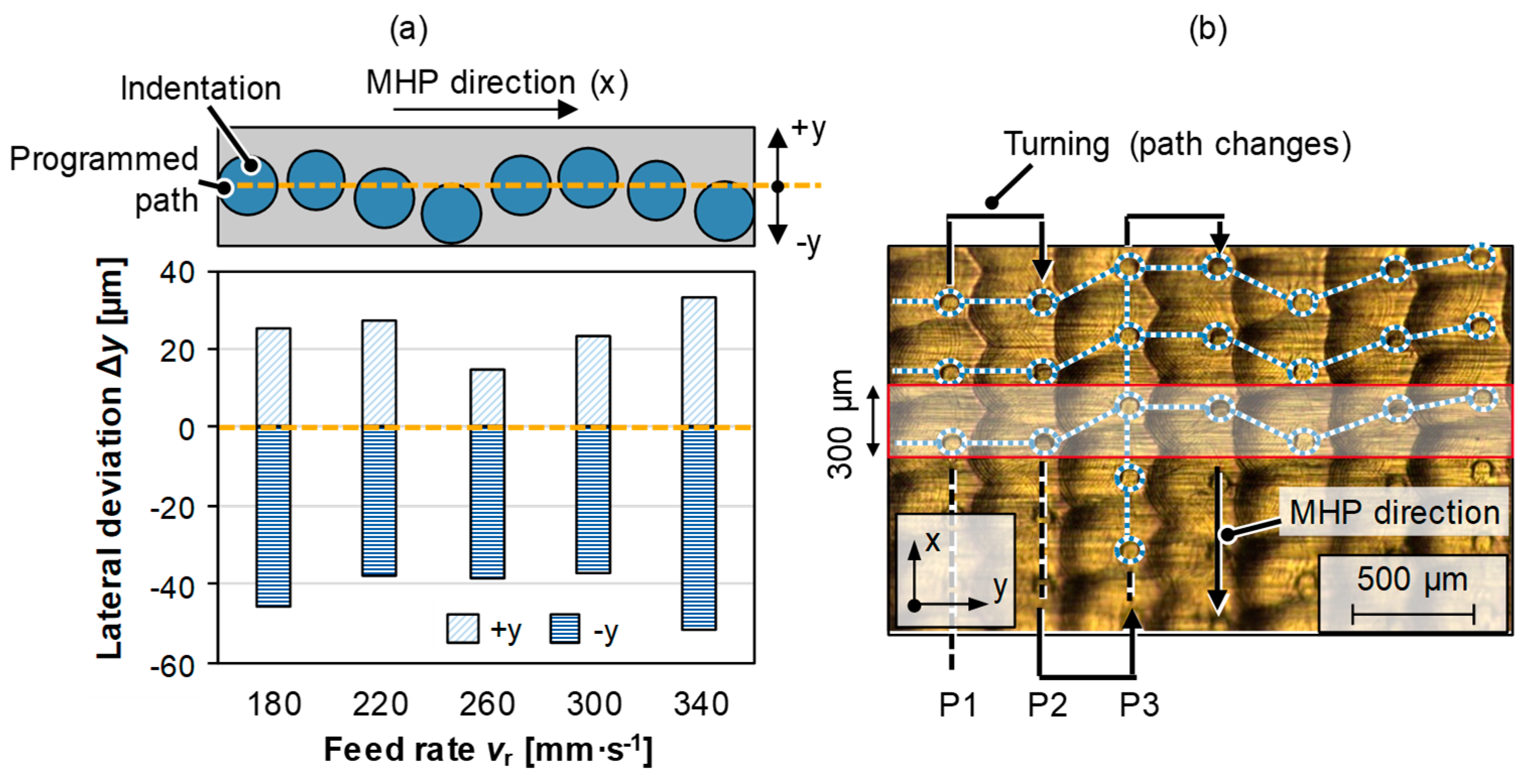
3.2. Robot Vibration and Indentation Profiles during MHP
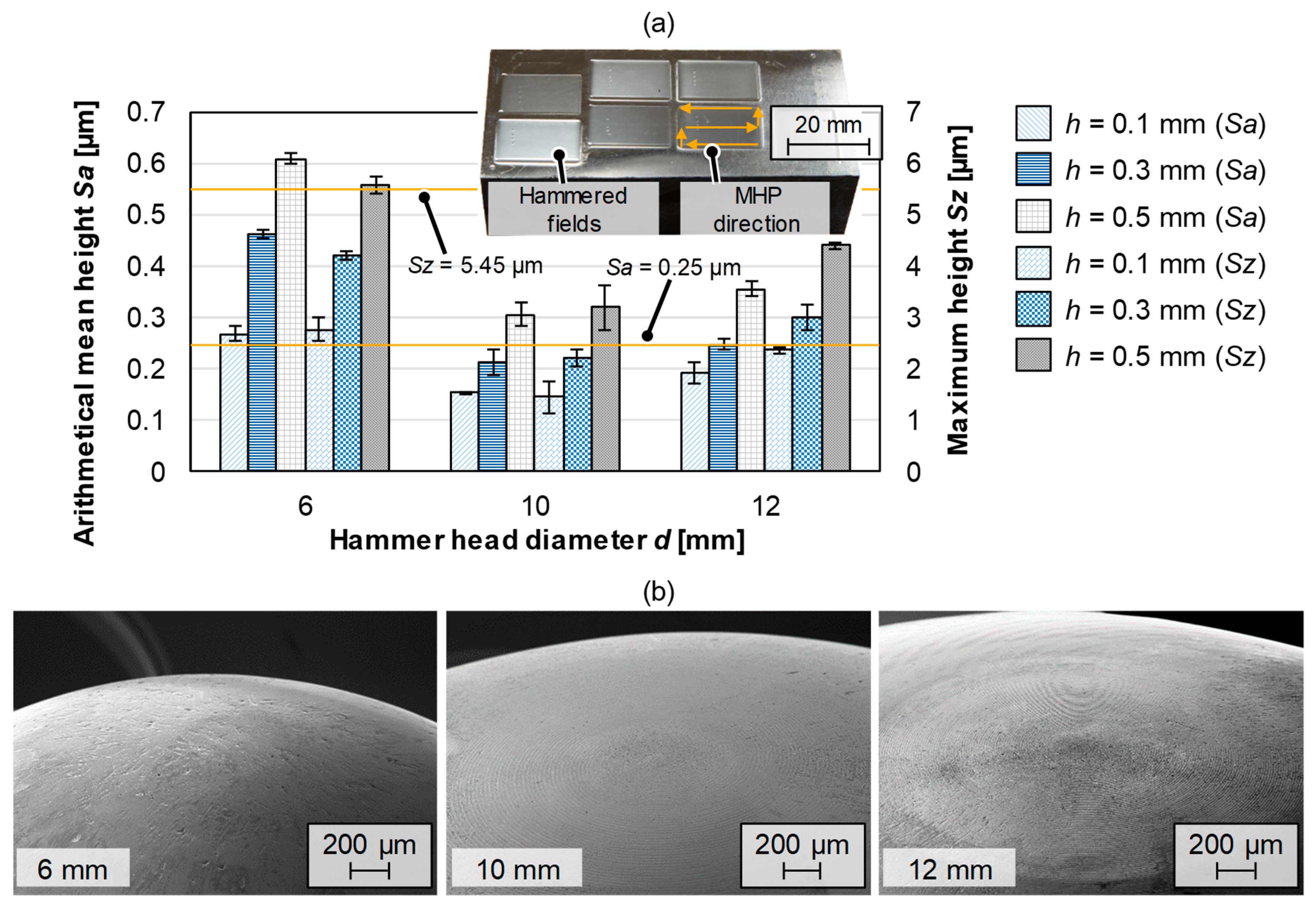
3.3. Surface Roughness
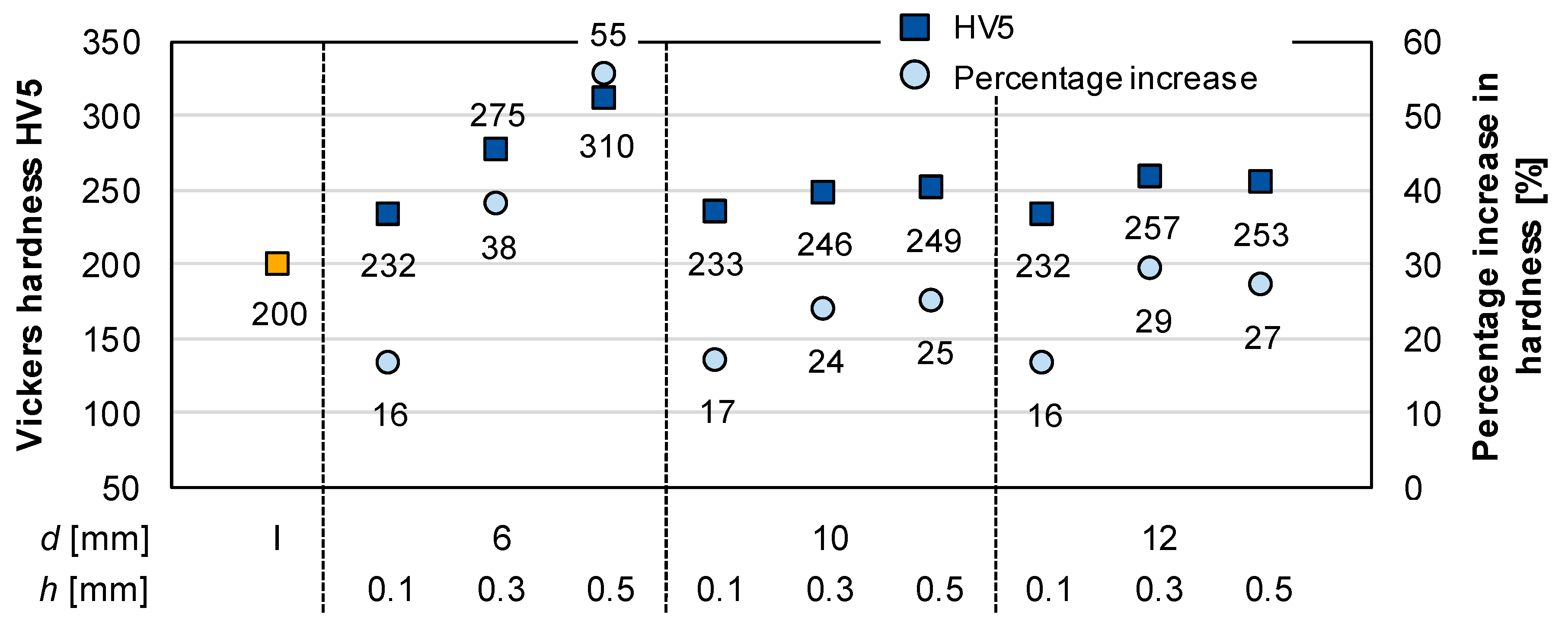
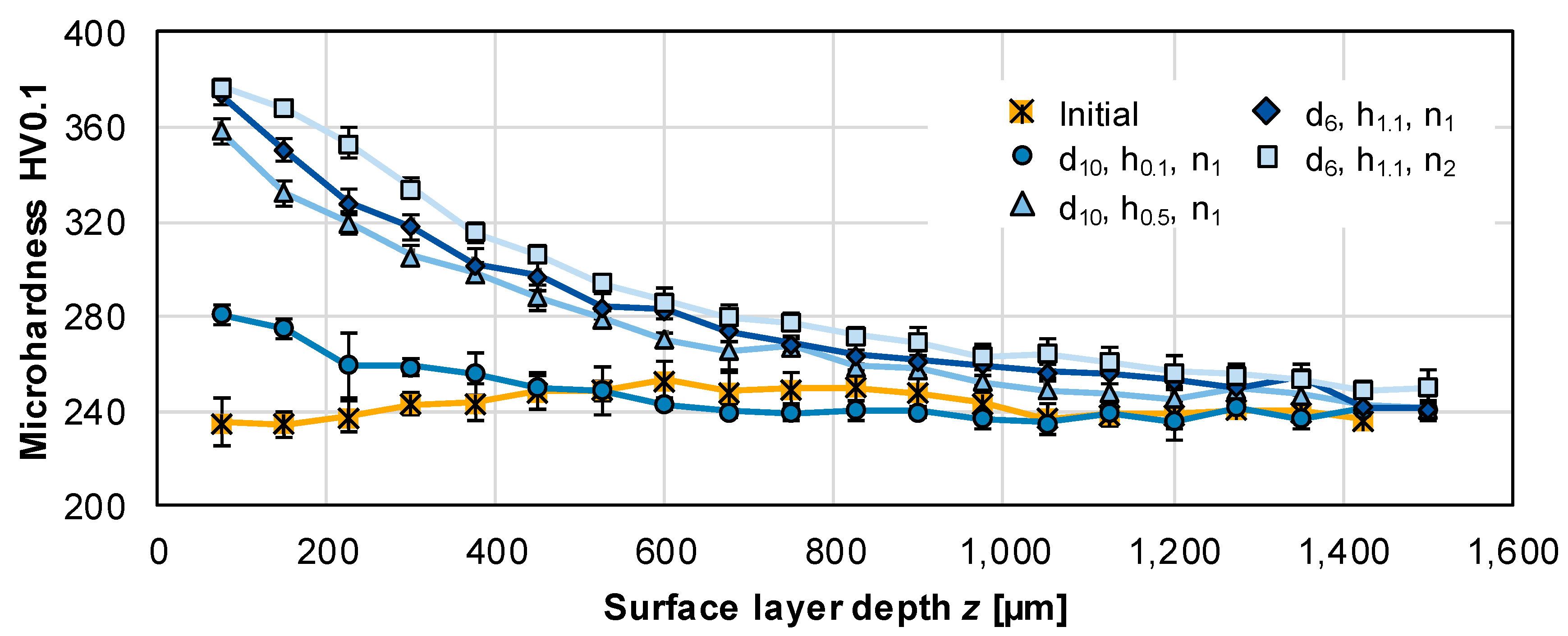
3.4. Surface Layer Hardness
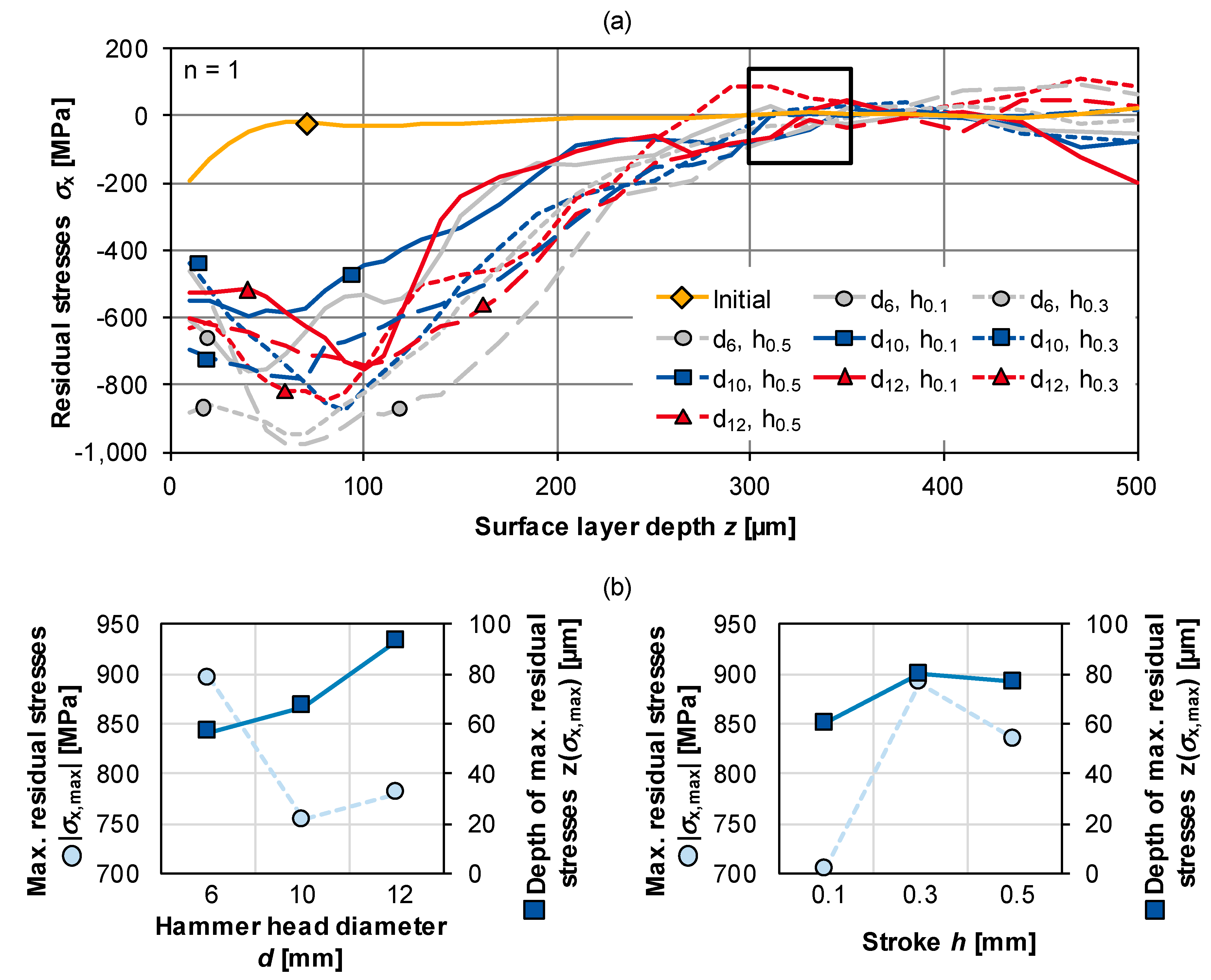
3.5. Residual Stresses
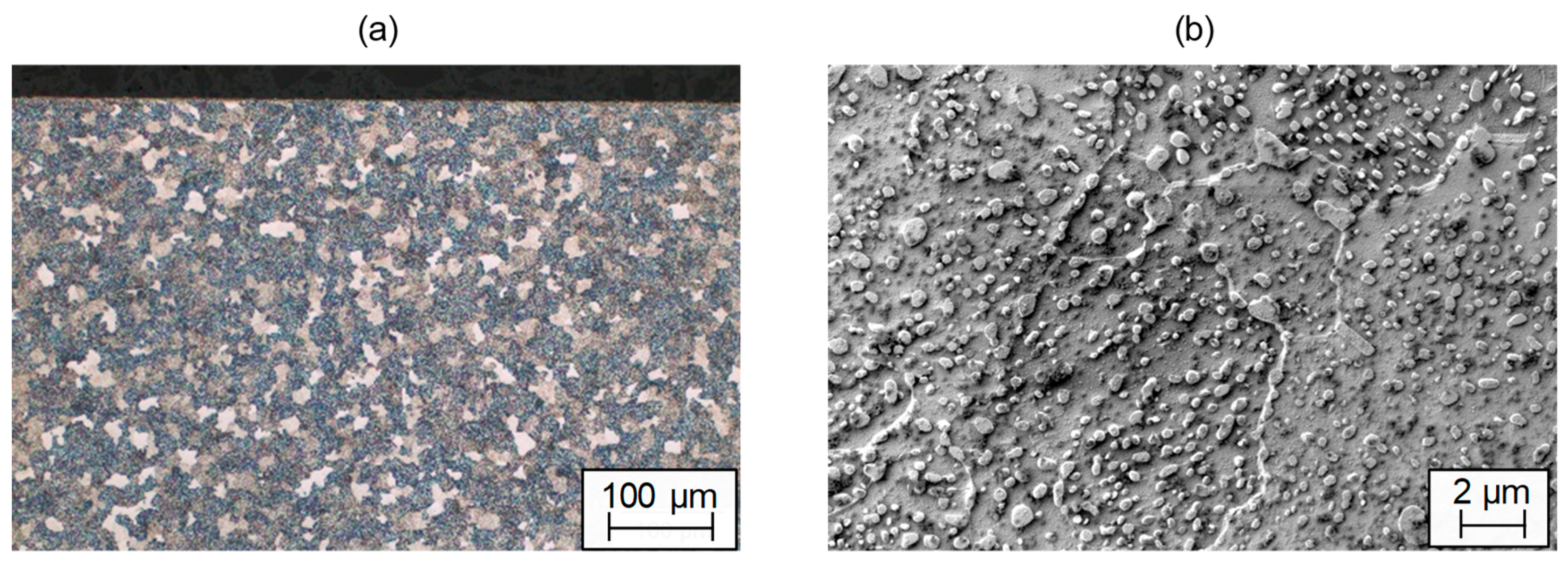
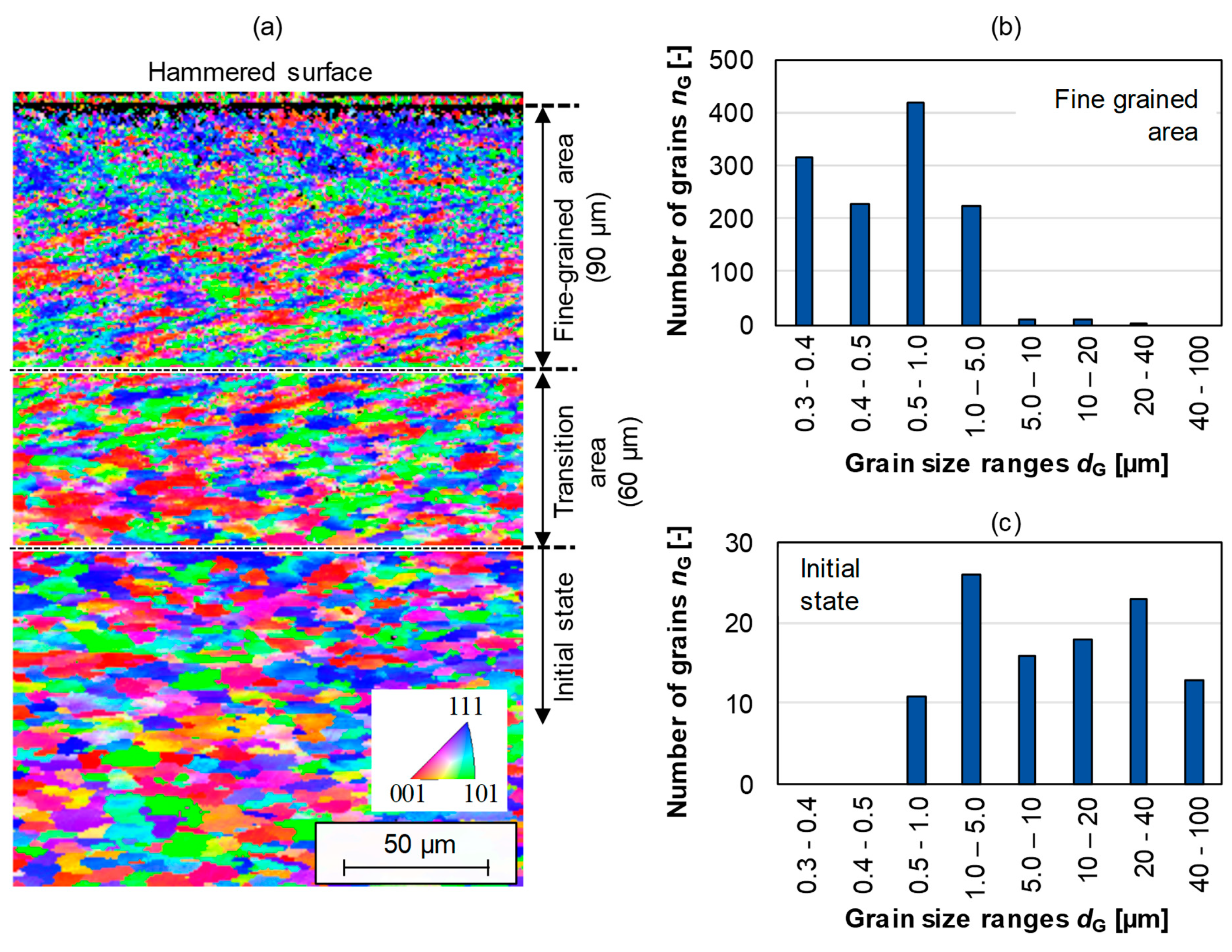
3.6. Grain Size
4. Conclusions
- Independent of the selected feed rate of the industrial robot, the range of constant feed rate and thus reproducible MHP conditions can be described mathematically.
- Because of the high robot stiffness (eigenfrequency fr = 42.5 Hz), the lateral deviation during MHP processing along a hammering path and along the impact axis are very small. Therefore, this robot enables reproducible MHP processing with regard to a defined energy density.
- Depending on the contact energy and energy density, the electrodynamic MHP can not only smoothen but also roughen the surface. A reduction of the roughness Sz by 60% compared to a milled surface can be achieved. A large hammer head diameter and a small stroke tend to be advantageous.
- The surface hardness can be increased by 75% in the considered MHP parameter range and an effective depth of hardening of z = 1500 µm can be achieved. A high plunger stroke and a small hammer head diameter are particularly advantageous.
- High compressive residual stress maxima σx,max = −950…−580 MPa were introduced below the surface. A small hammer head diameter and high stroke tend to lead to higher compressive residual stress maxima.
- A significant grain size reduction down to the submicron range could be achieved down to a surface layer depth z = 150 µm.
Author Contributions
Funding
Conflicts of Interest
References
- Šmelova, V.; Schwedt, A.; Wang, L.; Holweger, W.; Mayer, J. Electron microscopy investigations of microstructural alterations due to classical Rolling Contact Fatigue (RCF) in martensitic AISI 52100 bearing steel. Int. J. Fatigue 2017, 98, 142–154. [Google Scholar] [CrossRef]
- Kang, J.-H.; Rivera-Díaz-del-Castillo, P.E.J. Carbide dissolution in bearing steels. Comput. Mater. Sci. 2013, 67, 364–372. [Google Scholar] [CrossRef]
- Fu, H.; Song, W.; Galindo-Nava, E.I.; Rivera-Díaz-del-Castillo, P.E.J. Strain-induced martensite decay in bearing steels under rolling contact fatigue: Modelling and atomic-scale characterization. Acta Mater. 2017, 139, 163–173. [Google Scholar] [CrossRef]
- Hannes, D.; Alfredsson, B. Modelling of surface initiated rolling contact fatigue damage. Procedia Eng. 2013, 66, 766–774. [Google Scholar] [CrossRef][Green Version]
- Neubauer, T. Betriebs- und Lebensdauerverhalten Hartgedrehter und Festgewalzter Zylinderrollenlager. Ph.D. Thesis, Gottfried Wilhelm Leibniz University, Hannover, Germany, 2016. [Google Scholar]
- Barrow, A.T.W.; Rivera-Díaz-del-Castillo, P.E.J. Nanoprecipitation in bearing steels. Acta Mater. 2011, 59, 7155–7167. [Google Scholar] [CrossRef]
- Kreuser, J. Konstruktion und Aufbau eines Wälzlagerprüfstands zur Untersuchung von Hybrid- und Keramikwälzlagern. Ph.D. Thesis, RWTH Aachen University, Aachen, Germany, 2007. [Google Scholar]
- Warhadpande, A.; Sadeghi, F.; Evans, R.D. Microstructural alterations in bearing steels under rolling contact fatigue part 1—Historical overview. Tribol. Trans. 2013, 56, 349–358. [Google Scholar] [CrossRef]
- Rycerz, P.; Olver, A.; Kadiric, A. Propagation of surface initiated rolling contact fatigue cracks in bearing steel. Int. J. Fatigue 2017, 97, 29–38. [Google Scholar] [CrossRef]
- Kerscher, E.; Lang, K.H.; Vöhringer, O.; Löhe, D. Increasing the fatigue limit of a bearing steel by dynamic strain aging. Int. J. Fatigue 2008, 30, 1838–1842. [Google Scholar] [CrossRef]
- Yakimets, I.; Richard, C.; Béranger, G.; Peyre, P. Laser peening processing effect on mechanical and tribological properties of rolling steel 100Cr6. Wear 2004, 256, 311–320. [Google Scholar] [CrossRef]
- Röttger, K. Walzen Hartgedrehter Oberflächen. Ph.D. Thesis, RWTH Aachen University, Aachen, Germany, 2002. [Google Scholar]
- Schulze, V.; Bleicher, F.; Groche, P.; Guo, Y.B.; Pyun, Y.S. Surface modification by machine hammer peening and burnishing. CIRP Ann.-Manuf. Technol. 2016, 65, 809–832. [Google Scholar] [CrossRef]
- Trauth, D.; Klocke, F.; Welling, D.; Terhorst, M.; Mattfeld, P.; Klink, A. Investigation of the surface integrity and fatigue strength of Inconel718 after wire EDM and machine hammer peening. Int. J. Mater. Form. 2016, 9, 635–651. [Google Scholar] [CrossRef]
- Trauth, D. Tribology of Machine Hammer Peened Tool Surfaces for Deep Drawing. Ph.D. Thesis, RWTH Aachen University, Aachen, Germany, 2016. [Google Scholar]
- Bleicher, F.; Lechner, C.; Habersohn, C.; Kozeschnik, E.; Adjassoho, B.; Kaminski, H. Mechanism of surface modification using machine hammer peening technology. CIRP Ann.-Manuf. Technol. 2012, 61, 375–378. [Google Scholar] [CrossRef]
- Krall, S.; Lechner, C.; Nirtl, M.; Bleicher, F. Robot based machine hammer peening using an electromagnetic driven hammering device. In Annals of DAAAM, Proceedings of 26th DAAAM International Symposium on Intelligent Manufacturing and Automation, Zadar, Croatia, 21–24 October 2015; Katalinic, B., Ed.; Curran Associates, Inc.: New York, NY, USA, 2016. [Google Scholar]
- Habersohn, C. Analytische und Simulative Betrachtung eines Oberflächenhämmerprozesses. Ph.D. Thesis, Vienna University of Technology, Vienna, Austria, 2015. [Google Scholar]
- Mannens, R.; Uhlmann, L.; Feuerhack, A.; Bergs, T. Energy-Dependent Surface Integrity of Stainless Steel AISI 304 after Robot-Based Machine Hammer Peening. In Proceedings of the 13th International Conference on the Technology of Plasticity (ICTP), Columbus, OH, USA, 25–30 July 2021. (accepted). [Google Scholar]
- Schajer, G.S.; Rickert, T.J. Incremental Computation Technique for Residual Stress Calculations Using the Integral Method. Exp. Mech. 2011, 51, 1217–1222. [Google Scholar] [CrossRef]
- Krall, S. Beeinflussung der Randschicht Durch Diskrete Mikroumformung. Ph.D. Thesis, Vienna University of Technology, Vienna, Austria, 2019. [Google Scholar]
- Bleicher, F.; Lechner, C.; Habersohn, C.; Obermair, M.; Heindl, F.; Rodriguez-Ripoll, M. Improving the tribological characteristics of tool and mould surfaces by machine hammer peening. CIRP Ann.-Manuf. Technol. 2013, 62, 239–242. [Google Scholar] [CrossRef]
- Lechner, C. Oberflächenmodifikation unter Einsatz der Technologie des Schlagverdichtens (Machine Hammer Peenings). Ph.D. Thesis, Vienna University of Technology, Vienna, Austria, 2014. [Google Scholar]
- Wied, J. Oberflächenbehandlung von Umformwerkzeugen Durch Festklopfen. Ph.D. Thesis, Technical University of Darmstadt, Darmstadt, Germany, 2011. [Google Scholar]
- Adjassoho, B.; Kozeschnik, E.; Lechner, C.; Bleicher, F.; Goessinger, S.; Bauer, C. Induction of residual stresses and increase of surface hardness by machine hammer peening. In Annals of DAAAM, Proceedings of 23rd DAAAM International Symposium on Intelligent Manufacturing and Automation, Zadar, Croatia, 24–27 October 2012; Katalinic, B., Ed.; DAAAM International: Vienna, Austria, 2012. [Google Scholar]
- Scheil, J.; Müller, C.; Steitz, M.; Groche, P. Influence of Process Parameters on Surface Hardening in Hammer Peening and Deep Rolling. Key Eng. Mater. 2013, 554–557, 1819–1827. [Google Scholar] [CrossRef]
- Lienert, F. Bauteiloptimierung Mittels Piezopeening—Auswirkungen auf den Randschichtzustand und die Schwingfestigkeit von 42CrMo4 V450. Ph.D. Thesis, Karlsruhe Institute of Technology KIT, Karlsruhe, Germany, 2017. [Google Scholar]
- Klumpp, A.; Tamam, M.A.; Vollert, F.; Dietrich, S.; Schulze, V. Influence of conventional and cryogenic piezo peening on bending fatigue strength of hardened bearing steel AISI 52100. In Proceedings of the 13th International Conference on Shot Peening, Montréal, QC, Canada, 18–21 September 2017; pp. 435–440. [Google Scholar]
- Medvedeva, A.; Berglund, J.; Wied, J.; Gunnarsson, S. Surface characteristics of different tool steels and cast iron after machine hammer peening. In Proceedings of the 4th International Swedish Production Symposium, Lund, Sweden, 3–5 May 2011; pp. 212–218. [Google Scholar]
- Hessert, R.; Bamberg, J.; Satzger, W.; Taxer, T. Ultrasonic Impact Treatment for Surface Hardening of the Aero-Engine Material IN718. In Proceedings of the 10th International Conference on Shot Peening, Tokyo, Japan, 15–18 September 2008. [Google Scholar]
- Chen, T.; John, H.; Xu, J.; Lu, Q.; Hawk, J.; Liu, X. Influence of surface modification on pitting corrosion behaviour of nickel-base alloy 718. Part 1: Effect of machine hammer peening. Corros. Sci. 2013, 77, 230–245. [Google Scholar] [CrossRef]
| C | Si | Mn | P | S | Cr | Mo | Al | Cu | |
|---|---|---|---|---|---|---|---|---|---|
| wt% | 0.955 | 0.251 | 0.391 | 0.012 | 0.005 | 1.510 | 0.015 | 0.028 | 0.141 |
| σ (wt%) | 0.019 | 0.001 | 0.003 | <0.001 | <0.001 | 0.008 | <0.001 | 0.003 | <0.001 |
| Test Series | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| No. | 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 |
| a (mm) | 0.05 | ||||||||
| f (Hz) | 120 | ||||||||
| d (mm) | 6 | 10 | 12 | ||||||
| h (mm) | 0.1 | 0.3 | 0.5 | 0.1 | 0.3 | 0.5 | 0.1 | 0.3 | 0.5 |
| Ec (mJ) | 7 | 20 | 40 | 7 | 20 | 40 | 7 | 20 | 40 |
| w (mJ/mm2) | 2800 | 8000 | 16,000 | 2800 | 8000 | 16,000 | 2800 | 8000 | 16,000 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mannens, R.; Uhlmann, L.; Lambers, F.; Feuerhack, A.; Bergs, T. Surface Integrity of AISI 52100 Bearing Steel after Robot-Based Machine Hammer Peening. J. Manuf. Mater. Process. 2020, 4, 61. https://doi.org/10.3390/jmmp4020061
Mannens R, Uhlmann L, Lambers F, Feuerhack A, Bergs T. Surface Integrity of AISI 52100 Bearing Steel after Robot-Based Machine Hammer Peening. Journal of Manufacturing and Materials Processing. 2020; 4(2):61. https://doi.org/10.3390/jmmp4020061
Chicago/Turabian StyleMannens, Robby, Lars Uhlmann, Felix Lambers, Andreas Feuerhack, and Thomas Bergs. 2020. "Surface Integrity of AISI 52100 Bearing Steel after Robot-Based Machine Hammer Peening" Journal of Manufacturing and Materials Processing 4, no. 2: 61. https://doi.org/10.3390/jmmp4020061
APA StyleMannens, R., Uhlmann, L., Lambers, F., Feuerhack, A., & Bergs, T. (2020). Surface Integrity of AISI 52100 Bearing Steel after Robot-Based Machine Hammer Peening. Journal of Manufacturing and Materials Processing, 4(2), 61. https://doi.org/10.3390/jmmp4020061






