Precision Permittivity Measurement for Low-Loss Thin Planar Materials Using Large Coaxial Probe from 1 to 400 MHz
Abstract
1. Introduction
2. Large Coaxial Probe Design
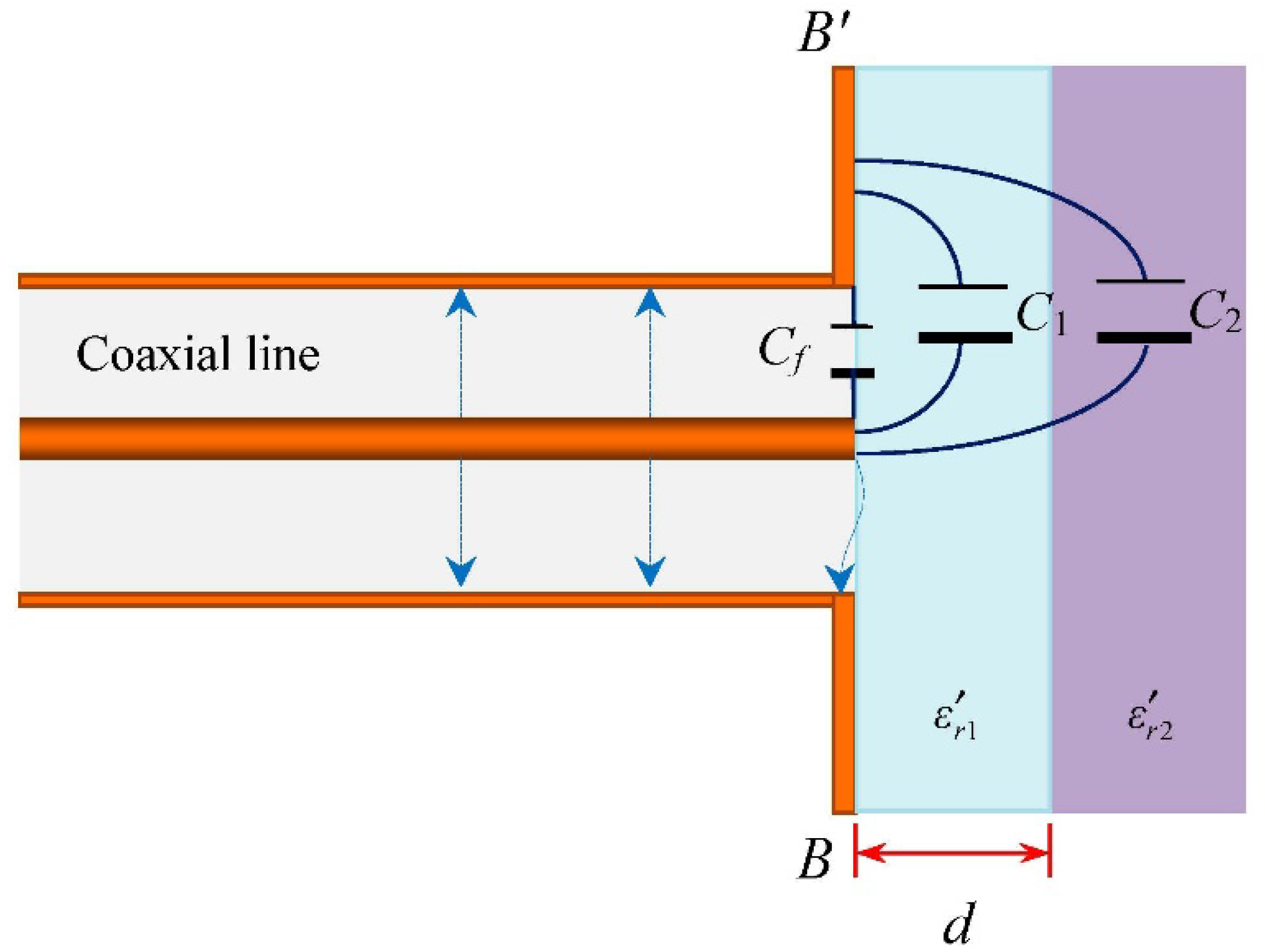
2.1. Coaxial Probe Formulations
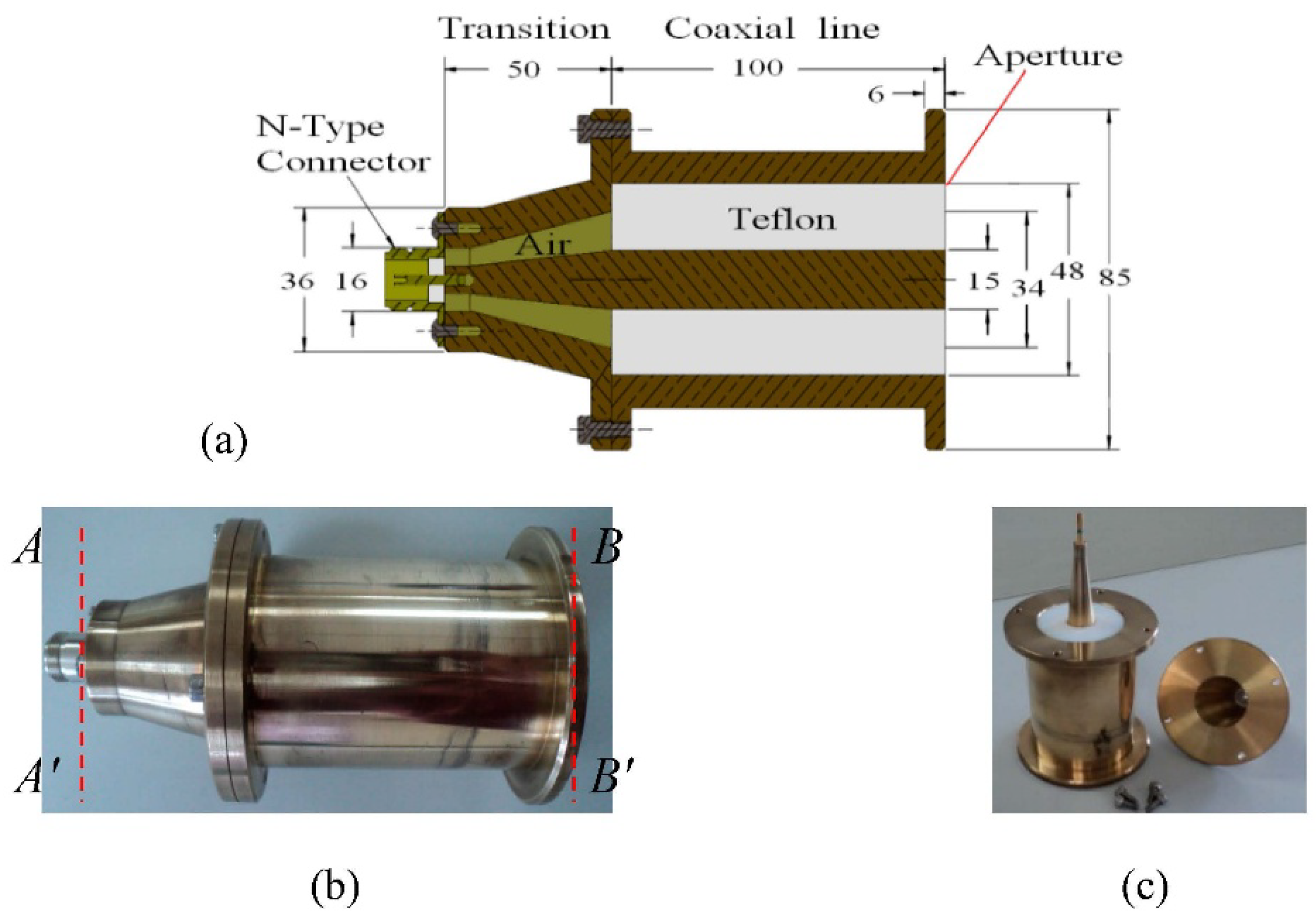
2.2. Dimensions and Structure
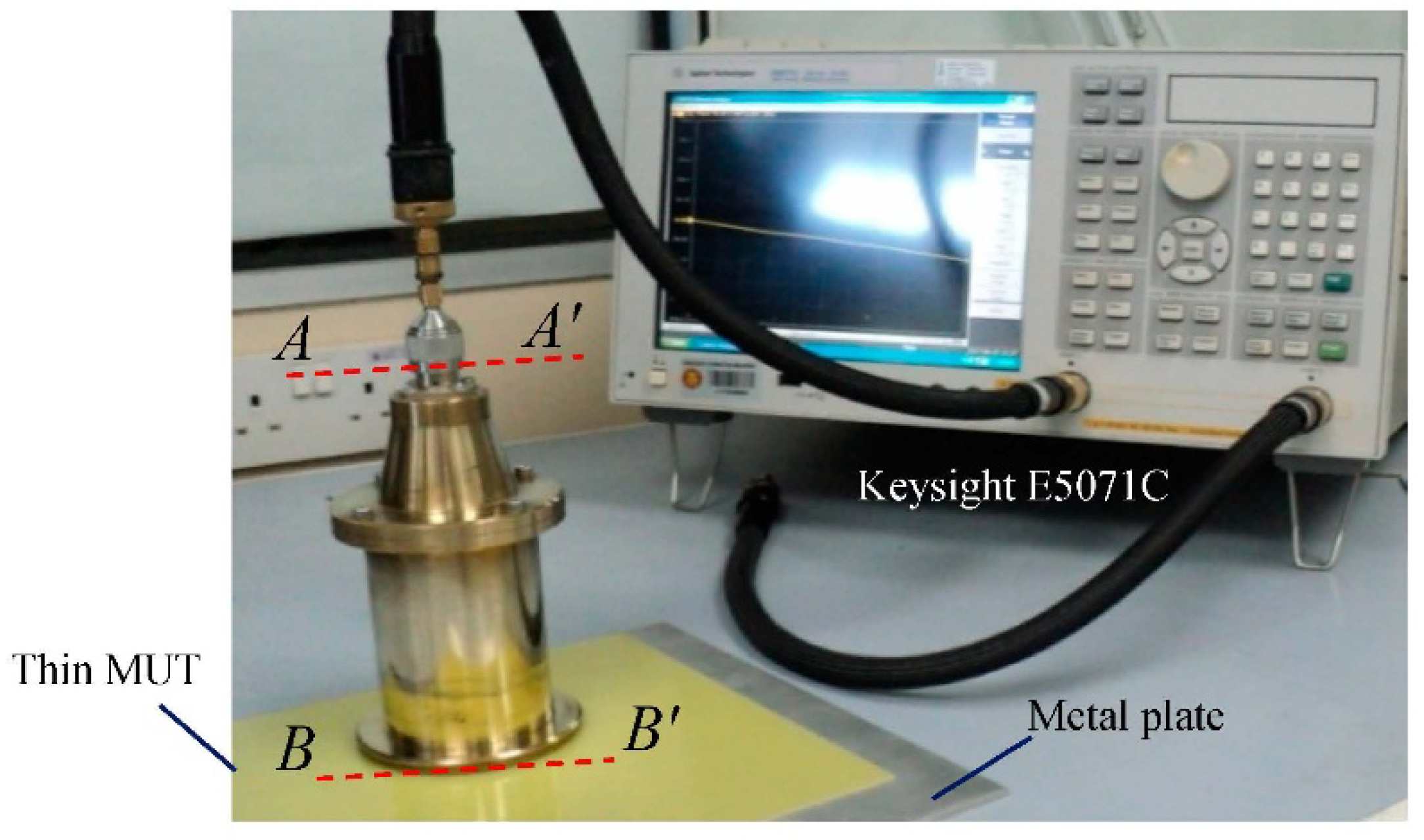
2.3. Probe Characterization Test
3. Calibrations
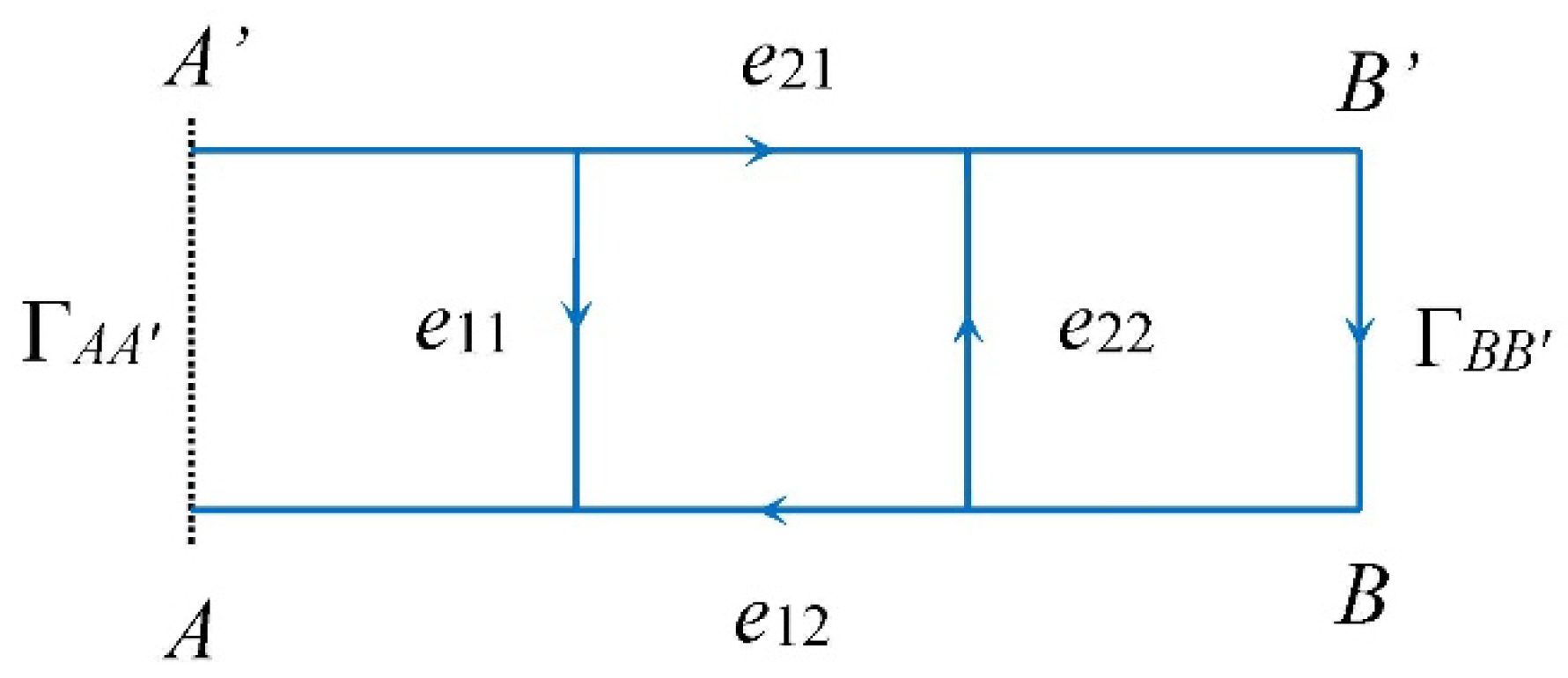
3.1. Calibration Formulations
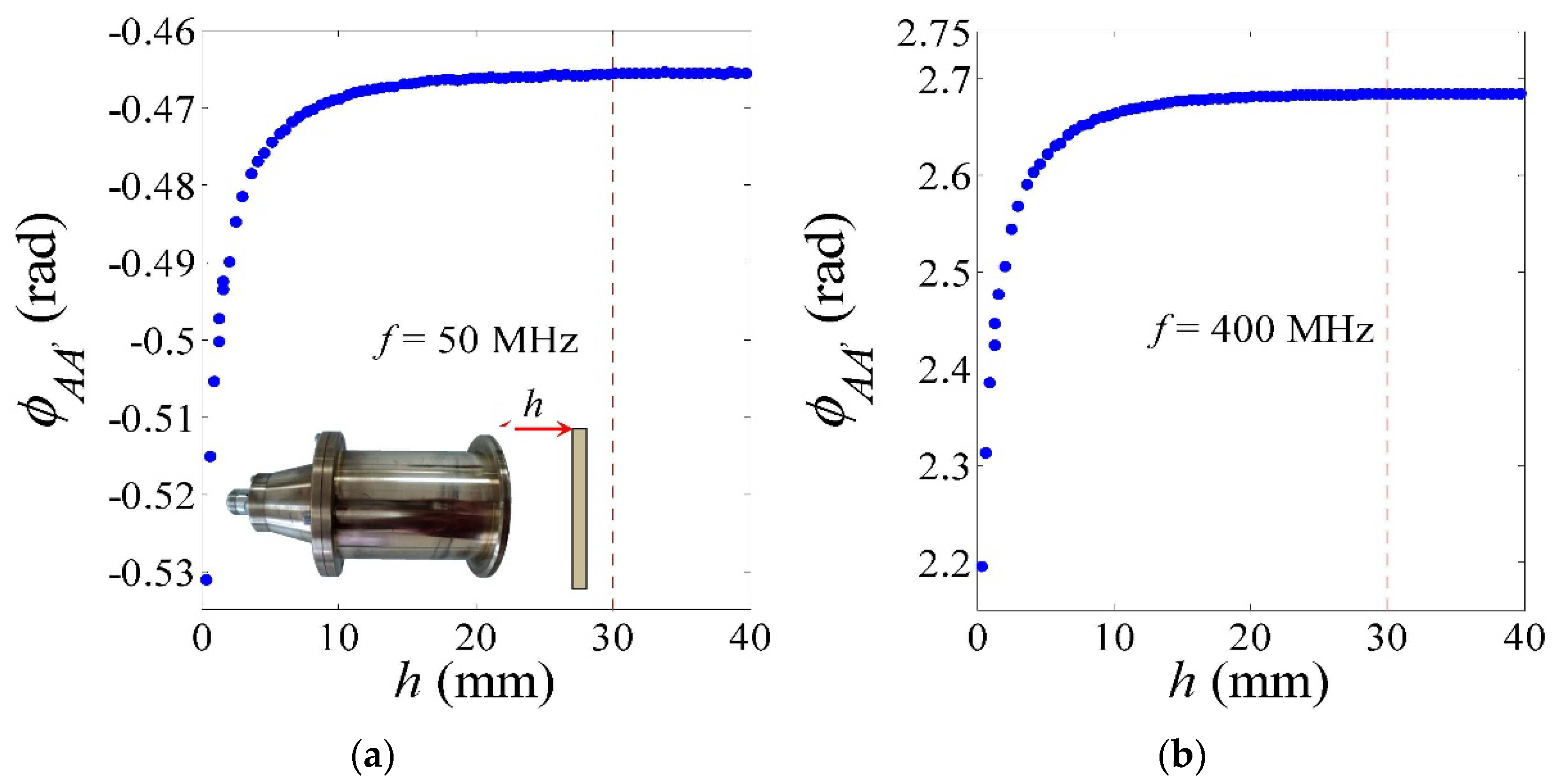
3.2. Probe Aperture Calibration
3.3. Effective Permittivity Calibration
4. Results and Discussion
4.1. Reflection Coefficient, ΓAA′
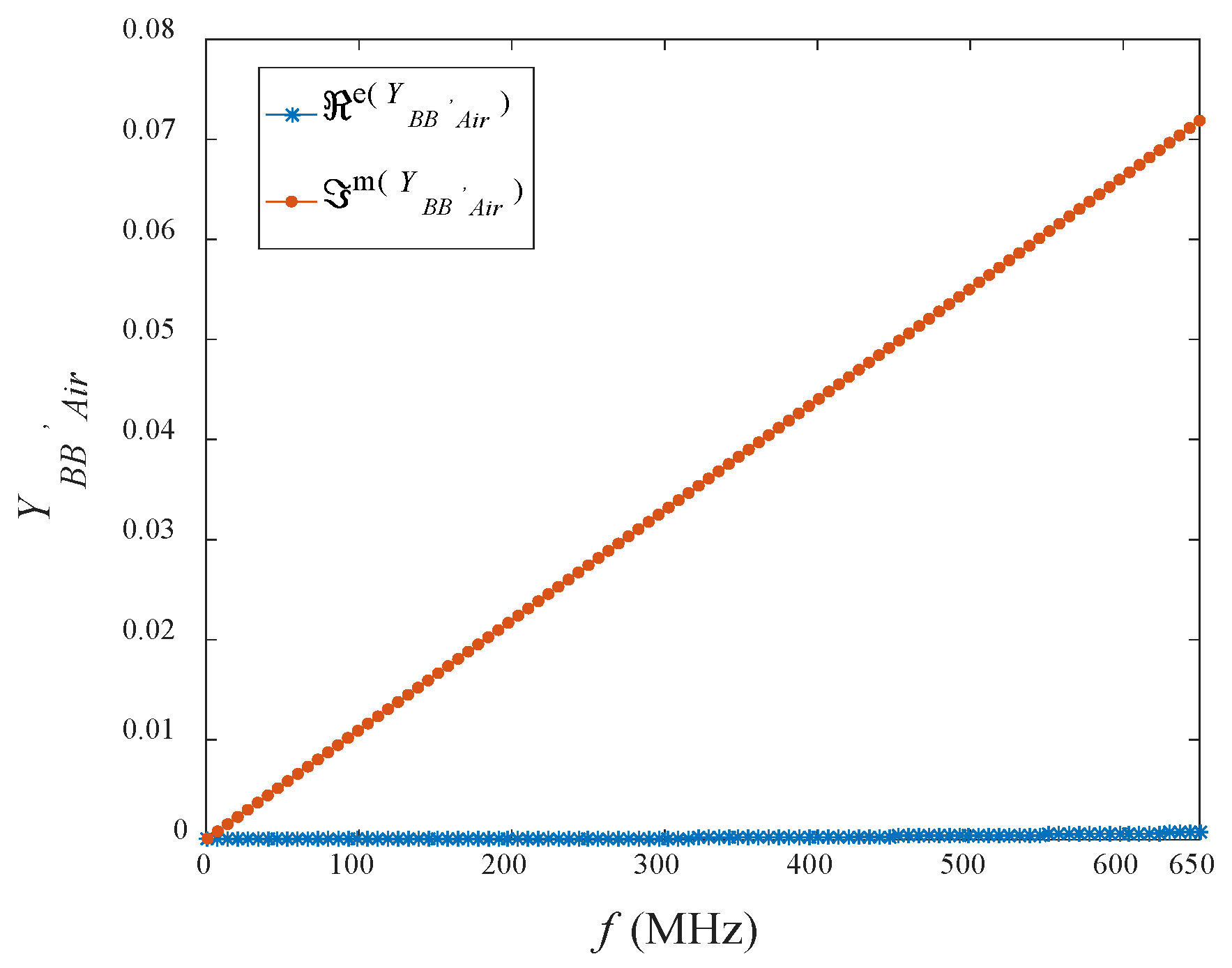
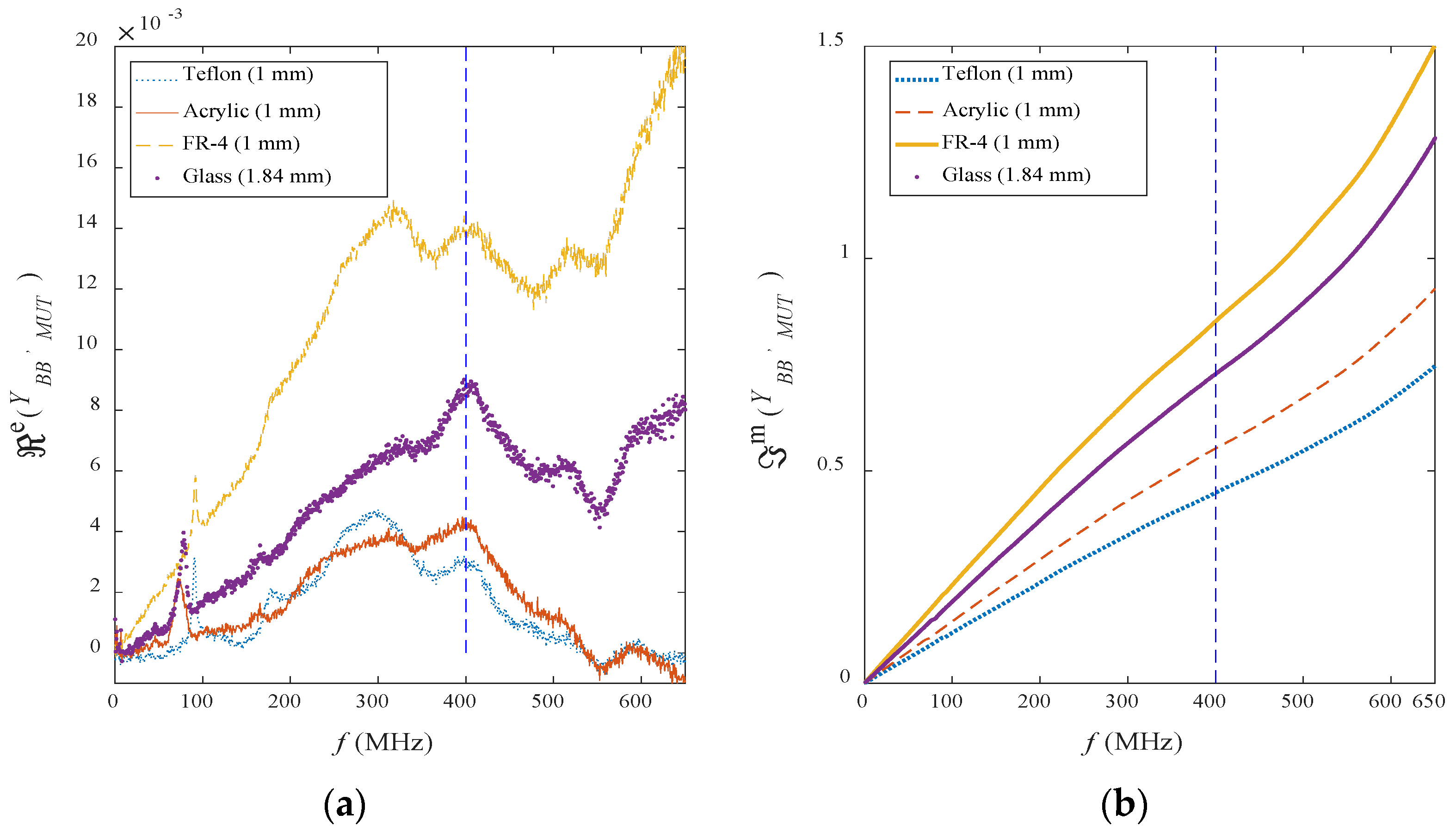
4.2. Normalized Admittance, ỸBB′
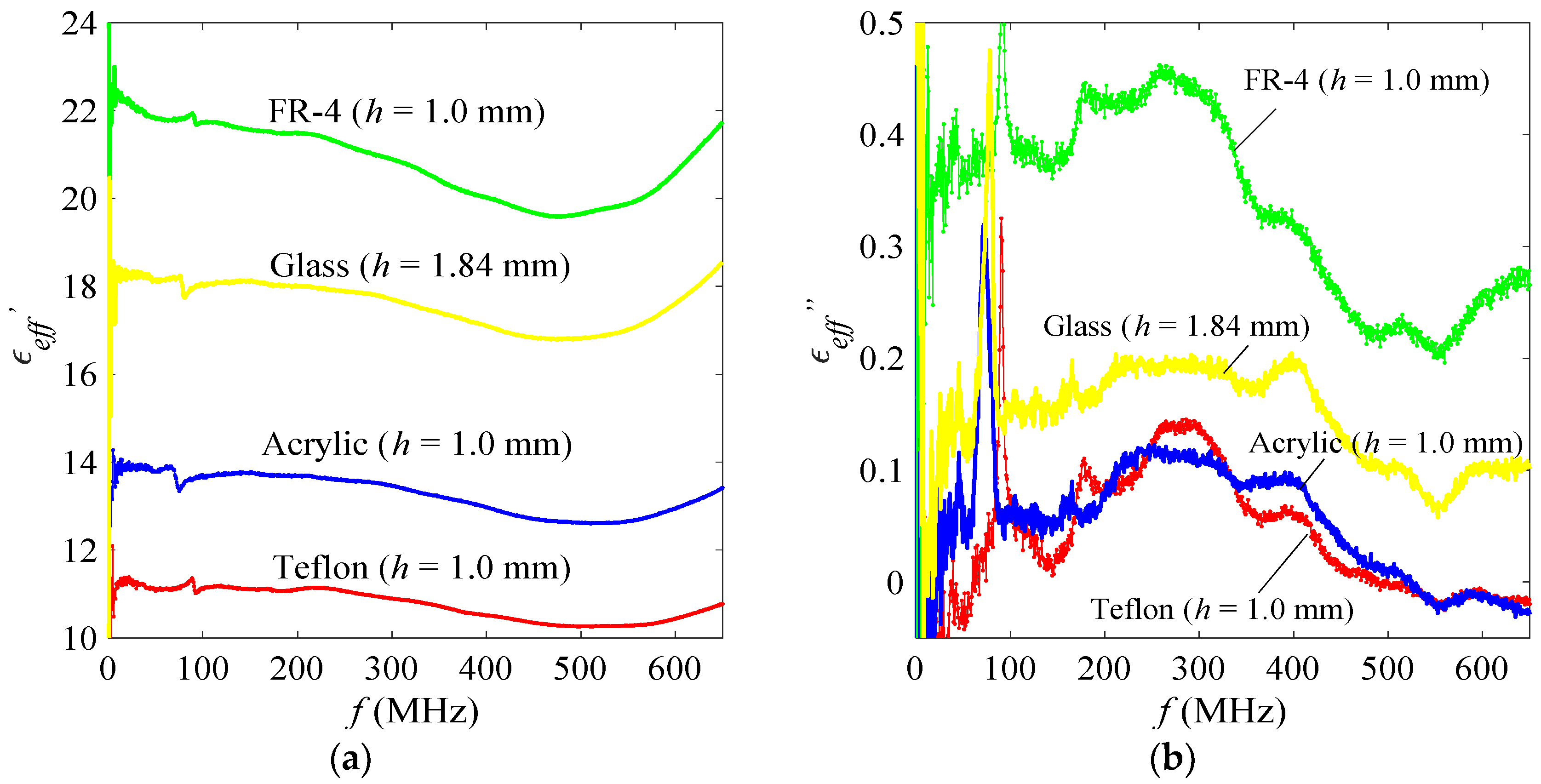
4.3. Effective Permittivity, εeff
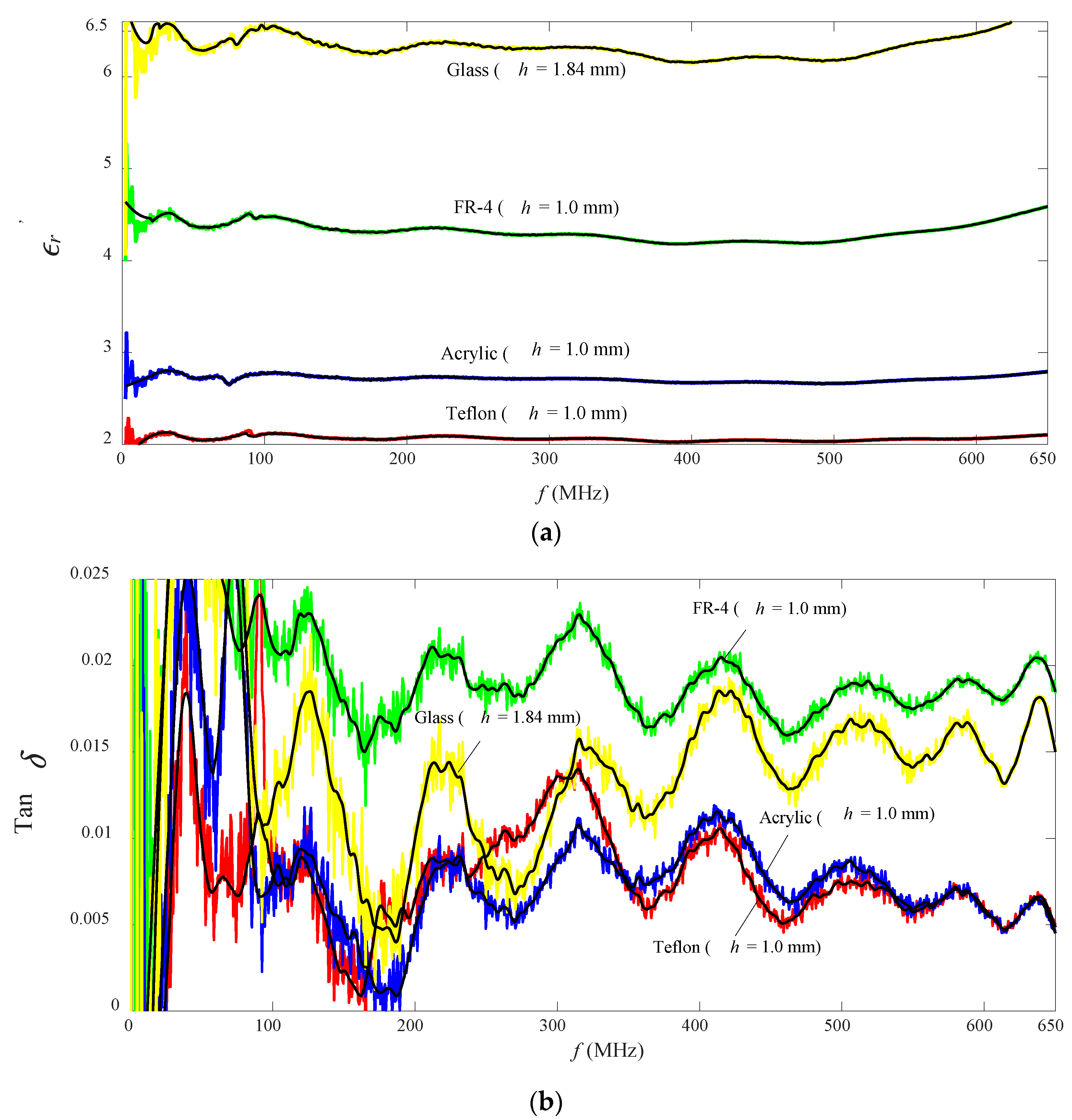
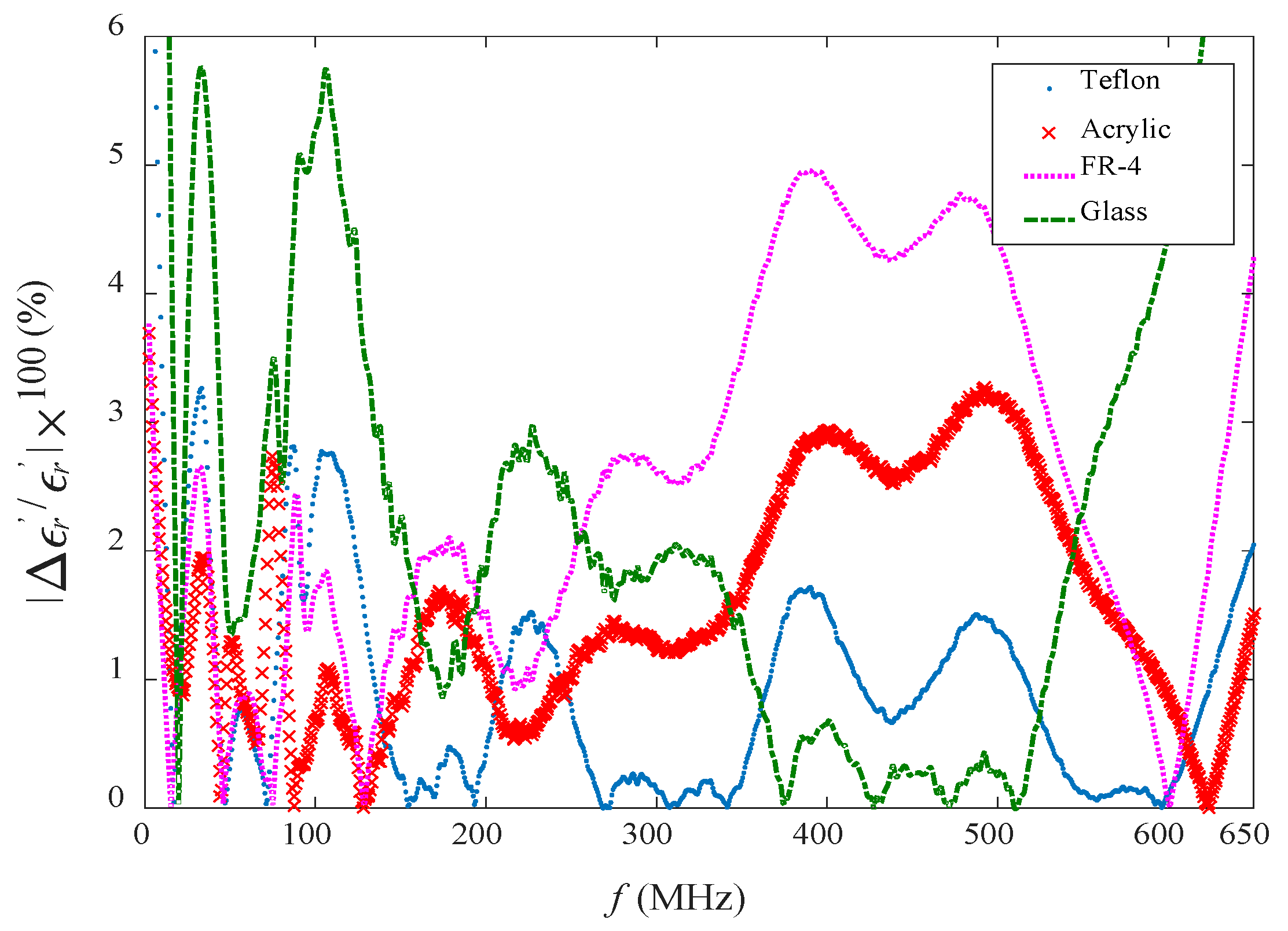
4.4. Actual Relative Permittivity, εr
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Bakhshiani, M.; Suster, M.A.; Mohseni, P. A Broadband Sensor Interface IC for Miniaturized Dielectric Spectroscopy from MHz to GHz. IEEE J. Solid-State Circuits 2014, 49, 1669–1681. [Google Scholar] [CrossRef]
- Hegde, V.J.; Gallot-Lavallée, O.; Heux, L. Dielectric study of Polycaprolactone: A biodegradable polymer. In Proceedings of the 2016 IEEE International Conference on Dielectrics (ICD), Montpellier, France, 3–7 July 2016; Volume 1, pp. 293–296. [Google Scholar]
- Noh, J.; Kim, S.M.; Heo, S.; Kang, S.C.; Kim, Y.; Lee, Y.G.; Park, H.; Lee, S.; Lee, B.H. Time Domain Reflectometry Analysis of the Dispersion of Metal–Insulator–Metal Capacitance. IEEE Electron Device Lett. 2017, 38, 521–524. [Google Scholar] [CrossRef]
- Hewlett-Packard. User’s Manual: HP 85070B Dielectric Probe Kit; Hewlett-Packard Company: Palo Alto, CA, USA, 1997. [Google Scholar]
- Schmid & Partner Engineering, AG. High Precision Dielectric Measurements: DAK; SPEAG: Zurich, Switzerland, 2018. [Google Scholar]
- KEYCOM. Open Mode Probe Method Dielectric Constant and Dielectric Loss Tangent Measurement System; KEYCOM Characteristic Technologies: Tokyo, Japan, 2000. [Google Scholar]
- APREL. Dielectric Probe Kit ALS-PR-DIEL; APREL Inc.: Kanata, ON, Canada, 2014. [Google Scholar]
- Filali, B.; Boone, F.; Rhazi, J.; Ballivy, G. Design and Calibration of a Large Open-Ended Coaxial Probe for the Measurement of the Dielectric Properties of Concrete. IEEE Trans. Microw. Theory Tech. 2008, 56, 2322–2328. [Google Scholar] [CrossRef]
- Otto, G.P.; Chew, W.C. Improved Calibration of a Large Open-Ended Coaxial Probe for Dielectric Measurements. IEEE Trans. Instrum. Meas. 1991, 40, 742–746. [Google Scholar] [CrossRef]
- Damme, S.V.; Franchois, A.; Zutter, D.D.; Taerwe, L. Nondestructive Determination of the Steel Fiber Content in Concrete Slabs with an Open-Ended Coaxial Probe. IEEE Trans. Geosci. Remote Sens. 2004, 42, 2511–2521. [Google Scholar] [CrossRef]
- Huang, Y. Design, Calibration and Data Interpretation for a One-Port Large Coaxial Dielectric Measurement Cell. Meas. Sci. Technol. 2001, 12, 111–115. [Google Scholar] [CrossRef]
- Kitić, G.; Crnojević-Bengin, V. A Sensor for the Measurement of the Moisture of Undisturbed Soil Samples. Sensors 2013, 13, 1692–1705. [Google Scholar] [CrossRef]
- Busssey, H. Dielectric Measurement in a Shielded Open Circuit Coaxial Line. IEEE Trans. Instrum. Meas. 1980, 29, 120–124. [Google Scholar] [CrossRef]
- Brady, M.M.; Symons, S.A.; Stuchly, S.S. Dielectric Behavior of Selected Animal Tissues In Vitro at Frequencies from 2 to 4 GHz. IEEE Trans. Biomed. Eng. 1981, 28, 305–307. [Google Scholar] [CrossRef]
- Atley, T.W.; Stuchly, M.A.; Stuchly, S.S. Measurement of Radio Frequency Permittivity of Biological Tissues with an Open-Ended Coaxial Line: Part I. IEEE Trans. Microw. Theory Tech. 1982, 30, 82–86. [Google Scholar] [CrossRef]
- Gajda, G.; Stuchly, S.S. An Equivalent Circuit of an Open-Ended Coaxial Line. IEEE Trans. Instrum. Meas. 1983, 32, 506–508. [Google Scholar] [CrossRef]
- Kraszewski, A.; Stuchly, S.S. Capacitance of Open-Ended Dielectric-Filled Coaxial Lines-Experimental Results. IEEE Trans. Instrum. Meas. 1983, 32, 517–519. [Google Scholar] [CrossRef]
- Grant, J.P.; Clarke, R.N.; Symm, G.T.; Spyrou, N.M. A Critical Study of the Open-Ended Coaxial Line Sensor Technique for RF and Microwave Complex Permittivity Measurements. J. Phys. E 1989, 22, 757–770. [Google Scholar] [CrossRef]
- Levine, H.; Papas, C.H. Theory of the Circular Diffraction Antenna. J.Appl. Phy. 1951, 22, 29–43. [Google Scholar] [CrossRef]
- Nevels, R.D.; Butler, C.M.; Yablon, W. The Annular Slot Antenna in a Lossy Biological Medium. IEEE Trans. Microw. Theory Tech. 1985, 33, 314–319. [Google Scholar] [CrossRef]
- Misra, D. A Quasi-Static Analysis of Open-Ended Coaxial Lines. IEEE Trans. Microw. Theory Techn. 1987, 35, 925–928. [Google Scholar] [CrossRef]
- Anderson, L.S.; Gajda, G.B.; Stuchly, S.S. Analysis of an Open-Ended Coaxial Line Sensor in Layered Dielectric. IEEE Trans. Instrum. Meas. 1986, 35, 13–18. [Google Scholar] [CrossRef]
- Fan, S.; Staebell, K.; Misra, D. Static Analysis of an Open-Ended Coaxial Line Terminated by Layered Media. IEEE Trans. Instrum. Meas. 1990, 39, 435–437. [Google Scholar] [CrossRef]
- Xu, Y.S.; Bosisio, R.G. Nondestructive Measurements of the Resistivity of Thin Conductive Films and the Dielectric Constant of Thin Substrates Using an Open-Ended Coaxial Line. IEE Proc. H Microw. Antennas Propag. 1992, 139, 500–506. [Google Scholar]
- Li, L.L.; Ismail, N.H.; Taylor, L.S.; Davis, C.C. Flanged Coaxial Microwave Probes for Measuring Thin Moisture Layers. IEEE Trans. Biomed. Eng. 1992, 39, 49–57. [Google Scholar] [CrossRef]
- Jiang, G.Q.; Wong, W.H.; Raskovich, E.Y.; Clark, W.G.; Hines, W.A.; Sanny, J. Measurement of the Microwave Dielectric Constant for Low-Loss Samples with Finite Thickness Using Open-Ended Coaxial-Line Probes. Rev. Sci. Instrum. 1993, 64, 1622–1626. [Google Scholar] [CrossRef]
- Chen, G.; Li, K.; Ji, Z. Bilayered Dielectric Measurement with an Open-Ended Coaxial Probe. IEEE Trans. Microw. Theory Tech. 1994, 42, 966–971. [Google Scholar] [CrossRef]
- Li, C.L.; Chen, K.M. Determination of Electromagnetic Properties of Materials Using Flanged Open-Ended Coaxial Probe-Full-Wave Analysis. IEEE Trans. Instrum. Meas. 1995, 44, 19–27. [Google Scholar] [CrossRef]
- Ganchev, S.I.; Qaddoumi, N.; Bakhtiari, S.; Zoughi, R. Calibration and Measurement of Dielectric Properties of Finite Thickness Composite Sheets with Open-Ended Coaxial Sensors. IEEE Trans. Instrum. Meas. 1995, 44, 1023–1029. [Google Scholar] [CrossRef]
- Lee, J.H.; Eom, H.J.; Jun, K.H. Reflection of a Coaxial Line Radiating into a Parallel Plate. IEEE Microw. Guid. Wave Lett. 1996, 6, 135–137. [Google Scholar] [CrossRef]
- Folgero, K.; Tjomsland, T. Permittivity Measurement of Thin Liquid Layers Using Open Ended Coaxial Probes. Meas. Sci. Technol. 1996, 7, 1164–1173. [Google Scholar] [CrossRef]
- Alanen, E.; Lahtinen, T.; Nuutinen, J. Variational Formulation of Open-Ended Coaxial Line in Contact with Layered Biological Medium. IEEE Trans. Biomed. Eng. 1998, 45, 1241–1248. [Google Scholar] [CrossRef] [PubMed]
- Noh, Y.C.; Eom, H.J. Radiation from a Flanged Coaxial Line into a Dielectric Slab. IEEE Trans. Microw. Theory Techn. 1999, 47, 2158–2161. [Google Scholar] [CrossRef]
- You, K.Y. RF Coaxial Slot Radiators: Modeling, Measurements, and Applications; Artech House: Norwood, MA, USA, 2015; pp. 19–56. ISBN 9781608078226. [Google Scholar]
- You, K.Y.; Then, Y.L. Simple Calibration and Dielectric Measurement Technique for Thin Material Using Coaxial Probe. IEEE Sens. J. 2015, 15, 5393–5397. [Google Scholar] [CrossRef]
- Gajda, G.B.; Stuchly, S.S. Numerical Analysis of Open-Ended Coaxial Lines. IEEE Trans. Microw. Theory Techn. 1983, 31, 380–384. [Google Scholar] [CrossRef]
- Chen, L.F.; Ong, C.K.; Neo, C.P.; Varadan, V.V.; Varadan, V.K. Microwave Electronics: Measurement and Materials Characterization; John Wiley & Sons, Ltd.: West Sussex, UK, 2004; ISBN 9780470844922. [Google Scholar]




| Ref. | Probe Size (cm) | f (MHz) | Transition Section | Sample Contact | Sample Size/Shape | Calibration Standards | Measured εr′ Range | Inverse Method |
|---|---|---|---|---|---|---|---|---|
| [8] | 2a = 1.00 2b = 3.25 L = 5.00 | 100–900 | without | Aperture probe | Half-space infinite | Air, short, NaCl solution. | ~5–80 | Iterative |
| [9] | 2a = 1.00 2b = 3.25 L = 5.00 | 1–10 or 10–3000 | without | Aperture probe | Half-space infinite | Short cavity orAir, short, short cavity | ~30–80 (Lossy) | Iterative |
| [10] | 2a = 1.18 2b = 4.00 L ≈ 13.0 | 200–1500 | with | Aperture probe | Half-space infinite | Air, copper plate, Teflon plate. | ~2–35 (Lossy) | Iterative |
| [11] | 2a = 4.50 2b = 10.3 L ≈ 41.0 | 50–1000 | with | Filled in coaxial line | Toroid-shaped | 3 positions short-circuit along the coaxial line | ~7–80 (Lossy) | Iterative |
| [12] | 2a = 2.35 2b = 5.4 L ≈ 4.4 | 100–250 | with | Filled in coaxial line | Toroid-shaped | Based on specified specimen under test | 1–50 (Low lossy) | Non-iterative |
| This study | 2a = 1.50 2b = 4.80 L = 15.0 | 1–400 | with | Aperture Probe | Thin planar backed by metal plate | Air, 3 offset shorts | 1–20 (Lossless) | Non-iterative |
| Probe Size | |ΓAA′_MUT| | Differentiation of ∆|ΓAA′_MUT| between Teflon and FR-4 | |||
|---|---|---|---|---|---|
| Teflon | FR-4 | ||||
| This study 2a = 1.5 cm 2b = 4.8 cm | 1.000213 | Average: 1.0001019 Standard deviation: 0.0001784 | 0.9954108 | Average: 0.9959747 Standard deviation: 0.0004091 | 0.0041272 |
| 1.000345 | 0.9961075 | ||||
| 0.9999458 | 0.9957603 | ||||
| 0.9999258 | 0.9964970 | ||||
| 1.00008 | 0.9960977 | ||||
| Probe 1 2a = 0.3 cm 2b = 1.0 cm | 1.001418 | Average: 1.001394 Standard deviation: 0.000155902 | 1.000593 | Average: 1.0005194 Standard deviation: 0.00010485 | 0.0008746 |
| 1.001521 | 1.000355 | ||||
| 1.001302 | 1.000626 | ||||
| 1.001177 | 1.000507 | ||||
| 1.001552 | 1.000516 | ||||
| Probe 2 2a = 0.24 cm 2b = 0.8 cm | 0.9998745 | Average: 0.9998884 Standard deviation: 0.000131850 | 0.9995621 | Average: 0.99951490 Standard deviation: 0.000038824 | 0.0003735 |
| 1.000060 | 0.9994846 | ||||
| 0.9998878 | 0.9995518 | ||||
| 0.9999274 | 0.9994819 | ||||
| 0.9996925 | 0.9994941 | ||||
| Probe 3 2a = 0.13 cm 2b = 0.42 cm | 0.9996894 | Average: 0.99972804 Standard deviation: 0.000077999 | 0.9995004 | Average: 0.99967564 Standard deviation: 0.00014613 | 0.0000524 |
| 0.9996297 | 0.9995625 | ||||
| 0.9998415 | 0.9998173 | ||||
| 0.9997365 | 0.9998234 | ||||
| 0.9997431 | 0.9996746 | ||||
| Probe 4 2a = 0.09 cm 2b = 0.3 cm | 1.000269 | Average: 1.000275 Standard deviation: 0.000159385 | 1.000199 | Average: 1.0002248 Standard deviation: 0.000084138 | 0.0000502 |
| 1.000183 | 1.000161 | ||||
| 1.000358 | 1.000366 | ||||
| 1.000075 | 1.000233 | ||||
| 1.000490 | 1.000165 | ||||
| Probe Size | φAA′_MUT (°) | Differentiation of ∆φAA′_MUT between Teflon and FR-4 | |||
|---|---|---|---|---|---|
| Teflon | FR-4 | ||||
| This study 2a = 1.5 cm 2b = 4.8 cm | −32.3000 | Average: −32.21717 Standard deviation: 0.134307 | −38.56207 | Average: −38.34329 Standard deviation: 0.495519 | 6.12612° |
| −32.13884 | −38.64224 | ||||
| −32.25762 | −38.43801 | ||||
| −32.02661 | −37.46768 | ||||
| −32.3628 | −38.60646 | ||||
| Probe 1 2a = 0.3 cm 2b = 1.0 cm | −1.943045 | Average: −1.93933 Standard deviation: 0.00692831 | −2.522396 | Average: −2.542644 Standard deviation: 0.0144045 | 0.603314° |
| −1.930690 | −2.554037 | ||||
| −1.934963 | −2.535734 | ||||
| −1.939431 | −2.542843 | ||||
| −1.948521 | −2.558213 | ||||
| Probe 2 2a = 0.24 cm 2b = 0.8 cm | −13.88491 | Average: −13.884262 Standard deviation: 0.0098386975 | −14.31968 | Average: −14.315222 Standard deviation: 0.00953097686 | 0.43096° |
| −13.89140 | −14.31454 | ||||
| −13.86970 | −14.32456 | ||||
| −13.89475 | −14.31788 | ||||
| −13.88055 | −14.29945 | ||||
| Probe 3 2a = 0.13 cm 2b = 0.42 cm | −1.426506 | Average: −1.4218634 Standard deviation: 0.00444004 | −1.589263 | Average: −1.585402 Standard deviation: 0.00375146 | 0.163539° |
| −1.417838 | −1.579990 | ||||
| −1.419884 | −1.588442 | ||||
| −1.418287 | −1.583723 | ||||
| −1.426802 | −1.585592 | ||||
| Probe 4 2a = 0.09 cm 2b = 0.3 cm | −2.60528 | Average: −2.6106474 Standard deviation: 0.00985552 | −2.71289 | Average: −2.688339 Standard deviation: 0.01568456 | 0.077692° |
| −2.60139 | −2.679226 | ||||
| −2.618748 | −2.694912 | ||||
| −2.623582 | −2.674806 | ||||
| −2.604237 | −2.679861 | ||||
| MUT | εr′ (Typ) | tan δ (Max) |
|---|---|---|
| Teflon | 2.06 | 0.0004 |
| Acrylic | 2.75 | 0.019 |
| FR-4 | 4.4 | 0.022 |
| Glass | 6.1 | 0.0036 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
You, K.Y.; Sim, M.S. Precision Permittivity Measurement for Low-Loss Thin Planar Materials Using Large Coaxial Probe from 1 to 400 MHz. J. Manuf. Mater. Process. 2018, 2, 81. https://doi.org/10.3390/jmmp2040081
You KY, Sim MS. Precision Permittivity Measurement for Low-Loss Thin Planar Materials Using Large Coaxial Probe from 1 to 400 MHz. Journal of Manufacturing and Materials Processing. 2018; 2(4):81. https://doi.org/10.3390/jmmp2040081
Chicago/Turabian StyleYou, Kok Yeow, and Man Seng Sim. 2018. "Precision Permittivity Measurement for Low-Loss Thin Planar Materials Using Large Coaxial Probe from 1 to 400 MHz" Journal of Manufacturing and Materials Processing 2, no. 4: 81. https://doi.org/10.3390/jmmp2040081
APA StyleYou, K. Y., & Sim, M. S. (2018). Precision Permittivity Measurement for Low-Loss Thin Planar Materials Using Large Coaxial Probe from 1 to 400 MHz. Journal of Manufacturing and Materials Processing, 2(4), 81. https://doi.org/10.3390/jmmp2040081





