Performance Analysis of UAV RF/FSO Co-Operative Communication Network with Co-Channel Interference
Abstract
1. Introduction
1.1. Background
1.2. Related Works
1.3. Motivations and Contributions
- In contrast to most of the literature, oriented toward single-hop models, we propose an MMW RF/FSO communication network model that includes three nodes. The proposed model comprises direct and DH links, employing a selective DF scheme for the relay. The multi-node model aligns better with the characteristics of the UAV network as a centerless, self-organized network.
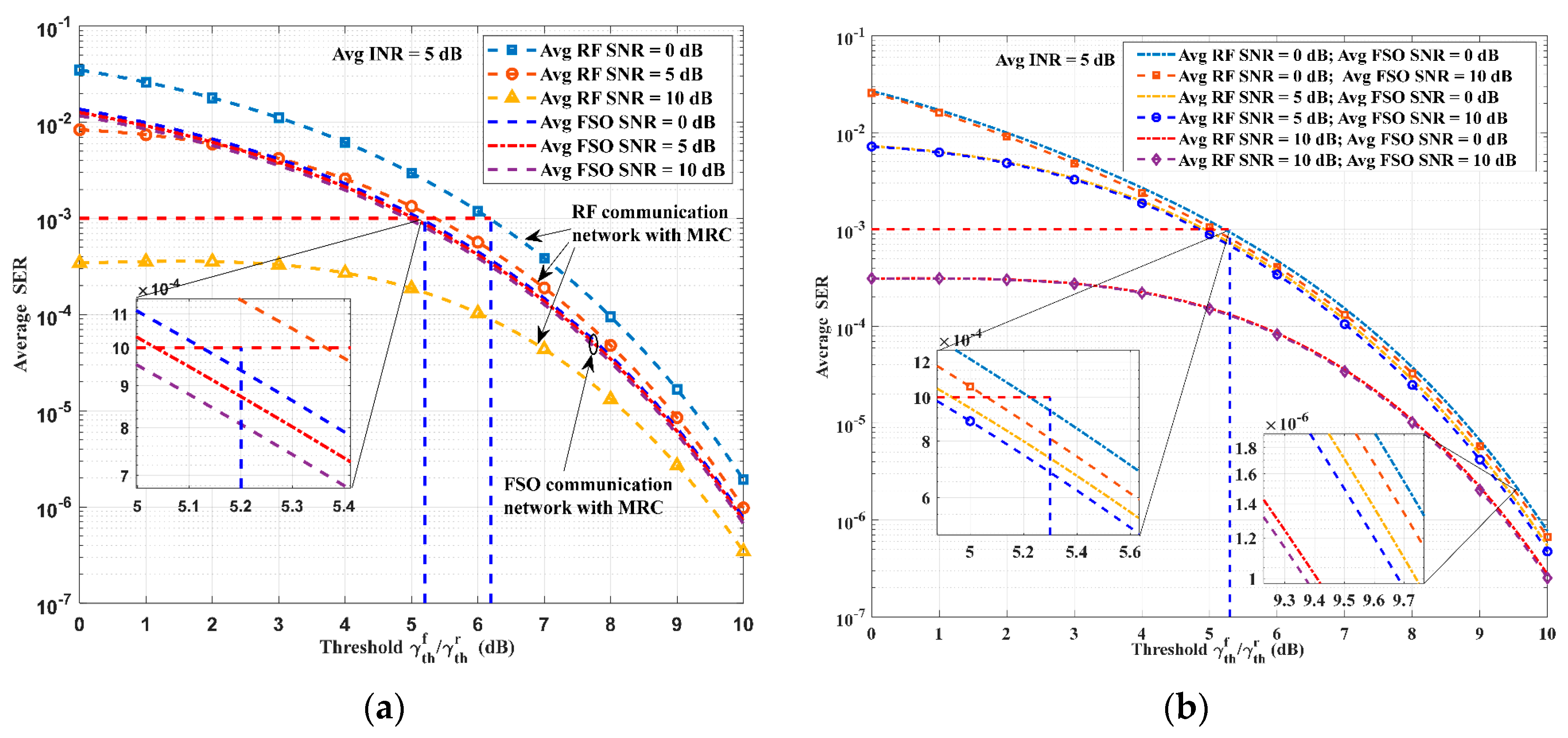
- We propose the ideal switching signal-to-interference-plus-noise ratio (SINR) values by plotting the average SER with respect to SINR threshold values. The ideal value is chosen to satisfy the target average SER value. The SINR value is determined based on the worst-case scenario to ensure it can fulfill communication requirements across different interference levels.
- We derive closed-form expressions for the OP and the average SER for the hybrid MMW RF/FSO communication network in the presence of CCI. RF and FSO links are analyzed using Nakagami-m and Μalaga models, respectively. Additionally, path loss and pointing error factors are considered, enhancing the models’ overall applicability. The exact expressions were verified using the Monte Carlo method.
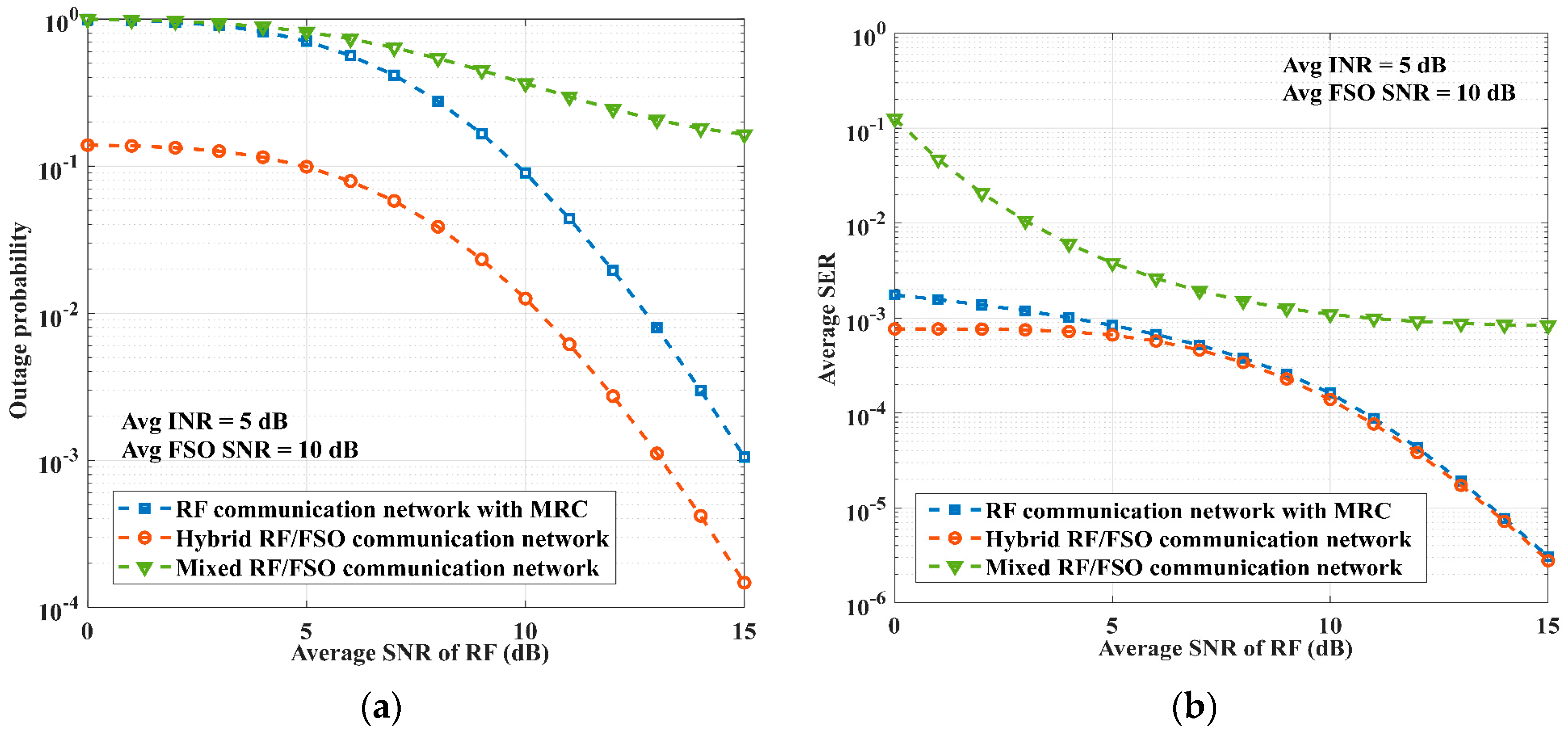
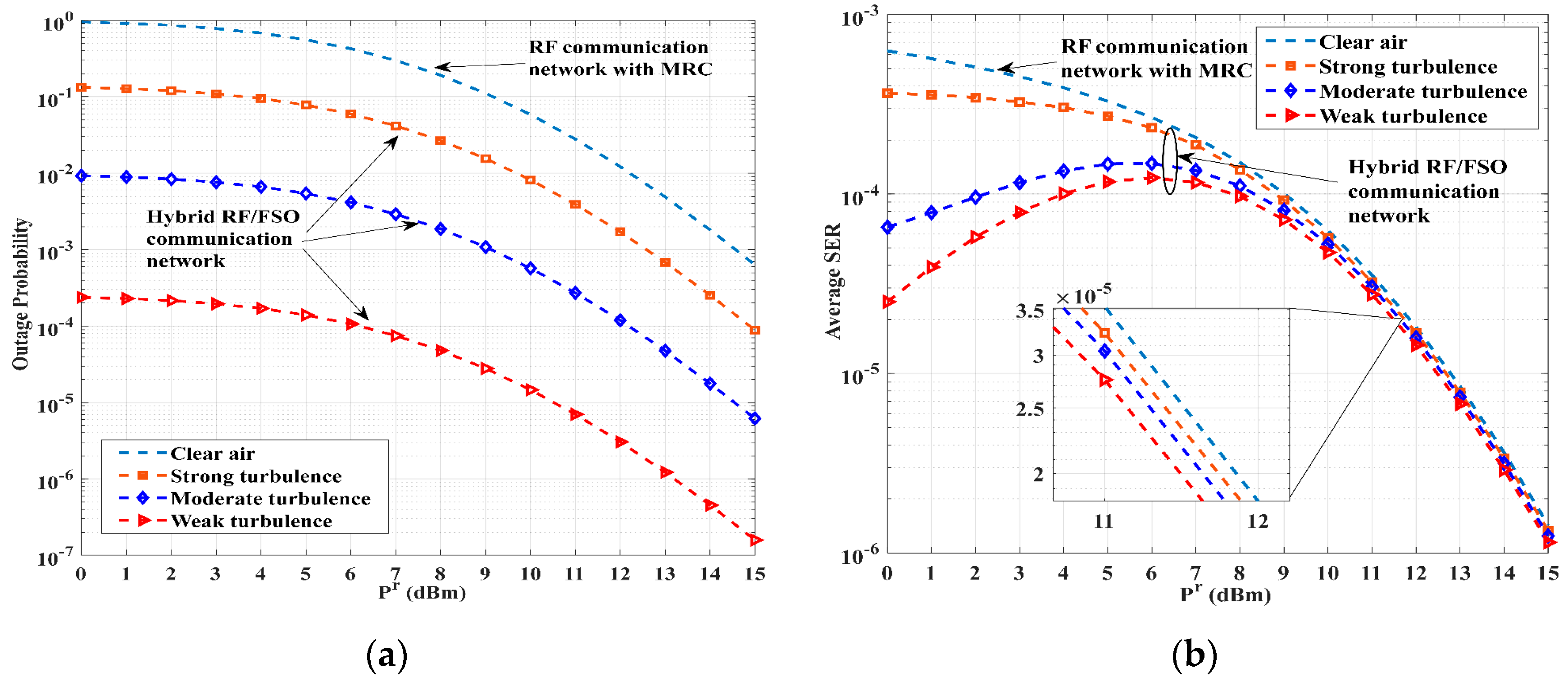
- We evaluated the effectiveness of a hybrid MMW RF/FSO communication network under various levels of interference, weather conditions, and turbulence. The simulation results indicate that the hybrid network can significantly improve overall performance when the RF link quality is less than optimal compared to a pure RF communication network.
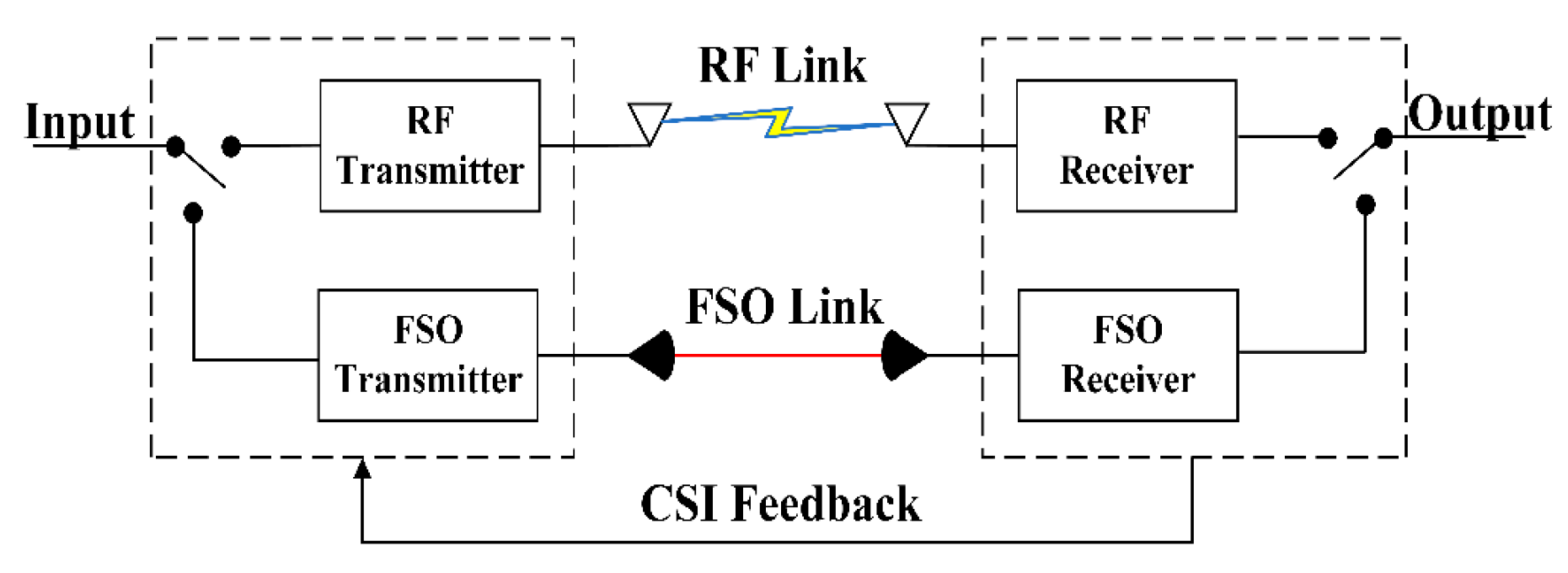
2. Network and Channel Models
2.1. MMW RF Channel Model
2.2. FSO Channel Model
3. Outage Probability Analysis
3.1. MMW RF Communication Subsystem
3.2. FSO Communication Subsystem
4. Average SER Analysis
5. Numerical Results and Discussions
5.1. SINR Switching Threshold
5.2. Network Performance Analysis
5.3. Effect of Weather Conditions on Performance
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Appendix A
Appendix B
Appendix C
Appendix C.1. Convergence Test for Equation (29)
Appendix C.2. Convergence Test for Equation (A6)
References
- Zhou, L.; Chen, X.; Hong, M.; Jin, S.; Shi, Q. Efficient Resource Allocation for Multi-UAV Communication Against Adjacent and Co-Channel Interference. IEEE Trans. Veh. Technol. 2021, 70, 10222–10235. [Google Scholar] [CrossRef]
- Zaheer, Z.; Usmani, A.; Khan, E.; Qadeer, M.A. Aerial surveillance system using UAV. In Proceedings of the 2016 Thirteenth International Conference on Wireless and Optical Communications Networks (WOCN), Hyderabad, India, 21–23 July 2016. [Google Scholar] [CrossRef]
- Waharte, S.; Trigoni, N. Supporting Search and Rescue Operations with UAVs. In Proceedings of the 2010 International Conference on Emerging Security Technologies, Canterbury, UK, 6–7 September 2010. [Google Scholar] [CrossRef]
- Rovira-Sugranes, A.; Razi, A.; Afghah, F.; Chakareski, J. A Review of AI-enabled Routing Protocols for UAV Networks: Trends, Challenges, and Future Outlook. Ad Hoc Netw. 2022, 130, 102790. [Google Scholar] [CrossRef]
- Mohsan, S.A.H.; Khan, M.A.; Noor, F.; Ullah, I.; Alsharif, M.H. Towards the Unmanned Aerial Vehicles (UAVs): A Comprehensive Review. Drones 2022, 6, 147. [Google Scholar] [CrossRef]
- Wang, H.; Wang, J.; Chen, J.; Gong, Y.; Ding, G. Network-Connected UAV Communications: Potentials and Challenges. China Commun. 2018, 15, 111–121. [Google Scholar]
- Wang, X.; Wang, J.; Xu, Y.; Chen, J.; Jia, L.; Liu, X.; Yang, Y. Dynamic Spectrum Anti-Jamming Communications: Challenges and Opportunities. IEEE Commun. Mag. 2020, 58, 79–85. [Google Scholar] [CrossRef]
- Liu, Y.C.; Wu, Z.Y.; Song, P.C. Online Trajectory Optimization for UAV-Assisted Hybrid FSO/RF Network with QoS-Guarantee. IEEE Commun. Lett. 2023, 27, 1357–1361. [Google Scholar] [CrossRef]
- Ge, X.; Tu, S.; Mao, G.; Wang, C.X.; Han, T. 5G Ultra-Dense Cellular Networks. IEEE Wirel. Commun. 2016, 23, 72–79. [Google Scholar] [CrossRef]
- Vishwakarma, N.; Swaminathan, S. On the maximal-ratio combining of FSO and RF links over generalized distributions and its applications in hybrid FSO/RF systems. Opt. Commun. 2022, 520, 128542. [Google Scholar] [CrossRef]
- Petkovic, M.I.; Cvetkovic, A.M.; Djordjevic, G.T.; Karagiannidis, G.K. Outage Performance of the Mixed RF/FSO Relaying Channel in the Presence of Interference. Wirel. Pers. Commun. 2017, 96, 2999–3014. [Google Scholar] [CrossRef]
- Trigui, I.; Cherif, N.; Affes, S.; Wang, X.; Leung, V.; Stephenne, A. Interference-limited mixed Málaga-M and generalized-K dual-hop FSO/RF systems. In Proceedings of the 2017 IEEE 28th Annual International Symposium on Personal, Indoor, and Mobile Radio Communications (PIMRC), Montreal, QC, Canada, 8–13 October 2017. [Google Scholar] [CrossRef]
- Soleimani-Nasab, E.; Uysal, M. Generalized Performance Analysis of Mixed RF/FSO Cooperative Systems. IEEE Trans. Wirel. Commun. 2016, 15, 714–727. [Google Scholar] [CrossRef]
- Wang, Z.; Shi, W.; Liu, W. Two-Way Mixed RF/FSO Relaying System in the Presence of Co-channel Interference. IEEE Photonics J. 2019, 11, 1–16. [Google Scholar] [CrossRef]
- Upadhya, A.; Meenalakshmi, M.; Chaturvedi, S.; Dwivedi, V.K. Full duplex mixed FSO/RF relaying systems with self-interference and outdated CSI. Opt. Quantum Electron. 2023, 55, 3. [Google Scholar] [CrossRef]
- Balti, E.; Guizani, M. Mixed RF/FSO Cooperative Relaying Systems with Co-Channel Interference. IEEE Trans. Commun. 2018, 66, 4014–4027. [Google Scholar] [CrossRef]
- Usman, M.; Hong-Chuan, Y.; Alouini, M.S. Practical Switching-Based Hybrid FSO/RF Transmission and Its Performance Analysis. IEEE Photonics J. 2014, 6, 1–13. [Google Scholar] [CrossRef]
- Shrivastava, S.K.; Sengar, S.; Singh, S.P. A new switching scheme for hybrid FSO/RF communication in the presence of strong atmospheric turbulence. Photonic Netw. Commun. 2019, 37, 53–62. [Google Scholar] [CrossRef]
- Alathwary, W.A.; Altubaishi, E.S. On the Performance Analysis of Decode-and-Forward Multi-Hop Hybrid FSO/RF Systems with Hard-Switching Configuration. IEEE Photonics J. 2019, 11, 1–12. [Google Scholar] [CrossRef]
- Sharma, S.; Madhukumar, A.S.; Swaminathan, R. Switching-based cooperative decode-and-forward relaying for hybrid FSO/RF networks. J. Opt. Commun. Netw. 2019, 11, 267. [Google Scholar] [CrossRef]
- Shrivastava, S.K.; Sengar, S.; Singh, S.P. On the Effect of Incorrect Channel Condition Information on Modified Switching Scheme of Hybrid FSO/RF System. IEEE Trans. Cogn. Commun. Netw. 2019, 5, 1208–1217. [Google Scholar] [CrossRef]
- Zhang, W.; Hranilovic, S.; Shi, C. Soft-switching hybrid FSO/RF links using short-length raptor codes: Design and implementation. IEEE J. Sel. Areas Commun. 2009, 27, 1698–1708. [Google Scholar] [CrossRef]
- He, B.; Schober, R. Bit-interleaved coded modulation for hybrid RF/FSO systems. IEEE Trans. Commun. 2009, 57, 3753–3763. [Google Scholar] [CrossRef]
- Laneman, J.N.; Tse, D.N.C.; Wornell, G.W. Cooperative diversity in wireless networks: Efficient protocols and outage behavior. IEEE Trans. Inf. Theory 2004, 50, 3062–3080. [Google Scholar] [CrossRef]
- Chatzidiamantis, N.D.; Karagiannidis, G.K.; Kriezis, E.E.; Matthaiou, M. Diversity Combining in Hybrid RF/FSO Systems with PSK Modulation. In Proceedings of the 2011 IEEE International Conference on Communications (ICC), Kyoto, Japan, 5–9 June 2011. [Google Scholar] [CrossRef]
- Jurado-Navas, A.; Garrido-Balsells, J.M.; Paris, J.F.; Castillo-Vázquez, M.; Puerta-Notario, A. Impact of pointing errors on the performance of generalized atmospheric optical channels. Opt. Express 2012, 20, 12550. [Google Scholar] [CrossRef] [PubMed]
- Ansari, I.S.; Yilmaz, F.; Alouini, M.S. Performance Analysis of Free-Space Optical Links Over Málaga Turbulence Channels with Pointing Errors. IEEE Trans. Wirel. Commun. 2016, 15, 91–102. [Google Scholar] [CrossRef]
- Farid, A.A.; Hranilovic, S. Outage Capacity Optimization for Free-Space Optical Links with Pointing Errors. J. Light. Technol. 2007, 25, 1702–1710. [Google Scholar] [CrossRef]
- The Wolfram Functions Site [Online]. Available online: https://functions.wolfram.com/ (accessed on 5 September 2023).
- Gradshteyn, I.S.; Ryzhik, I.M. Table of Integrals, Series, and Products, 7th ed.; Academic: San Diego, CA, USA, 2007. [Google Scholar]
- Prudnikov, A.; Brychkov, Y.; Marichev, O. Volume 3: More Special Functions. In Integrals and Series; CRC Press: Boca Raton, FL, USA, 1999. [Google Scholar]
- Ansari, I.; Al-Ahmadi, S.; Yilmaz, F.; Alouini, M.S.; Yanikomeroglu, H. A New Formula for the BER of Binary Modulations with Dual-Branch Selection over Generalized-K Composite Fading Channels. IEEE Trans. Commun. 2011, 59, 2654–2658. [Google Scholar] [CrossRef]




| Type | Ref. | Model | FSO | RF | Interference | Metrics |
|---|---|---|---|---|---|---|
| Mixed RF/FSO system | [11] | DH (RF-FSO) | Gamma-Gamma | Nakagami-m | Relay | OP |
| [12] | DH (FSO-RF) | Malaga | generalized-κ | Destination | OP, BER, EC | |
| [13] | DH (RF-FSO) Direct link (RF) | Double GG | Nakagami-m | Relay, Destination | OP, BEP | |
| [14] | Two-way DH (RF-FSO) | Gamma-Gamma | Nakagami-m | Relay, Destination | OP, BER | |
| [15] | Multi-relay DH (FSO-RF) | Malaga | α-μ | Relay | OP, BER, EC | |
| [16] | Multi-branch DH (RF-FSO) | Double GG | Nakagami-m | Relay | OP, BEP, EC | |
| Hybrid RF/FSO system | [17] | Single-link | Log-normal | Nakagami-m | Not included | OP, BER, EC |
| [18] | Single-link | Negative exponential | Rayleigh | Not included | OP, BER, | |
| [19] | MH | Gamma-Gamma | Nakagami-m | Not included | OP, EC | |
| [20] | DH and direct links | Gamma-Gamma | Nakagami-m | Not included | OP, SER |
| Parameters | Properties |
|---|---|
| Large-scale fading parameter, | |
| Small-scale fading parameter, | |
| The average power of the line of sight (LOS) term | |
| The average power of the scattering component received by off-axis eddies, | |
| The average power of the total scatter components | |
| Factor expressing the amount of scattering power coupled to the LOS component, | |
| Deterministic phases of the LOS terms | |
| Deterministic phases of the coupled-to-LOS scatter terms | |
| Fraction of the collected power at | |
| Pointing error coefficient, | |
| Equivalent beam waist |
| RF Parameter | Symbol | Value | |
| Carrier frequency | 60 GHz | ||
| Transmit antenna gain | 44 dBi | ||
| Receive antenna gain | 44 dBi | ||
| Attenuation (oxygen) | 15.1 dB/km | ||
| Noise variance | |||
| FSO Parameter | Symbol | Value | |
| Wavelength | 1550 nm | ||
| Noise variance | |||
| Responsivity | 0.5 A/W | ||
| Average power (total scatter) | 0.1079 | ||
| Average power (LOS) | 1.3265 | ||
| Phase difference | |||
| Jitter variance | 0.3 m | ||
| Laser beam waist | 0.025 m | ||
| Receiver aperture radius | 0.1 m | ||
| Weather-dependent parameters of FSO and RF channels | |||
| Weather conditions | (dB/km) | (dB/km) | () |
| Clear air | 0.43 | 0 | |
| Haze | 4.2 | 0 | |
| Rain | 5.8 | 5.6 | |
| RF Communication Network vs. Hybrid RF/FSO Communication Network () | ||||
|---|---|---|---|---|
| 23.2% | ||||
| 13.3% | ||||
| 8.7% | ||||
| 58.8% | ||||
| 28.8% | ||||
| 17.6% | ||||
| 60.0% | ||||
| 44.6% | ||||
| 19.8% | ||||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, X.; Zhao, S.; Wang, X.; Li, X.; Tian, Q. Performance Analysis of UAV RF/FSO Co-Operative Communication Network with Co-Channel Interference. Drones 2024, 8, 70. https://doi.org/10.3390/drones8030070
Song X, Zhao S, Wang X, Li X, Tian Q. Performance Analysis of UAV RF/FSO Co-Operative Communication Network with Co-Channel Interference. Drones. 2024; 8(3):70. https://doi.org/10.3390/drones8030070
Chicago/Turabian StyleSong, Xinkang, Shanghong Zhao, Xiang Wang, Xin Li, and Qin Tian. 2024. "Performance Analysis of UAV RF/FSO Co-Operative Communication Network with Co-Channel Interference" Drones 8, no. 3: 70. https://doi.org/10.3390/drones8030070
APA StyleSong, X., Zhao, S., Wang, X., Li, X., & Tian, Q. (2024). Performance Analysis of UAV RF/FSO Co-Operative Communication Network with Co-Channel Interference. Drones, 8(3), 70. https://doi.org/10.3390/drones8030070






