Freeform Optimization of an Ultrasonic Horn Coupled to an Airborne MEMS Transducer ^†

Abstract

We designed an ultrasonic horn using a physics-based freeform optimization method to achieve the target frequency response of an airborne ultrasonic MEMS transducer operating in transmitting mode. The radial profile of the ultrasonic horn was parametrized using a Bezier curve, and its shape was optimized using a genetic algorithm. A computationally fast compact model of the full system, wherein the horn was described analytically using transmission line theory, was used to calculate the frequency response of the transducer and evaluate the optimization objective. The result shows very good agreement with the experimental measurement of a realized prototype.

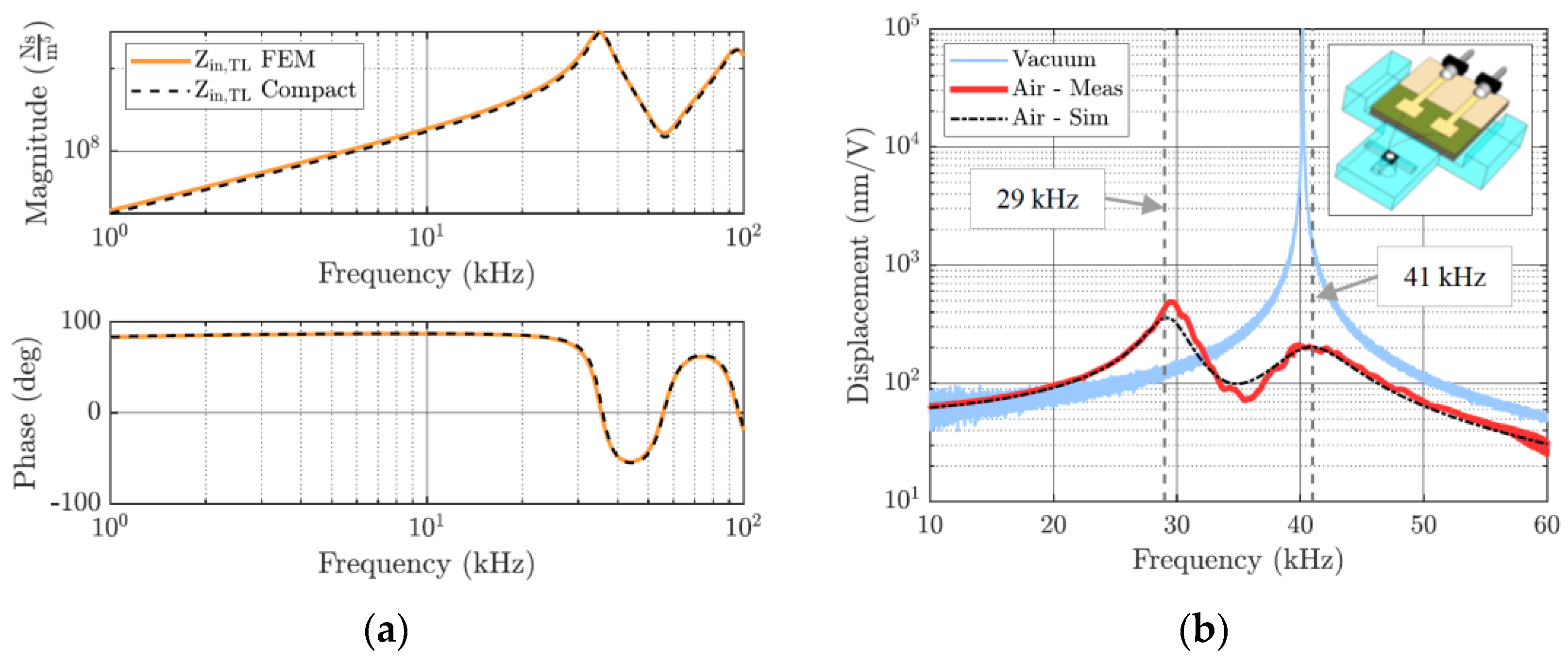
1. Introduction

MEMS-based airborne ultrasonic systems have gained popularity due to their compactness, low power consumption, and low-cost applications in ranging and gesture recognition. It is well known that the coupling between the device and the acoustic surroundings defined by the package has a significant impact on the dynamic behavior of membrane-based ultrasonic transducers [1]. Figure 1a shows dynamic measurements of an electrically actuated piezoelectric membrane attached to a cylindrical horn. One can recognize that the transducer, air, and horn form a coupled oscillator system whose resonances define the optimal operating frequencies of the ultrasonic system. Hence, a specifically designed horn shape enables us to tailor the system frequency response to a specific target response.

2. Methods

As a first step, the radial profile of the horn is parametrized by Bezier curves, which provide a flexible yet controllable description of the geometry and allow for the definition of a freeform and continuous radial profile by introducing a limited number of control points (Figure 1b). Moreover, this mathematical framework enables a clear definition of the start and end points of the curve, which is necessary to match the throat radius of the horn to the etched cavity of the chip and to set a defined mouth radius. In the presented example, we define control points, which are equally spaced over the length of the horn; the more control points, the more complex the geometries. In this work, the goal is to find a horn geometry which tunes the system response to exhibit two resonance peaks at 29 kHz and 41 kHz. A genetic algorithm is employed to search for the optimal freeform control points, which are used to calculate the new horn geometry at each evaluation step. The optimization goal, i.e., the desired displacement frequency response, is evaluated for each horn geometry by applying an analytical, physics-based compact model, where the input acoustic impedance of the horn Zin,TL is determined by a discretization approach based on transmission line theory [2]. The optimal design is extracted by minimizing the error between the simulated and the target resonance frequencies. The full-analytical nature of the whole optimization loop ensures very fast computation times and consistent convergence.

3. Discussion

Because of the exotic nature of the shape of the optimized horn (see Figure 1b), we checked the agreement between the input acoustic impedance calculated by the compact models with FEM simulations (see Figure 2a). Figure 2b shows the displacement response of the optimized and analytically predicted system design. It meets the target values very well, which is also confirmed by measurements of a prototype. The exceptional results of this study demonstrate the feasibility and predictive power of the presented optimization method and, therefore, lay the foundation towards tailoring the frequency response of airborne MEMS transducers by ultrasonic horns.