A Note on the “Optimal” Design of Disc-Shaped Heat Exchangers
Abstract
:1. Introduction
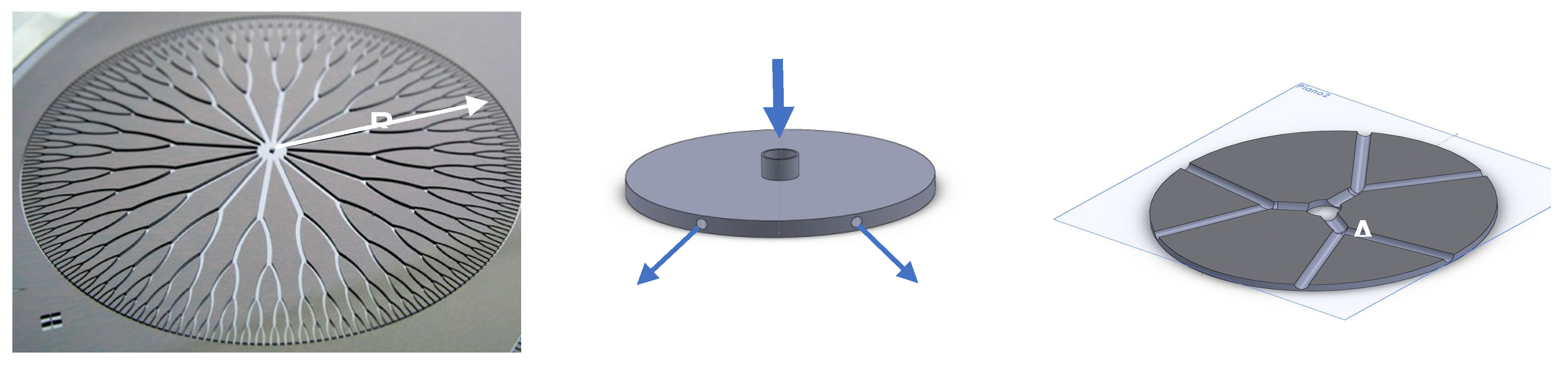
2. The Physiology of a Disc Heat Exchanger as a Function of Its Operational Mode
- i -
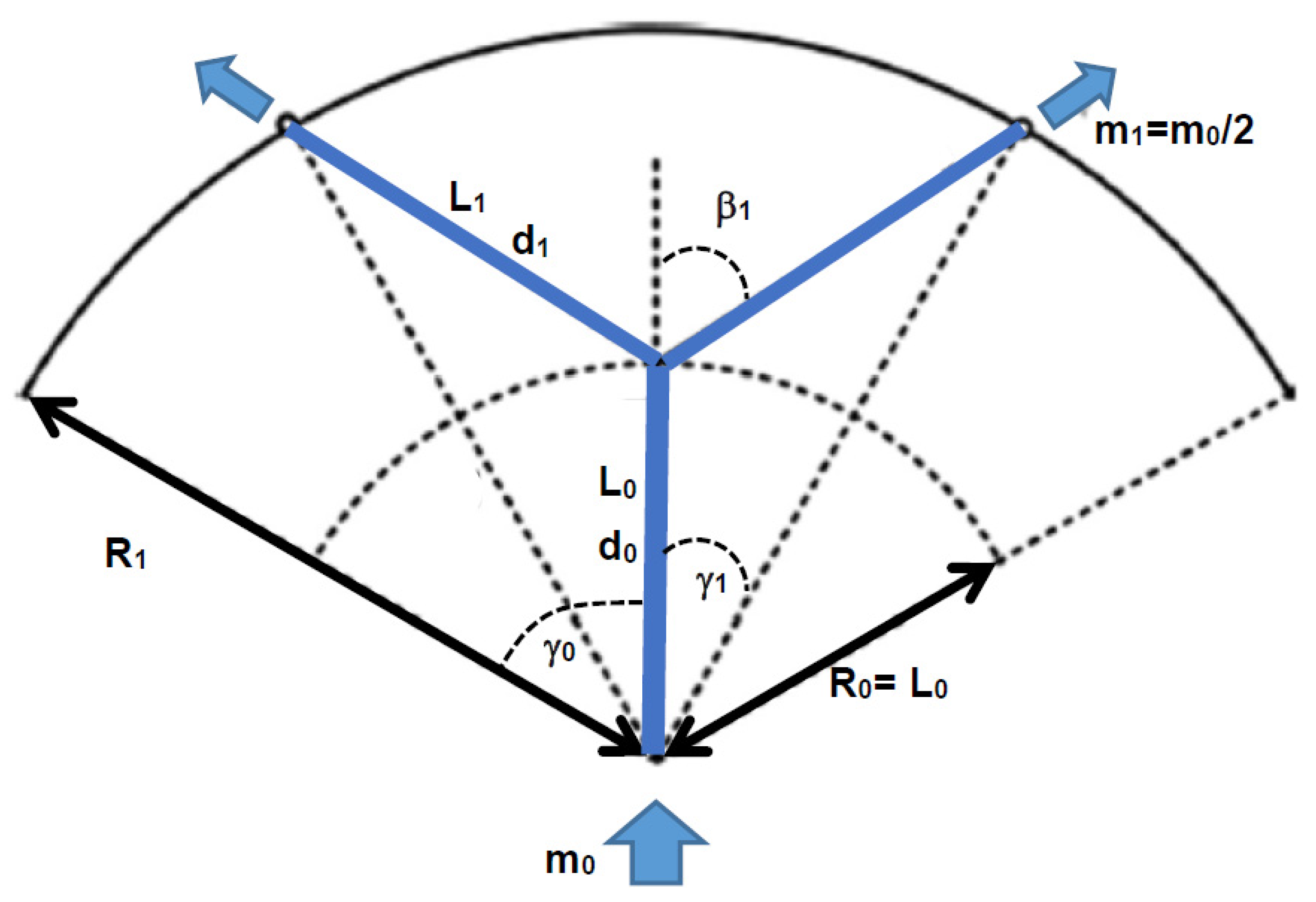
- the ratio of the diameters of successive branches, δj = dj+1/dj;
- ii -
- the ratio of successive lengths, λj = Lj+1/Lj;
- iii -
- the shape ratio defined as the ratio of the diameter of the first branch to the disc radius, δ0=d0/R;
- iv -
- the initial number of “sectors” in which the disc is subdivided, z0;
- v -
- the total number of branchings, zb.
- (a)
- The thermal flux qin the DSHE receives by conduction or electrical input -if any;
- (b)
- The inlet and outlet coolant temperatures Tin and Tout;
- (c)
- The final ΔT between the disc and the fluid: TD-Tout;
- (d)
- The average temperature Text of the immediate surroundings;
- (e)
- The density ρf, specific heat cp,f, viscosity μf of the coolant;
- (f)
- The density ρD and specific heat cp,D of the disc material;
- (g)
- An average heat transfer coefficient for the convection on the outside surface of the disc, hext if present.
3. The Correct Design Procedure Leads to an Underspecified Problem
4. The Proper Constraints
5. Examples of Application
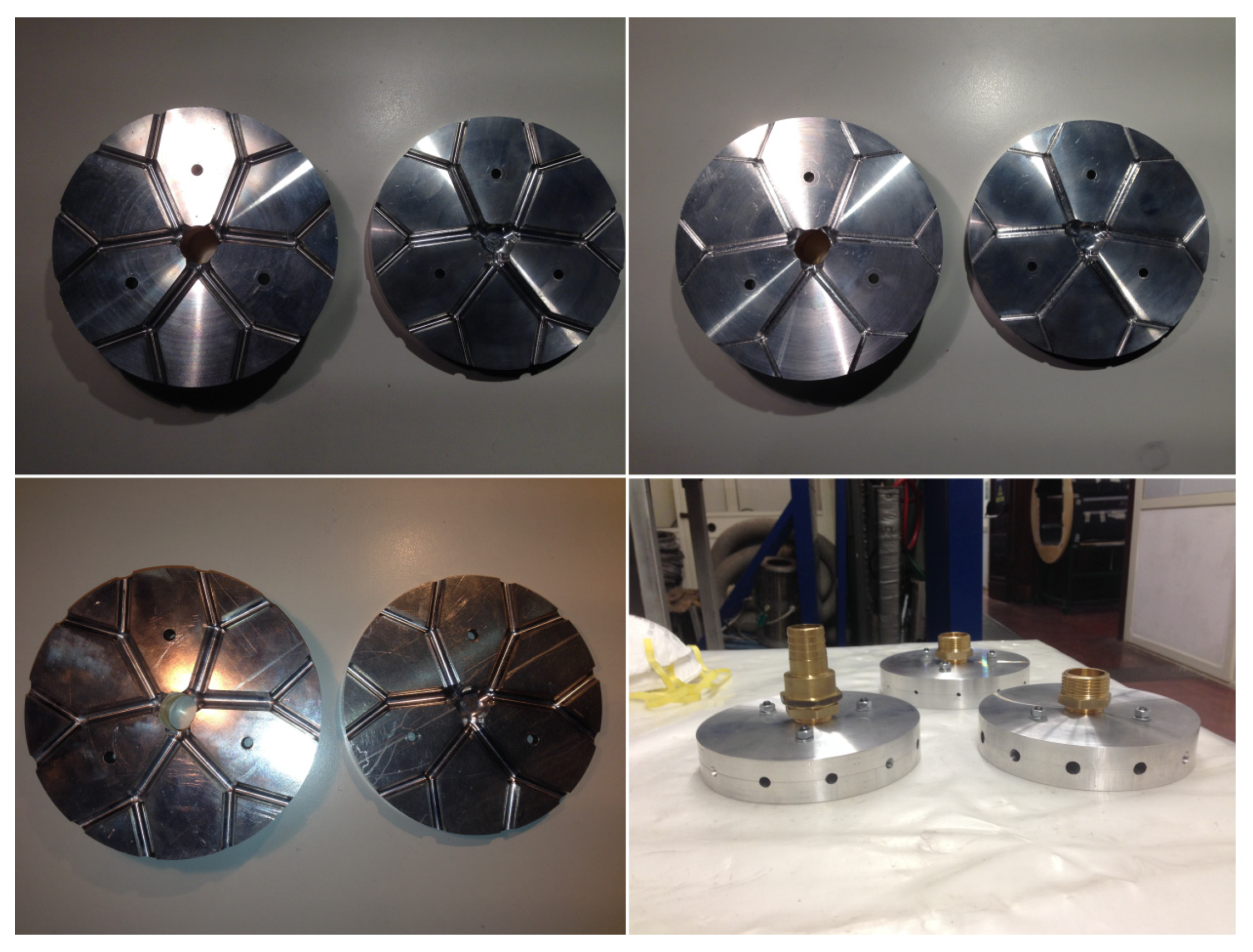
5.1. Comparison of Possible Dshe Configurations for an Oil Cooler
- (a)
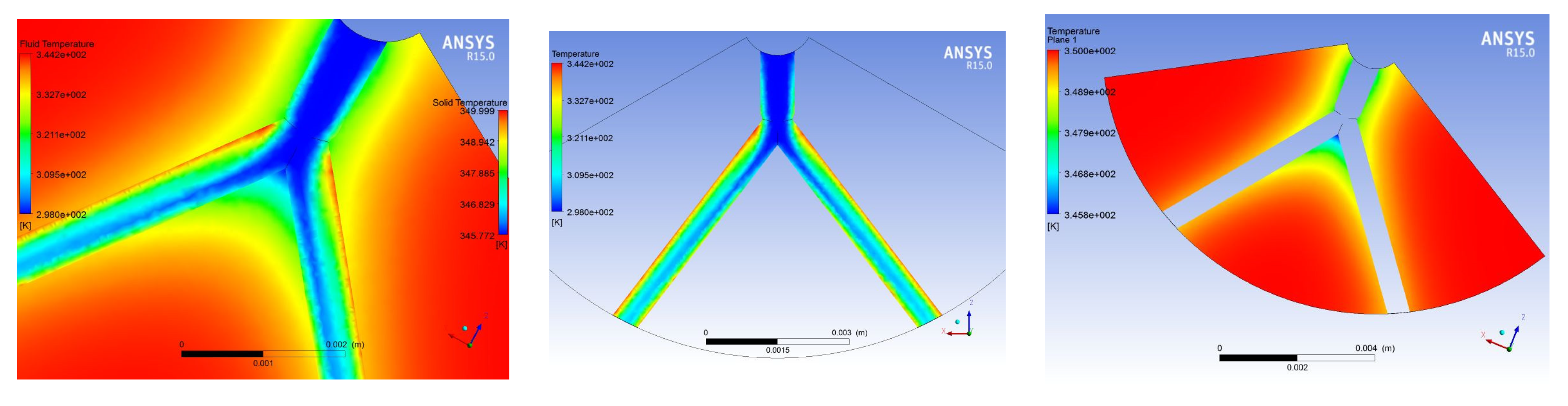
- The 3 branches at level 0 are far too short, and therefore, the temperature of the portion of the disc within the radius R0 (refer to Figure 6) is excessively high (the heat exchange area z0πd0L0 is too small, even if the Nu0 is rather high);
- (b)
- Since the branches at levels 1 and 2 do not respect the constant-Re prescription (, i.e., constant velocity in successive branches), there is a disuniformity in the heat transfer (and therefore, in the disc bulk temperature) between R1 and R2;
- (c)
- The κg is not the same in branches 1 and 2, and this adds up to the non-constant Re effect, increasing the disuniformity in the disc body.
5.2. Selection of the DSHE Configuration for the Cooling of an Electronic Chip
- (a)
- The level 0 branch is too short: the flow is not completely developed, and the Nu is high, but, as in the case examined in Section 5.1 above, the heat transfer is not optimal (the portion of disc within the circle R0 is too hot);
- (b)
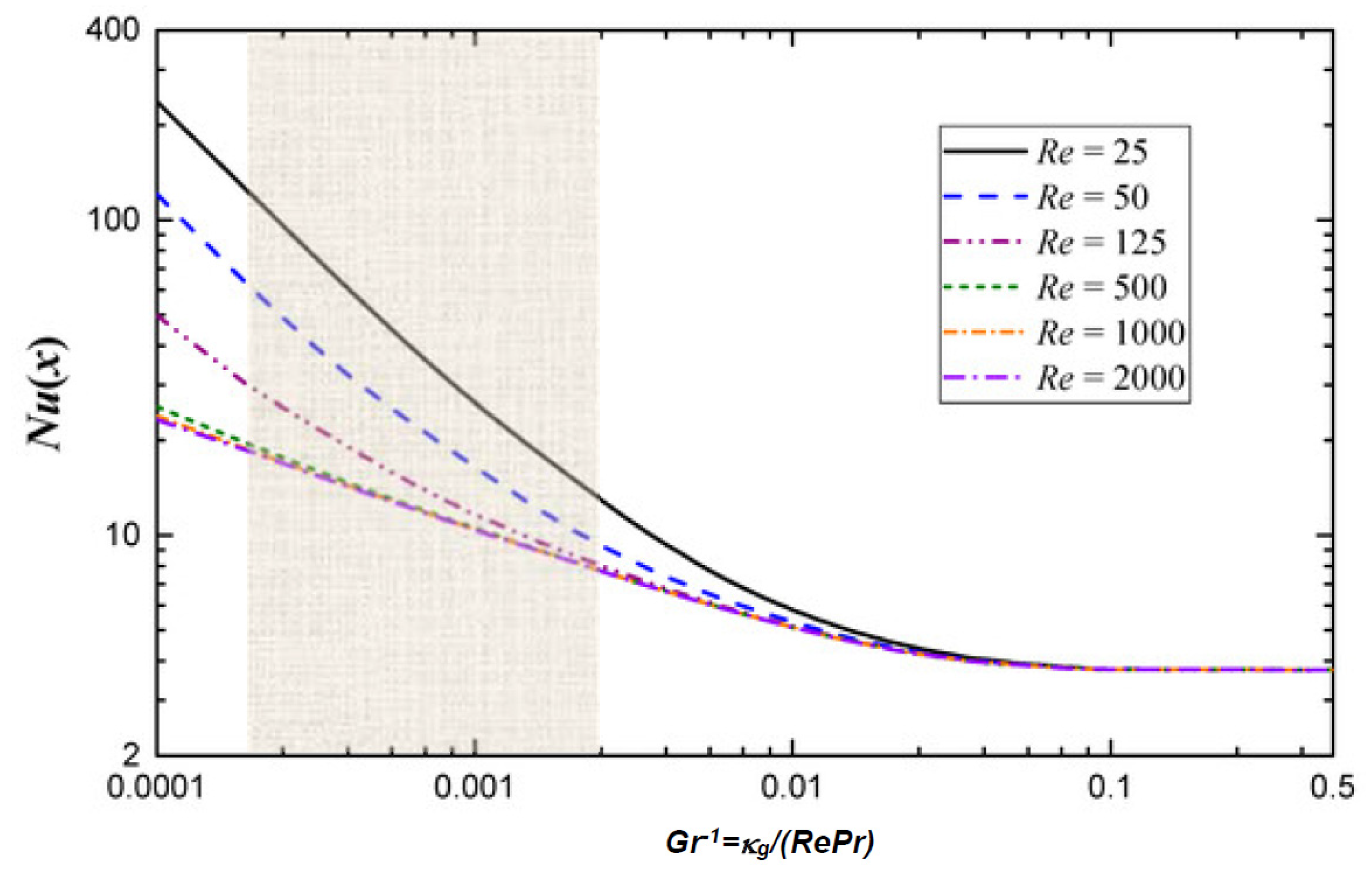
- The branches at level 1 are, on the contrary, too long, and the flow becomes fully developed towards the end of the channels, lowering the overall Nu1;
- (c)
- In spite of the above shortcomings, the temperature of the disc is approximately constant, validating the assumption made in Section 2 as to the external convection loss.
6. Conclusions
References
- Boichot, R.; Fan, Y. A genetic algorithm for topology optimization of area-to-point heat conduction problem. Int. J. Thermal Sci. 2016, 108, 209–217. [Google Scholar] [CrossRef]
- Feng, H.-J.; Chen, L.-G.; Xie, Z.-H.; Sun, F.R. Constructal entropy generation rate minimization for asymmetric vascular networks in a disc-shaped body. Int. J. Heat Mass Transf. 2015, 91, 1010–1017. [Google Scholar] [CrossRef]
- Hess, W.R. Eine mechanisch bedingte Gesetzmäßigkeit im Bau des Blutgefäßsystems, (A mechanically-based general rule for the construction of the blood circulatory system). Arch. Entwicklungsmech. Org. 1903, 16, 632–641. [Google Scholar] [CrossRef]
- Miguel, A.F. A study of entropy generation in tree-shaped flow structures. Int. J. Heat Mass Transf. 2016, 92, 349–359. [Google Scholar] [CrossRef]
- Murray, C.D. The physiological principle of minimum work applied to the angle of branching of arteries. J. General Physiol. 1926, 9, 835–841. [Google Scholar] [CrossRef]
- Murray, C.D. The physiological principle of minimum work—I: The vascular system and the cost of blood volume. Proc. Natl. Acad. Sci. USA 1926, 12, 207–214. [Google Scholar] [CrossRef]
- Hess, W.R. Über die periphere Regulierung der Blutzirkulation, (On the peripheral regulatory actions of blood circulation), Pflügers Archiv für die gesamte Physiologie des Menschen und der Tiere, Aug 1917, v.168, n.9, 439–490.
- Murray, C.D. The physiological principle of minimum work—II: Oxygen exchange in capillaries. Proc. Natl. Acad. Sci. USA 1926, 12, 299–304. [Google Scholar] [CrossRef]
- Robbe, M.; Sciubba, E.; Bejan, A.; Lorente, S. Numerical analysis of a tree-shaped cooling structure for a 2-D slab: A validation of a “constructally optimal” configuration. In Proceedings of the ESDA-ASME 8th Conference, Torino, Italy, 4–7 July 2006. [Google Scholar]
- Sciubba, E. A Critical Reassessment of the Hess–Murray Law. Entropy 2016, 18, 283–300. [Google Scholar] [CrossRef]
- Wechsatol, W.; Lorente, S.; Bejan, A. Dendritic convection on a disc. Int. J. Heat Mass Transf. 2003, 46, 4381–4391. [Google Scholar] [CrossRef]
- Sciubba, E. Entropy Generation Minimization as a Design Tool. Part 1: Analysis of different configurations of branched and non-branched laminar isothermal flow through a circular pipe. Int. J. Thermodyn. 2011, 14, 11–20. [Google Scholar]
- Bejan, A. Constructal-theory network of conducting paths for cooling a heat generating volume. Int. J. Heat Mass Transf. 1997, 40, 799–816. [Google Scholar] [CrossRef]
- Capata, R.; Beyene, A. Experimental evaluation of three different configurations of constructal disc-shaped heat exchangers. Int. J. Heat Mass Transf. 2017, 115, 92–101. [Google Scholar] [CrossRef]
- da Silva, A.K.; Bejan, A. Dendritic counterflow heat exchanger experiments. Int. J. Thermal Sci. 2006, 45, 860–869. [Google Scholar] [CrossRef]
- Su, L.-B.; Duan, Z.-P.; He, B.-S.; Ma, H.; Xu, Z. Thermally Developing Flow and Heat Transfer in Elliptical Minichannels with Constant Wall Temperature. Micromachines 2019, 10, 713–728. [Google Scholar] [CrossRef] [PubMed]
- Wechsatol, W.; Lorente, S.; Bejan, A. Optimal tree-shaped networks for fluid flow in a disc-shaped body. Int. J. Heat Mass Transf. 2002, 45, 4911–4924. [Google Scholar] [CrossRef]
- Bejan, A.; Alalaimi, M.; Sabau, A.S.; Lorente, S. Entrance-length dendritic plate heat exchangers. Int. J. Heat Mass Transf. 2017, 114, 1350–1356. [Google Scholar] [CrossRef]
- Bejan, A.; Sciubba, E. The optimal spacing of parallel plates cooled by forced convection. Int. J. Heat Mass Transf. 1992, 35, 3259–3264. [Google Scholar] [CrossRef]
- Bejan, A.; Lorente, S. Constructal theory of energy-system and environment flow configurations. Int. J. Exergy 2005, 2, 335–347. [Google Scholar] [CrossRef]
- Luo, L. (Ed.) Heat and Mass Transfer Intensification and Shape Optimization: A Multi-scale Approach; Springer: London, UK, 2013. [Google Scholar]
- Mereu, S.; Sciubba, E.; Bejan, A. The optimal cooling of a stack of heat generating boards with fixed pressure drop, flowrate or pumping power. Int. J. Heat Mass Transf. 1993, 6, 3677–3686. [Google Scholar] [CrossRef]
- Qureshi, M.U.; Vaughan, G.D.A.; Sainsbury, C.; Johnson, M.; Peskin, C.S.; Olufsen, M.S.; Hill, N.A. Numerical simulation of blood flow and pressure drop in the pulmonary arterial and venous circulation. Biomech. Model. Mechanobiol. 2014, 13, 1137–1154. [Google Scholar] [CrossRef]
- Robbe, M.; Sciubba, E. Derivation of the optimal internal cooling geometry of a prismatic slab: Comparison of constructal and non-constructal geometries. Energy 2009, 34, 2167–2174. [Google Scholar] [CrossRef]
- West, G.B.; Brown, J.H. The origin of allometric scaling laws in biology from genomes to ecosystems: Towards a quantitative unifying theory of biological structure and organization. J. Exp. Biol. 2005, 208, 1575–1592. [Google Scholar] [CrossRef] [PubMed]
- Zamir, M.; Wrigley, S.M.; Langille, B.L. Arterial bifurcations in the cardiovascular system of a rat. J. General Phys. 1983, 81, 325–335. [Google Scholar] [CrossRef] [PubMed]
- Errera, M.R.; Bejan, A. Deterministic tree networks for river drainage basins. Fractals 1998, 6, 245–261. [Google Scholar] [CrossRef]
- Sherman, T.F. On Connecting Large Vessels to Small: The Meaning of Murray’s Law. J. General. Physiol. 1981, 78, 431–453. [Google Scholar] [CrossRef]
- Roy, A.G.; Woldenberg, M.J. A generalization of the optimal models of arterial branching. Bull. Math. Biol. 1982, 44, 349–360. [Google Scholar] [CrossRef]
- Sciubba, E. Entropy Generation Minima in Different Configurations of the Branching of a Fluid-Carrying Pipe in Laminar Isothermal Flow. Entropy 2010, 12, 1855–1866. [Google Scholar] [CrossRef]
- Cancellario, A. Waste Heat Recovery System at Nanoscales: Process Simulation, Optimization, Design of Heat Exchanger. Master’s Thesis, U. Roma Sapienza, Rome, Italy, 2017. [Google Scholar]

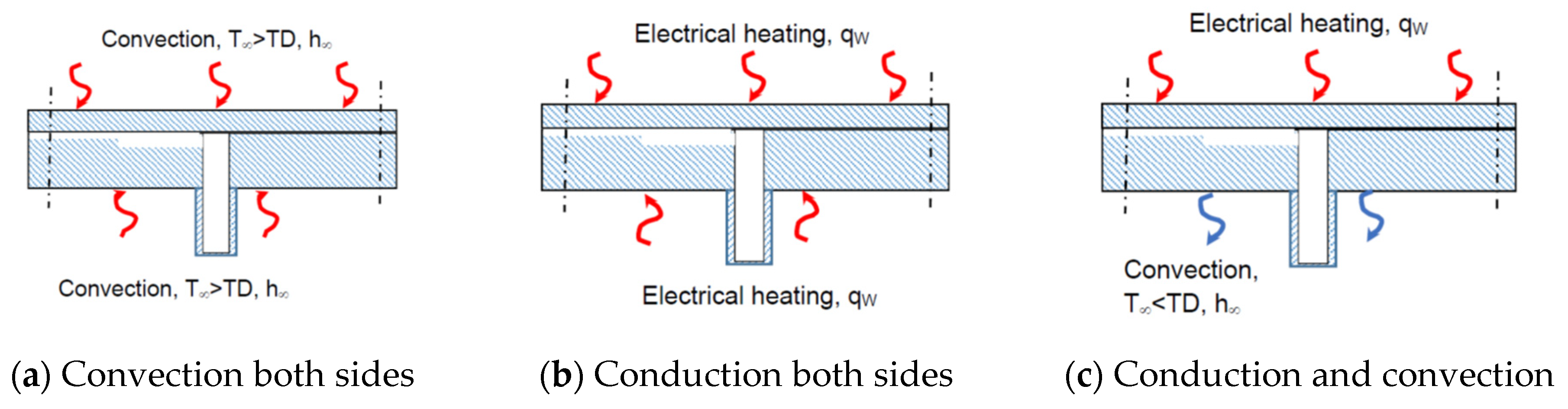

| Type of Operation (Heater) | Needed Design Specifications | Qdes |
|---|---|---|
| Heat input by convection on both sides | hext, Text, TD | |
| Electrical or conduction heating on both sides | qin, sD, kD | |
| Electrical or conduction heating on one surface, cooling by free convection on the opposite one | hext, Text, TD, qin, sD, kD |
| Disc radius, m | 0.075 | Oil mass flow rate, kg/s | 2.17 × 10−3 |
| Disc thickness, m | 0.015 | Oil density at inlet, kg/m3 | 920 |
| Disc conductivity k, W/(mK) | 190 | Oil viscosity, νoil,323K, m2/s | 6.7 × 10−5 |
| Texternal air, K | 293 | Toil,in, K | 301 |
| External air viscosity νair, m2/s | 1.56 × 10−5 | Toil,out, K | 349 |
| Disc radius, m | 0.015 | Air mass flow rate, kg/s | 1.27 × 10−4 |
| Disc thickness, m | 0.0014 | Air density at inlet, kg/m3 | 1.157 |
| Disc conductivity k, W/(mK) | 120 | Air viscosity νair,298, m2/s | 1.56 × 10−5 |
| External h.t. coefficient h, W/(m2K) | 30 | Tair,in, K | 298 |
| Texternal air, K | 293 | Tair,out, K | 324 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sciubba, E. A Note on the “Optimal” Design of Disc-Shaped Heat Exchangers. Proceedings 2020, 58, 8. https://doi.org/10.3390/WEF-06904
Sciubba E. A Note on the “Optimal” Design of Disc-Shaped Heat Exchangers. Proceedings. 2020; 58(1):8. https://doi.org/10.3390/WEF-06904
Chicago/Turabian StyleSciubba, Enrico. 2020. "A Note on the “Optimal” Design of Disc-Shaped Heat Exchangers" Proceedings 58, no. 1: 8. https://doi.org/10.3390/WEF-06904
APA StyleSciubba, E. (2020). A Note on the “Optimal” Design of Disc-Shaped Heat Exchangers. Proceedings, 58(1), 8. https://doi.org/10.3390/WEF-06904





