The Role of Friction and Tangential Compliance on the Resultant Launch Angle of a Golf Ball †
Abstract
:1. Introduction
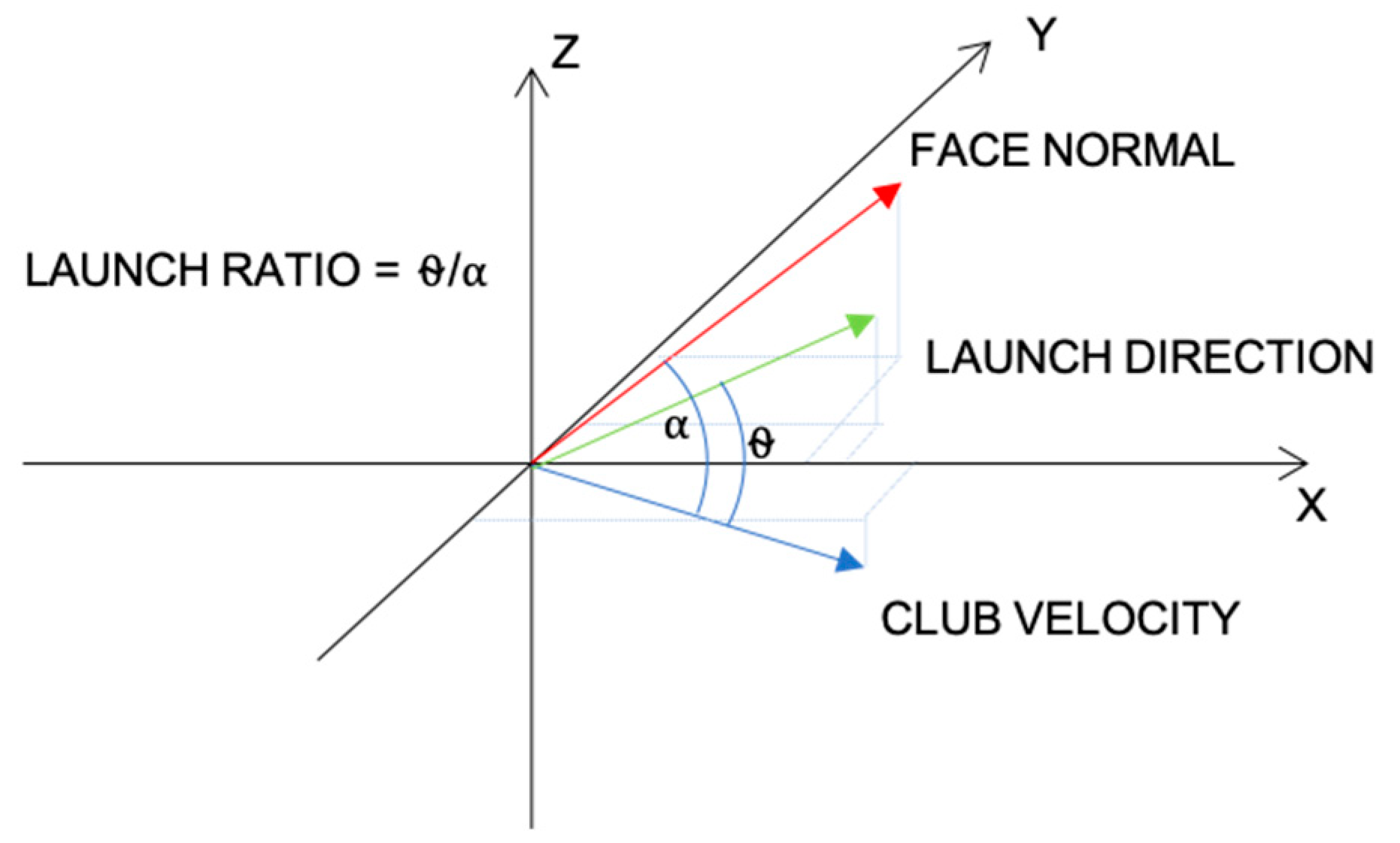
2. Theoretical Model
2.1. Overview
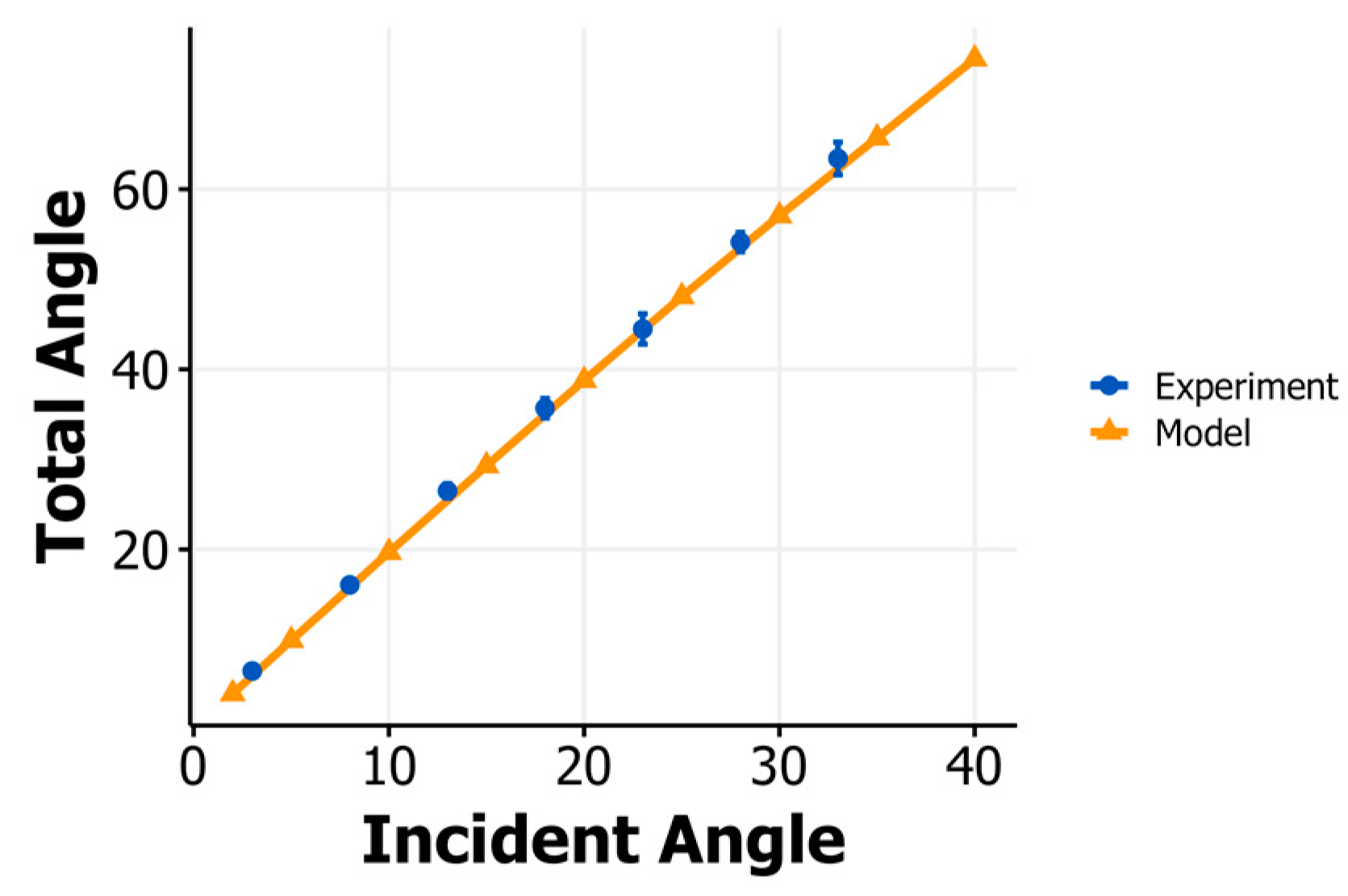
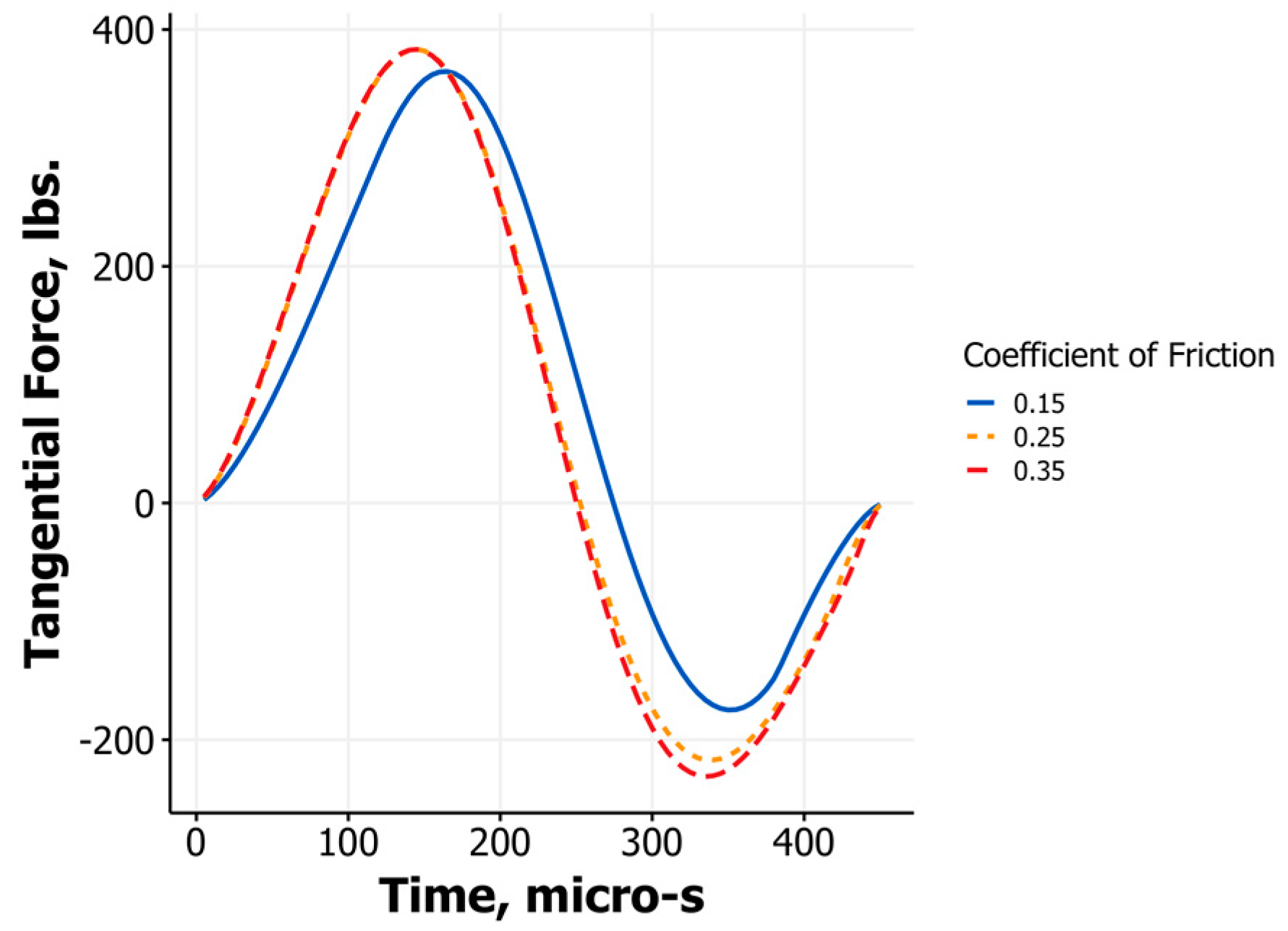
2.2. Validation
3. Experimental Methods
3.1. Motion Capture Testing
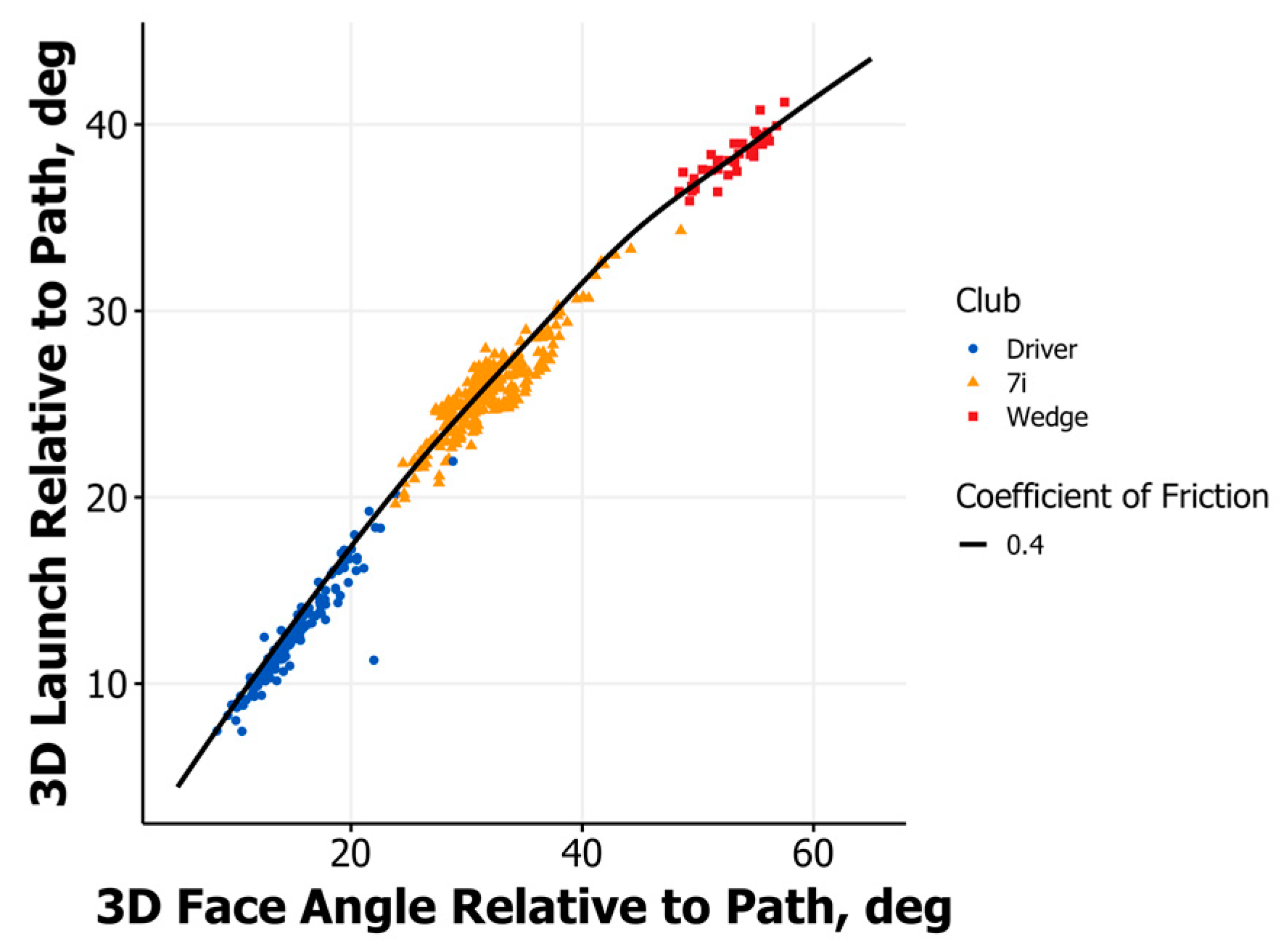
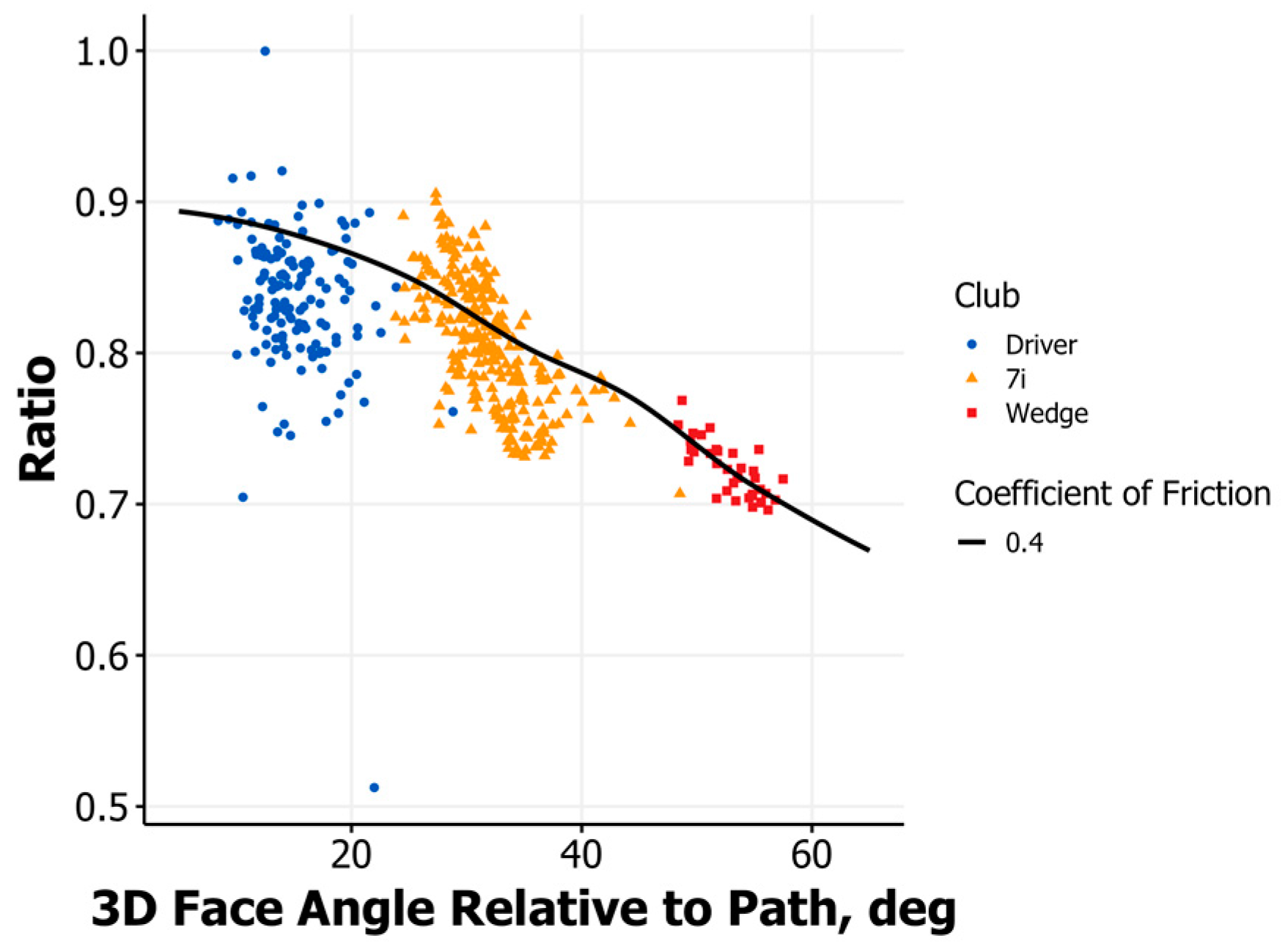
4. Results
5. Discussion
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Cochran, A.; Stobbs, J. Search for the Perfect Swing; Lippincott: Philadelphia, PA, USA, 1968. [Google Scholar]
- Jorgensen, T. The Physics of Golf; Springer Science: Philadelphia, PA, USA, 1999. [Google Scholar]
- Penner, A. The physics of golf. Rep. Prog. Phys. 2002, 66, 121. [Google Scholar] [CrossRef]
- Dewhurst, P. The Science of the Perfect Swing, 1st ed.; Oxford University Press: New York, NY, USA, 2015. [Google Scholar]
- Broadie, M. Every Shot Counts; Penguin Books: New York, NY, USA, 2014. [Google Scholar]
- Wood, P.; Henrikson, E.; Broadie, C. The influence of face angle and club path on the resultant launch angle of a golf ball. In Proceedings of the 12th Conference of the International Sports Engineering Association, Brisbane, Australia, 26–29 March 2018; Volume 2. [Google Scholar]
- Cornish, J.; Otto, S.; Strangwood, M. Modelling the Oblique Impact of Golf Balls (P128). Eng. Sport 2008, 7, 669–675. [Google Scholar]
- Arakawa, K.; Mada, T.; Komatsu, H.; Shimizu, T.; Satou, M.; Takehara, K.; Etoh, G. Dynamic deformation behavior of a golf ball during normal impact. Exp. Mech. 2009, 49, 471–477. [Google Scholar] [CrossRef]
- Cross, R.; Dewhurst, P. Launch speed, angle and spin in golf. Eur. J. Phys. 2018, 39, 065003. [Google Scholar] [CrossRef]
- Kensrud, J.R.; Nathan, A.M.; Smith, L.V. Oblique collisions of baseballs and softballs with a bat. Am. J. Phys. 2017, 85, 503–509. [Google Scholar] [CrossRef]
- Rokach, I.; Shimizu, T.; Takahashi, K.; Komatsu, H.; Satoh, M. Science and Golf IV; Routledge: Abingdon, UK, 2002; pp. 515–523. [Google Scholar]
- Roh, W.; Lee, C. Correlation Between the Linear Impulse and Golf Ball Spin Rate (P35). Eng. Sport 2008, 7, 199–206. [Google Scholar]
- Johnson, K. Contact Mechanics; Cambridge University Press: Cambridge, UK, 1985. [Google Scholar]
- Maw, N.; Barber, J.R.; Fawcett, J.N. The oblique impact of elastic spheres. Wear 1976, 38, 101–114. [Google Scholar] [CrossRef]
- Maw, N.; Barber, J.R.; Fawcett, J.N. The role of elastic tangential compliance in oblique impact. J. Lubr. Technol. 1981, 103, 74. [Google Scholar] [CrossRef]
- United States Golf Association. Interim Report on Study of Spin Generation; Tech. Rep.; United States Golf Association: Far Hills, NJ, USA, 2006. [Google Scholar]
- Leach, R.; Forrester, S.; Smith, A.; Roberts, J. How valid and accurate are measurements of golf impact parameters obtained using commercially available radar and stereoscopic optical launch monitors? Measurement 2017, 112, 125. [Google Scholar] [CrossRef]


| Club | Trackman, % | Wood, et al., % |
|---|---|---|
| Driver | 87 | 83 ± 8 |
| 7-iron | 75 | 81 ± 5 |
| Pitching wedge | 70 | 72 ± 6 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Henrikson, E.; Wood, P.; Broadie, C.; Nuttall, T. The Role of Friction and Tangential Compliance on the Resultant Launch Angle of a Golf Ball. Proceedings 2020, 49, 27. https://doi.org/10.3390/proceedings2020049027
Henrikson E, Wood P, Broadie C, Nuttall T. The Role of Friction and Tangential Compliance on the Resultant Launch Angle of a Golf Ball. Proceedings. 2020; 49(1):27. https://doi.org/10.3390/proceedings2020049027
Chicago/Turabian StyleHenrikson, Erik, Paul Wood, Chris Broadie, and Tom Nuttall. 2020. "The Role of Friction and Tangential Compliance on the Resultant Launch Angle of a Golf Ball" Proceedings 49, no. 1: 27. https://doi.org/10.3390/proceedings2020049027
APA StyleHenrikson, E., Wood, P., Broadie, C., & Nuttall, T. (2020). The Role of Friction and Tangential Compliance on the Resultant Launch Angle of a Golf Ball. Proceedings, 49(1), 27. https://doi.org/10.3390/proceedings2020049027




