Applicability of Thermoplastic Elastomers to Impact Load Reduction in Sports Equipment †
Abstract
:1. Introduction
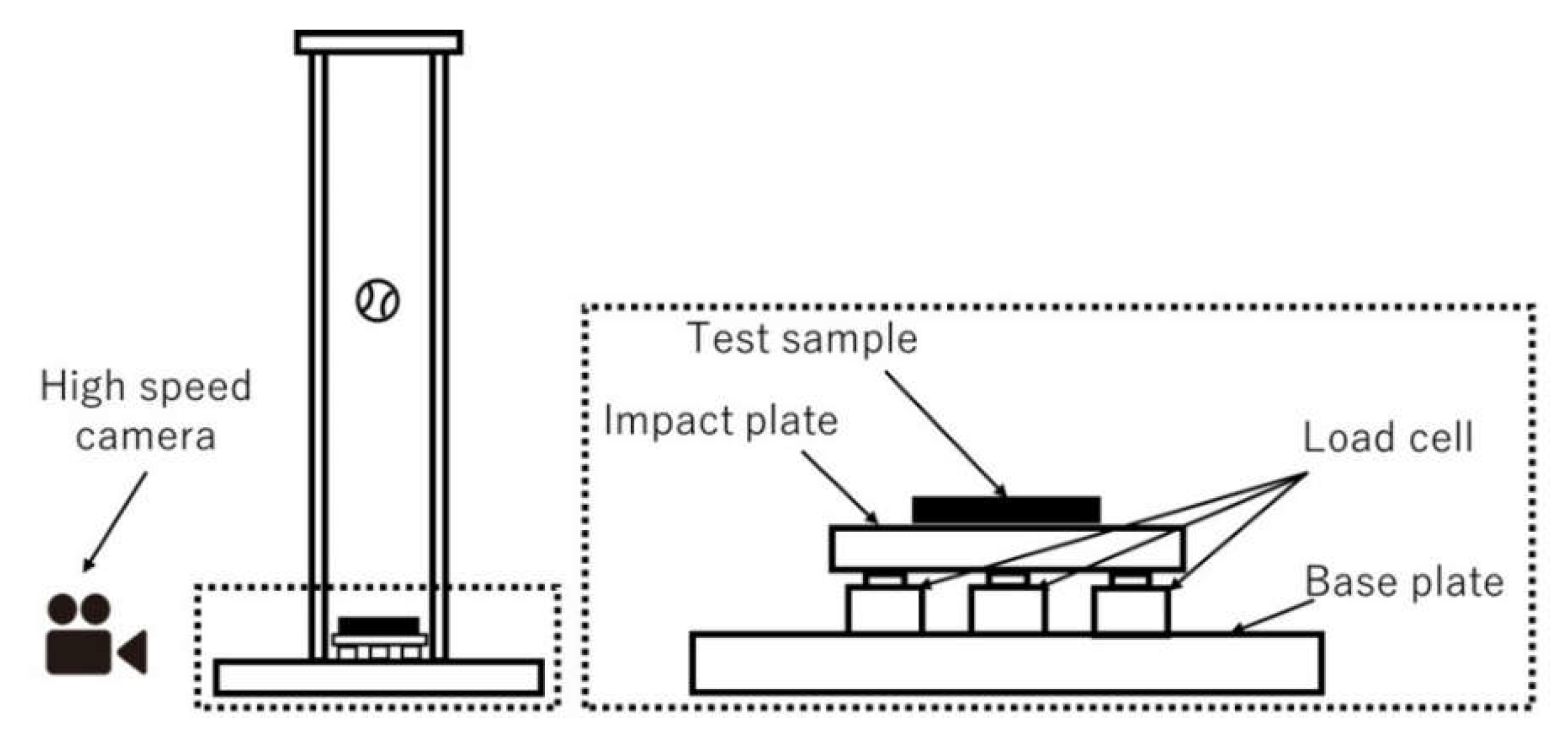
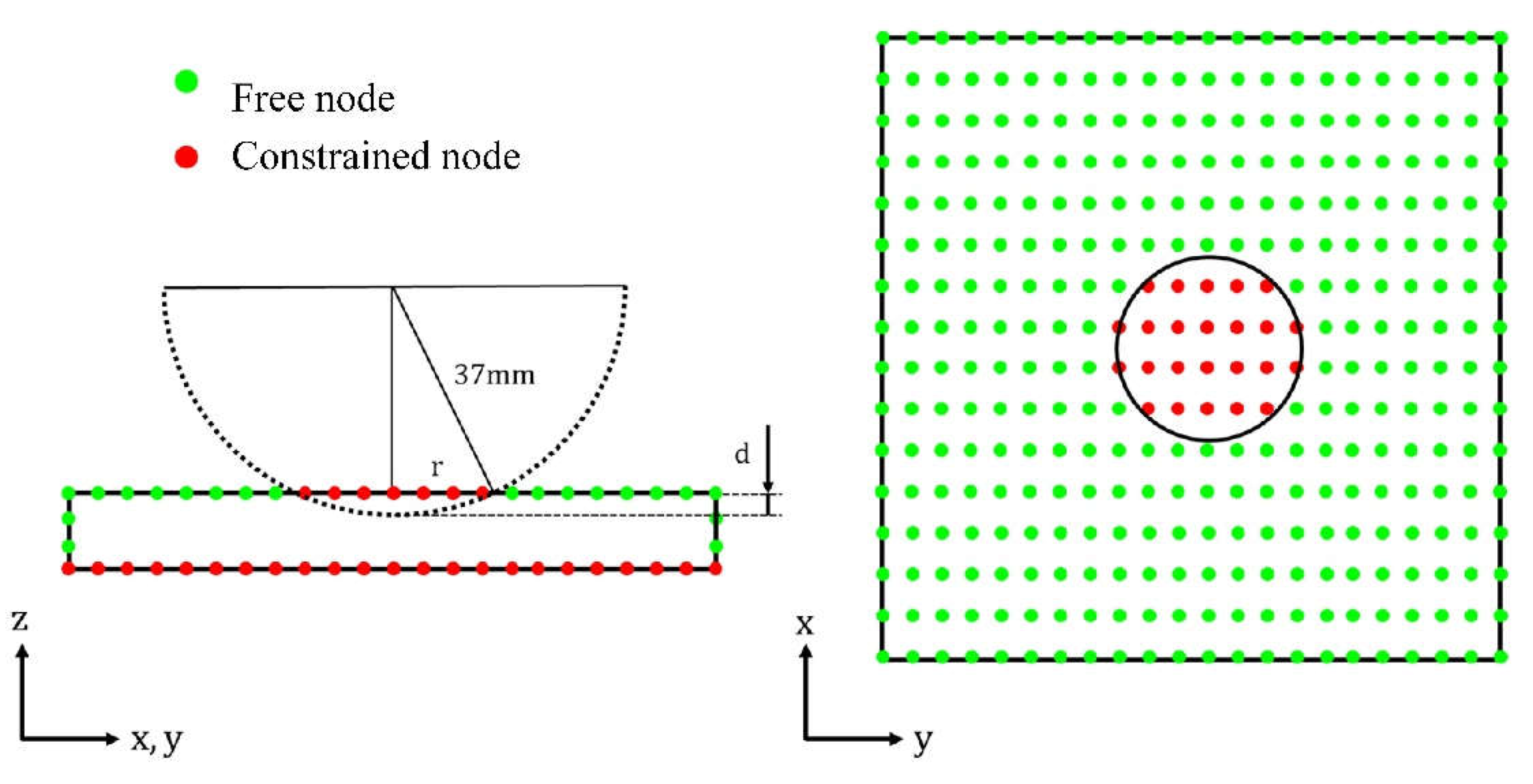
2. Materials and Methods
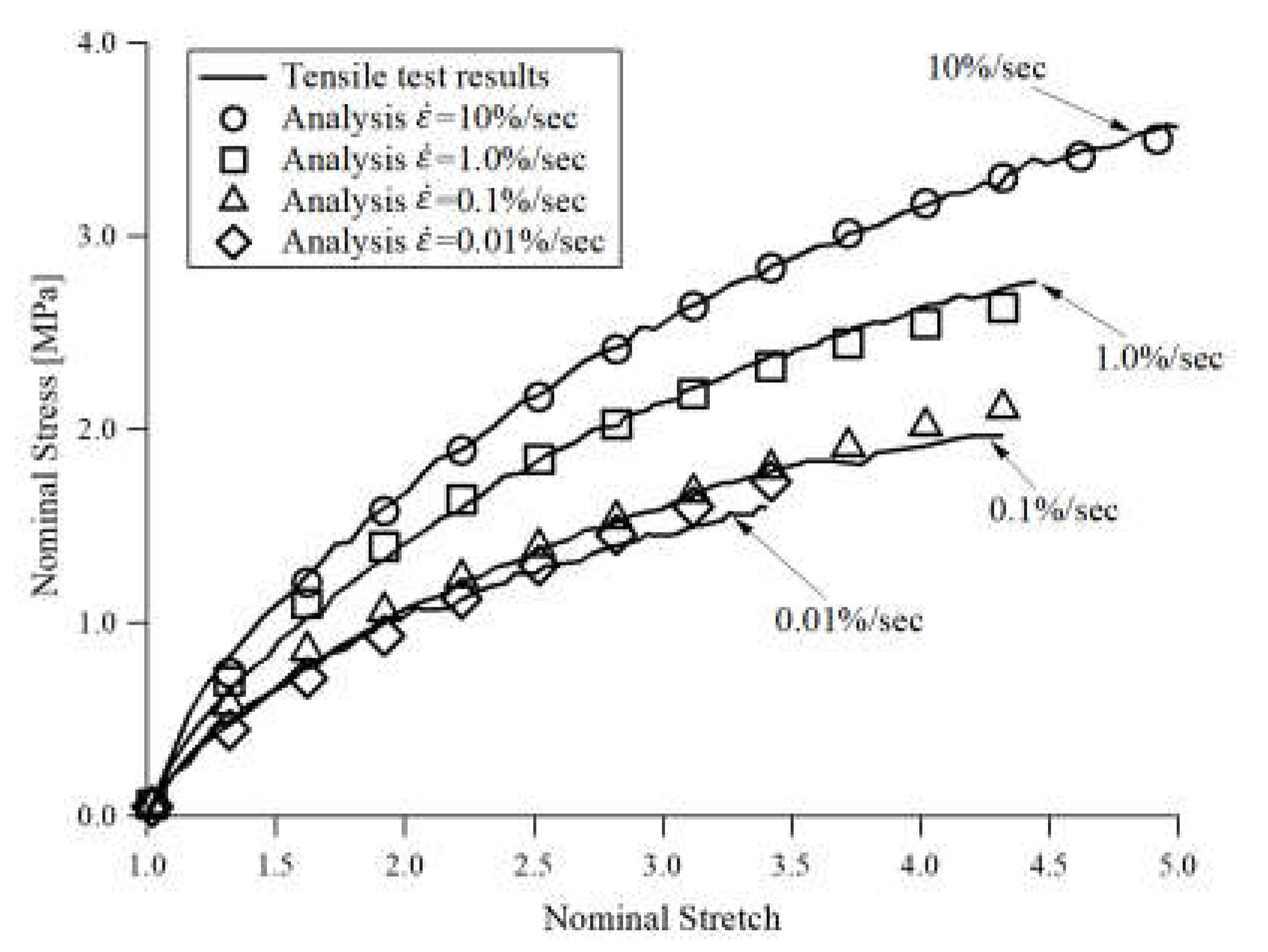
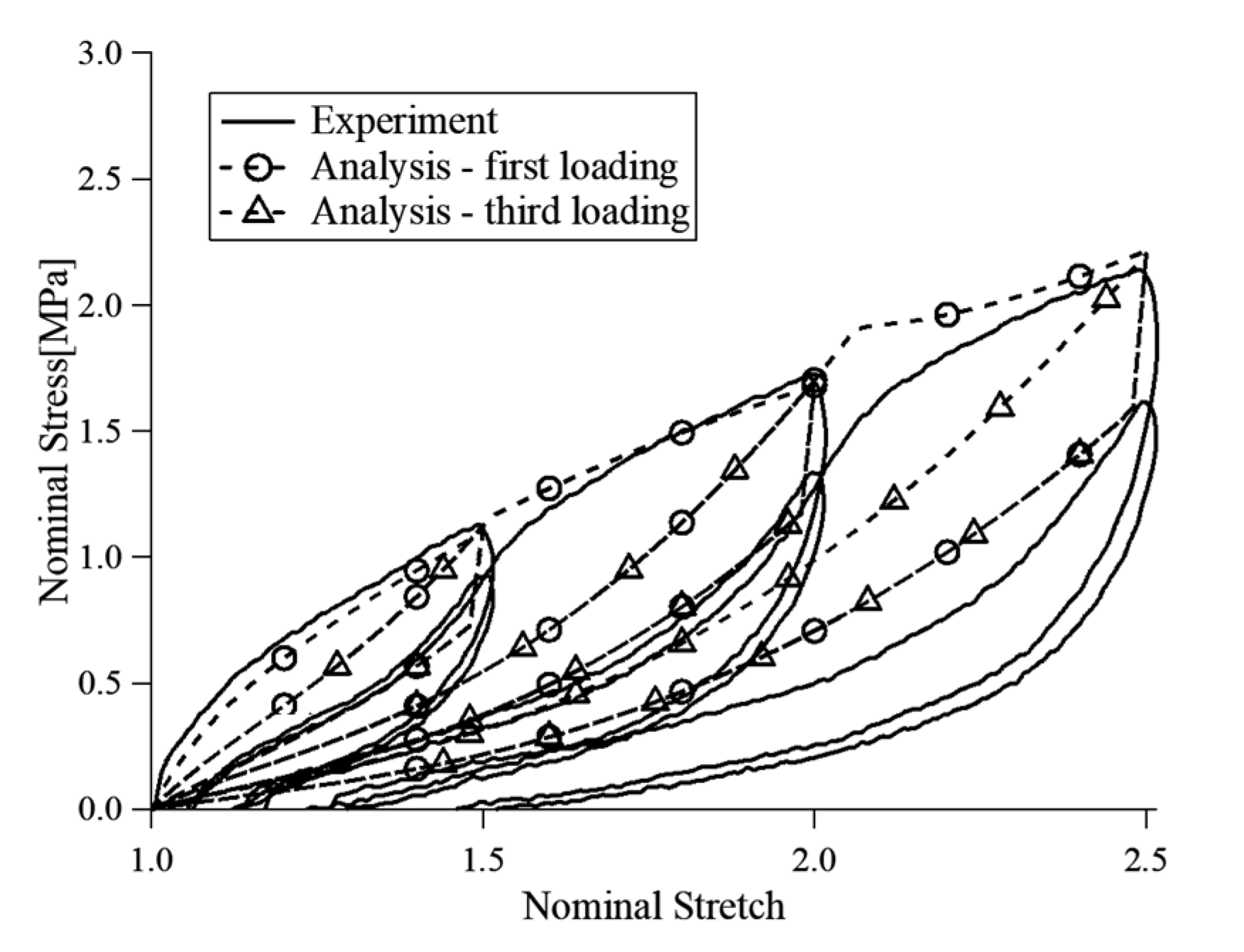
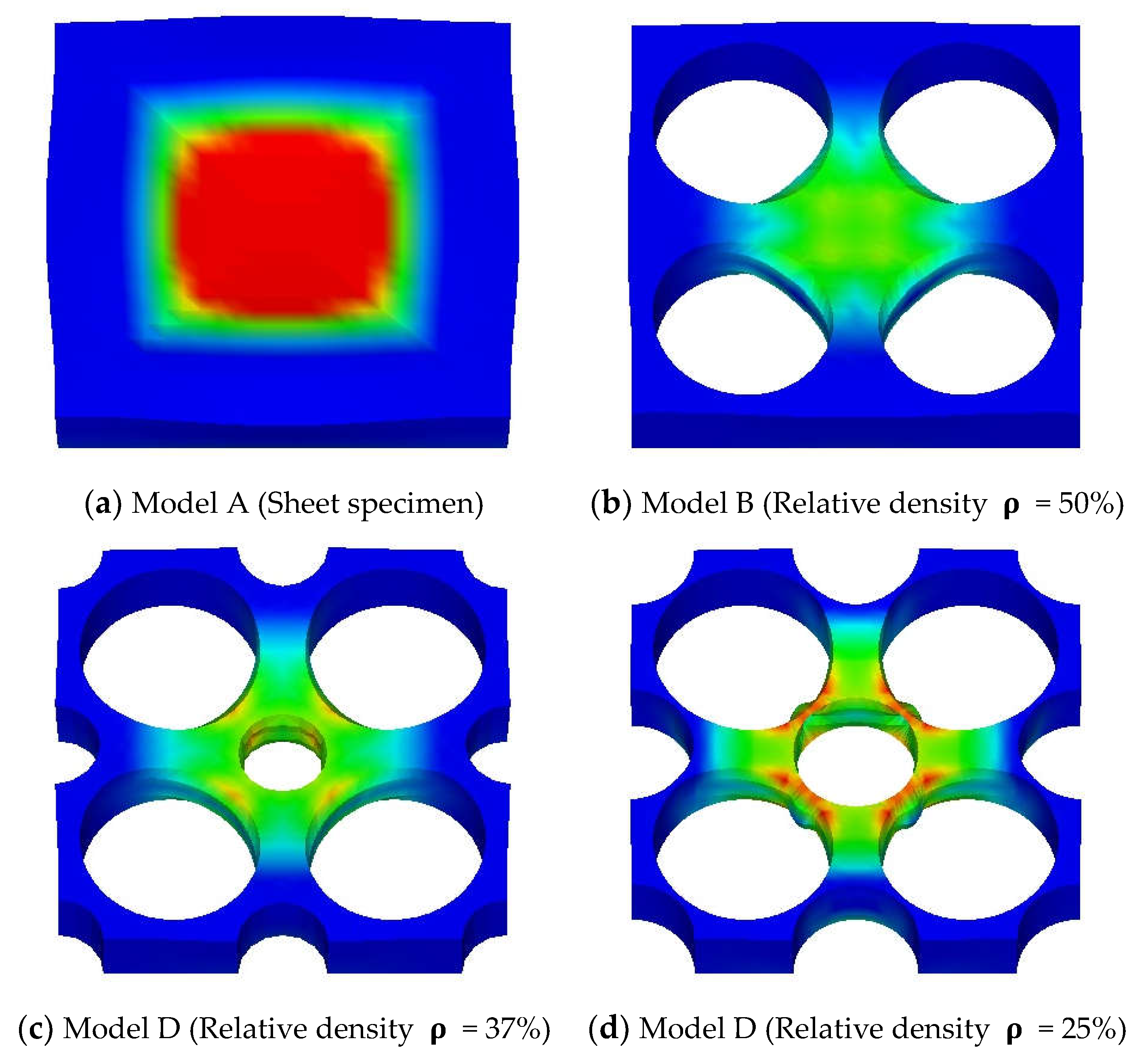
3. Results and Discussion
4. Conclusions
References
- Holden, G. Applied Plastics Engineering Handbook, 2nd ed.; William Andrew: Norwich, NY, USA, 2017; pp. 91–107. [Google Scholar]
- Fraser, M.A.; Grooms, D.R.; Guskewicz, K.M.; Kerr, Z.Y. Ball-Contact Injuries in 11 National Collegiate Athletic Association Sports: The injury Surveillance Program 2009–2010 through 2014–2015. J. Athl. Train. 2017, 52, 698–707. [Google Scholar] [CrossRef] [PubMed]
- Maron, B.J.; Ploiac, L.C.; Kaplan, J.A.; Muller, F.O. Blunt Impact to the Chest Leading to Sudden Death from Cardiac Arrest during Sports Activities. N. Engl. J. Med. 1995, 333, 337–342. [Google Scholar] [CrossRef] [PubMed]
- Simo, J.C.; Huges, T.J.R. Computational Inelasticity; Springer: Berlin/Heidelberg, Germany, 1998; pp. 336–373. [Google Scholar]
- Simo, J.C. On a Fully Three-Dimensional Finite-Strain Viscoelastic Damage Model: Formulation and Computational aspects. Comput. Methods Appl. Mech. Eng. 1987, 60, 153–173. [Google Scholar] [CrossRef]
- Sover, A.; Fomrmanna, L.; Kipscholl, R. High Impact-Testing Machine for Elastomers Investigation under Impact Loads. Polym. Test. 2009, 28, 871–874. [Google Scholar] [CrossRef]
- Velasco, J.I.; Martinez, A.B.; Arencón, D.; Rodórigues-Perezj, A.; De Saja, J.A. Application of Instrumented Falling Dart Impact to the Mechanical Characterization of Thermoplastic Foams. J. Mater. Sci. 1999, 34, 431–438. [Google Scholar] [CrossRef]
- Zhan, W.; Chen, S.; Liu, Y. Effect of Weight and Drop Height of Hammer on the Flexural Impact Performance of Fiber-Reinforced Concrete. Constr. Build. Mater. 2017, 140, 31–35. [Google Scholar] [CrossRef]
- Farooq, U.; Myler, P. Finite Element Simulation of Damage and Failure Predictions of Relatively Thick Carbon Fibre-Reinforced Laminated Composite Panels Subjected to Flat and Round Noses Low Velocity Drop-Weight Impact. Thin Walled Struct. 2016, 104, 82–105. [Google Scholar] [CrossRef]
- Yajima, N.; Kawahara, S.; Matsuda, A. Evaluation of Impact Absorbing Properties of Thermoplastic Elastomers. IOP Conf. Ser. Mater. Sci. Eng. 2019, 548, 012008. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Matsuda, A.; Kawahara, S. Applicability of Thermoplastic Elastomers to Impact Load Reduction in Sports Equipment. Proceedings 2020, 49, 163. https://doi.org/10.3390/proceedings2020049163
Matsuda A, Kawahara S. Applicability of Thermoplastic Elastomers to Impact Load Reduction in Sports Equipment. Proceedings. 2020; 49(1):163. https://doi.org/10.3390/proceedings2020049163
Chicago/Turabian StyleMatsuda, Akihiro, and Shigeru Kawahara. 2020. "Applicability of Thermoplastic Elastomers to Impact Load Reduction in Sports Equipment" Proceedings 49, no. 1: 163. https://doi.org/10.3390/proceedings2020049163
APA StyleMatsuda, A., & Kawahara, S. (2020). Applicability of Thermoplastic Elastomers to Impact Load Reduction in Sports Equipment. Proceedings, 49(1), 163. https://doi.org/10.3390/proceedings2020049163



