Auxetic Films with a Miniaturized Cellular Structure ^†

Abstract

Auxetic materials represent a relatively new class of materials that are characterized by a cellular structure and a negative Poisson coefficient. Auxetics are extremely useful for morphing applications thanks to their synclastic deformation capability. Most of these materials have been developed with macro-scaled cellular units. However there are some applications (e.g., micro-air vehicles or biomedical applications) for which polymeric morphing materials need to be applied in relatively small areas. In these cases, a material scale reduction that leads to lightweight auxetic films with a miniaturized cellular structure could be of great interest. With this in mind, an experimental study was conducted to analyze the response of films that are characterized by a miniaturized cellular structure. The unit cells in this study were made of an aggregation of microwires and micronodes that were strategically interconnected to form auxetic expansions and contractions. The reduction in scale of the cellular units has a significant impact on the material characterization and properties. The response of polymeric micro-scaled cells is in fact here demonstrated to be strongly influenced by surface forces and dramatic changes in gaseous or liquid environment. This represents the most critical aspect and key variation when comparing these films with standard macro-auxetics. The extremely challenging (at this length-scale) fabrication and testing processes were optimized. Single cells, were thus successfully tested in different environments with programmed and digital micro-stages while being monitored under a microscope with a video camera. Digital image correlation techniques were used to highlight the deformation. The expansion/contraction process was found to be fully reversible after several cycles and at different deformation speeds.

1. Introduction

Auxetic materials were introduced for the first time by Evans in 1991. The key property auxetics is the negative Poisson’s ratio for which the material becomes wider when stretched and thinner when compressed [1]. Most auxetic materials are man-made, but a few can also be found in nature. The natural auxetic materials can be found in biological tissues and pyrolytic graphite while man-made auxetics were first used in moderator core of Magnox nuclear reactor [2,3,4,5,6]. The cellular materials have several advantages if compared with solid materials and these include low density, light weight, high acoustic isolation and damping, durability at dynamic loading and fatigue etc. [7].

To date, a variety of auxetic materials and structures have been discovered, fabricated, or synthesized ranging from the macro-scale down to the molecular scale. The auxetic polymeric materials include: polyester urethane (PU), polyimide (PI), polytetrafluoroethylene (PTFE), Ultra-High molecular weight polyethylene (UHMWPE), polypropylene (PP), polyester, nylon and etc, when they are in the form of foams, fibers and composites. Over the past decades, several geometrical structures were proposed, studied and tested.

The auxetic material exploits a negative Poisson ratio which allows a transversal expansion while the material is being stretched. This unique mechanical response is related to special geometries in the material cellular structure. Auxeticity was demonstrated in foams [8], polymers [9] and composite structures [10,11,12,13] mostly by means of macro-scaled cells. In this context, chiral and hexachiral structures are the most widely used because of their excellent response in terms of auxeticity. Several applications could benefit from the use of micro-scaled cellular materials especially when lightweight but outperforming functional “films” need to be used. Auxetic lightweight films could be of great interest for morphing micro-robots, aerial vehicles, filters and smart textiles. The bio-medical field would also take advantage of such materials for all cases where biological cells need to interact with a foreign object, as in implantable devices or non-invasive surgical tools [14]. A recent effort by the authors [15] showed the great potential of a miniaturized auxetic design for smaller scale applications. However in that case the experimental campaign was limited by fabrication and testing challenges.

In this study miniaturized auxetic cell [16] that can be used for small-scale applications are presented. The reduction in scale of the cellular units has a significant impact on the material fabrication, characterization and properties. The response of polymeric micro-scaled cells is in fact strongly influenced by surface forces and changes dramatically in air or in a liquid environment. These issues are investigated in broad detail as reported in the following sections.

Liquid Polyimide (PI 4110, Microsystem) is spin coated on a silicon wafer so as to achieve a film thickness of 40µm. Soft bake processing is performed on a hotplate at 90 °C and 110 °C. The sample is then exposed for 20s and is developed with a spray system. The development procedure is quite complex and critical for optimal feature results. This step is performed in eight sub-steps of spray/puddle with a nominal develop time of 20 seconds per spray/puddle step and for a total time of 160 seconds. The sample is then rinsed and post-baked. The resulting polyimide micro-structures is analyzed after development using an optical microscope and a profilometer. The sample is finally lifted-off in an HF (49%) bath and then is washed in water. The polyimide samples are then cured in an air oven.

The major challenge is found to be related to the wire shape which is easily lost during the above described fabrication process.

2. Results and Discussion

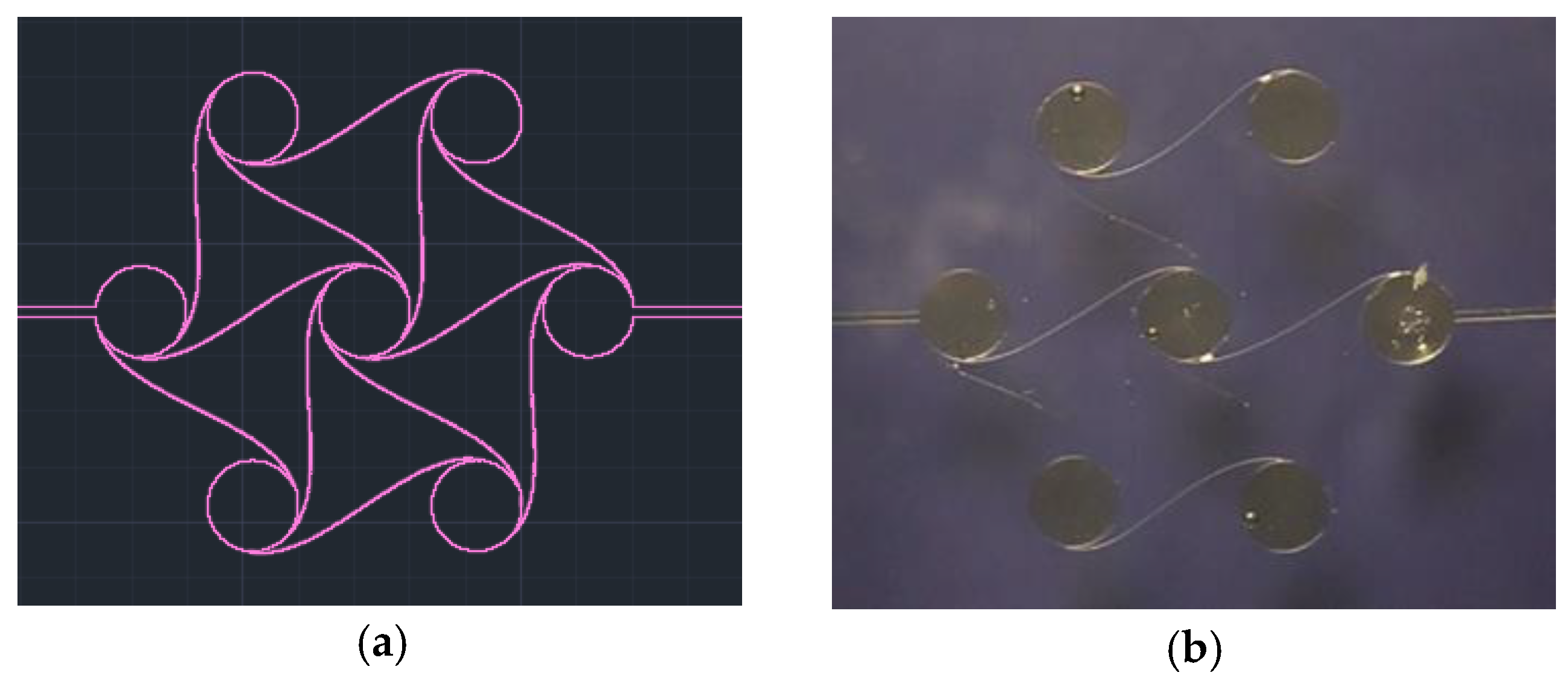
Figure 1 presents an optical microscope image of a fabricated cell and its comparison with the original design. The images clearly show the great accuracy of the developed fabrication procedure.

The fabricated samples are then experimentally tested using an ad-hoc designed set-up. For this purpose samples were tested in air and in water to simulate different application environment (e.g., biological applications for water, and air-vehicles for air). Testing is performed by bonding the two opposite sides of the sample on two movable stages, which are digitally controlled to move simultaneously, but oppositely, along the same direction. The movement of the stages allow the cell stretching. A major challenge when transferring the sample to be tested from the wafer to the stages, is to avoid undesired pre-stretching which could compromise the test data. The deformation of the cell during stretching was monitored via a high resolution video camera coupled with a microscope. and data were analyzed following a digital image correlation technique. Major focus is given to tracing the nodes movement during loading/unloading as well as the wires shape. Figure 2 shows that the cell expands uniformly according to the expected mechanism. The internal wires that interconnect the central and the transversal micronodes, push the transversal nodes when the central node rotates due to the stages pulling.

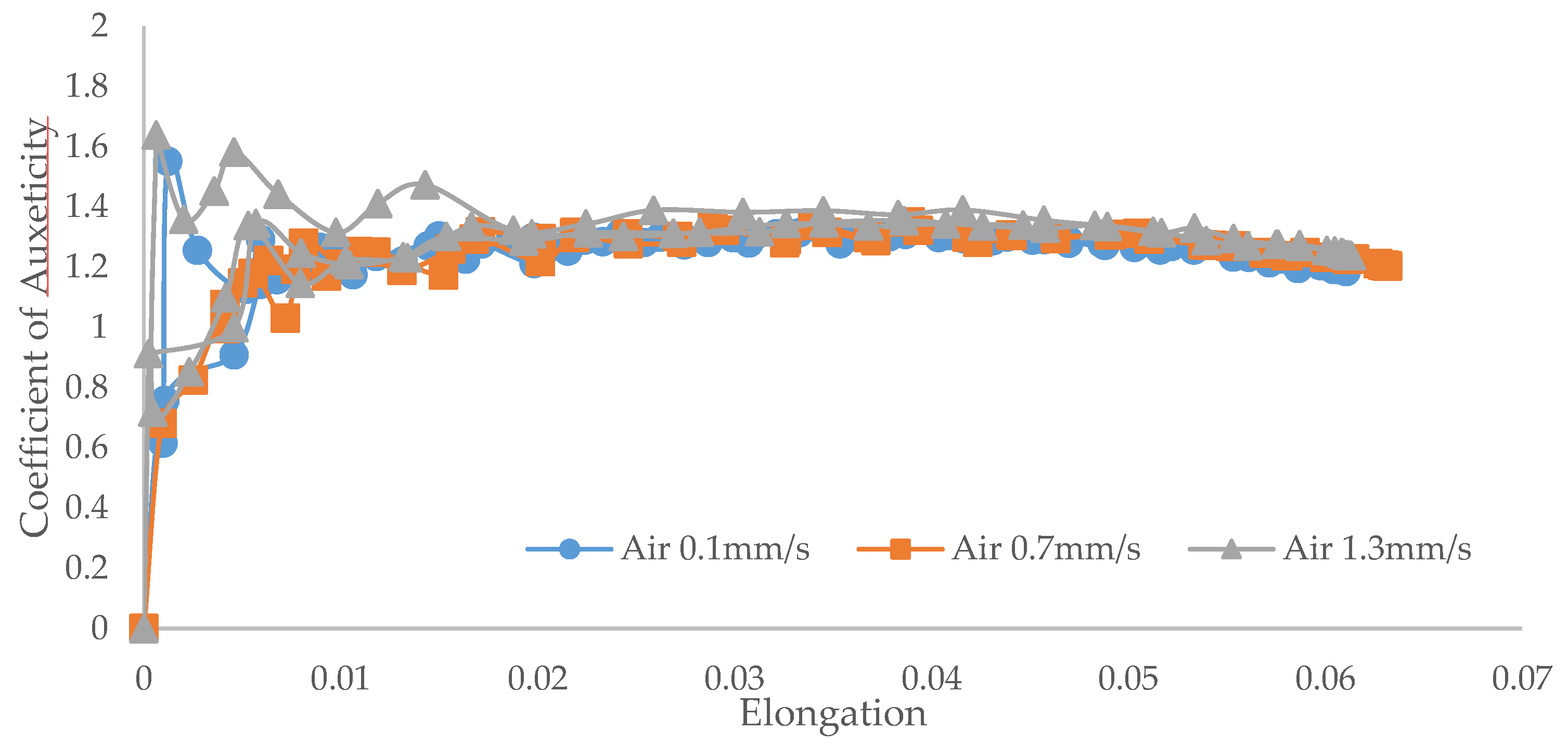
Figure 3 shows the experimental coefficient of auxeticity [16] of single cell, tested in air, with different loading speeds (from 0.1 mm/s to 1.3 mm/s). The coefficient of auxeticity is defined as: anisotropic auxetic response of the cell when loaded in different directions starting from horizontal to the vertical axis.

The experimental trends show a smooth increase in coefficient of auxeticity with increasing elongation until reaching a plateau. The experimental data are very similar to each other in terms of trend and coefficient values which highlights that the cellular design is not truly affected by speed variations and that viscous effect of the forming material are not activated for this case study. However, it is observed that the lowest speed (0.1 mm/s) presents an initial peak in coefficient of auxeticity. It is believed that this is related to the initial acceleration that in this case is the lowest since in the same time-frame the stage moves from a stopped configuration to 0.1 mm/s. A lower acceleration implies a lower force that is applied to the center node. Consequently, the expansion is delayed. Once this force is great enough to rotate the center node winning the inertia force, the mechanism is immediately activated and a peak is recorded.

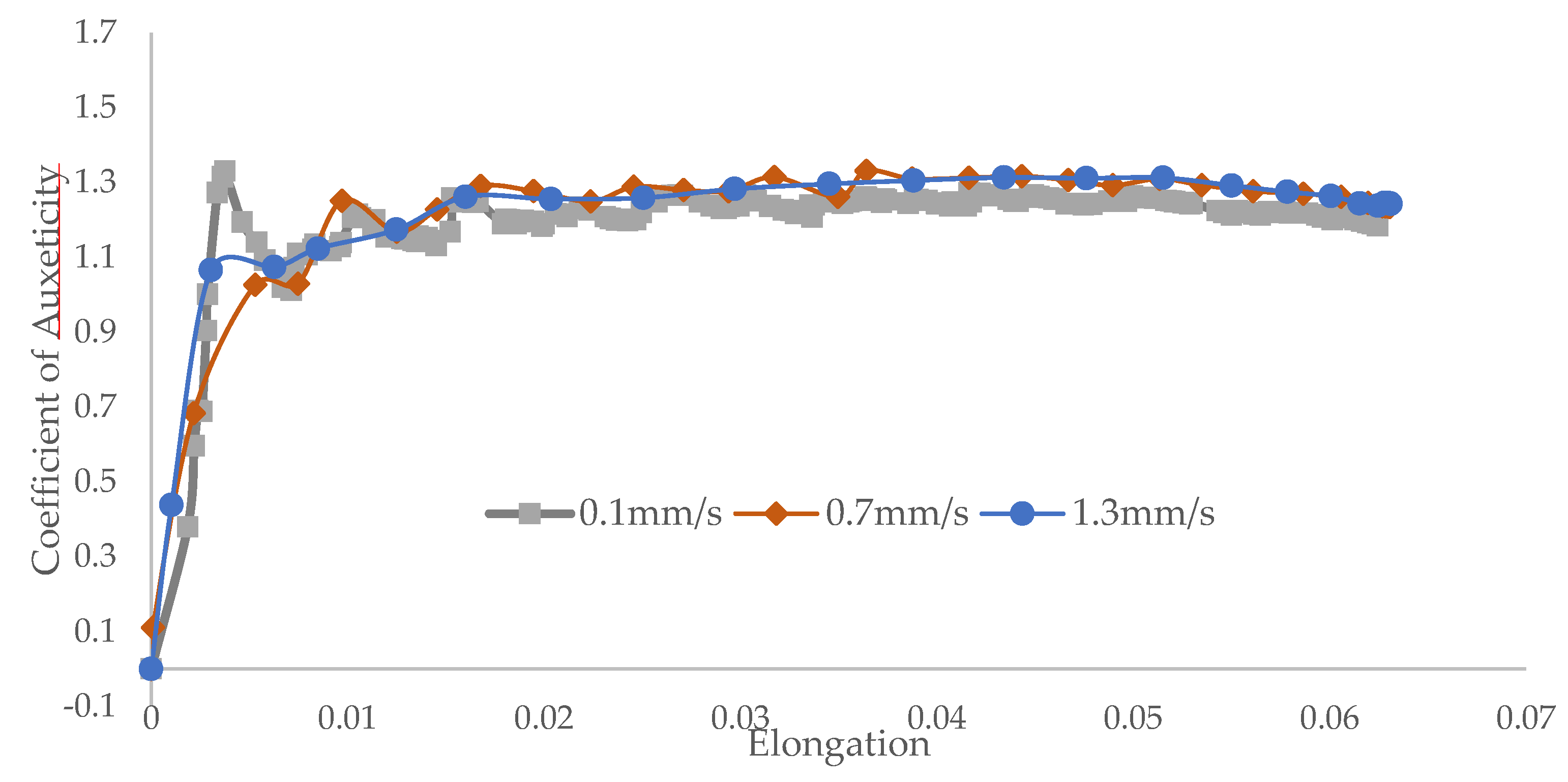
The cyclic response was then investigated to see the behavior of the cell within a full stretching-unstretching cycle. Figure 4 shows the average of 5 cycles for the lowest and highest loading speeds in air. The loading phases show of course the same response discussed above, but during unloading a slight hysteresis is observed which is most probably due to the fact that during stretching the central node rotates anticlockwise while during unstretching the central node has a clockwise rotation. The latter rotation is against the natural rotation of the cell lowering the coefficient of auxeticity.

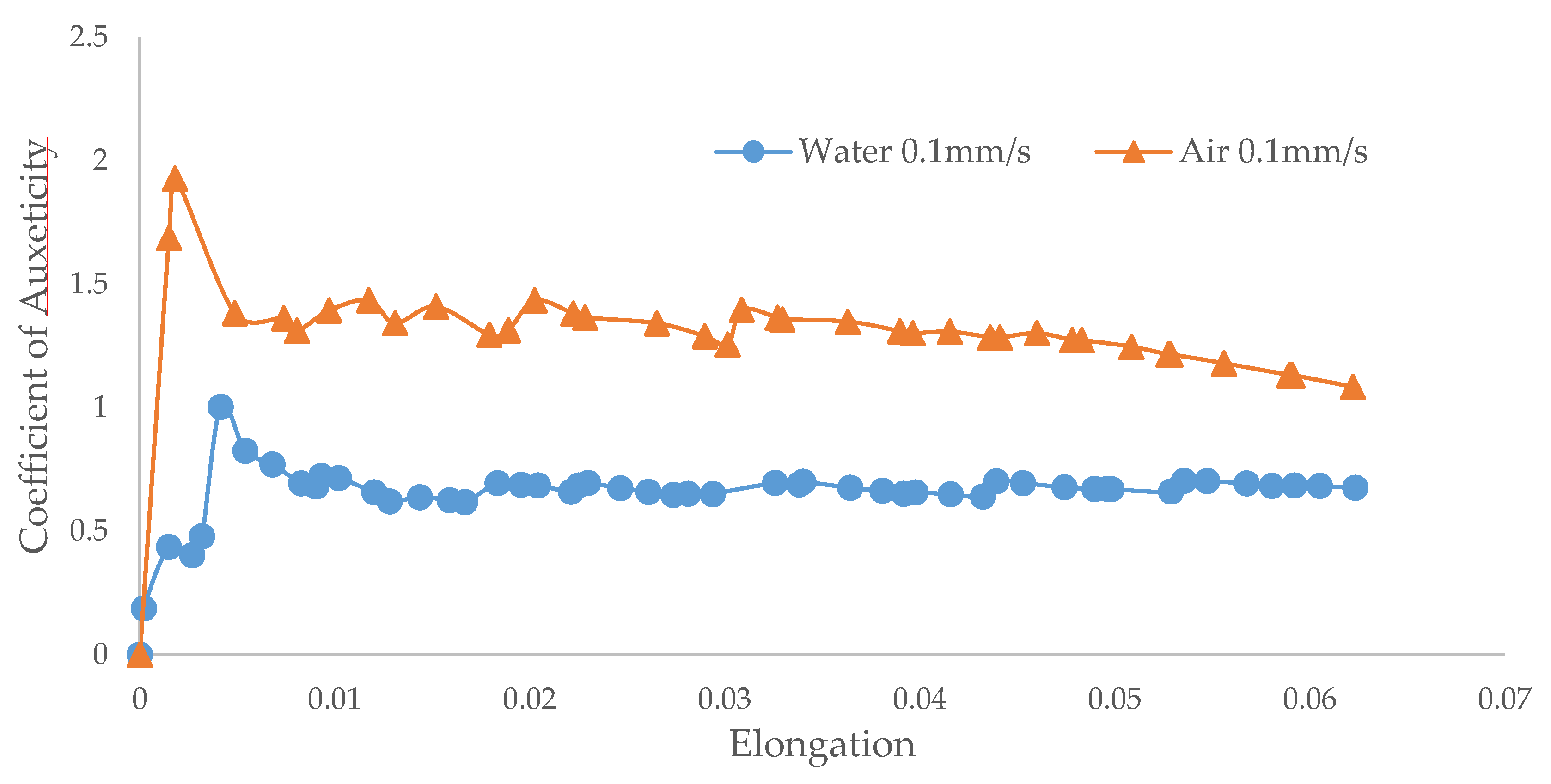
The cell behaviour for fluid-based applications is also performed and the response of the cell under a gaseous or liquid (water) environment is compared. This is because it is expected that water adds additional forces against motion. The major challenge in this case is the transfer of the cell from the wafer to a bath because during transfer the wires might lose their initial designed shape. The results are reported in Figure 5 that clearly shows that the cell has highest coefficient of auxeticity response in air and that the expansion of the cell is smoother in water. The previously discussed initial peak (0.1mm/s case) appears in fact only in air. This is due to a sort of damping effect that the liquid provides to the moving structure.

3. Conclusions

In this study, microstructured auxetic cells were fabricated, tested and characterized. The cells shape takes inspiration from the classical hexachiral structure. A novel fabrication process based on non-standard microfabrication techniques, was designed and optimized. The fabricated samples were experimentally tested under air and water to simulate different application environments. The cells were found to be fully elastic. The loading/unloading cycles show a geometric hysteresis behavior that is affected by the loading speed, and the presence of water.