Influence of Micro-Structure on the Fatigue Crack Propagation in Bridge Steel †
Abstract
:1. Introduction
2. Theoretical Background
CT Specimen
3. Material
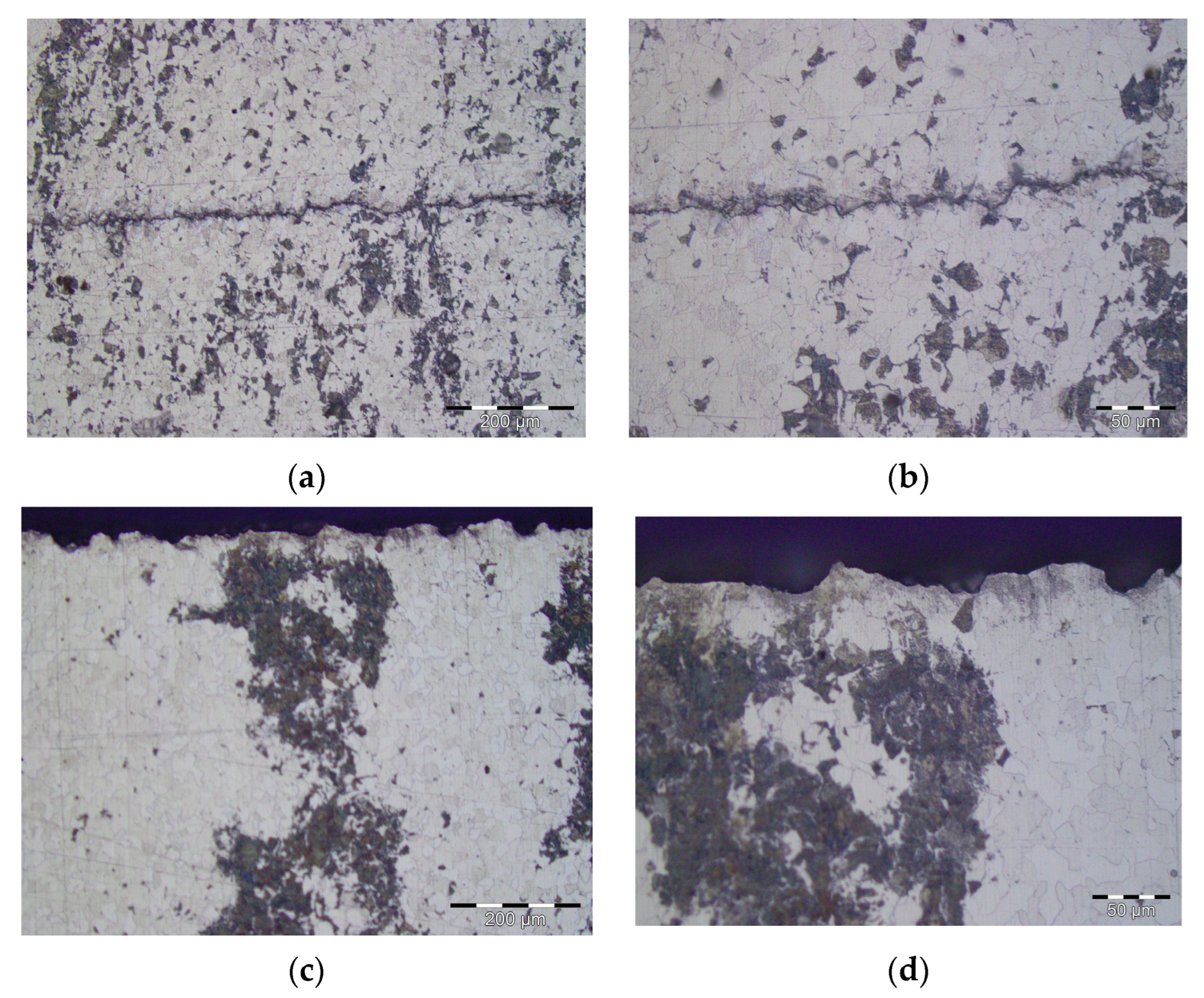
4. Results and Discussion
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- ASTM E647. Standard test method for measurement of fatigue crack growth rates. In Annual book of astm standards. Am. Soc. Test. Mater. 1991, 3, 591–629. [Google Scholar]
- De Jesus, A.M.P.; Matos, R.; Fontoura, B.F.C.; Rebelo, C.; Simões da Silva, L.; Veljkovic, M. A comparison of the fatigue behavior between s355 and s690 steel grades. J. Construct. Steel Res. 2012, 79, 140–150. [Google Scholar] [CrossRef]
- Adedipe, O.; Brennan, F.; Kolios, A. Review of corrosion fatigue in offshore structures: Present status and challenges in the offshore wind sector. Renew. Sustain. Energy Rev. 2016, 61, 141–154. [Google Scholar] [CrossRef]
- Seitl, S.; Miarka, P.; Klusák, J.; Fintová, S.; Kunz, L. Comparison of the fatigue crack propagation rates in S355 J0 and S355 J2 steel grades. Key Eng. Mater. 2018, after revision in press. [Google Scholar] [CrossRef]
- Paris, P.; Erdogan, F. A critical analysis of crack propagation laws. J. Basic Eng. 1963, 85, 528–533. [Google Scholar] [CrossRef]
- EN 10025—Part 2: Technical Delivery Conditions for Non-Alloy Structural Steels. In Hot Rolled Products of Structural Steels; Europian Comittee for Standartization: Geneva, Switzerland, 2004; p. 33.
- Branco, R.; Antunes, F.V.; Ferreira, J.M.; Silva, J.M. Determination of Paris law constatnts with a reverse engineering technique. Eng. Fail. Anal. 2008, 16, 631–638. [Google Scholar] [CrossRef]
- Fernández Canteli, A.; Przybilla, C.; Nogal, M.; López Aenlle, M.; Castillo, E. ProFatigue: A software program for probabilistic assessment of experimental fatigue data sets. Procedia Eng. 2014, 74, 236–241. [Google Scholar] [CrossRef]
- Castillo, E.; Fernández-Canteli, A. A Unified Statistical Methodology for Modeling Fatigue Damage; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar]
- Castillo, E.; Canteli, A.; Siegele, D. Obtaining S-N curves from crack growth curves: An alternative to self-similarity. Int. J. Fract. 2014, 187, 159–172. [Google Scholar] [CrossRef]
- Krejsa, M.; Kala, Z.; Seitl, S. Inspection based probabilistic modeling of fatigue crack progression. Procedia Eng. 2016, 142, 146–153. [Google Scholar] [CrossRef]
- Kala, Z. Identification of stochastic interactions in nonlinear models of structural mechanics. AIP Conf. Proc. 2017, 1863, 480004. [Google Scholar]
- Kala, Z.; Omishore, A. Fuzzy stochastic approaches for analysing the serviceability limit state of load bearing systems. Int. J. Math. Comput. Simul. 2016, 10, 294–301. [Google Scholar]
- Krejsa, M.; Koubová, L.; Flodr, J.; Protivinsky, J.; Nguyen, Q.T. Probabilistic prediction of fatigue damage based on linear fracture mechanics. Frattura ed Integrita Strutturale 2017, 11, 143–159. [Google Scholar] [CrossRef]
- Krejsa, M.; Janas, P.; Krejsa, V.; Kala, Z.; Seitl, S. DOProC-based reliability assessment of steel structures exposed to fatigue. Perspect. Sci. 2016, 7, 228–235. [Google Scholar] [CrossRef]
- Krejsa, M.; Seitl, S.; Brozovsky, J.; Lehner, P. Fatigue damage prediction of short edge crack under various load: Direct Optimized Probabilistic Calculation. Procedia Struct. Integr. 2017, 5, 1283–1290. [Google Scholar] [CrossRef]



| Steel Grade | C | Mn | Si | P | S | N | Cu | CEV |
|---|---|---|---|---|---|---|---|---|
| S355 J0 | 0.2 | 1.6 | 0.55 | 0.035 | 0.035 | 0.012 | 0.55 | 0.47 |
| Material | C [mm/(cycle⋅MPa⋅m0.5)] | m [-] |
|---|---|---|
| de Jesus | 6.0 × 10−10 | 3.561 |
| S355_J0_FM | 1.0 × 10−8 | 2.697 |
| S355_J0_RM | 6.0 × 10−10 | 3.553 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Seitl, S.; Miarka, P.; Pokorný, P.; Fintová, S.; Klusák, J. Influence of Micro-Structure on the Fatigue Crack Propagation in Bridge Steel. Proceedings 2018, 2, 470. https://doi.org/10.3390/ICEM18-05373
Seitl S, Miarka P, Pokorný P, Fintová S, Klusák J. Influence of Micro-Structure on the Fatigue Crack Propagation in Bridge Steel. Proceedings. 2018; 2(8):470. https://doi.org/10.3390/ICEM18-05373
Chicago/Turabian StyleSeitl, Stanislav, Petr Miarka, Pavel Pokorný, Stanislava Fintová, and Jan Klusák. 2018. "Influence of Micro-Structure on the Fatigue Crack Propagation in Bridge Steel" Proceedings 2, no. 8: 470. https://doi.org/10.3390/ICEM18-05373
APA StyleSeitl, S., Miarka, P., Pokorný, P., Fintová, S., & Klusák, J. (2018). Influence of Micro-Structure on the Fatigue Crack Propagation in Bridge Steel. Proceedings, 2(8), 470. https://doi.org/10.3390/ICEM18-05373





