Experimental Investigation on Crack Growth in Pre-Notched Concrete Beams †
Abstract
:1. Introduction
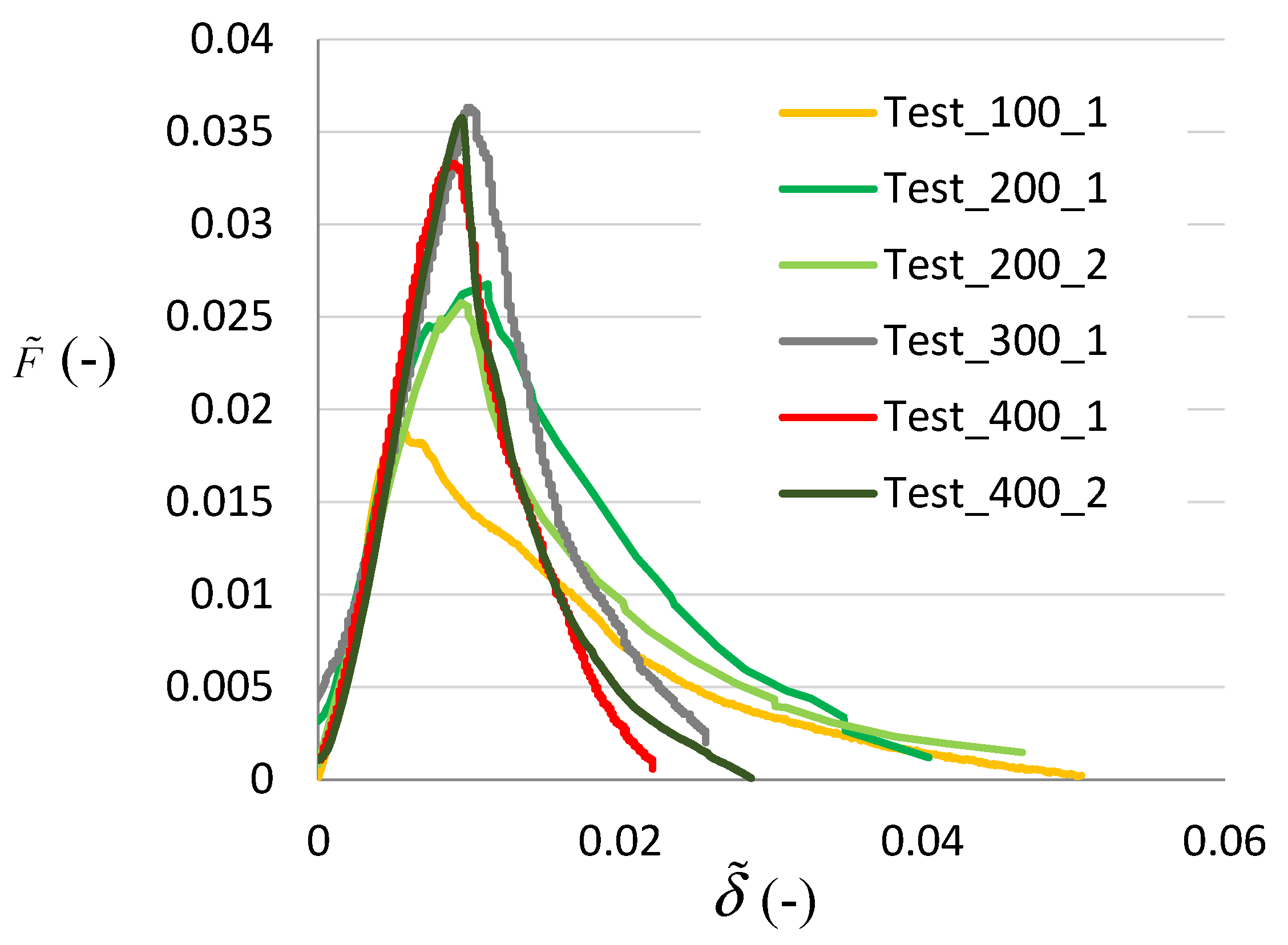
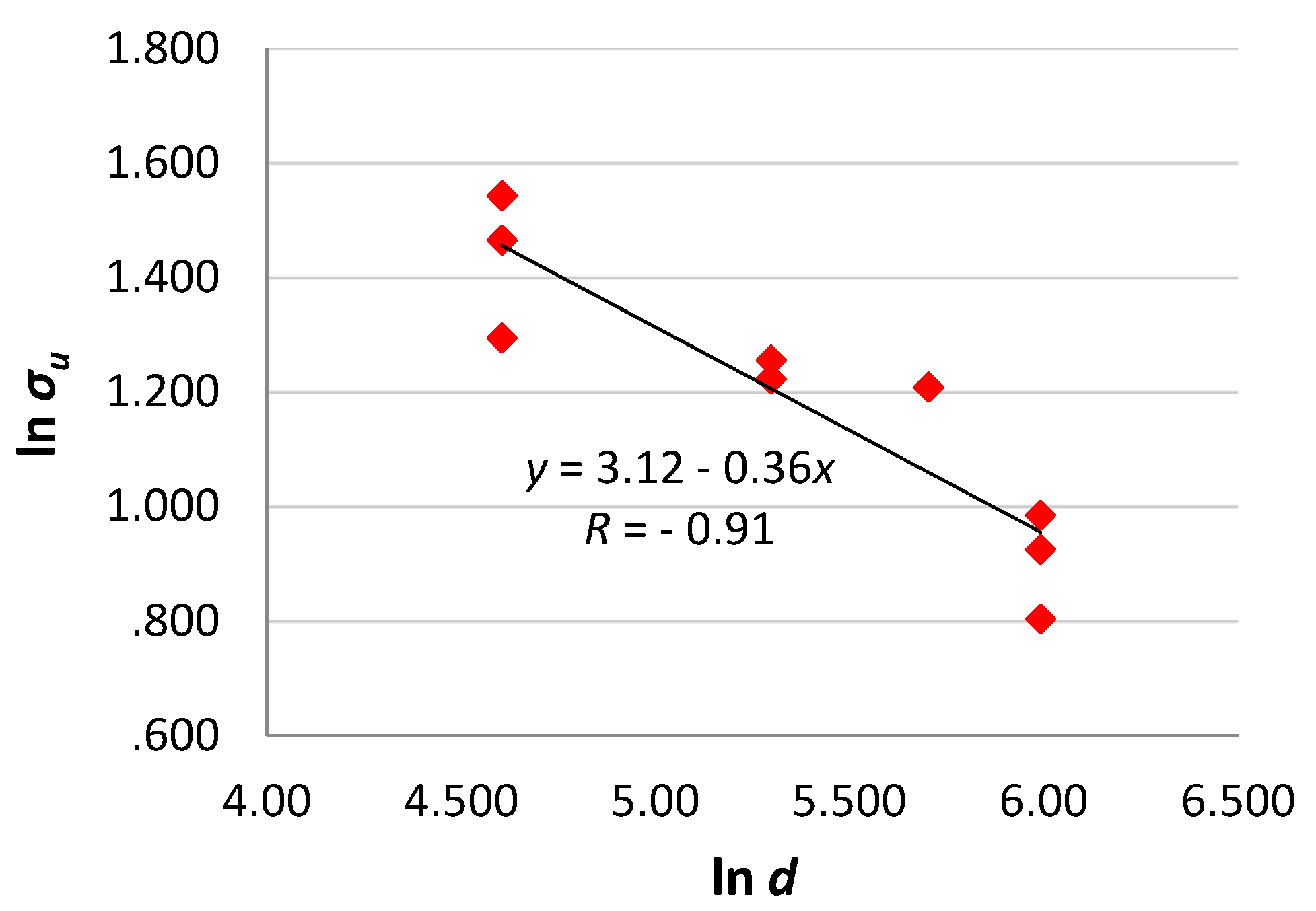
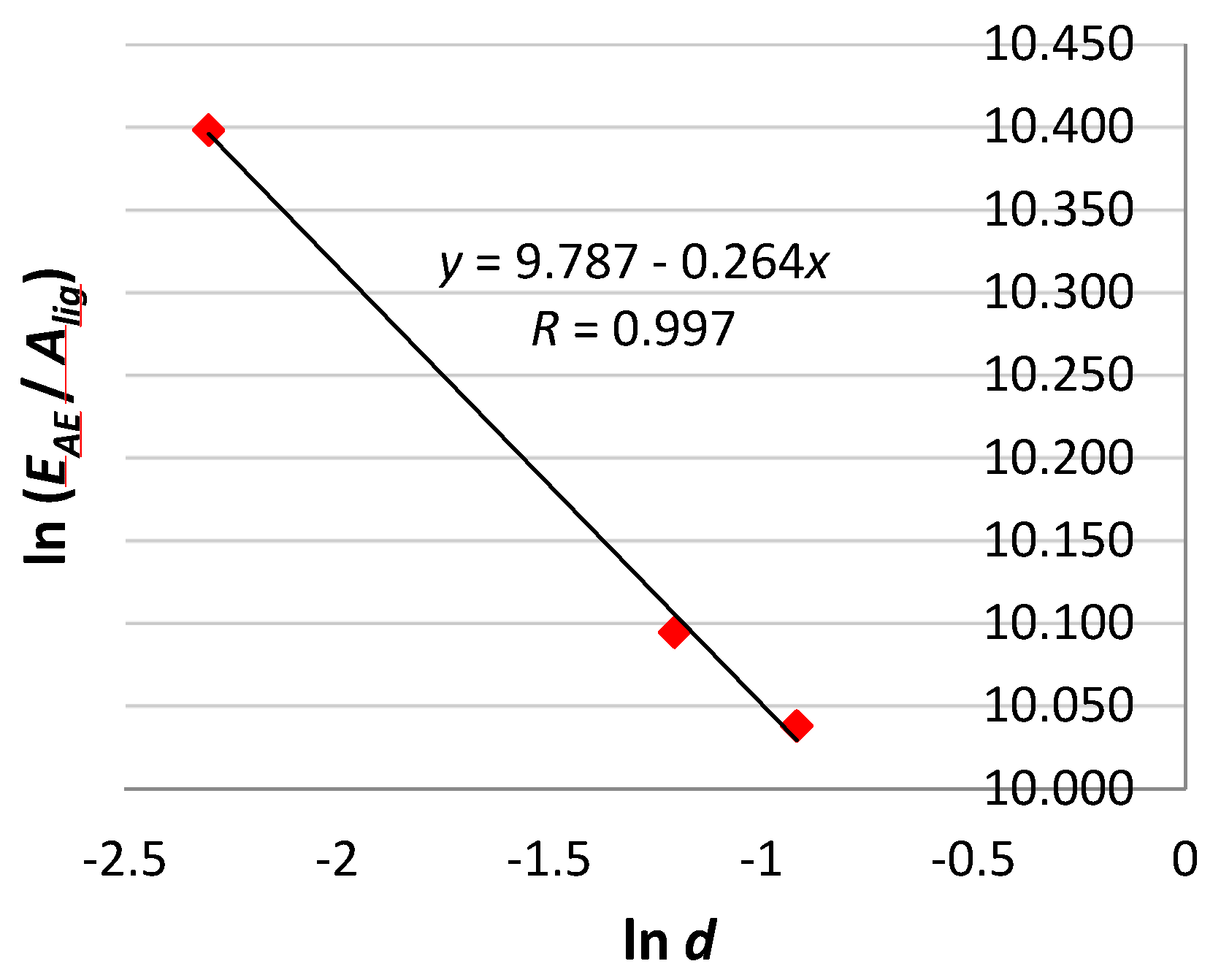
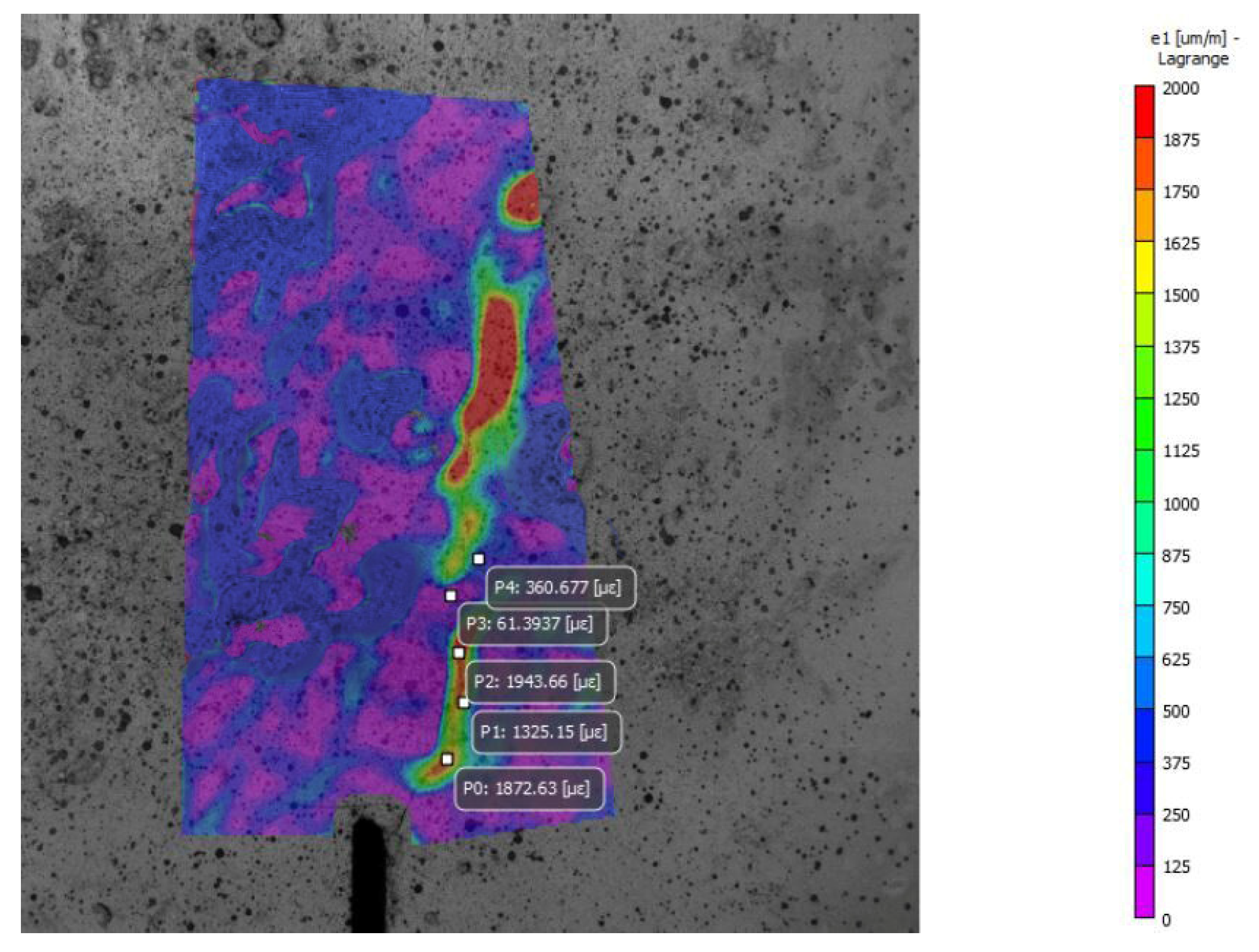
2. Results
3. Conclusions
Author Contributions
Conflicts of Interest
References
- Carpinteri, A. Mechanical Damage and Crack Growth in Concrete. Plastic Collapse to Brittle Fracture; Martinus Nijhoff Publishers: Dordrecht, The Netherlands, 1986. [Google Scholar]
- RILEM TC50-FMC Draft Recommendation. Determination of fracture energy of mortar and concrete by means of three-point bend tests on notched beams. Mater. Struct. 1985, 18, 285–290. [Google Scholar]
- RILEM TC QFS Recommendation. Quasibrittle fracture scaling and size effect—Final report. Mater. Struct. 2004, 37, 547–568. [Google Scholar]
- Lacidogna, G.; Manuello, A.; Niccolini, G.; Accornero, F.; Carpinteri, A. Acoustic emission wireless monitoring of structures. In Acoustic Emission and Related Non-destructive Evaluation Techniques in the Fracture Mechanics of Concrete; Ohtsu, M., Ed.; Chapter 2; Woodhead Publishing: Cambridge, UK, 2015; pp. 15–40. [Google Scholar]
- Lacidogna, G.; Accornero, F.; Carpinteri, A. Masonry structures. In Innovative AE and NDT Techniques for On-site Measurement of Concrete and Masonry Structures (RILEM); Ohtsu, M., Ed.; Chapter 3; Springer: Heidelberg, Germany, 2016; pp. 27–46. [Google Scholar]
- Carpinteri, A.; Lacidogna, G.; Invernizzi, S.; Accornero, F. The Sacred Mountain of Varallo in Italy: Seismic risk assessment by Acoustic Emission and structural numerical models. Sci. World J. 2013, 2013, 170291. [Google Scholar] [CrossRef] [PubMed]
- Carpinteri, A.; Accornero, F. Multiple snap-back instabilities in progressive microcracking coalescence. Eng. Fract. Mech. 2018, 187, 272–281. [Google Scholar] [CrossRef]
- Lacidogna, G.; Accornero, F.; Corrado, M.; Carpinteri, A. Crushing and fracture energies in concrete specimens monitored by Acoustic Emission. In Proceedings of the 8th International Conference on Fracture Mechanics of Concrete and Concrete Structures, FraMCoS 2013, Toledo, Spain, 11–14 March 2013; pp. 1726–1736. [Google Scholar]
- Carpinteri, A.; Lacidogna, G.; Corrado, M.; di Battista, E. Cracking and crackling in concrete-like materials: A dynamic energy balance. Eng. Fract. Mech. 2016, 155, 130–144. [Google Scholar] [CrossRef]
- Sutton, M.A.; Orteu, J.J.; Schreie, H. Image Correlation for Shape, Motion and Deformation Measurements. Basic Concepts, Theory and Application; Springer: New York, NY, USA, 2009. [Google Scholar]
- Aggelis, D.G.; Verbruggen, S.; Tsangouri, E.; Tysmans, T.; van Hemelrijck, D. Characterization of mechanical performance of concrete beams with external reinforcement by acoustic emission and digital image correlation. Constr. Build. Mater. 2013, 47, 1037–1045. [Google Scholar] [CrossRef]
- Carpinteri, A. Decrease of apparent tensile and bending strength with specimen size: Two different explanations based on fracture mechanics. Int. J. Solids Struct. 1989, 25, 407–429. [Google Scholar] [CrossRef]
- Carpinteri, A. Fractal nature of material microstructure and size effects on apparent mechanical properties. Mech. Mater. 1994, 18, 89–101. [Google Scholar] [CrossRef]
- Carpinteri, A. Scaling laws and renormalization groups for strength and toughness of disordered materials. Int. J. Solids Struct. 1994, 31, 291–302. [Google Scholar] [CrossRef]




Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lacidogna, G.; Piana, G.; Accornero, F.; Carpinteri, A. Experimental Investigation on Crack Growth in Pre-Notched Concrete Beams. Proceedings 2018, 2, 429. https://doi.org/10.3390/ICEM18-05287
Lacidogna G, Piana G, Accornero F, Carpinteri A. Experimental Investigation on Crack Growth in Pre-Notched Concrete Beams. Proceedings. 2018; 2(8):429. https://doi.org/10.3390/ICEM18-05287
Chicago/Turabian StyleLacidogna, Giuseppe, Gianfranco Piana, Federico Accornero, and Alberto Carpinteri. 2018. "Experimental Investigation on Crack Growth in Pre-Notched Concrete Beams" Proceedings 2, no. 8: 429. https://doi.org/10.3390/ICEM18-05287
APA StyleLacidogna, G., Piana, G., Accornero, F., & Carpinteri, A. (2018). Experimental Investigation on Crack Growth in Pre-Notched Concrete Beams. Proceedings, 2(8), 429. https://doi.org/10.3390/ICEM18-05287







