A Novel Geometry for Shear Test Using Axial Tensile Setup †
Abstract
1. Introduction
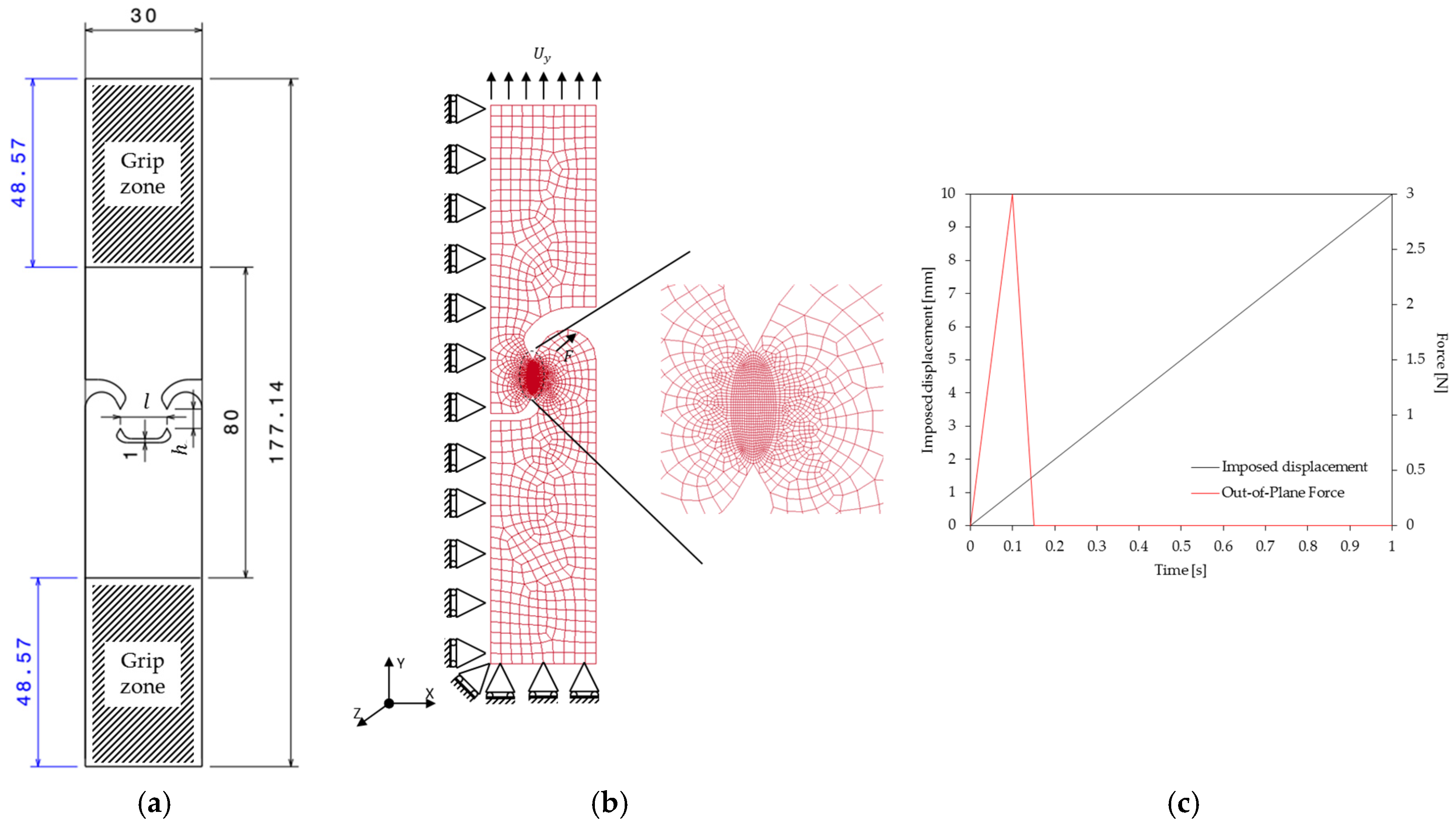
2. Numerical Modeling
3. Numerical Results
3.1. Influence of and Parameters
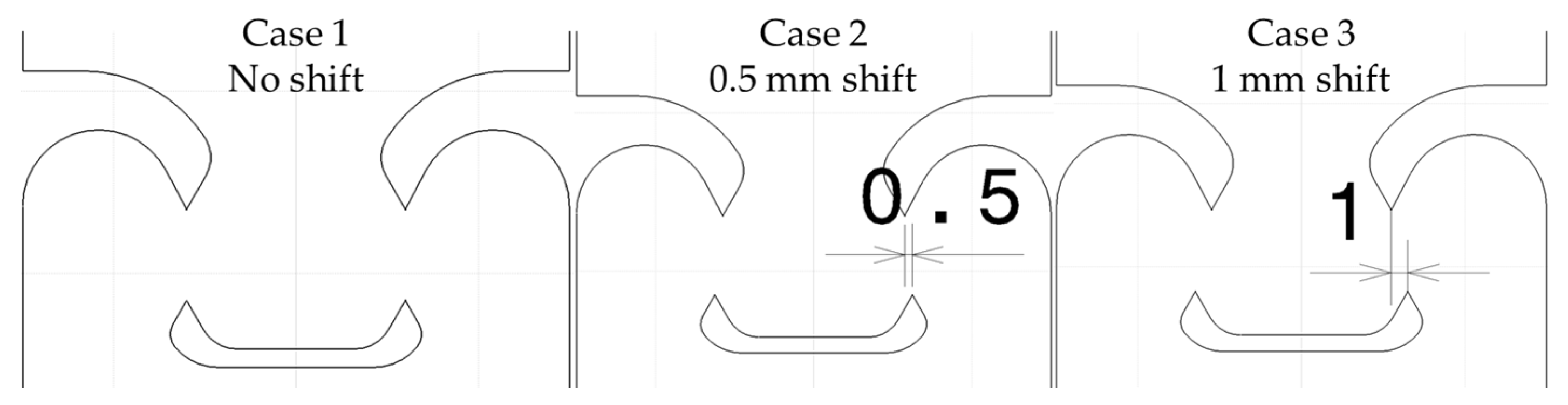
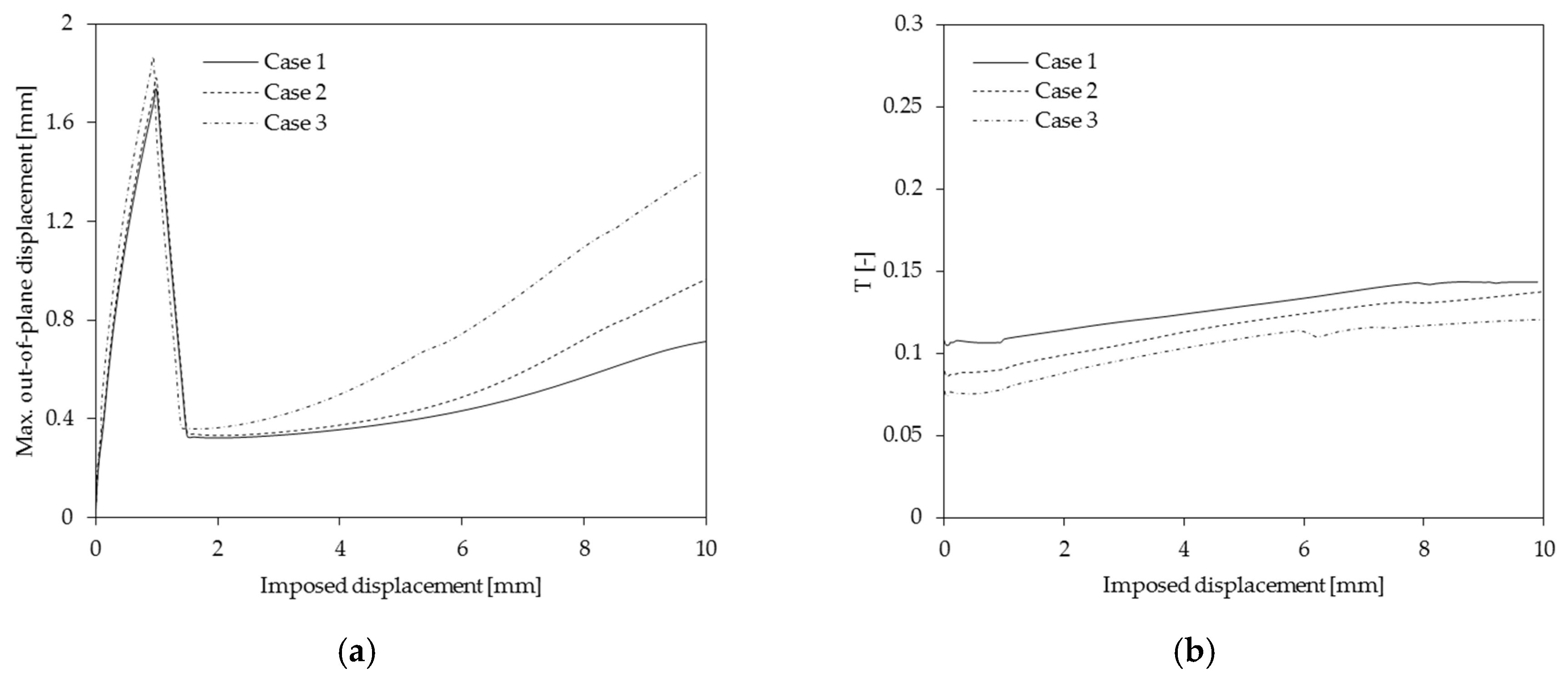
3.2. Influence of Shift of Shear Zone
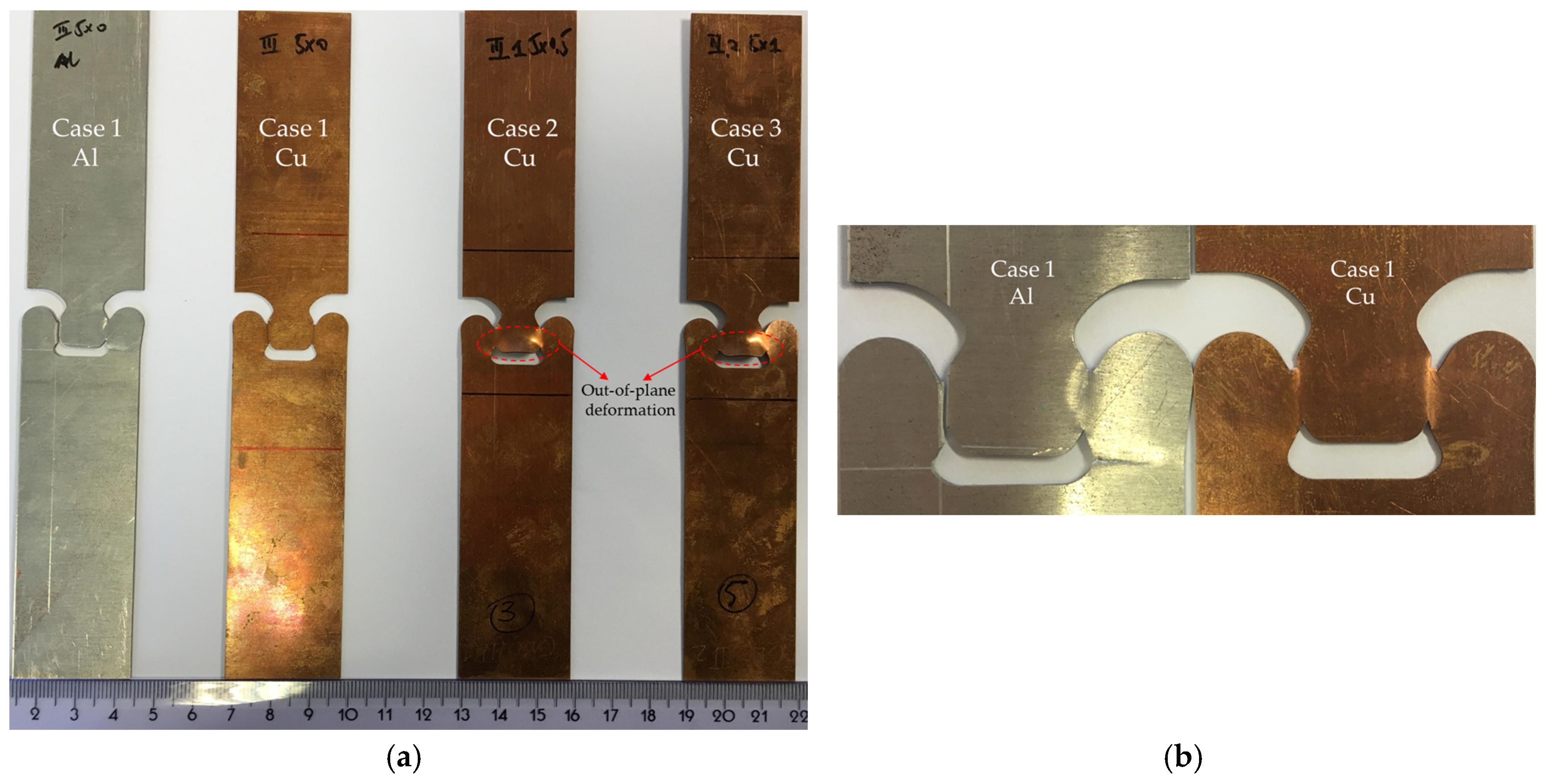
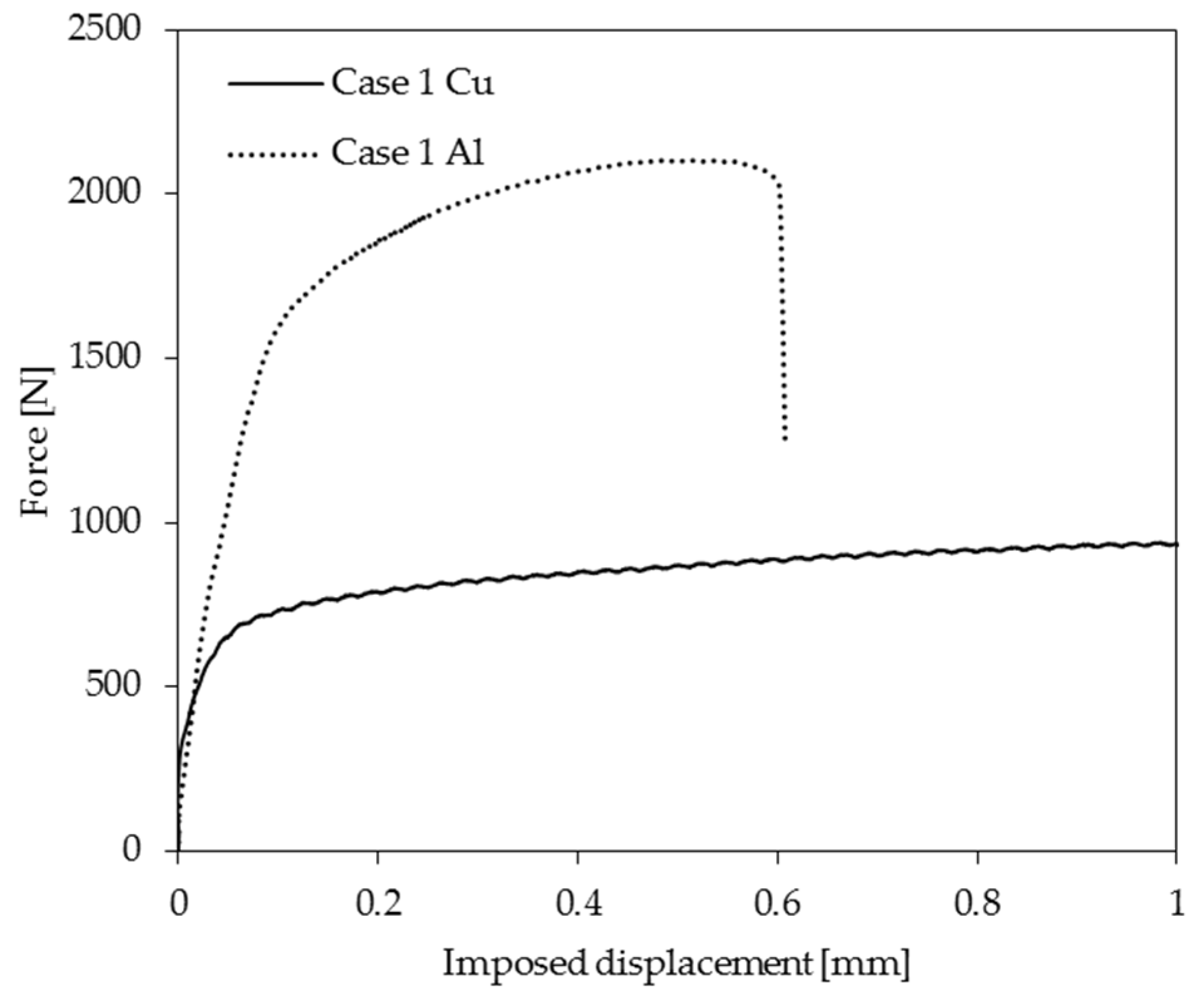
4. Experimental Results
5. Conclusions
Acknowledgments
Conflicts of Interest
References
- Hug, E.; Dubos, P.A.; Keller, C. Temperature dependence and size effects on strain hardening mechanisms in copper polycrystals. Mater. Sci. Eng. A 2013, 574, 253–261. [Google Scholar] [CrossRef]
- Keller, C.; Hug, E.; Chateigner, D. On the origin of the stress decrease for nickel polycrystals with few grains across the thickness. Mater. Sci. Eng. A 2009, 500, 207–215. [Google Scholar] [CrossRef]
- Keller, C.; Hug, E.; Retoux, R.; Feaugas, X. TEM study of dislocation patterns in near-surface and core regions of deformed nickel polycrystals with few grains across the cross section. Mech. Mater. 2010, 42, 44–54. [Google Scholar] [CrossRef]
- Dubos, P.A. Influence de la Température et du Trajet de Chargement sur les Transitions Volume-Surface des Métaux Cubiques à Faces Centrées. Ph.D. Thesis, Université de Caen/Basse-Normandie, Caen, France, 2013. [Google Scholar]
- Li, H.; Fu, M.W.; Lu, J.; Yang, H. Ductile fracture: Experiments and computations. Int. J. Plast. 2011, 27, 147–180. [Google Scholar] [CrossRef]
- Peirs, J.; Verleysen, P.; Degrieck, J. Experimental study of the influence of strain rate on fracture of Ti6Al4V. Procedia Eng. 2011, 10, 2336–2341. [Google Scholar] [CrossRef][Green Version]
- Peirs, J.; Verleysen, P.; Paepegem, W.V.; Degrieck, J. Novel pure-shear sheet specimen geometry for dynamic material characterization. In Proceedings of the DYMAT 2009 Conference, Brussels, Belgium, 7–11 September 2009; pp. 35–41. [Google Scholar] [CrossRef]
- Peirs, J.; Verleysen, P.; Degrieck, J.; Coghe, F. The use of hat-shaped specimens to study the high strain rate shear behaviour of Ti-6Al-4V. Int. J. Impact Eng. 2010, 37, 703–714. [Google Scholar] [CrossRef]
- Reyes, A.; Eriksson, M.; Lademo, O.; Hopperstad, O.S.; Langseth, M. Assessment of yield and fracture criteria using shear and bending tests. Mater. Des. 2009, 30, 596–608. [Google Scholar] [CrossRef]
- Keller, C. Etude expérimentale des transitions volume/surface des propriétés mécaniques du nickel polycristallin de haute pureté. Ph.D. Thesis, Université de Caen/Basse-Normandie, Caen, France, 2009. [Google Scholar]


| Material Parameters | Symbols | Unit | Magnitude |
|---|---|---|---|
| Young’s modulus | E | MPa | 210,000 |
| Poisson’s ratio | ν | — | 0.31 |
| Hardening constant | c | MPa | 934.25 |
| Exponent hardening | n | — | 0.57157 |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yuan, S.; Duchêne, L.; Milis, O.; Keller, C.; Hug, E.; Habraken, A.-M. A Novel Geometry for Shear Test Using Axial Tensile Setup. Proceedings 2018, 2, 422. https://doi.org/10.3390/ICEM18-05277
Yuan S, Duchêne L, Milis O, Keller C, Hug E, Habraken A-M. A Novel Geometry for Shear Test Using Axial Tensile Setup. Proceedings. 2018; 2(8):422. https://doi.org/10.3390/ICEM18-05277
Chicago/Turabian StyleYuan, Sibo, Laurent Duchêne, Olivier Milis, Clément Keller, Eric Hug, and Anne-Marie Habraken. 2018. "A Novel Geometry for Shear Test Using Axial Tensile Setup" Proceedings 2, no. 8: 422. https://doi.org/10.3390/ICEM18-05277
APA StyleYuan, S., Duchêne, L., Milis, O., Keller, C., Hug, E., & Habraken, A.-M. (2018). A Novel Geometry for Shear Test Using Axial Tensile Setup. Proceedings, 2(8), 422. https://doi.org/10.3390/ICEM18-05277




