Predicting Ground Reaction Forces in Sprint Running Using a Shank Mounted Inertial Measurement Unit †
Abstract
:1. Introduction
- Start Phase—athlete set in blocks until instance where both feet have left the blocks.
- Drive/Pickup phase—end of start phase until the trunk is fully erect.
- Maintenance phase—until the sprint is completed.
2. Materials and Methods
2.1. Materials
2.2. Methods
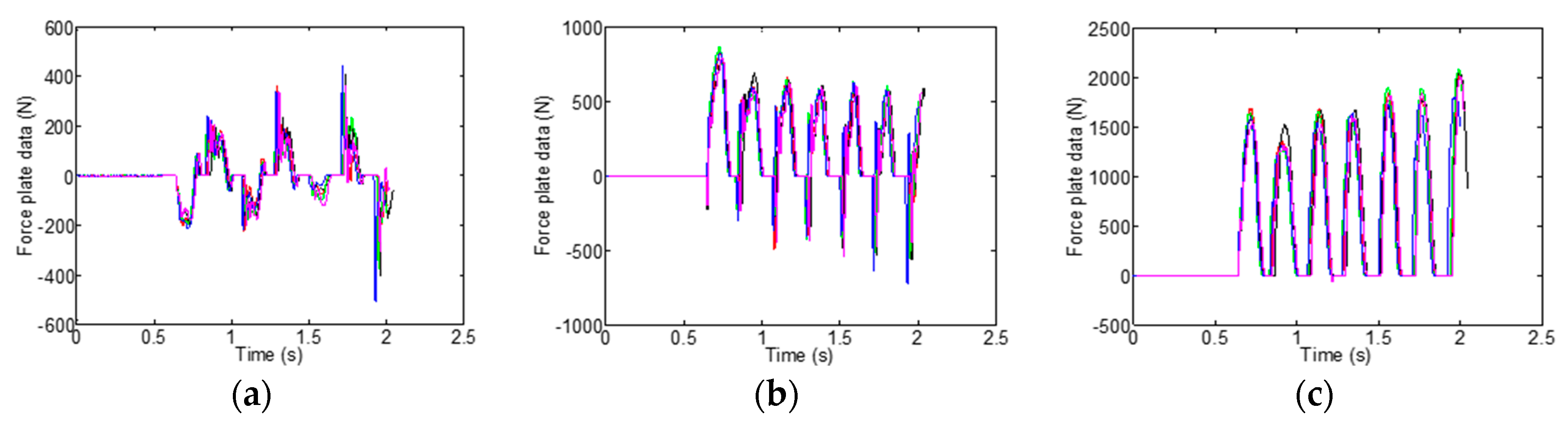
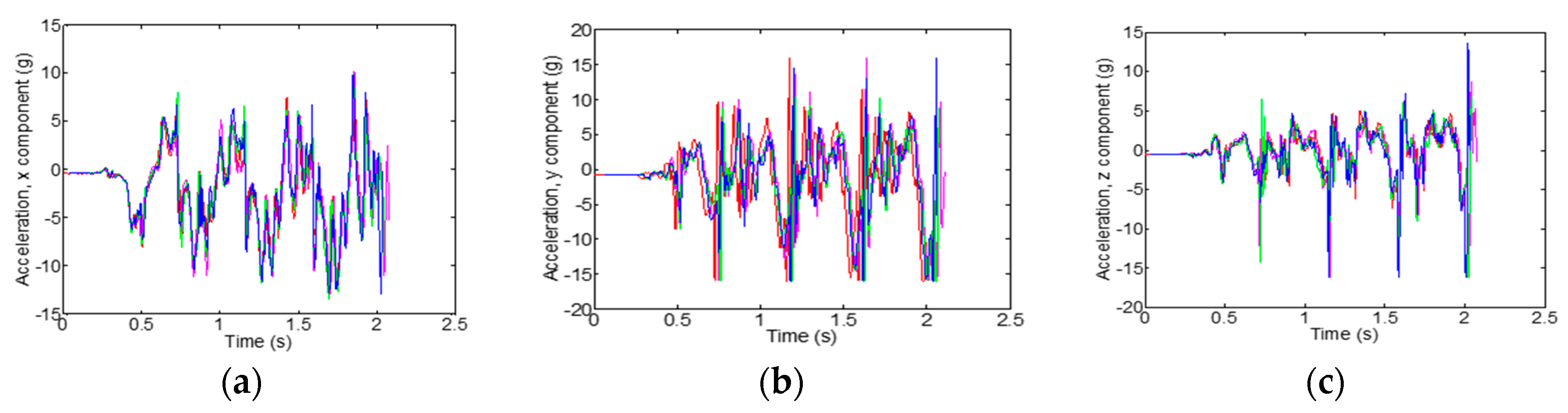
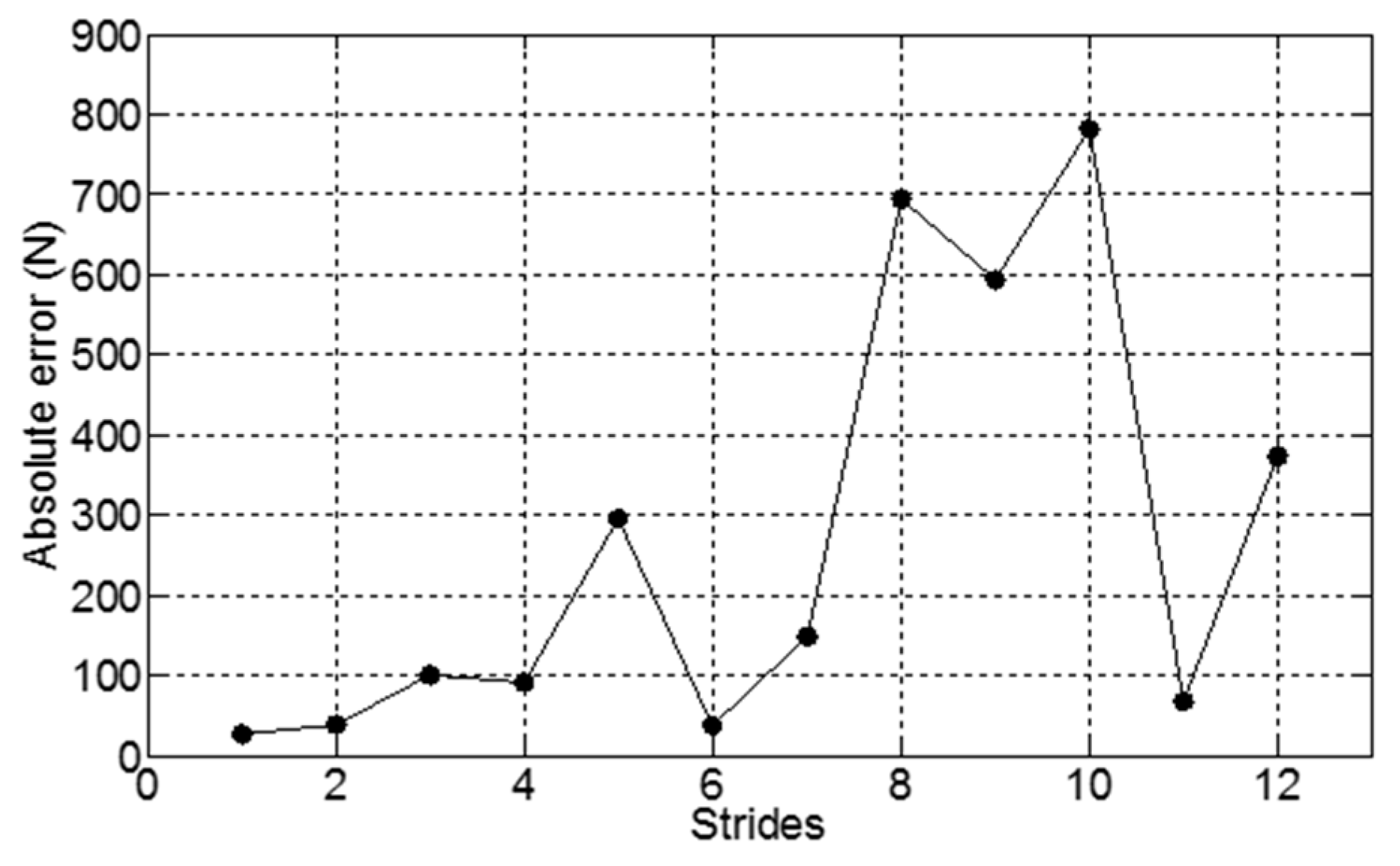
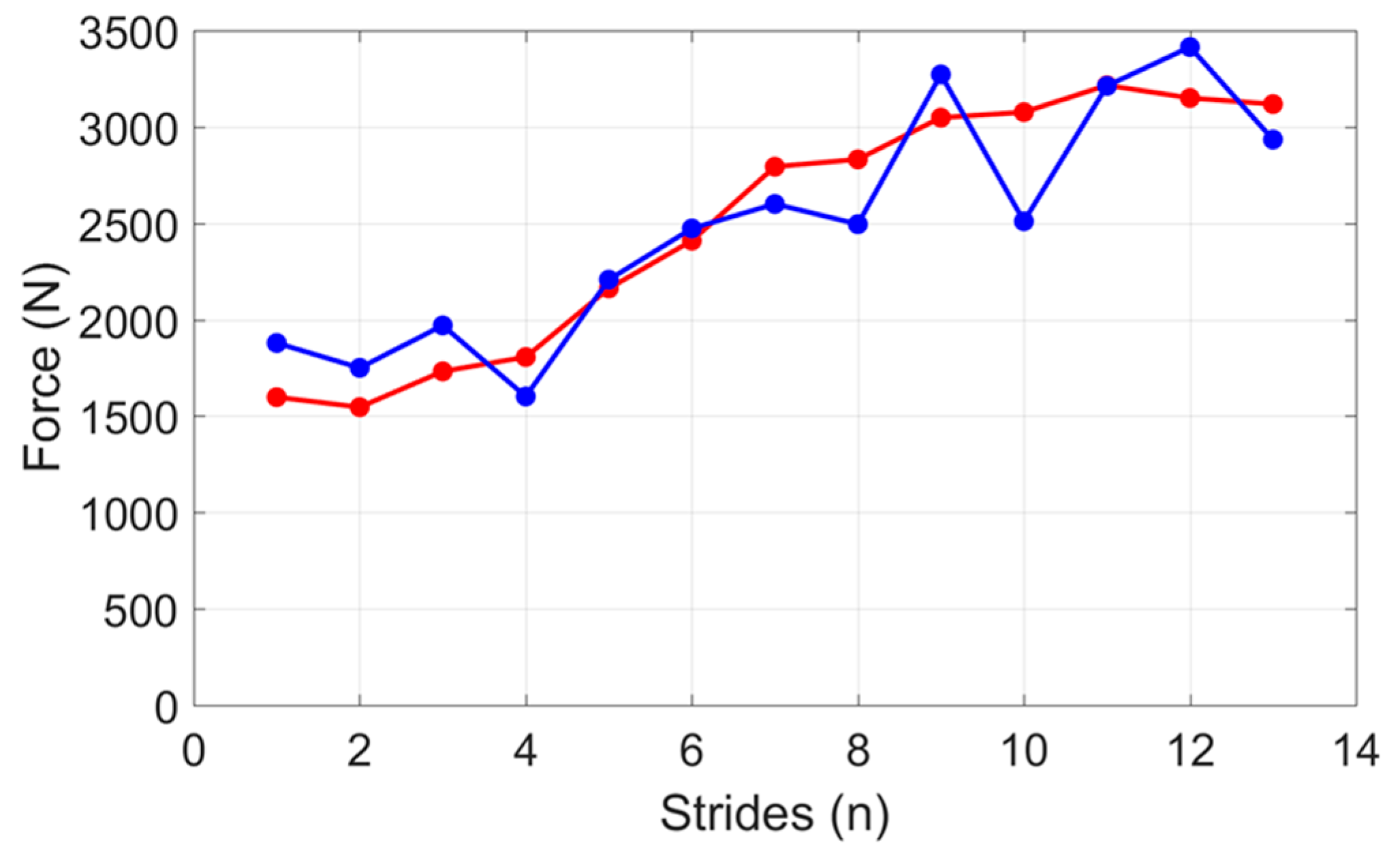
3. Results
4. Discussion
5. Conclusions
Acknowledgments
Conflicts of Interest
References
- International Association of Athletics Federations (IAAF). 100 Metres Men. In Senior Outdoor 2016 100 Metres Men; Monte IAAF: Carlo, Monaco, 2016; Available online: https://www.iaaf.org/records/toplists/sprints/100-metres/outdoor/men/senior/2016 (accessed on 5 November 2017).
- Philpott, L.K. Assessing wireless inertia measurement units for monitoring athletics sprint performance. In Proceedings of the 2014 IEEE Sensors, Valencia, Spain, 2–5 November 2014. [Google Scholar]
- Kawamori, N.; Nosaka, K.; Newton, R.U. Relationships between ground reaction impulse and sprint acceleration performance in team sport athletes. J. Strength Cond. Res. 2013, 27, 568–573. [Google Scholar] [CrossRef] [PubMed]
- Enoka, R. Neuromechanics of Human Movement, 5th ed.; Human Kinetics: Champaign, IL, USA, 2015. [Google Scholar]
- Liu, Y.; Chen, C.-K.; Chen, C.-Y. Optimization of ground reaction force during 100 m-sprint. In Proceedings of the ISBS, San Francisco, CA, USA, 30 March 2001; Volume 1, pp. 76–78. [Google Scholar]
- Rabita, G.; Dorel, S.; Slawinski, J.; Saez-de-Villarreal, E.; Couturier, A.; Samozino, P.; Morein, J. Sprint mechanics in world-class athletes: A new insight into the limits of human locomotion: Sprint mechanics in elite athletes. Scand. J. Med. Sci. Sports 2015, 25, 583–594. [Google Scholar] [CrossRef] [PubMed]
- Ortega, D.R.; Bíes, E.C.R.; de la Rosa, F.J.B. Analysis of the vertical ground reaction forces and temporal factors in the landing phase of a countermovement jump. J. Sports Sci. Med. 2010, 9, 282–287. [Google Scholar] [PubMed]
- Schmidt, M.; Rheinländer, C.; Nolte, K.F.; Wille, S.; Wehn, N.; Jaitner, T. IMU-based determination of stance duration during sprinting. Procedia Eng. 2016, 147, 747–752. [Google Scholar] [CrossRef]
- Espinosa, H.G.; Lee, J.; James, D.A. The inertial sensor: A base platform for wider adoption in sports science applications. J. Fit. Res. 2015, 4, 13–20. [Google Scholar]
- ALai, *!!! REPLACE !!!*; James, D.A.; Hayes, J.P.; Harvey, E.C. Semi-automatic calibration technique using six inertial frames of reference. In Proceedings of the SPIE Microelectronics: Design, Technology, and Packaging, Perth, Australia, 10–12 December 2003; Volume 5274, pp. 531–542. [Google Scholar]
- Leadbetter, R.; James, D. Platform technologies and visualisation tools for sensors in sport and health applications. Hum. Technol. 2016, 1, 9–10. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Thiel, D.V.; Shepherd, J.; Espinosa, H.G.; Kenny, M.; Fischer, K.; Worsey, M.; Matsuo, A.; Wada, T. Predicting Ground Reaction Forces in Sprint Running Using a Shank Mounted Inertial Measurement Unit. Proceedings 2018, 2, 199. https://doi.org/10.3390/proceedings2060199
Thiel DV, Shepherd J, Espinosa HG, Kenny M, Fischer K, Worsey M, Matsuo A, Wada T. Predicting Ground Reaction Forces in Sprint Running Using a Shank Mounted Inertial Measurement Unit. Proceedings. 2018; 2(6):199. https://doi.org/10.3390/proceedings2060199
Chicago/Turabian StyleThiel, David V., Jonathan Shepherd, Hugo G. Espinosa, Megan Kenny, Katrien Fischer, Matthew Worsey, Akifumi Matsuo, and Tomohito Wada. 2018. "Predicting Ground Reaction Forces in Sprint Running Using a Shank Mounted Inertial Measurement Unit" Proceedings 2, no. 6: 199. https://doi.org/10.3390/proceedings2060199
APA StyleThiel, D. V., Shepherd, J., Espinosa, H. G., Kenny, M., Fischer, K., Worsey, M., Matsuo, A., & Wada, T. (2018). Predicting Ground Reaction Forces in Sprint Running Using a Shank Mounted Inertial Measurement Unit. Proceedings, 2(6), 199. https://doi.org/10.3390/proceedings2060199






