An Experimental Study on the Propagation of the Pressure Fluctuations Induced in a Tube Array under Forced Vibration †
Abstract
:1. Introduction
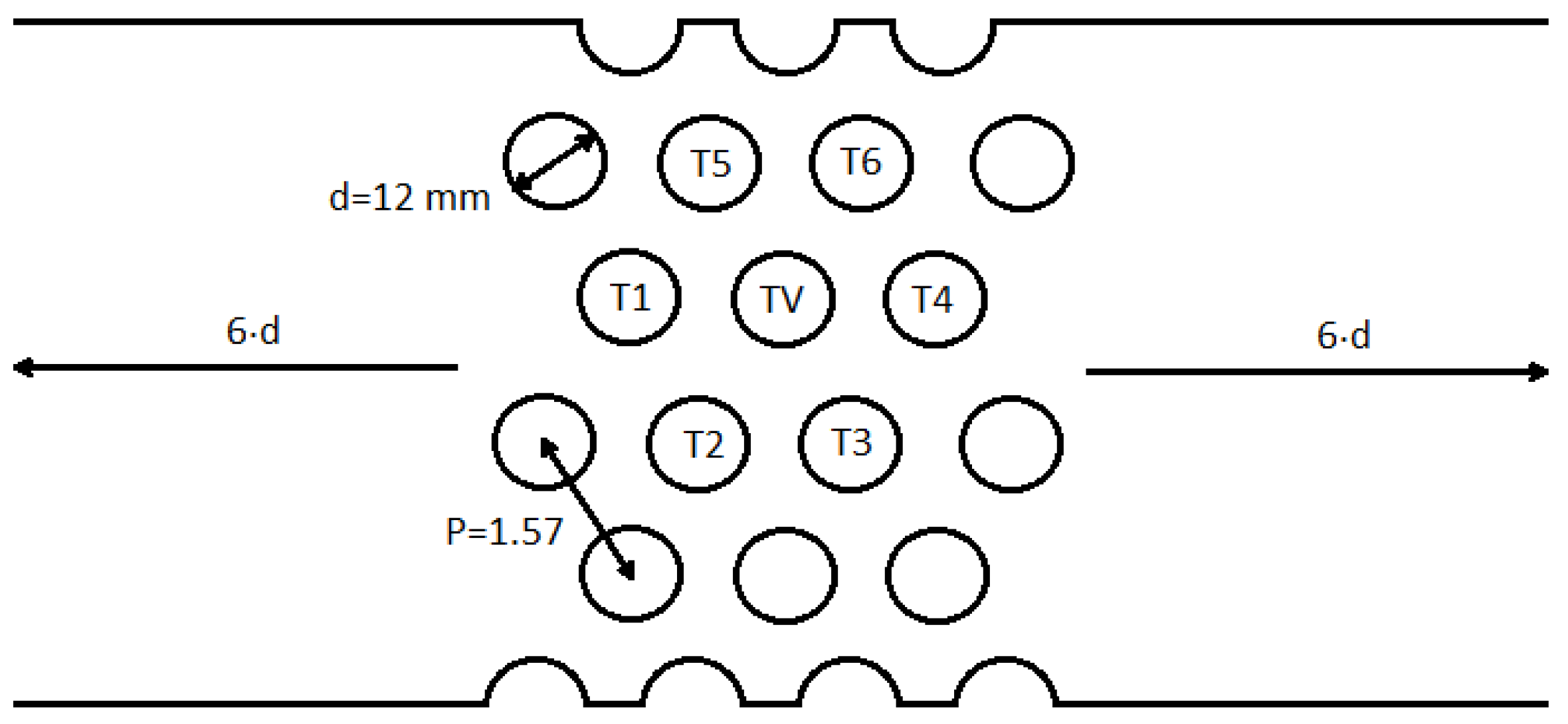
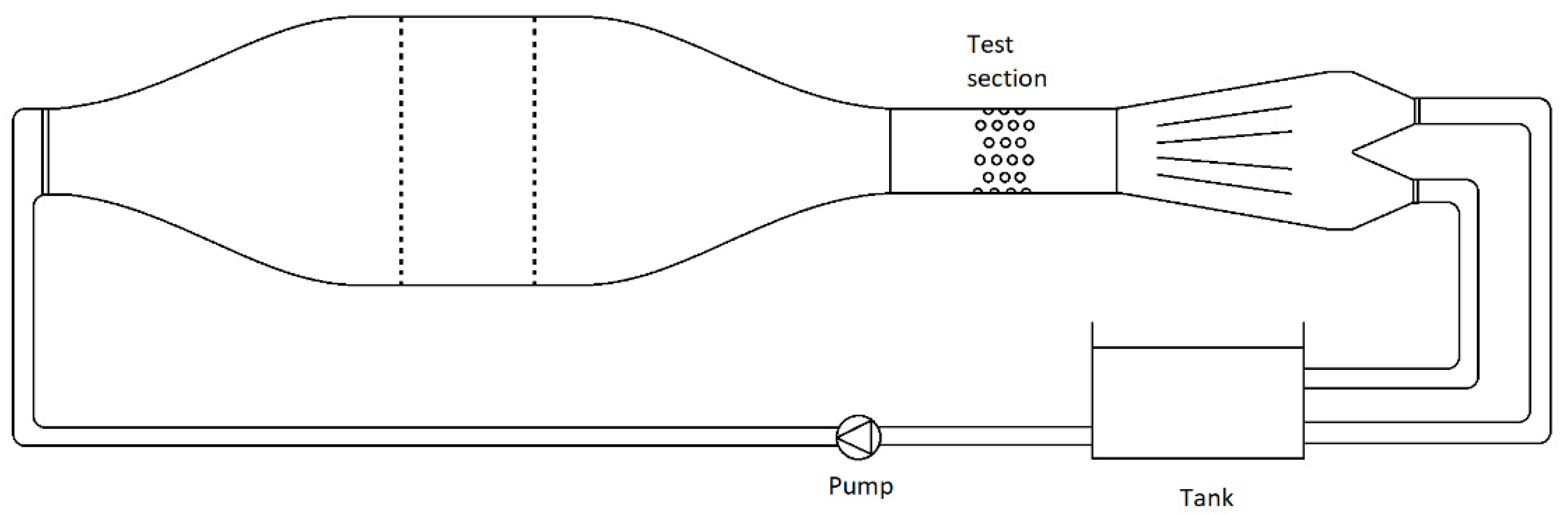
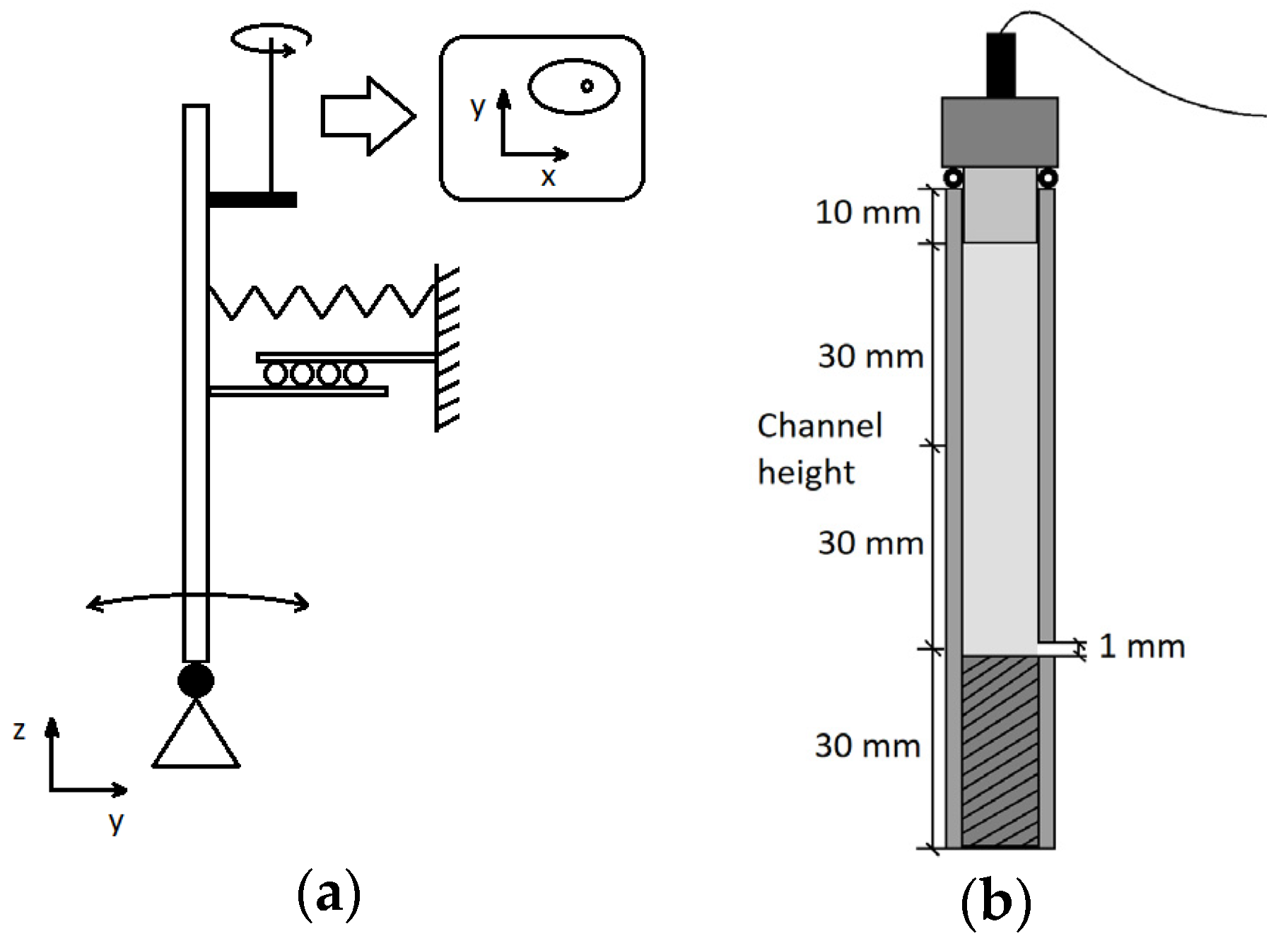
2. Experimental Set-Up
3. Experimental Procedure and Signal Processing
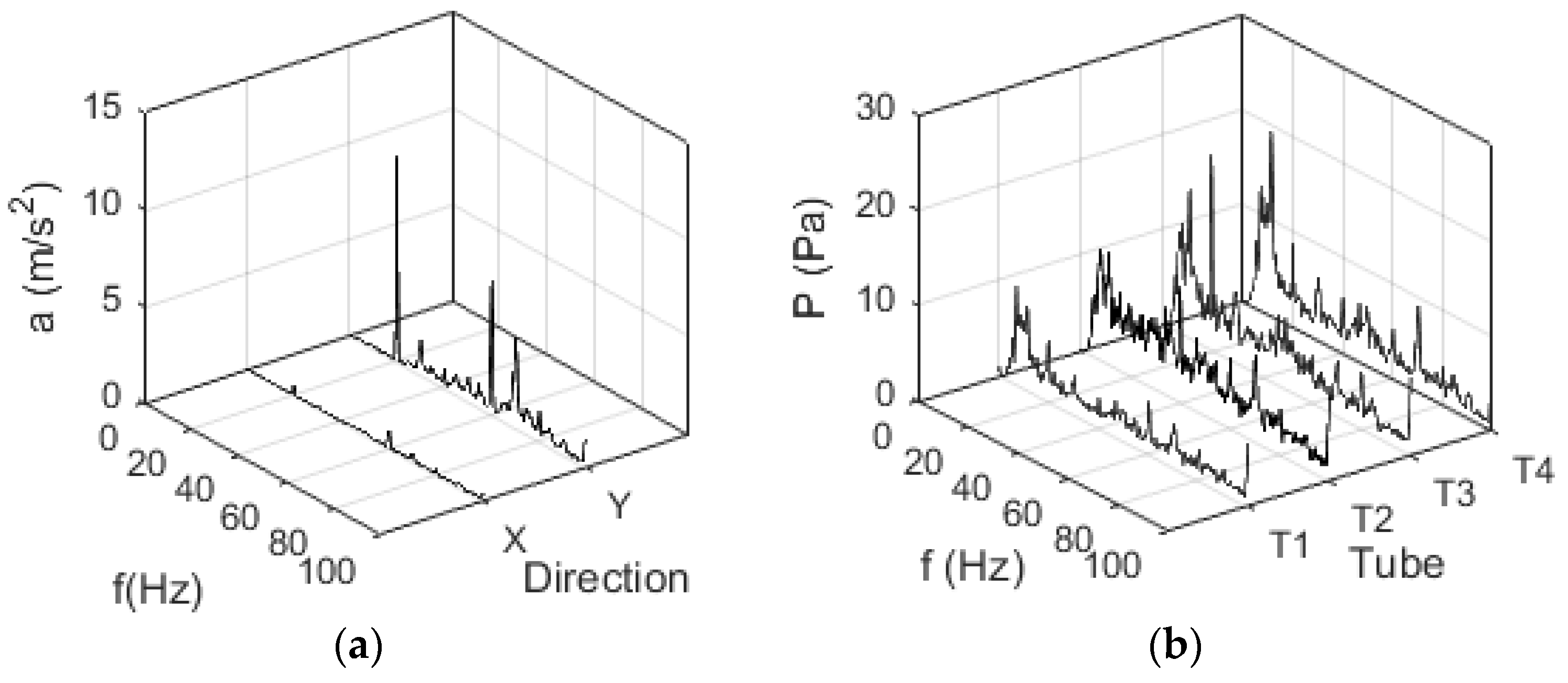
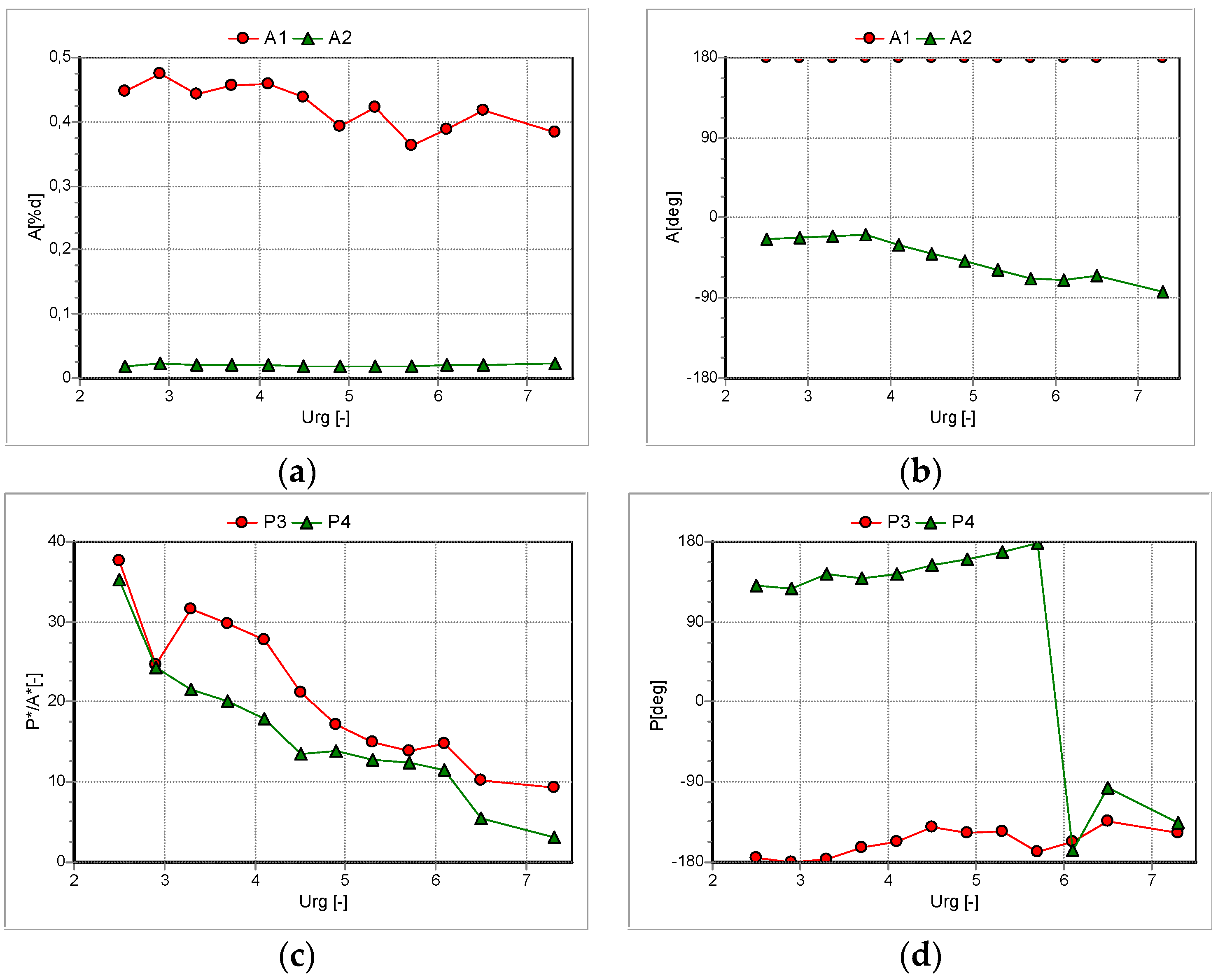
4. Results
5. Conclusions
- Amplitude of pressure perturbations in the cylinder downstream the vibrating tube is higher and also phase is closer to tube motion one compared to results of the tube upstream, as perturbations that are being transmitted through the water stream also are dragged downstream for it.
- Pressure perturbations are lower for higher cross flow reduced velocities, as tube velocity generating those perturbations is getting less important in comparison to cross flow velocity.
- Although the present work is still an ongoing study, results obtained with the experimental methodology developed are promising, future experimental campaigns will be undertaken to extract more information of the empirical test and contrast results with CFD calculations obtained with the previous numerical model [5].
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Lever, J.; Weaver, D. On the stability of heat exchanger tube bundles. Part I: Modified theoretical model. J. Sound Vib. 1986, 107, 375–392. [Google Scholar] [CrossRef]
- Lever, J.; Weaver, D. On the stability of heat exchanger tube bundles. Part II: Numerical results and comparison with experiments. J. Sound Vib. 1986, 107, 393–410. [Google Scholar] [CrossRef]
- Connors, H.J. Fluidelastic vibration of tube arrays excited by cross flow. In Flow-Induced Vibration in Heat Exchangers; Reiff, D.D., Ed.; ASME: New York, NY, USA, 1970; pp. 42–56. [Google Scholar]
- Price, S.J.; Paidoussis, M.P. An improved mathematical model for the stability of cylinder rows subject to cross-flow. J. Sound Vib. 1984, 97, 615–640. [Google Scholar] [CrossRef]
- de Pedro, B.; Parrondo, J.; Meskell, C.; Fernández-Oro, J. CFD modelling of the cross-flow through normal triangular tube arrays with one tube undergoing forced vibrations or fluidelastic instability. J. Fluids Struct. 2016, 64, 67–86. [Google Scholar] [CrossRef]
- Price, S.J. A review of theoretical models for fluidelastic instability of cylinder arrays in cross-flow. J. Fluids Struct. 1995, 9, 463–518. [Google Scholar] [CrossRef]
- Chen, S.S. Instability mechanisms and stability criteria of a group of circular cylinders subjected to cross-flow, part II: Numerical results and discussion. J. Vib. Acoust. Stress Reliab. Des. 1983, 105, 253–260. [Google Scholar] [CrossRef]
- Paidoussis, M.P.; Price, S.J. The mechanisms underlying flow-induced instabilities of cylinder arrays in cross-flow. J. Fluid Mech. 1988, 187, 45–59. [Google Scholar] [CrossRef]
- Granger, S.; Paidoussis, M. An improvement to the quasi-steady model with application to cross-flow-induced vibration of tube arrays. J. Fluid Mech. 1996, 320, 163–184. [Google Scholar] [CrossRef]
- Khalifa, A.; Weaver, D.; Ziada, S. An experimental study of flow-induced vibration and the associated flow perturbations in a parallel triangular tube array. J. Press. Vessel Technol. 2013, 135, 030904. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tufiño, L.; Pedro, B.d.; Laine, G.; Parrondo, J. An Experimental Study on the Propagation of the Pressure Fluctuations Induced in a Tube Array under Forced Vibration. Proceedings 2018, 2, 1501. https://doi.org/10.3390/proceedings2231501
Tufiño L, Pedro Bd, Laine G, Parrondo J. An Experimental Study on the Propagation of the Pressure Fluctuations Induced in a Tube Array under Forced Vibration. Proceedings. 2018; 2(23):1501. https://doi.org/10.3390/proceedings2231501
Chicago/Turabian StyleTufiño, Luis, Beatriz de Pedro, Guillermo Laine, and Jorge Parrondo. 2018. "An Experimental Study on the Propagation of the Pressure Fluctuations Induced in a Tube Array under Forced Vibration" Proceedings 2, no. 23: 1501. https://doi.org/10.3390/proceedings2231501
APA StyleTufiño, L., Pedro, B. d., Laine, G., & Parrondo, J. (2018). An Experimental Study on the Propagation of the Pressure Fluctuations Induced in a Tube Array under Forced Vibration. Proceedings, 2(23), 1501. https://doi.org/10.3390/proceedings2231501




