How Do We Create Experiences for Students That Connect with What They Care About? ^†

Abstract

The main aim of this work is to describe the process by which some students, participating in a teaching experiment, recreate with guidance material and personal attendance some advanced concepts at the doctoral level. More precisely, the students deal with concepts related to pure abstract algebra, beginning with an exploration of the well known mathematical game of the Hanoi Towers on the three rods.

1. Introduction

As it is said in Steffe and Thompson (2000) [1], “A primary purpose for using teaching experiment methodology is for researchers to experience, first-hand, students’ mathematical learning and reasoning. Without the experiences afforded by teaching, there would be no basis for coming to understand the powerful mathematical concepts and operations students construct or even for suspecting that these concepts and operations may be distinctly different from those of researchers”.

Inspired by the ideas of Steffe and Thompson (2000) [1] and Burn (1996) [2] we conducted an educational project doing research on how students might be able to recognize the abstract theory of automorphisms of regular rooted trees based on their background in the context of abstract algebra, and at the same time handling a mathematical puzzle for kids, called the Hanoi Towers.

To conduct the teaching experiment mentioned in this work, we selected two doctoral students who showed in several seminars a high level knowledge on the abstract theory of automorphisms of trees. They voluntarily offered themselves to participate in the experimental project. The guide teacher provided them with different materials and numerous videos related to the Hanoi Towers game and to graph theory. The three of them met several times to discuss the most important insights provided by the analysis of the materials. These discussions forced the students to develop the new formal concepts and to find the resemblances with what they previously knew. One of our fundamental goals was that the students should be able to recreate formally, with guidance and personal attendance, their background in the subject. Discovering students’ strategies and ways of thinking that anticipated the fundamental description of the involved concepts in the process, and developing an useful autonomous mechanism to carry out the understanding of the formal theory were crucial to achieve that objective. The importance of the experiment is twofold. Firstly, the guided recreation of the developed concepts allowed learners to regard the knowledge they obtained as their own individual knowledge for which they themselves were the unique responsible. Secondly, by manipulating applied examples under the conventional rules of algebra, these students gained the ability to transform some everyday life problems into the language of mathematics.

2. Background

Background of the Game

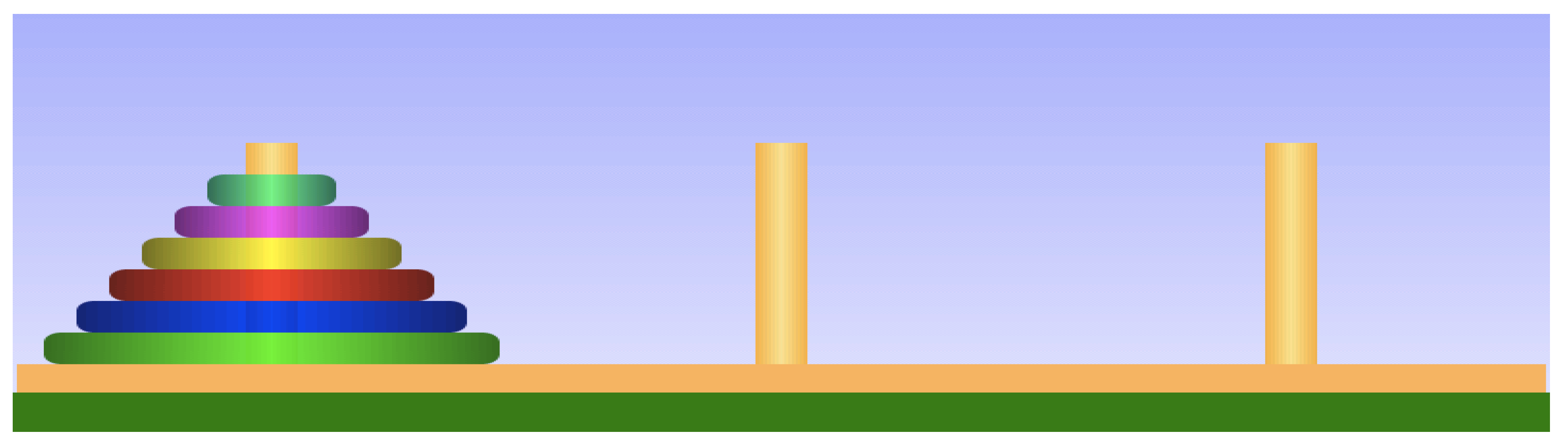
The Hanoi Towers game was invented by the French mathematician Èdouard Lucas in 1883. There is a story about an Indian monastery in Vietnam which contains a large room with three old posts in it surrounded by 64 golden disks. Brahmin priests, acting under the orders of an ancient prophecy, have been moving these disks in accordance with some rules. According to the legend, when the last movement is completed, the world will end. To illustrate the challenge hidden in the prophecy, if the legend were true, and if the priests were able to move the disks on an average of one per second, using the smallest number of moves it would take 2⁶⁴-1 s, approximately 585 billion years, to reach the last movement.

The game consists of three rods and a number of disks of different sizes. It starts with the disks in a neat stack in descending order of size on one rod, the smallest at the top, thus making a conical shape. The purpose of the game is to move the entire stack to another rod, obeying some simple rules:

(1)

Only one disk can be moved at a time;

(2)

Each move consists of taking the upper disk from one of the stacks and placing it on the top of another stack;

(3)

No disk may be placed on the top of a smaller disk.

The puzzle (see Figure 1) can be played with any number of disks, although many toy versions have around 7 to 9 of them.

3. General Motivation and Justification of the Process

The traditional teaching dynamic posits the teacher as the expert and students as recipients of an externally located knowledge. The unilateral transmission of ideas from teacher to students often fails to engage students, since it focuses on the result rather than the process of education. Attending and listening skills are essential to create positive interpersonal relations in any context. Examples of these skills include: active listening, attending and encouraging, open questions, closed questions, rewording and summarizing. Some of the schemes of utmost importance in the process were: the reflective observation, to work on the necessary bonds, taking action and getting involved. Generally, formal definitions would only appear at the end of a period of exploration.

Another essential scheme was that students should obtain, through non-expected strategies, the necessary skills to manipulate elementary algebraic expressions. Along this process, graphical representation played a key role in conceptual understanding. Looking behind what students do, an effort to understand their mathematical realities is an essential part of this teaching experiment.

Our experiment was based on the case of two doctoral students who voluntarily offered themselves to participate in it. The teaching experiment was carried out in the form of several critical episodes for 2 months. Each of those episodes was based on the constructivist experiment involved in the reinvention process and on our learning activity as instructional designers. (Due to the length restriction of the work, the discussed episodes and the used mathematical materials are omitted.)

Elena and Marialaura are two PHD strong students who had received an A grade in both their Mathematics degree and Master studies. After providing Elena and Marialaura with several materials related to the game and some videos related to graph theory, the guide met them several times (each session lasted 50 min). The first meeting was two weeks after receiving all the material. The three of them met to discuss the most important insights provided by the analysis of those materials. This discussion forced them to develop the new formal concepts and to find the resemblances with what they previously knew. Having checked everyone’s availability, the next meetings took place on successive days. All of these meetings consisted of problem based pair work combined with discussion. The guide occasionally interjected with interview type questions in order to know more about the individual student’s thinking, and as a teacher and as a guide also played a student role in many occasions, learning form the doctoral students several mathematical concepts in a different rewarding way.

The sessions were videotaped and the students’ work collected. Thus, the retrospective data study mainly consisted of multiple stages of iterative analysis of those gathered videos and students’ written work, and of reflective observations of the mathematical activities in the group tasks.

The small group formed by the students became a problem-solving instrument. They learned how to work together in order to accomplish group goals, and how to find ways to play their strengths and to complement each other’s effort.

We firmly believe that this kind of work with a small number of students can support the development of an instructional theory to be used in designing curriculum.

4. Conclusions

The mathematical results obtained during the process were mainly the following:

(1)

A better understanding of the n-th Mersenne number, which corresponds to the minimal number of moves required to solve a Hanoi Towers puzzle, being n the number of disks.

(2)

A development of the recursive method for any mathematical proof, and a high understanding of the process of seeking the resemblances between the Sierpinski triangle, the graphical representation of the solution of the game, and the study of the automorphisms of a regular tree.

(3)

During all the process, the students constructed a valuable procedure to determine those resemblances between the automorphisms of rooted regular trees and the graphical representation of the solution of the initial game, reinventing at the same time all the concepts involved in them.

Related to the learning process, we emphasize that the potential value of the context in which the problem was placed and the dynamic group work were really appreciated by the guide, as well as the great interest in the proposed challenge shown by the participants. The guide felt that the role had been reversed, being sometimes the students the ones that inculcated the guide reinvented proofs and ways of thinking with non-conventional representational forms.

Our team’s goal is to perform in the future this type of experiments with a greater number of graduate students.