Optimization of Si-Based Waveguides for Evanescent-Field Sensors †
Abstract
:1. Introduction
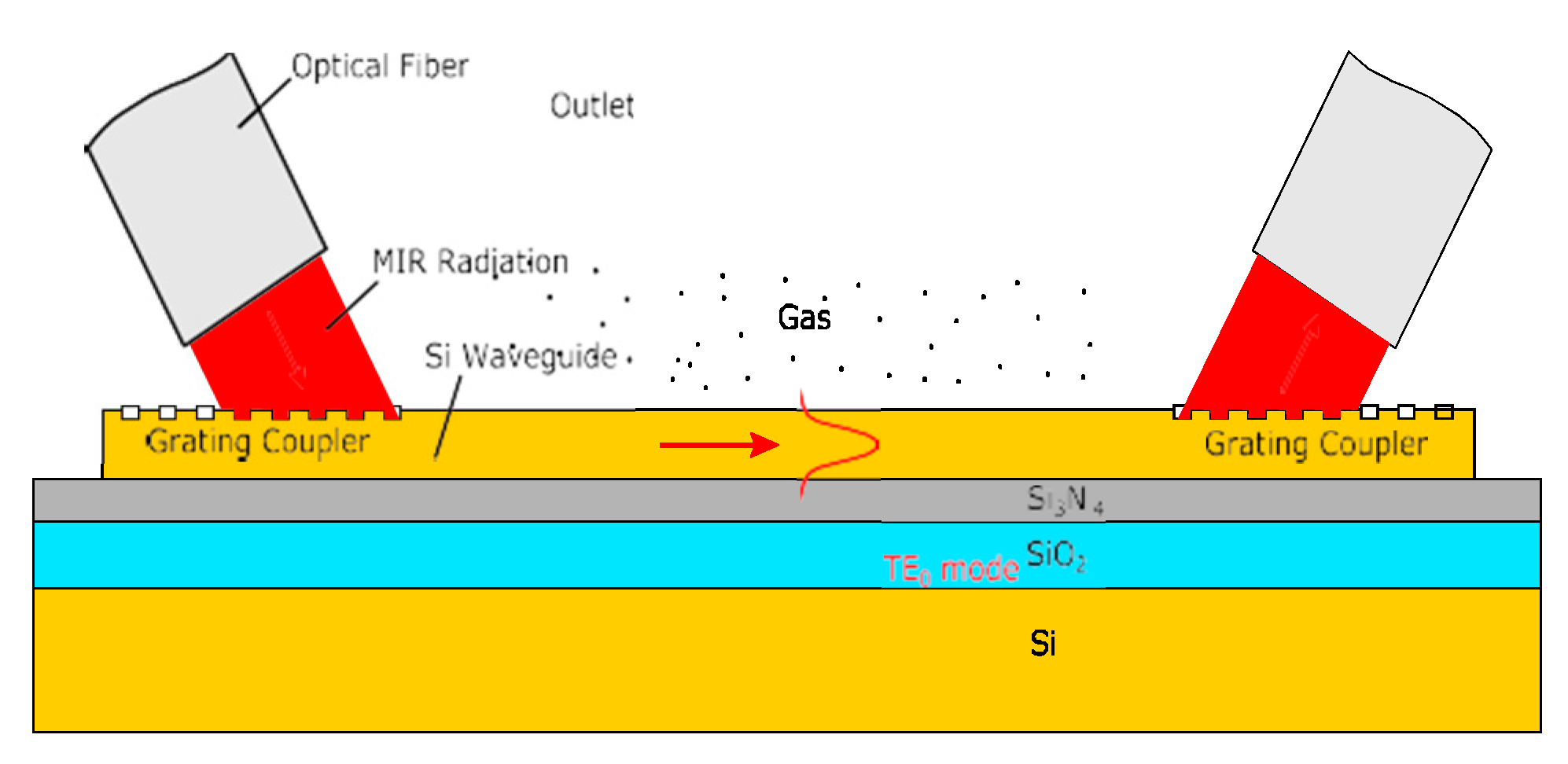
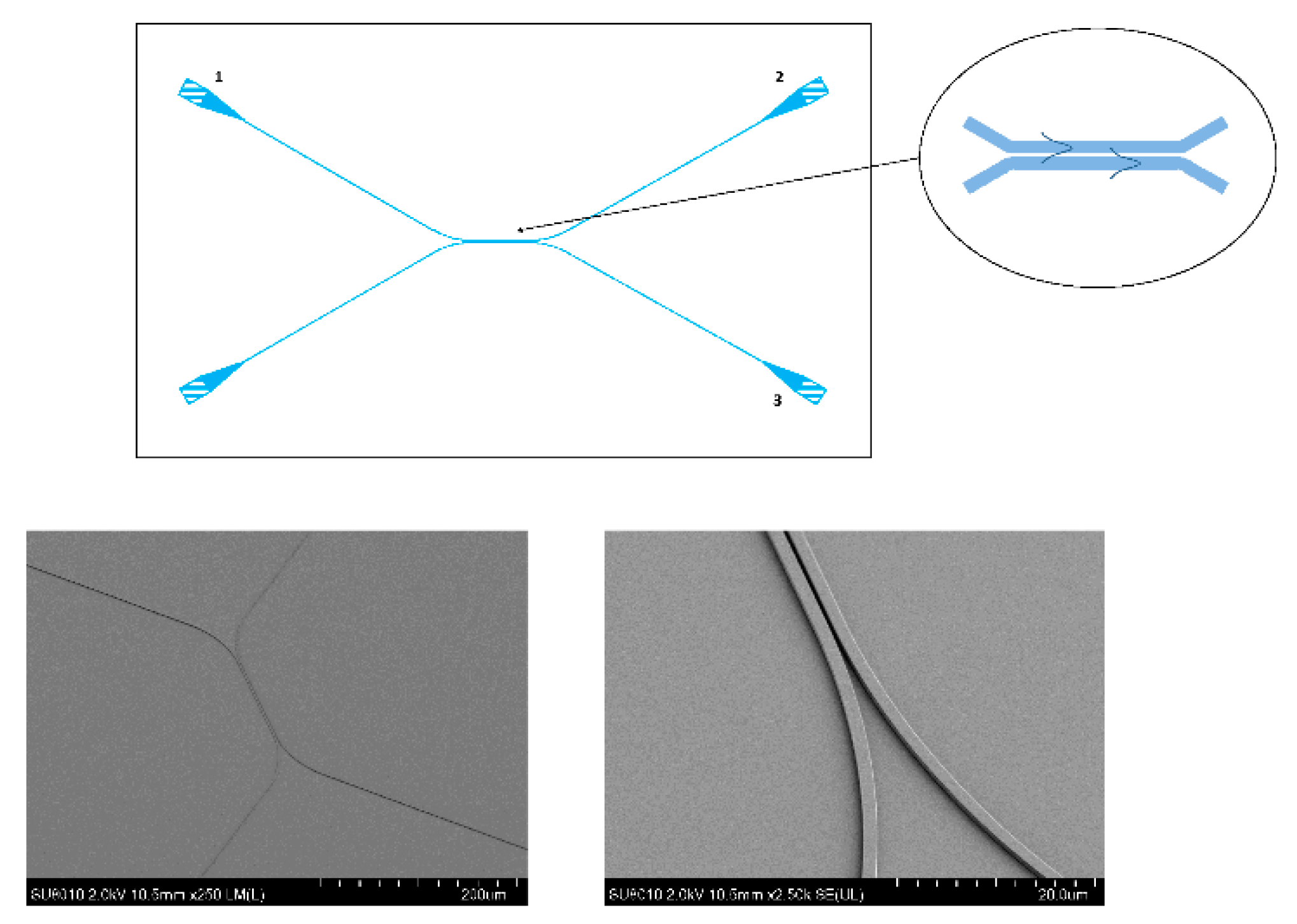
2. Experimental
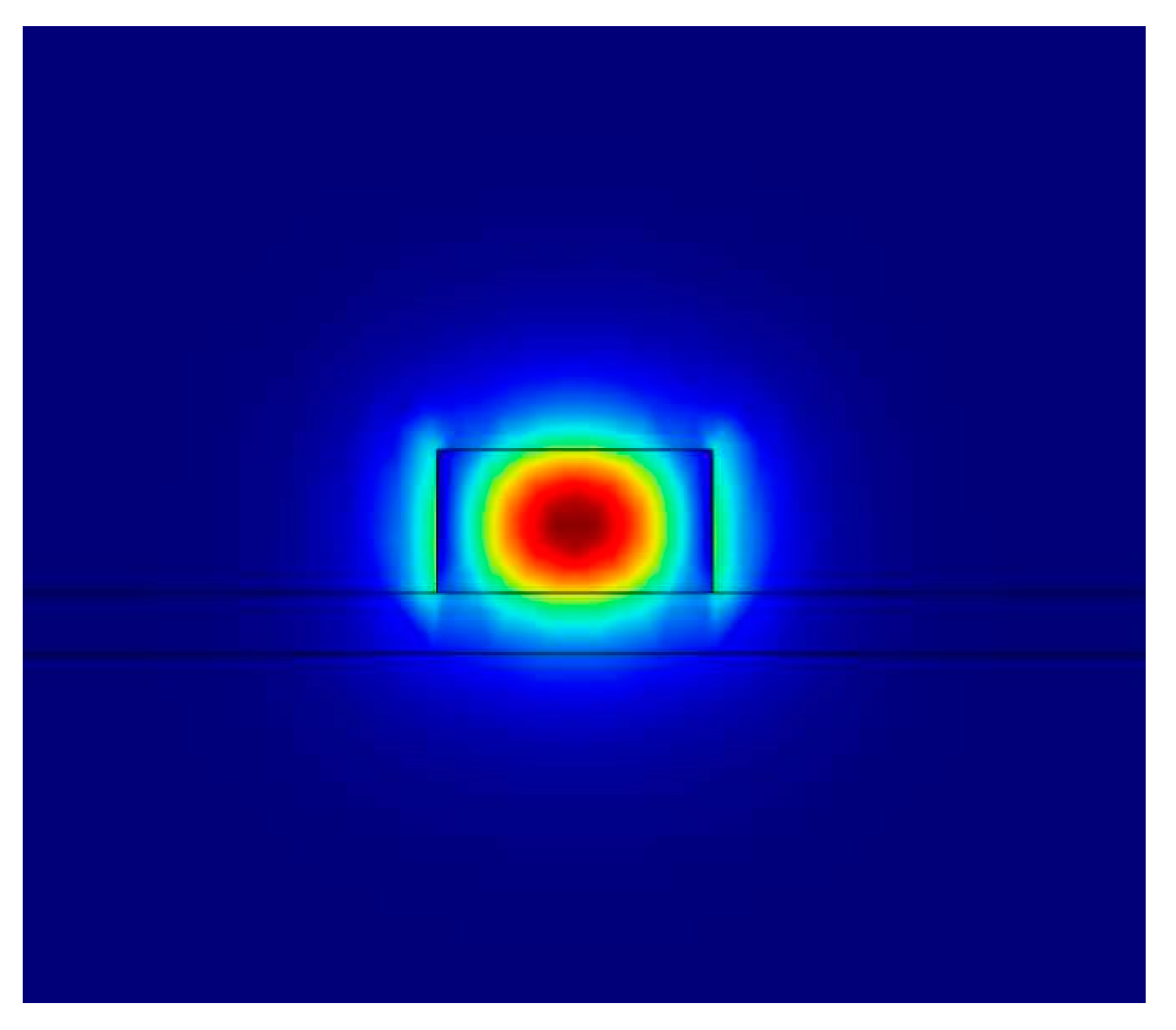
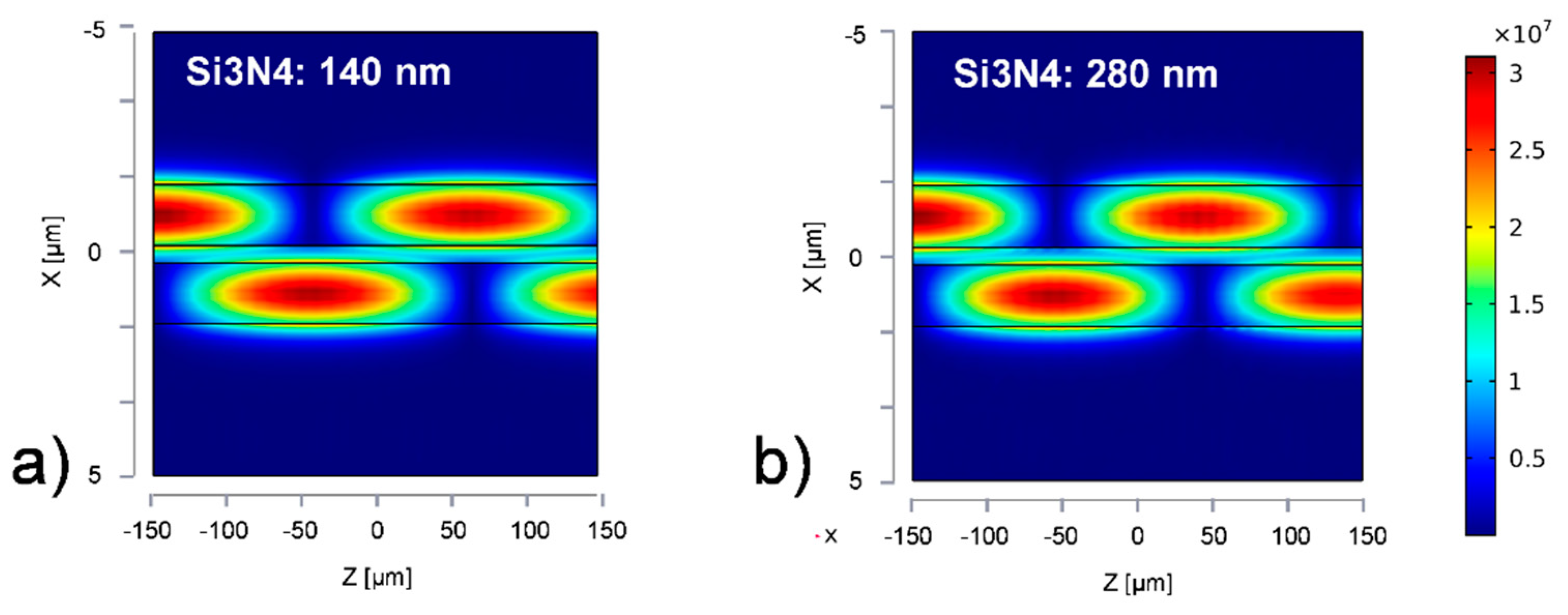
3. Results
4. Conclusions
Acknowledgments
Conflicts of Interest
References
- Lavchiev, V.; Jakoby, B. Photonics in the Mid-Infrared: Challenges in Single-Chip Integration and Absorption Sensing. IEEE J. Sel. Top. Quantum Electron. 2017, 23, 452–463. [Google Scholar] [CrossRef]
- Ranacher, C.; Consani, C.; Hedenig, U.; Grille, T.; Lavchiev, V.; Jakoby, B. A photonic silicon waveguide gas sensor using evanescent-wave absorption. In Proceedings of the 2016 IEEE SENSORS, Orlando, FL, USA, 30 October–3 November 2016; IEEE: Orlando, FL, USA, 2016; pp. 1–3. [Google Scholar]
- Ranacher, C.; Consani, C.; Tortschanoff, A.; Jannesari, R.; Bergmeister, M.; Grille, T.; Jakoby, B. Mid-infrared absorption gas sensing using a silicon strip waveguide. Sens. Actuators A Phys. 2018, 277, 117–123. [Google Scholar] [CrossRef]
- Polyanskiy, M.N. Refractive Index Database. Available online: https://refractiveindex.info (accessed on 2 May 2018).

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tortschanoff, A.; Ranacher, C.; Consani, C.; Grille, T.; Moridi, M. Optimization of Si-Based Waveguides for Evanescent-Field Sensors. Proceedings 2018, 2, 739. https://doi.org/10.3390/proceedings2130739
Tortschanoff A, Ranacher C, Consani C, Grille T, Moridi M. Optimization of Si-Based Waveguides for Evanescent-Field Sensors. Proceedings. 2018; 2(13):739. https://doi.org/10.3390/proceedings2130739
Chicago/Turabian StyleTortschanoff, Andreas, Christian Ranacher, Cristina Consani, Thomas Grille, and Mohssen Moridi. 2018. "Optimization of Si-Based Waveguides for Evanescent-Field Sensors" Proceedings 2, no. 13: 739. https://doi.org/10.3390/proceedings2130739
APA StyleTortschanoff, A., Ranacher, C., Consani, C., Grille, T., & Moridi, M. (2018). Optimization of Si-Based Waveguides for Evanescent-Field Sensors. Proceedings, 2(13), 739. https://doi.org/10.3390/proceedings2130739




