Screen-Printed Microcantilevers for Environmental Sensing †
Abstract
:1. Introduction
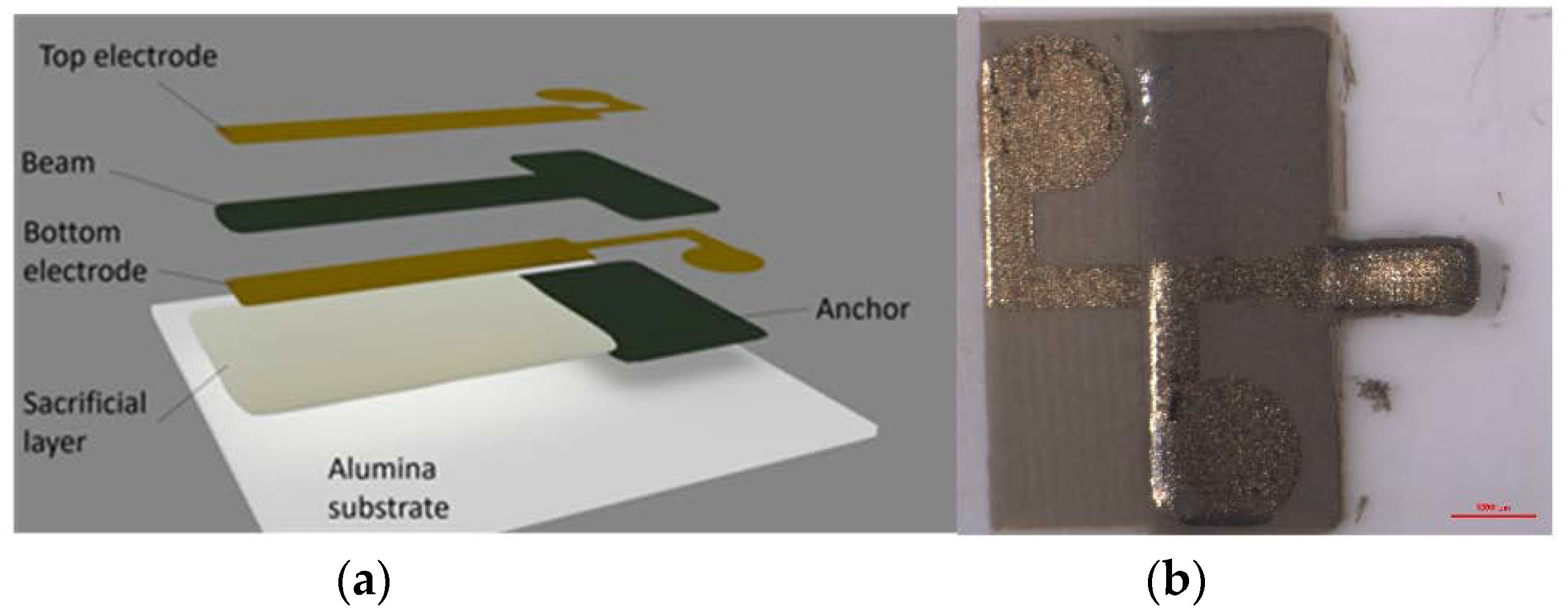
2. Materials and Methods
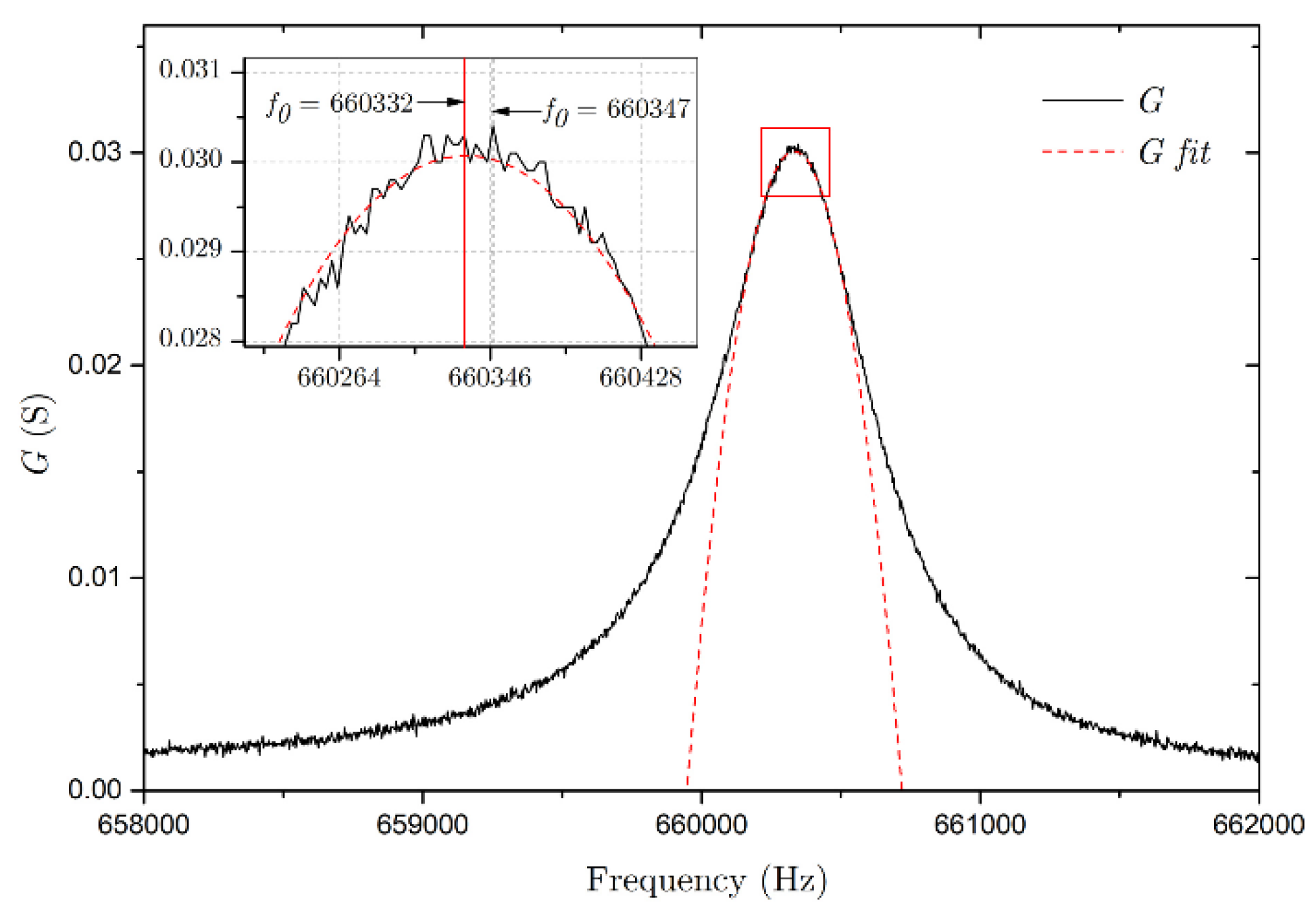
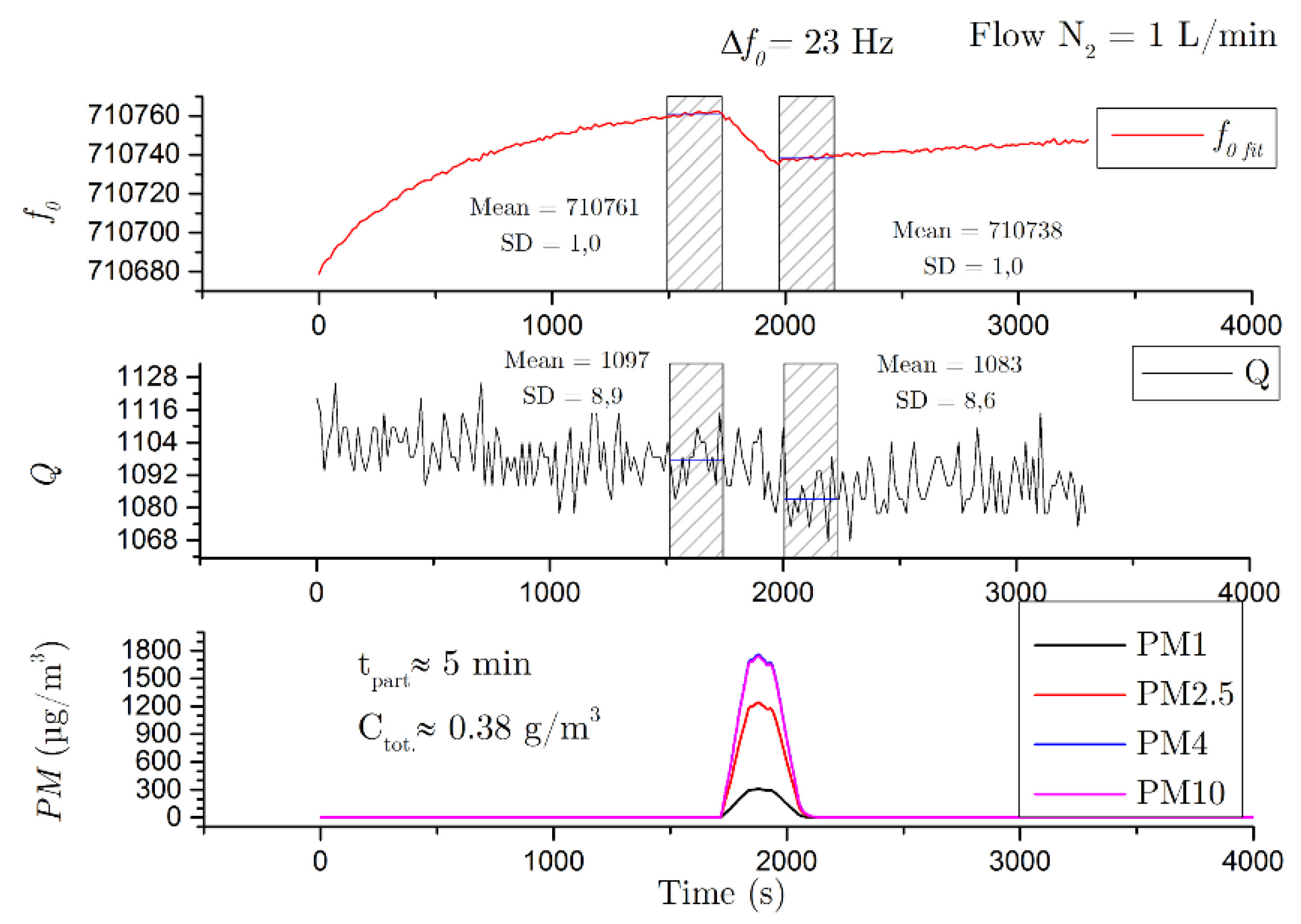
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Fabrication: The Microcantilevers are Entirely Screen-Printed in the Order Indicated Table A1.
| Step | Layer | Material | Thickness (μm) 1 |
|---|---|---|---|
| 1 | Anchor | PZT | 35 |
| 2 | Sacrificial Layer | Polyester | 35 |
| 3 | Bottom Electrode | Gold | 15 |
| 4 | Beam | PZT | 120 |
| 5 | Top Electrode | Gold | 8 |
Appendix B. Equations
References
- World Health Organization. Evolution of WHO Air Quality Guidelines—Past, Present and Future. 2017; p. 21. Available online: http://www.euro.who.int (accessed on 6 December 2018).
- Boisen, A.; Dohn, S.; Keller, S.S.; Schmid, S.; Tenje, M. Cantilever-like micromechanical sensors. Rep. Prog. Phys. 2011, 74, 36101. [Google Scholar] [CrossRef]
- Wasisto, H.S.; Uhde, E.; Peiner, E. Enhanced performance of pocket-sized nanoparticle exposure monitor for healthy indoor environment. Build. Environ. 2016, 95, 13–20. [Google Scholar] [CrossRef]
- Clément, P.; Perez, E.D.; Gonzalez, O.; Calavia, R.; Lucat, C.; Llobet, E.; Debéda, H. Gas discrimination using screen-printed piezoelectric cantilevers coated with carbon nanotubes. Sens. Actuator, B Chem. 2016, 237, 1056–1065. [Google Scholar] [CrossRef]
- Debéda, H.; Lucat, C.; Pommier-Budinger, V. Printed Piezoelectric Materials for Vibration-based Damage Detection. Procedia Eng. 2016, 168, 708–712. [Google Scholar] [CrossRef]
- Liu, J.; Hao, W.; Liu, M.; Liang, Y.; He, S. A Novel Particulate Matter 2.5 Sensor Based on Surface Acoustic Wave Technology. Appl. Sci. 2018, 8, 82. [Google Scholar] [CrossRef]
- Hong, C.H.; Kim, H.P.; Choi, B.Y.; Han, H.S.; Son, J.S.; Ahn, C.W.; Jo, W. Lead-free piezoceramics—Where to move on? J. Mater. 2016, 2, 1–24. [Google Scholar] [CrossRef]


| L/w (mm) | f0 th. (kHz) | f0 exp. (kHz) | σ(Hz) | Q exp. | S (Hz/μg) |
|---|---|---|---|---|---|
| 8/2 | 90 | 90 | 1.5 | 100 | −5 |
| 8/1 | 90 | 100 | 3.6 | 150 | −10 |
| 4/2 | 170 | 180 | 0.9 | 250 | −20 |
| 4/1 | 170 | 180 | 0.9 | 300 | −40 |
| 2/2 | 370 | 340 | 0.8 | 250 | −100 |
| 2/1 | 360 | 340 | 0.7 | 600 | −200 |
| 1/2 | 810 | 780 | 1.2 | 800 | −400 |
| 1/1 | 790 | 620 | 0.6 | 1300 | −1000 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grall, S.; Debéda, H.; Dufour, I.; Aubry, V. Screen-Printed Microcantilevers for Environmental Sensing. Proceedings 2018, 2, 722. https://doi.org/10.3390/proceedings2130722
Grall S, Debéda H, Dufour I, Aubry V. Screen-Printed Microcantilevers for Environmental Sensing. Proceedings. 2018; 2(13):722. https://doi.org/10.3390/proceedings2130722
Chicago/Turabian StyleGrall, Simon, Hélène Debéda, Isabelle Dufour, and Vincent Aubry. 2018. "Screen-Printed Microcantilevers for Environmental Sensing" Proceedings 2, no. 13: 722. https://doi.org/10.3390/proceedings2130722
APA StyleGrall, S., Debéda, H., Dufour, I., & Aubry, V. (2018). Screen-Printed Microcantilevers for Environmental Sensing. Proceedings, 2(13), 722. https://doi.org/10.3390/proceedings2130722





