Optimal Selection of Pumps As Turbines in Water Distribution Networks ^†

Abstract

Pumps As Turbines (PATs) can be installed in Water Distribution Networks (WDNs) to couple pressure regulation and small-scale hydropower generation. The selection of PATs in WDNs needs proper knowledge about both the performances of machines available in the market and the operating conditions of the network. In this paper, a procedure for the preliminary selection of a PAT is proposed, based on the design of the main parameters (the head drop and the produced power at the Best Efficiency Point, the impeller diameter and the rotational speed) to both maximize the producible power and regulate the exceeding pressure.

1. Introduction

In the last years, the use of micro-turbines and/or Pumps As Turbines (PATs) in Water Distribution Networks (WDNs), instead of Pressure Reducing Valves (PRVs), is attracting significant attention, because they allow both pressure regulation and small-scale hydropower generation.

The installation of PATs is an effective alternative to micro-turbines, since can combine high efficiency, low investment and maintenance costs, ease of installation and spare-parts procurement [1]. Conversely, one of the main issues arising from the use of PATs for power generation is the lack of knowledge about their characteristic curves, only rarely provided by manufactures.

In the literature, several models were proposed to predict the PAT performances; many of them were devoted to predict the characteristics at the Best Efficiency Point (BEP), as a function of the performances at BEP in pump mode, through one-dimensional formulations, as summarized in [2]. The Affinity Laws [3] are widely applied to reproduce the performances of turbo-machines operating in similitude, as well. However, their reliability results not highly effective for several pump models running as turbines, such as semi-axial and submersible models [4]. Further approaches were devoted to predict the characteristic curves of PATs by means of laboratory experiments. Among them, Derakhshan and Nourbakhsh [5] proposed second-order and third-order polynomial equations to estimate the head drop curve and the power curve, respectively for single-stage horizontal axis centrifugal PATs. In both cases, equations were given in dimensionless terms with respect to the BEP. The reliability of such curves was limited to specific speeds in direct operation N_sp up to 60 and flow rate numbers φ up to 0.40, where:

$$N_s=60N{Q}^{1/2}/H^{3/4}$$

(1)

$$\varphi =Q/N{D}^3$$

(2)

with N the rotational speed (rps), Q the flow rate (m³/s), H the head (m) and D the impeller diameter (m). The subscript p refers to the pump mode.

By means of laboratory experiments, Pugliese et al. [2] showed the reliability of the head curve given in [5] for flow numbers up to 1.30 for both horizontal and vertical axis centrifugal PATs. Conversely, the power curve is unreliable for flow numbers greater than 0.40, thus the authors provided alternative formulations, valid again for φ up to 1.30. They also proposed alternative formulations to predict the characteristic curves of both single-stage and multi-stage vertical axis PATs [6]. Stefanizzi et al. [7] developed a predictive model of single-stage centrifugal PATs model to estimate both the flow rate and the head ratios, as a function of the specific speed in direct mode N_s. Barbarelli et al. [8] implemented a recursive procedure to predict both the flow rate and head ratios and the characteristic curves of centrifugal PATs.

Despite the contributions available in the literature, the optimal selection of a PAT in WDNs is still a complex issue, due to the variable operating conditions of the system. Fontana et al. [9] applied a genetic algorithm to assess the energy recoverable by PATs in a district of the Naples (IT) WDN, observing attractive profits and short capital payback periods. Similar approach was considered by [10], aimed at installing PATs in the WDN proposed by [11]. In [12], the PAT reliability in the Kozani (GR) Municipality was tested, as a complementary practice to the District Metered Areas (DMAs) sectorization, resulting the application effective when the energy consumption was nearby the energy recovery site. Venturini et al. [13] analyzed the influence of the PAT application and selection in WDNs, whereas Fecarotta and McNabola [14] tested the effectiveness of an original optimization model to a benchmark WDN, aimed at maximizing both the energy production and the economic savings related to the leakage reduction.

Moreover, for the effective PATs regulation in WDNs, the activation of Hydraulic Regulation (HR) and/or Electrical Regulation (ER) could be considered, to extend their flexibility to the variable hydraulic conditions of the network. In [15] the Variable Operative Strategy (VOS) was proposed to provide the optimal selection of a PAT in WDNs, among a set of available models, able to maximize the overall plant efficiency, under the hypothesis of HR. The VOS was also applied to the case of ER [16], as well. Nevertheless, models available in the literature are mainly based on the use of either huge time-consuming simulation models or recursive and trial-and-errors procedures, in some cases requiring specific setting parameters referring to geometric and technical properties of the selected PAT model.

Aimed at design the main characteristic parameters for the optimal PAT selection in WDNs, in this paper a simple and effective procedure is proposed, to both maximize the producible power and energy and perform the pressure regulation, in compliance with the hydraulic and technical constraints of the system.

2. Materials and Methods

A procedure for the optimal selection of centrifugal PATs in WDNs is proposed, aimed at the design of the PAT characteristic parameters, namely the flow rate and the head drop at BEP Q_tb and H_tb (where the subscripts t and b refer to the turbine mode and the BEP, respectively), the impeller diameter D and the rotational speed N. The model was developed under the hypothesis of performing the ER by varying the electrical frequency f (Hz), so as to modulate the PAT rotational speed N at any operation in the range [N_min; N_max].

The first step allows to design Q_tb and H_tb, as a function of the maximum flow rate Q_t,max. To both maximize the producible power P_t,max and exploit the whole available head drop H_{t,av_max}, second and third order polynomial functions are applied to reproduce the H_t(Q_t) and P_t(Q_t) curves, respectively, in compliance with [2,5]. Experimental data from [2] are considered, by refining the parameters estimation for φ ≤ 0.30, aimed at improving the interpolation around the BEP, which was found at φ = 0.18 from experiments. Equation (3) is derived to represent the H_t curve, setting the equivalence between the head drop H_t and the available head H_{t,av_max} when Q_t,max flows:

$$H_{t,av\_max}/H_{tb}=0.950{\left(Q_{t,max}/Q_{tb}\right)}^2-0.338\left(Q_{t,max}/Q_{tb}\right)+0.388$$

(3)

The power curve from [2] is also considered to calculate the produced power P_t,max for Q_t,max:

$$P_{t,max}/P_{tb}=-0.012{\left(Q_{t,max}/Q_{tb}\right)}^3+1.495{\left(Q_{t,max}/Q_{tb}\right)}^2-0.483\left(Q_{t,max}/Q_{tb}\right)$$

(4)

The power at BEP P_tb in Equation (4) can be calculated as:

$$P_{tb}={\eta }_{tb}\gamma Q_{tb}{H}_{tb}$$

(5)

where η_tb (-) is the PAT efficiency at BEP and γ (N/m³) the fluid specific weight. Expressing H_tb by means of Equation (3), from Equation (4) it follows:

$$P_{t,max}={\eta }_{tb}\gamma Q_{t,max}{H}_{t,av\_max}\frac{-0.012{\left(Q_{t,max}/Q_{tb}\right)}^3+1.495{\left(Q_{t,max}/Q_{tb}\right)}^2-0.483\left(Q_{t,max}/Q_{tb}\right)}{0.950{\left(Q_{t,max}/Q_{tb}\right)}^3-0.338{\left(Q_{t,max}/Q_{tb}\right)}^2+0.388\left(Q_{t,max}/Q_{tb}\right)}$$

(6)

The maximum produced power P_t,max at varying Q_t,max/Q_tb is estimated as:

$$\frac{\partial P_{t,max}}{\partial \left(Q_{t,max}/Q_{tb}\right)}=0\Rightarrow Q_{t,max}/Q_{tb}=0.951$$

(7)

Once calculated Q_tb from Equation (7), Equation (3) can be used to calculate the head drop at BEP H_tb. From Equation (7) it is observed that the power maximization is achieved at flow rate ratios Q_t,max/Q_tb close to 1. Thus, from Equation (3) to optimize the exploitation of the available head, the head at BEP H_tb should be approximately equal to H_{t,av_max}.

The specific speed in turbine mode N_st and the specific diameter D_st are set equal to 29.39 and 2.52, respectively in order to design machines with efficiency η_tb of the order of 80% [17]:

$$N_{st}=60N{Q}_{tb}^{1/2}/H_{tb}^{3/4}=29.39$$

(8)

$$D_{st}=D{H}_{tb}^{1/4}/Q_{tb}^{1/2}=2.52$$

(9)

By combining Equations (8) and (9), the flow rate number at BEP φ_b can be derived, according to the following Equation (10):

$${\varphi }_b=0.128$$

(10)

By applying an optimization procedure validated with numerical and laboratory experiments, Fontana et al. [18] observed that the maximum producible energy can be achieved by setting φ = 0.185, corresponding to Q_t,max/Q_tb ratio equal to 1.450.

From Equations (8) and (9), the rotational speed N and the impeller diameter D able to both maximize P_t,max and exploit the available head H_{t,av_max} can be calculated, as a function of Q_t,max.

Finally, by applying one of the one-dimensional flow rate and head ratios models available in the literature (e.g., the Yang et al. [19]), the flow rate and the head at BEP in pump mode Q_pb and H_pb can be assessed, so as to identify the most effective PAT, among those commercially available, with impeller diameter D.

In case P_t,max achieves a value of N higher than the upper limit N_max, then N_max can be set to design the impeller diameter D. In this case, as a function of the flow rate Q_t,max and the available head H_{t,av_max}, it is possible to choose whether:

• to maximize the producible power P_t,max, by calculating H_tb from Equation (8) as a function of Q_tb derived from Equation (7);
• to exploit the available head (e.g., H_t,exp = H_{t,av_max}), by calculating Q_tb and H_tb from Equations (3) and (8) and then the producible power P_t,max with Equation (4).

After the PAT has been selected, the operation in the other conditions should be analysed, e.g., in case of daily demand pattern for a WDN. At any flow rate, N can vary in the range [N_min; N_max], choosing either to exploit the whole available head H_t,av or to maximize the produced power P_t. The value of N able to maximize P_t in Equation (4) is calculated, by combining Equations (2) and (11). Equation (12) expresses P_tb as a function of the power number π_b at the BEP:

$${\pi }_b=P_t{}_b/\rho N^3{D}^5$$

(11)

where ρ (kg/m³) is the fluid density, resulting:

$$P_t={\pi }_b\rho N^3{D}^5\left[-0.012{\left(\frac{Q_t}{{\varphi }_{b}N{D}^3}\right)}^3+1.495{\left(\frac{Q_t}{{\varphi }_{b}N{D}^3}\right)}^2-0.483\left(\frac{Q_t}{{\varphi }_{b}N{D}^3}\right)\right]\Rightarrow \frac{\partial P_t}{\partial N}=0\Rightarrow N=1.549\frac{Q_t}{{\varphi }_b{D}^3}$$

(12)

The flow rate number φ_b and the head number ψ_b at BEP can be also calculated using Equation (2) and the following Equation (13), respectively:

$${\psi }_b=g{H}_{tb}/N^2{D}^2$$

(13)

with g (m/s²) the acceleration of gravity. Being φ_b, ψ_b and π_b constant at varying the rotational speed N [2], their calculation is useful to estimate the flow rate Q_tb, the head drop H_tb and the power P_tb at BEP at different N, respectively.

By applying the proposed procedure, the main parameters of PATs can be designed, so as to assess the overall producible energy and the corresponding payback periods.

Similar approach can also be applied to WDNs without ER, in which the PAT runs at constant rotational speed. In this latter case, Equations (3) and (7) can be applied either to exploit the available head drop or to maximize the produced power, respectively.

3. Results and Discussions

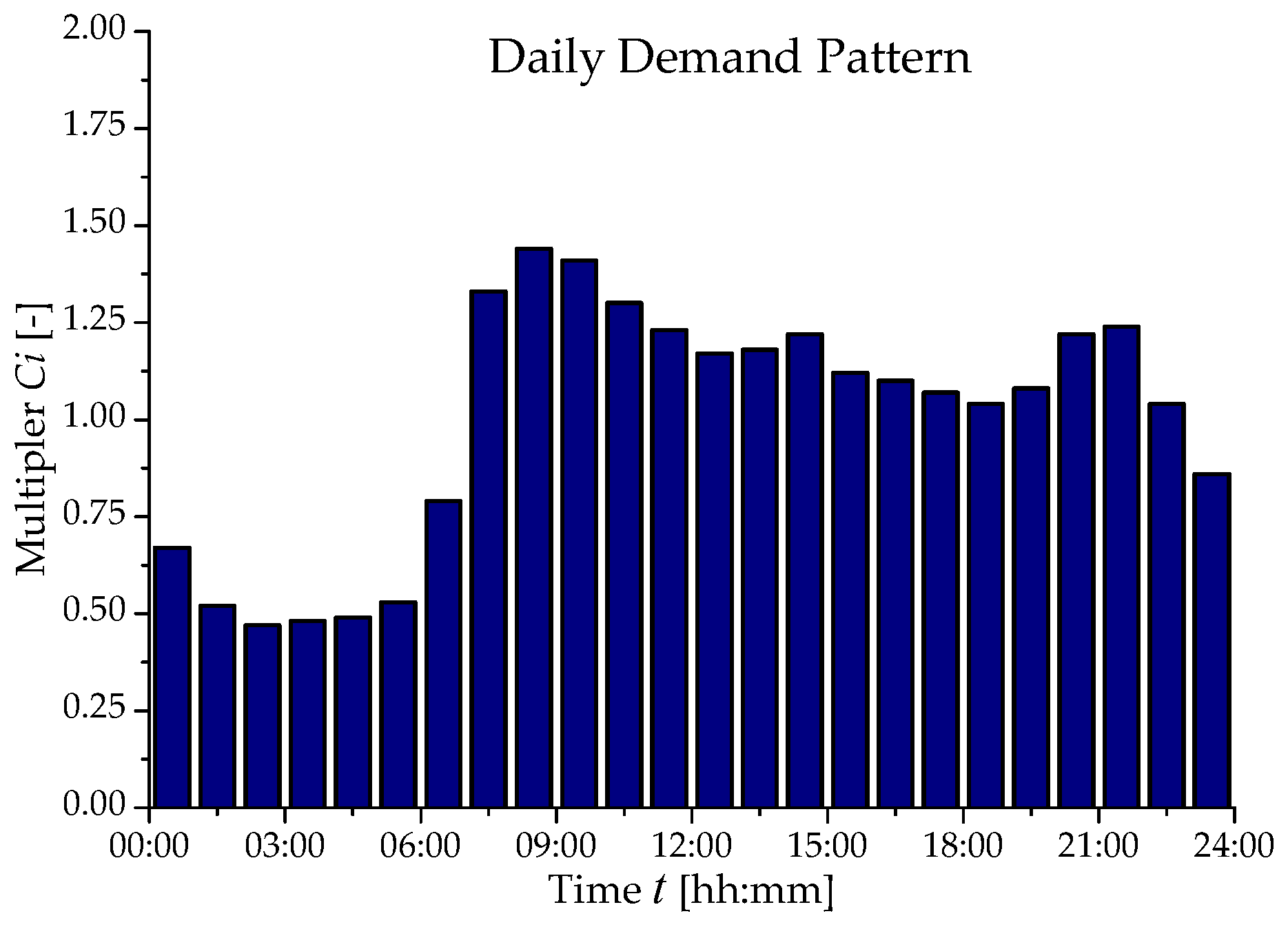
The procedure proposed above was applied to a WDN serving 20,000 inhabitants, with average flow rate Q_tm = 57.9 L/s and maximum flow rate Q_t,max = 83.3 L/s. The daily demand pattern provided by [9] and plotted in Figure 1 was considered. Two Scenarios were simulated, at varying the available head as summarized in

Table 1. PAT design parameters as a function of Q_t,max/Q_tb ratio—Scenario 1.

Qtmax/Qtb (-)	Flow Rate at BEP Qtb (L/s)	Head Drop at BEP Htb (m)	Impeller Diameter D (m)	Rotational Speed at Qt,max N (rps)	Power Range Pt (kW)	Daily Energy Ed (kWh/day)	Efficiency Range ηt (-)
0.951	87.7	19.7	0.354	15.5	0.5 ÷ 12.0	134.30	0.68 ÷ 0.80
1.450	57.5	9.6	0.343	11.2	0.5 ÷ 11.4	146.14	0.68 ÷ 0.80

. A horizontal centrifugal PAT was assumed in the example. For any scenario, a value of 0.5 kW was set as the minimum produced power. For lower produced power, the exploitation of the head drop H_t,av was assumed.

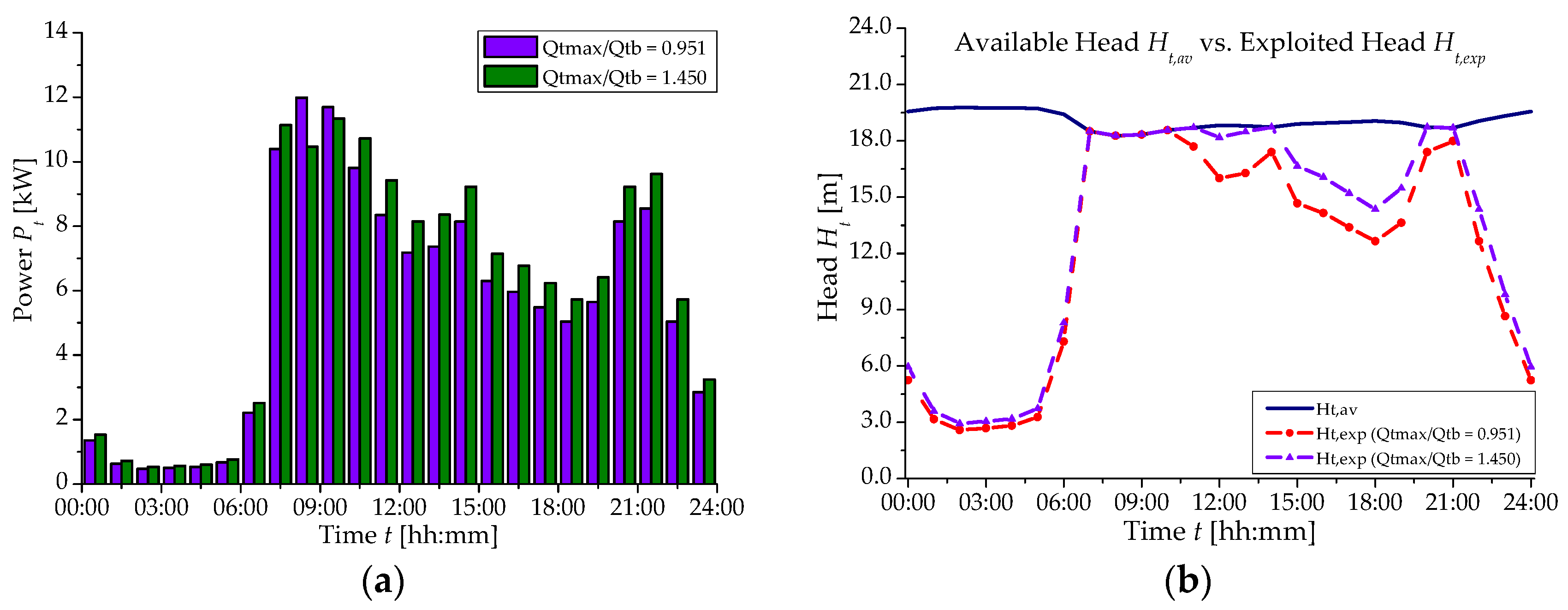
For both Scenarios, the maximum flow rate Q_tmax was equal to 83.3 L/s, whereas the available head H_t,av at Q_t,max was 18.30 m for Scenario 1 and 88.30 m for Scenario 2. In Scenario 1, the procedure was applied as per following steps, by first setting Q_tmax/Q_tb = 0.951 (Equation (7)) and then Q_tmax/Q_tb = 1.450 to maximize the produced power and the daily producible energy, respectively. Q_t,max was achieved at 08:00.

• Power Maximization at Q_tmax: Q_tmax/Q_tb = 0.951

From Equation (7) the flow rate at BEP Q_tb = 87.6 L/s was estimated as a function of Q_t,max. The head drop at BEP H_tb = 19.7 m was calculated with Equation (3). By setting N_st = 29.39 (Equation (8)) and D_st = 2.52 (Equation (9)), the rotational speed N = 15.5 rps and the impeller diameter D = 0.354 m were derived, respectively. According to Equation (10), the flow rate number at BEP φ_b = 0.128 and the power at BEP P_tb = 13.6 kW was estimated by Equation (5) as a function of the efficiency at BEP η_tb = 0.80. By applying the Equation (4), a maximum produced power at Q_t,max P_t,max = 12.0 kW was thus obtained. Being N < N_max (set equal to 50 rps) for further time-steps, the rotational speed N which maximized the producible power was derived by using Equation (12). N was assessed in the range from 7.5 to 19.5 rps. From Equations (11) and (13), the power number π_b and the head number ψ_b at BEP were defined equal to 6.44 and 0.66, respectively.

Being φ_b, π_b and ψ_b constant at varying N, from Equations (2), (11) and (13) the flow rate at BEP Q_tb, the power at BEP P_tb and the head drop at BEP H_tb were calculated, respectively, at any hourly time-step, as a function of the flowing rate Q_t and the corresponding N. From Equation (3) H_t,exp was estimated at any time-step. For time-steps with H_t,exp > H_t,av the rotational speed N which set the equivalence H_t,exp = H_t,av was thus applied. Finally, with Equation (4) the produced power P_t was assessed at any hourly time-step and the corresponding PAT efficiency η_tb was estimated with Equation (5), resulting in the range 68 ÷ 80%. The daily producible energy of 134.30 kWh/day was thus estimated.

The power maximization was considered for any time-step, because P_t was always higher than 0.5 kW. At higher flow rates (time-steps 07:00, 09:00 and 10:00) the power maximization was not feasible because achieved at rotational speed N so that H_t,exp > H_t,av. Thus, N was lowered in order to match the available head. As an example, at 09:00, N which maximized the power P_t was 22.4 rps. By applying the Equation (13), the head number ψ_b = 3.64 was calculated, corresponding to an exploited head H_t,exp = 23.3 m, against the available head H_t,av = 18.3 m. Equation (3) was thus applied by setting H_t,_exp = H_t,av to exploit the available head, resulting, combined with Equation (2), N = 16.5 rps. It corresponded to a produced power P_t = 11.70 kW and efficiency η_t = 80%. At lower flow rates, the maximum power was reached at lower N, defining a head exploitation H_t,exp lower than the available one H_t,av. The residual head was dissipated by using a PRV.

• Daily Energy Maximization: Q_tmax/Q_tb = 1.450

By applying the procedure mentioned above, results obtained by setting Q_tmax/Q_tb = 1.450 were summarized in Table 1 and compared with those from Q_tmax/Q_tb = 0.951. It was observed lower values of the design parameters (Q_tb, H_tb, D and N), resulting in a lower maximum power P_t,max = 10.5 kW, but a greater daily produced energy E_d = 146.14 kWh/day. Due to the exploitation of heads higher than the available ones, the power maximization is unfeasible at several time steps (07:00, 09:00–11:00, 14:00, 21:00–22:00); in such cases, the rotational speed N able to exploit the available head was set. At time steps 07:00, 09:00–11:00, the produced power resulted higher than that at Q_t,max, as a consequence of the higher available head against lower Q_t but, however, close to Q_t,max.

In the following Figure 2a,b the produced power P_t (Figure 2a) and the comparison between available head H_t,av and exploited head H_t,exp (Figure 2b) are plotted at any time-step.

Scenario 2: High Available Head H_t,av

• Power Maximization at Q_tmax: Q_tmax/Q_tb = 0.951

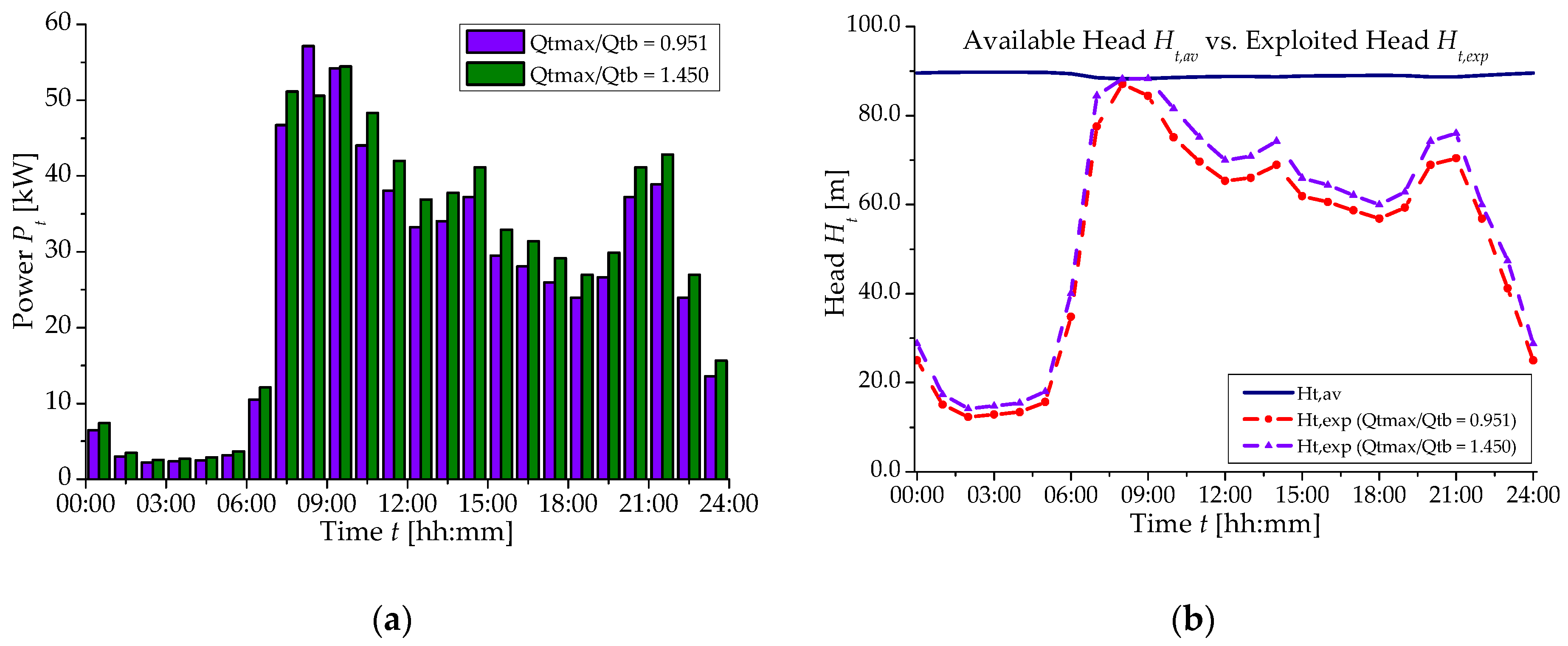
In Scenario 2, a higher available head H_t,av was considered. As introduced in Par. 3.1, Q_tb = 87.6 L/s and H_tb = 95.4 m were calculated by Equations (3) and (7), respectively. From Equation (8), the rotational speed at Q_t,max N = 50.5 rps; thus, being N > N_max, N = N_max = 50 rps was set. To maximize the produced power, the flow rate ratio Q_t,max/Q_tb = 0.951 was set obtaining H_tb = 94.1 m from Equation (8) and D = 0.240 m from Equation (9), respectively. From Equation (2) φ_b = 0.128 was thus assessed and the power at BEP P_tb = 64.72 kW was calculated by Equation (5), corresponding to the efficiency at BEP η_tb = 0.80. The maximum produced power at Q_t,max of 57.15 kW was thus derived with Equation (4). The rotational speed N able to maximize the power at any time-step was thus derived by Equation (12), resulting N > N_max from 07:00 to 23:00. For the latter, N = N_max was thus set. The power number π_b and the head number ψ_b at BEP were estimated equal to 0.66 and 6.44 with Equations (11) and (13), respectively and the flow rate at BEP Q_tb, the power at BEP P_tb and the head drop at BEP H_tb were obtained from Equations (2), (11) and (13), respectively, at any hourly time-step, as a function of the flowing rate Q_t and the corresponding N, being φ_b, π_b and ψ_b constant at varying N. From Equation (4) the produced power P_t was assessed at any hourly time-step, resulting in the range 2.22 ÷ 57.15 kW. The PAT efficiency η_t, estimated with Equation (5), ranged between 68% and 80%. The daily producible energy of 622.89 kWh/day was thus evaluated. A residual head (H_t,av–H_t,exp) was observed at each time-step. A PRV was combined with PAT to exploit the residual head. Alternatively, multi-stage PATs could be applied for both increasing the hydropower generation and exploiting the residual head.

• Daily Energy Maximization: Q_tmax/Q_tb = 1.450

By applying the abovementioned procedure setting Q_tmax/Q_tb = 1.450, results summarized in

Table 2. PAT design parameters as a function of Q_t,max/Q_tb ratio—Scenario 2.

Qtmax/Qtb (-)	Flow Rate at BEP Qtb (L/s)	Head Drop at BEP Htb (m)	Impeller Diameter D (m)	Rotational Speed at Qt,max N (rps)	Power Range Pt (kW)	Daily Energy Ed (kWh/day)	Efficiency Range ηt (-)
0.951	87.7	34.11	0.240	50.00	2.22 ÷ 57.15	622.89	0.68 ÷ 0.80
1.450	57.5	46.6	0.231	36.43	2.55 ÷ 54.46	674.17	0.68 ÷ 0.80

were achieved, compared with those from the Q_tmax/Q_tb = 0.951 setting. The designed rotational speed N at Q_tmax was 36.43 rps with Q_tb = 57.5 L/s, H_tb = 46.6 m and D = 0.231 m. The produced power at Q_tmax was lowered to 50.58 kW, however achieving higher powers at time steps 07:00 and 09:00 (Figure 3). The daily produced energy was higher than that at Q_tmax/Q_tb = 0.951, being equal to 674.17 kWh/day.

Finally, in Figure 4 comparison between the available head H_t,av and the exploited head H_t,exp is plotted at varying the flow rate Q_t for both Scenarios and Q_tmax/Q_tb ratios. In both Scenarios, Q_tmax/Q_tb = 1.450 resulted in higher H_t,exp, showing a better capability to reduce the exceeding pressure in the WDN. In Scenario 1, the designed PAT was able to exploit the whole exceeding head for flow rates higher than 67 L/s, whereas in Scenario 2, the exceeding head was exploited only at flow rates very close to Q_t,max.

4. Conclusions

A procedure for the optimal selection of PATs in WDNs was presented and applied to a WDN to assess its effectiveness at varying the available head in the network. The model was devoted to the preliminary design of PAT main parameters under the hypothesis of Electrical Regulation. Results pointed out that the analytic model is able to assess the operating conditions at BEP, the impeller diameter and the rotational speed, in order to both maximize the power (or the overall producible energy) and regulate the pressure in the network. The procedure can be applied to both single-stage and multi-stage centrifugal PATs, depending on the available head in the network.