Modelling of Environmental Parameters of a Harbor Basin in a Coastal Zone with a Seawall †
Abstract
:1. Introduction
2. Material and Methods
2.1. Laboratory Experiments
2.2. Numerical Model
3. Results and Discussion
4. Conclusions
- (1)
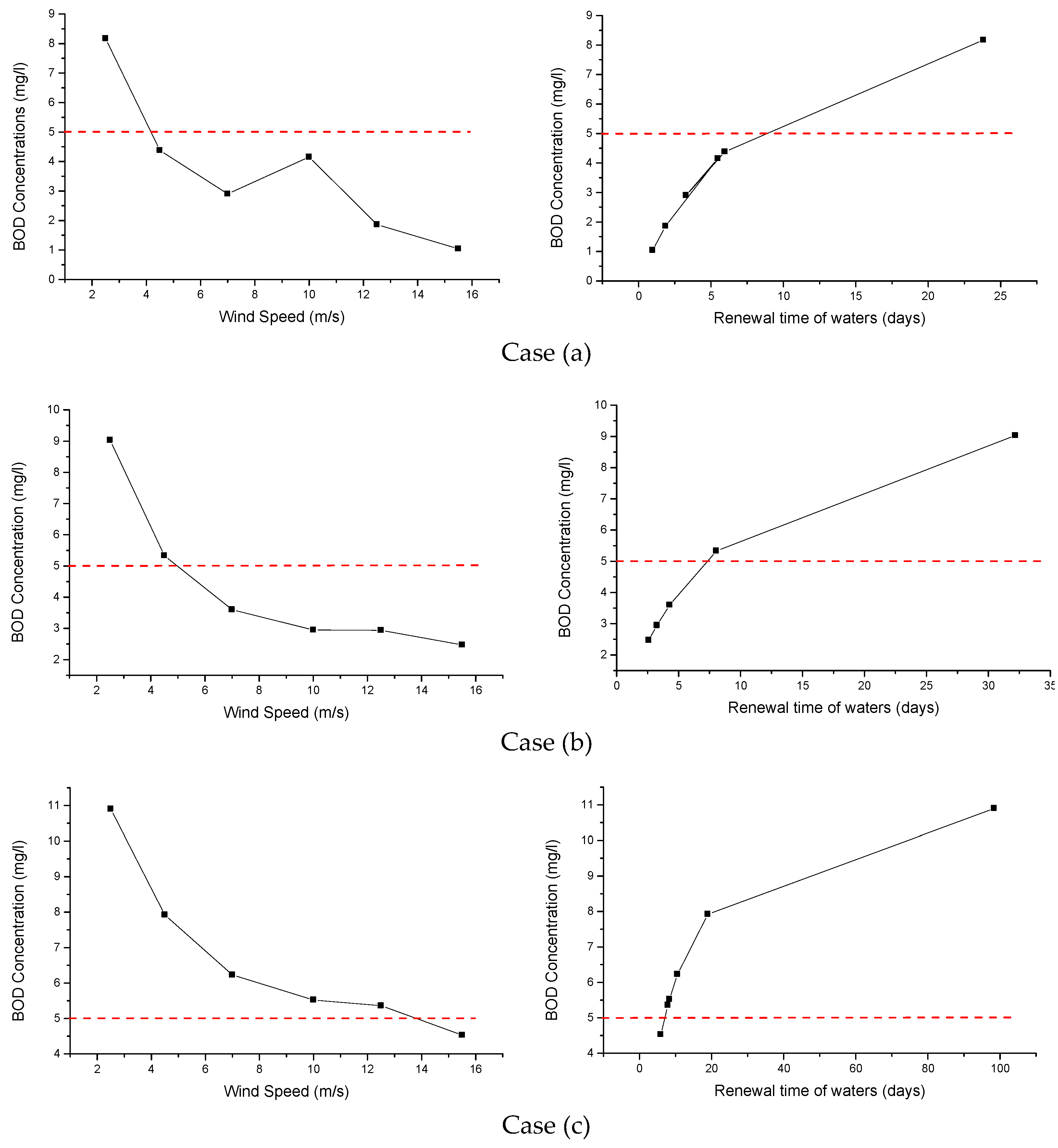
- Low wind speeds, less than 2.5 m/s (2 Beaufort), lead to unacceptable values of BOD concentration (>5 mg/L) for all the cases of different formation of the opening. However, it seems that moderate winds (4–7 m/s) ensure acceptable values of BOD concentrations apart from the case of a 90 m mole centered in the opening of the harbor. The detailed statistical analysis of the meteorological data of the study area (winds’ frequency and speed), is the key process which will finally determine the right choice between different alternative cases.
- (2)
- Large waters renewal times result, as expected, from low wind speeds and become even larger for the case of a 90 m mole in the opening; in fact, for this case, waters renewal times reach approx. 100 days for low wind speeds, becoming multiple of the ones corresponding to the case of free opening or the case of a 50 m mole centered in the opening. This result obviously leads to unpleasant and hazardous environmental situations.
- (3)
- Between the case of totally free opening and the partial closing of it with a mole of 50 m, it seems that the latter is the best possible technical solution since wave protection is also considered.
Author Contributions
References
- Pechlivanidis, G.; Savvidis, Y.; Keramaris, E.; Koutitas, C. Investigation of the optimum connection of a fish pond with the adjacent main riverine flow. Procedia Eng. 2016, 162, 349–356. [Google Scholar] [CrossRef]
- Savvidis, Y.; Keramaris, E.; Pechlivanidis, G.; Koutitas, C. Optimum design of the entrance of a fishpond laterally to the main stream of an open channel. Environ. Sci. Pollut. Res. J. 2017, 24, 20122–20133. [Google Scholar] [CrossRef]
- Savvidis, Y.; Keramaris, E. Numerical study of particle transport in a river with a lateral harbor basin. Spec. Top. Rev. Porous Media—Int. J. Porous Media J. 2017, 8, 127–135. [Google Scholar] [CrossRef]
- Adrian, R.J. Particle-Imaging techniques for experimental fluid mechanics. Annu. Rev. Fluid Mech. 1991, 23, 261–304. [Google Scholar] [CrossRef]
- Raffel, M.; Willert, C.; Wereley, S.; Kompenhans, J. Particle Image Velocimetry: A Practical Guide; Springer: Berlin, Germany, 2007. [Google Scholar]
- Smagorinsky, J. General circulation experiments with the primitive equations. I. The basic experiment. Mon. Weather Rev. 1963, 91, 99–164. [Google Scholar] [CrossRef]
- Kougolos, A. Introduction in Environmental Engineering; Tziolas Thessaloniki: Thessaloniki, Greece, 2005; p. 344. (In Greek) [Google Scholar]
- Tchobanoglous, G.; Burton, F.L. Wastewater Engineering Treatment Disposal and Reuse, 3rd ed.; McGraw-Hill: New York, NY, USA, 1991; p. 1334. [Google Scholar]
- Savvidis, Y.; Georgiadis, C.; Datsi, E. Investigating the waters’ flushing of the inner basin of Nea Moudania Harbor (Northern Greece). J. Environ. Prot. Ecol. 2009, 10, 732–742. [Google Scholar]
- Beaufort Wind Force Scale. Available online: https://www.metoffice.gov.uk/guide/weather/marine/Beaufort-scale (accessed on 10 February 2018).

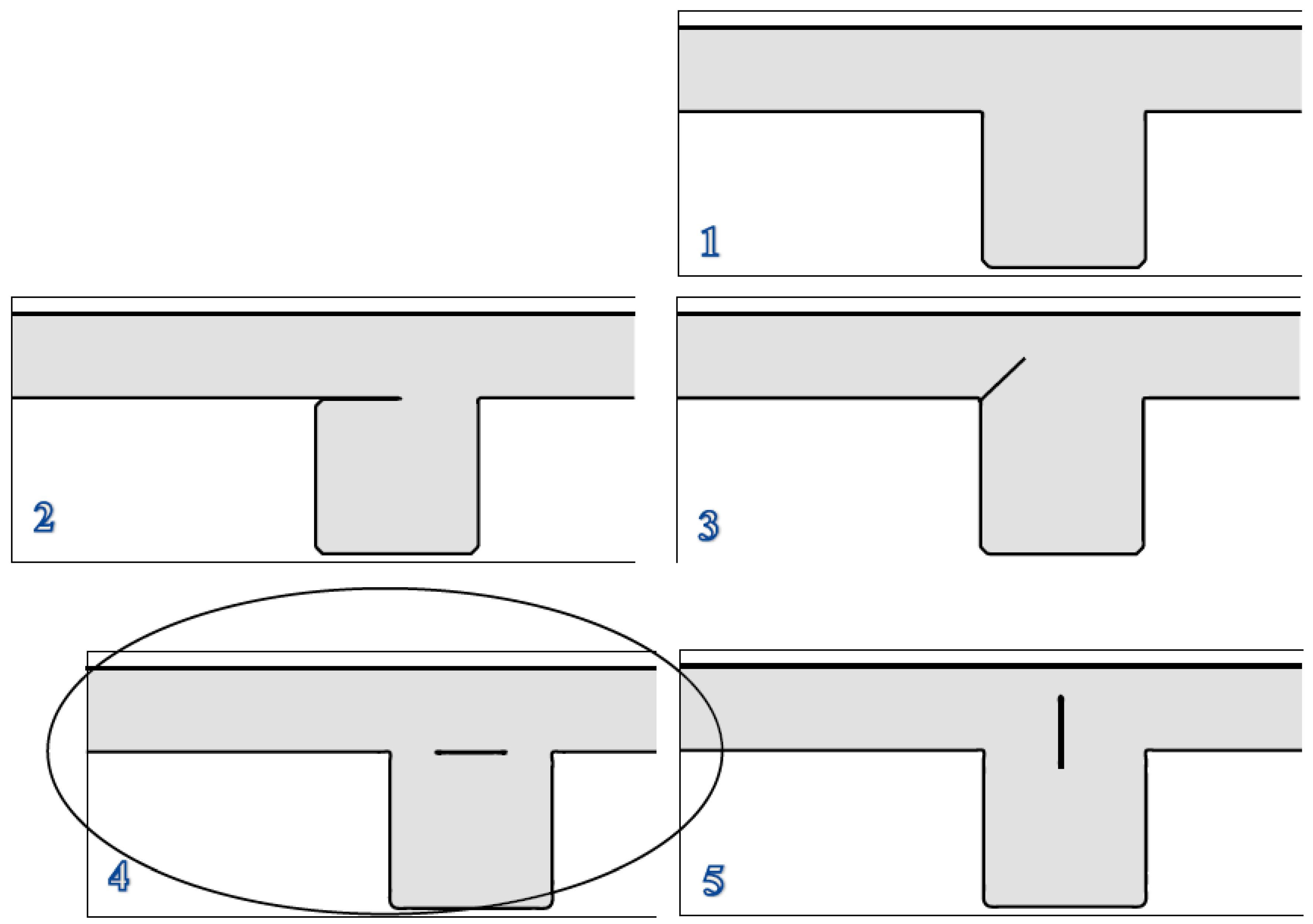
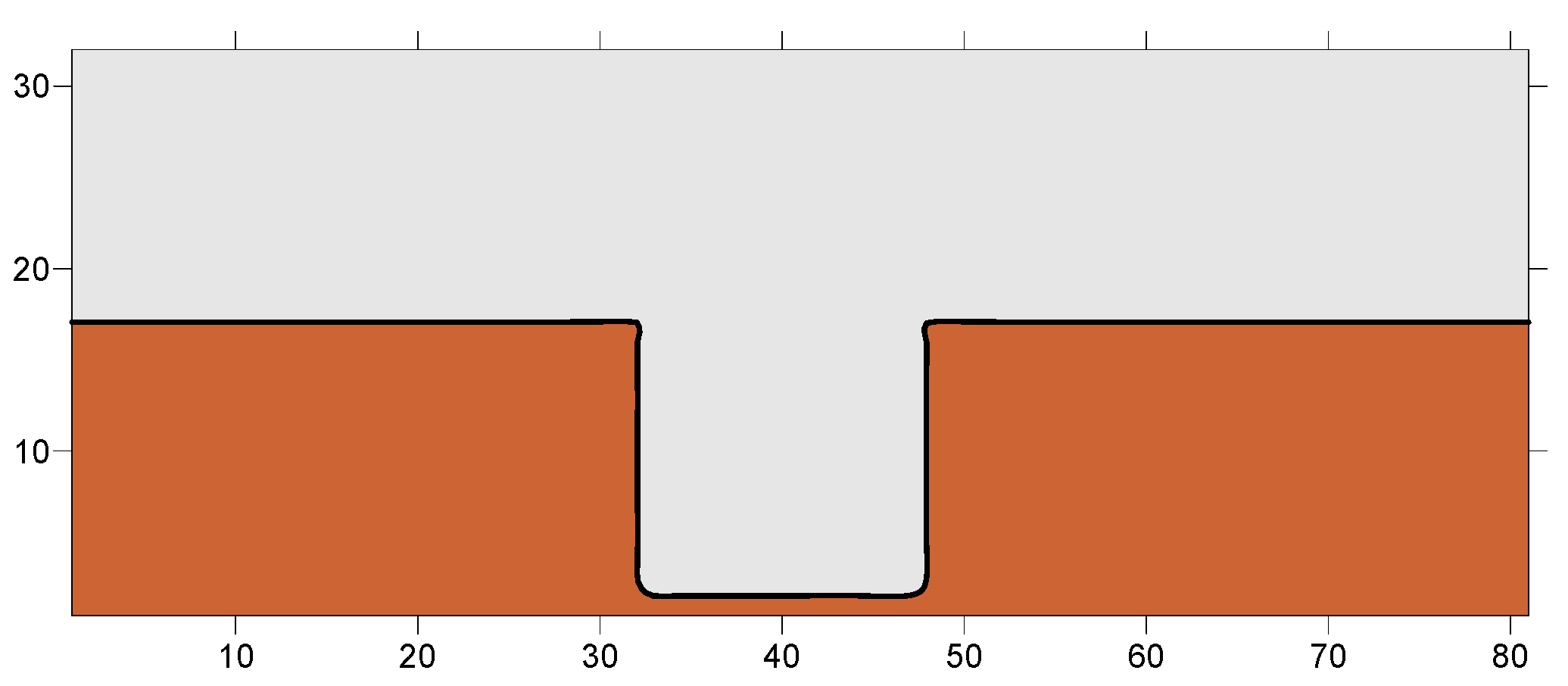


| m/s | 2.5 | 4.5 | 7 | 10 | 12.5 | 15.5 |
| Beaufort | 2 | 3 | 4 | 5 | 6 | 7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2018 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Savvidis, Y.; Keramaris, E.; Pechlivanidis, G.; Koutitas, C. Modelling of Environmental Parameters of a Harbor Basin in a Coastal Zone with a Seawall. Proceedings 2018, 2, 575. https://doi.org/10.3390/proceedings2110575
Savvidis Y, Keramaris E, Pechlivanidis G, Koutitas C. Modelling of Environmental Parameters of a Harbor Basin in a Coastal Zone with a Seawall. Proceedings. 2018; 2(11):575. https://doi.org/10.3390/proceedings2110575
Chicago/Turabian StyleSavvidis, Yiannis, Evangelos Keramaris, George Pechlivanidis, and Christopher Koutitas. 2018. "Modelling of Environmental Parameters of a Harbor Basin in a Coastal Zone with a Seawall" Proceedings 2, no. 11: 575. https://doi.org/10.3390/proceedings2110575
APA StyleSavvidis, Y., Keramaris, E., Pechlivanidis, G., & Koutitas, C. (2018). Modelling of Environmental Parameters of a Harbor Basin in a Coastal Zone with a Seawall. Proceedings, 2(11), 575. https://doi.org/10.3390/proceedings2110575





