A New Method to Determine the Annual Energy Output of Liquid-Based Solar Collectors †
Abstract
:1. Introduction
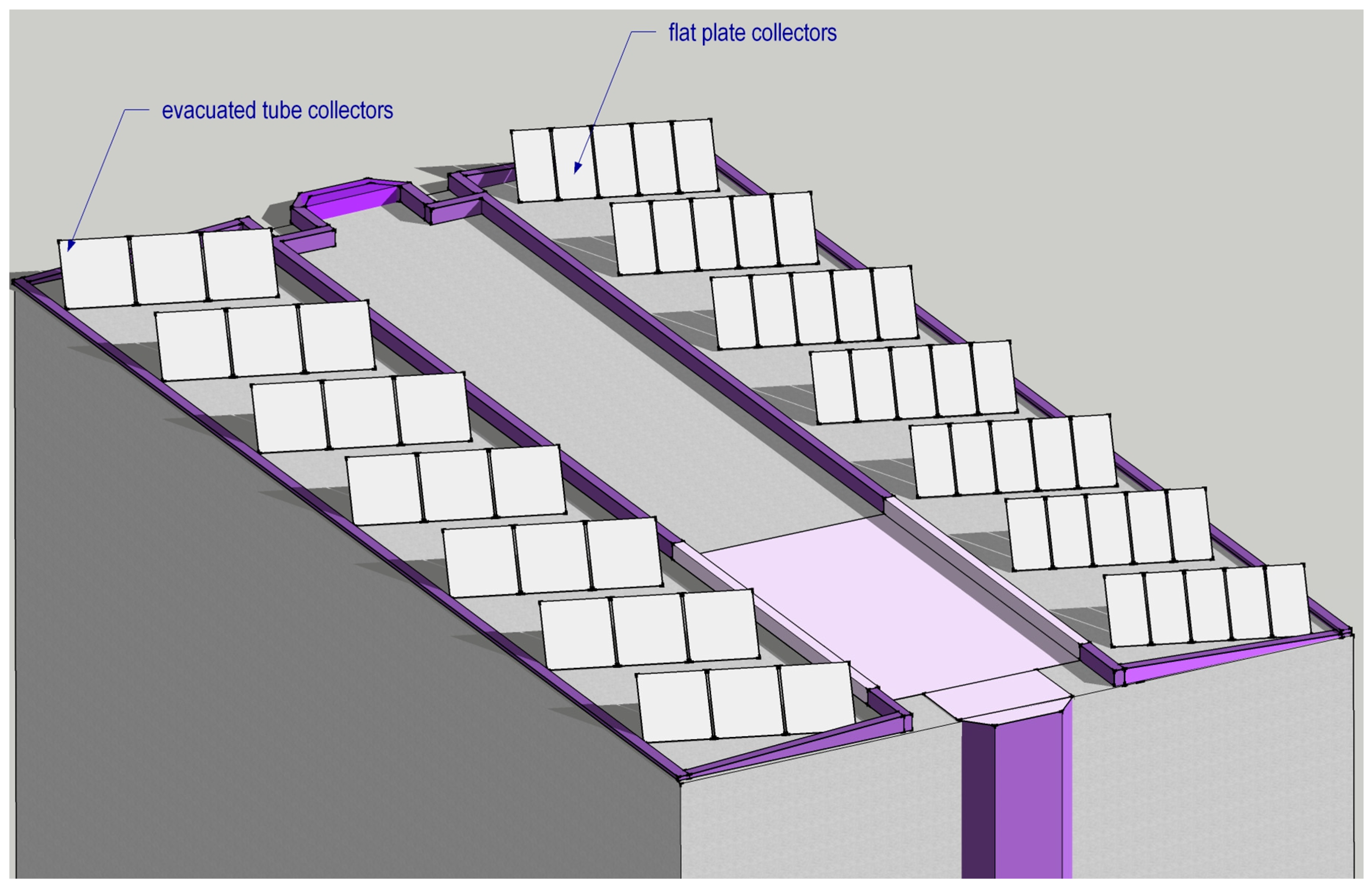
2. Description of the Method to Determine the Annual Energy Output of Solar Collectors
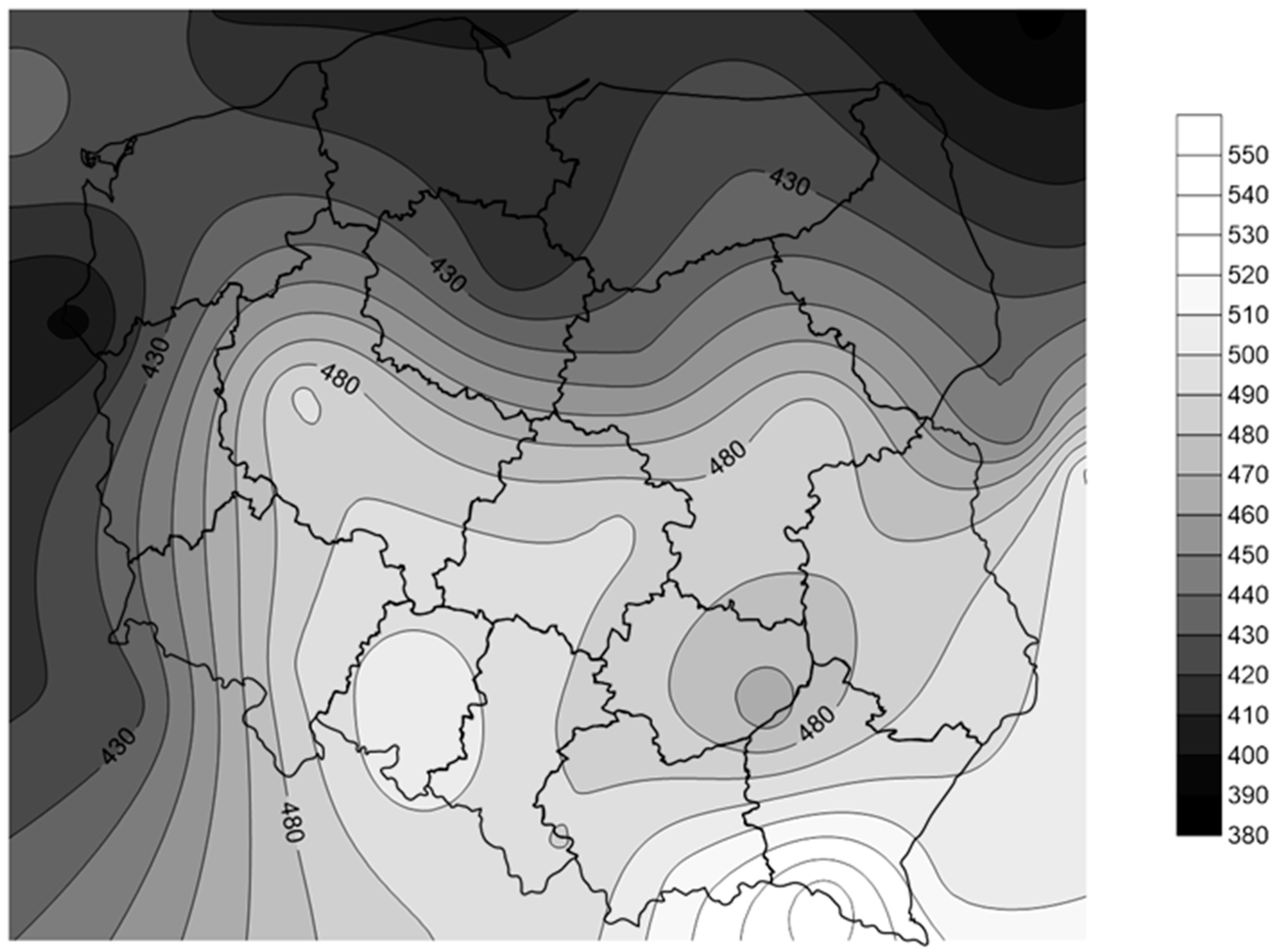
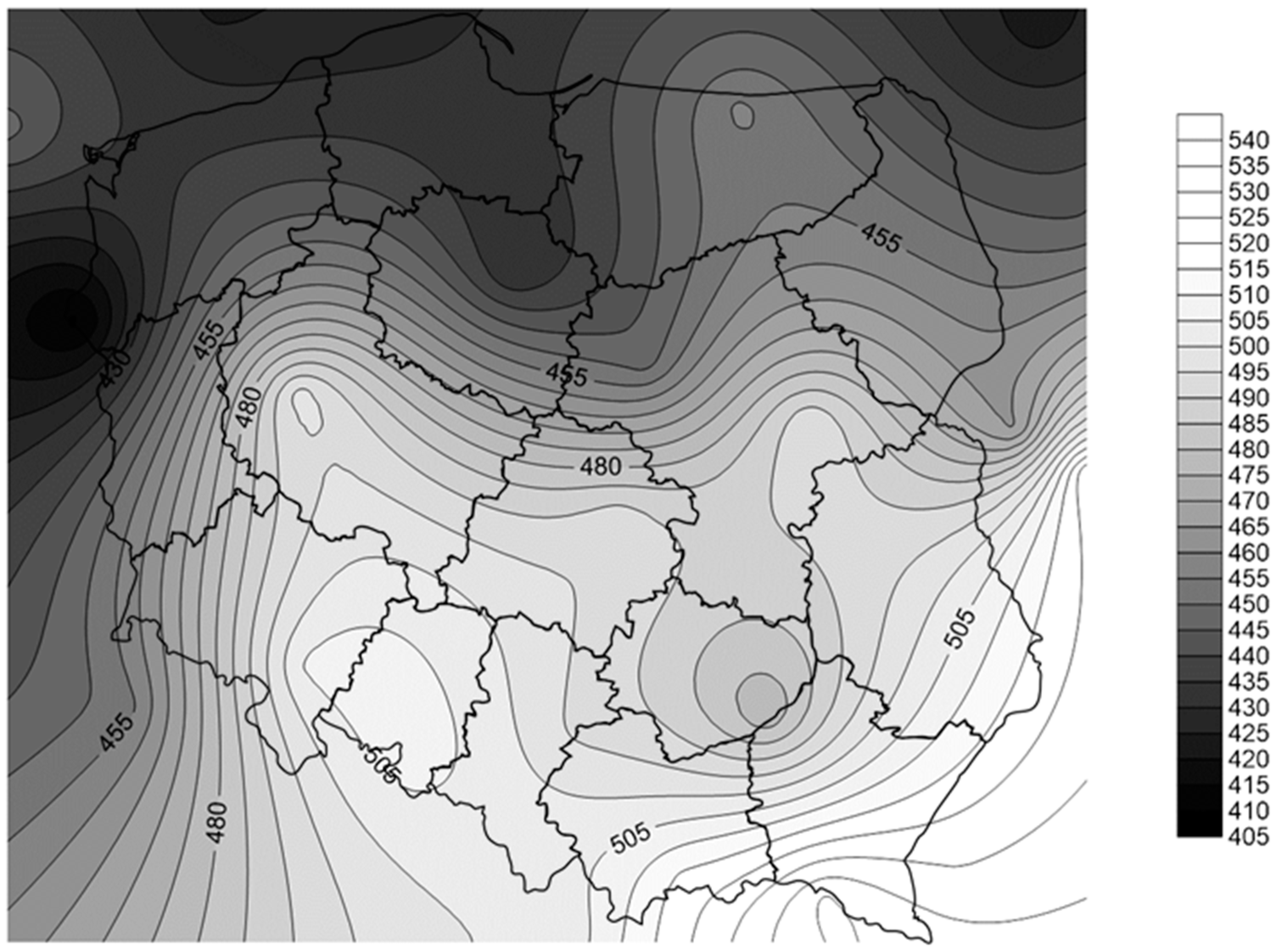
3. Results and Their Discussion
4. Summary and Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Sujith, K. Design Of Active Solar Water Heating System Using F-Chart Method. Int. J. Eng. Res. Technol. 2013, 7, 296–299. [Google Scholar]
- Gordon, J.M.; Rabl, A. Design analysis and optimization of industrial process heat plants without storage. Sol. Energy 1982, 28, 519–530. [Google Scholar] [CrossRef]
- Pareira, M.C.; Gordon, J.M.; Rabl, A.; Zarmi, Y. Design and optimization of solar industrial hot water systems with storage. Sol. Energy 1984, 32, 121–133. [Google Scholar] [CrossRef]
- Klein, S.A.; Beckman, W.A.; Duffie, J.A.A. Design Procedure For Solar Heating Systems. Sol. Energy 1976, 18, 113–126. [Google Scholar] [CrossRef]
- Haberl, J.S.; Cho, S. Literature Review of Uncertainty of Analysis Methods (F-Chart Program). Report to the Texas Commission on Environmental Quality, 2004, Energy Systems Laboratory, Texas A&M Univeristy, http://hdl.handle.net/1969.1/2069.
- Zukowski, M. HVAC systems supported by renewable energy sources - case studies carried out at the Bialystok University of Technology. In Book Advances in Renewable Energy Research; Pawłowska, M., Pawłowski, A., Pawłowski, L., Eds.; CRC Press/Taylor & Francis, 2017; pp. 33–44. [Google Scholar]
- Zukowski, M. Energy efficiency of a solar domestic hot water system. Proceedings of the International Conference on Advances in Energy Systems and Environmental Engineering; ASEE17; Wroclaw; 2017. Vol. 22, E3S Web of Conferences e-ISSN 2267-1242. [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zukowski, M.; Radzajewska, P. A New Method to Determine the Annual Energy Output of Liquid-Based Solar Collectors. Proceedings 2019, 16, 39. https://doi.org/10.3390/proceedings2019016039
Zukowski M, Radzajewska P. A New Method to Determine the Annual Energy Output of Liquid-Based Solar Collectors. Proceedings. 2019; 16(1):39. https://doi.org/10.3390/proceedings2019016039
Chicago/Turabian StyleZukowski, Miroslaw, and Paulina Radzajewska. 2019. "A New Method to Determine the Annual Energy Output of Liquid-Based Solar Collectors" Proceedings 16, no. 1: 39. https://doi.org/10.3390/proceedings2019016039
APA StyleZukowski, M., & Radzajewska, P. (2019). A New Method to Determine the Annual Energy Output of Liquid-Based Solar Collectors. Proceedings, 16(1), 39. https://doi.org/10.3390/proceedings2019016039




