Multimode Cavity Optomechanics †
Abstract
1. Introduction
2. Results
2.1. Theory
2.2. Estimation of the Optomechanical Coupling Strength
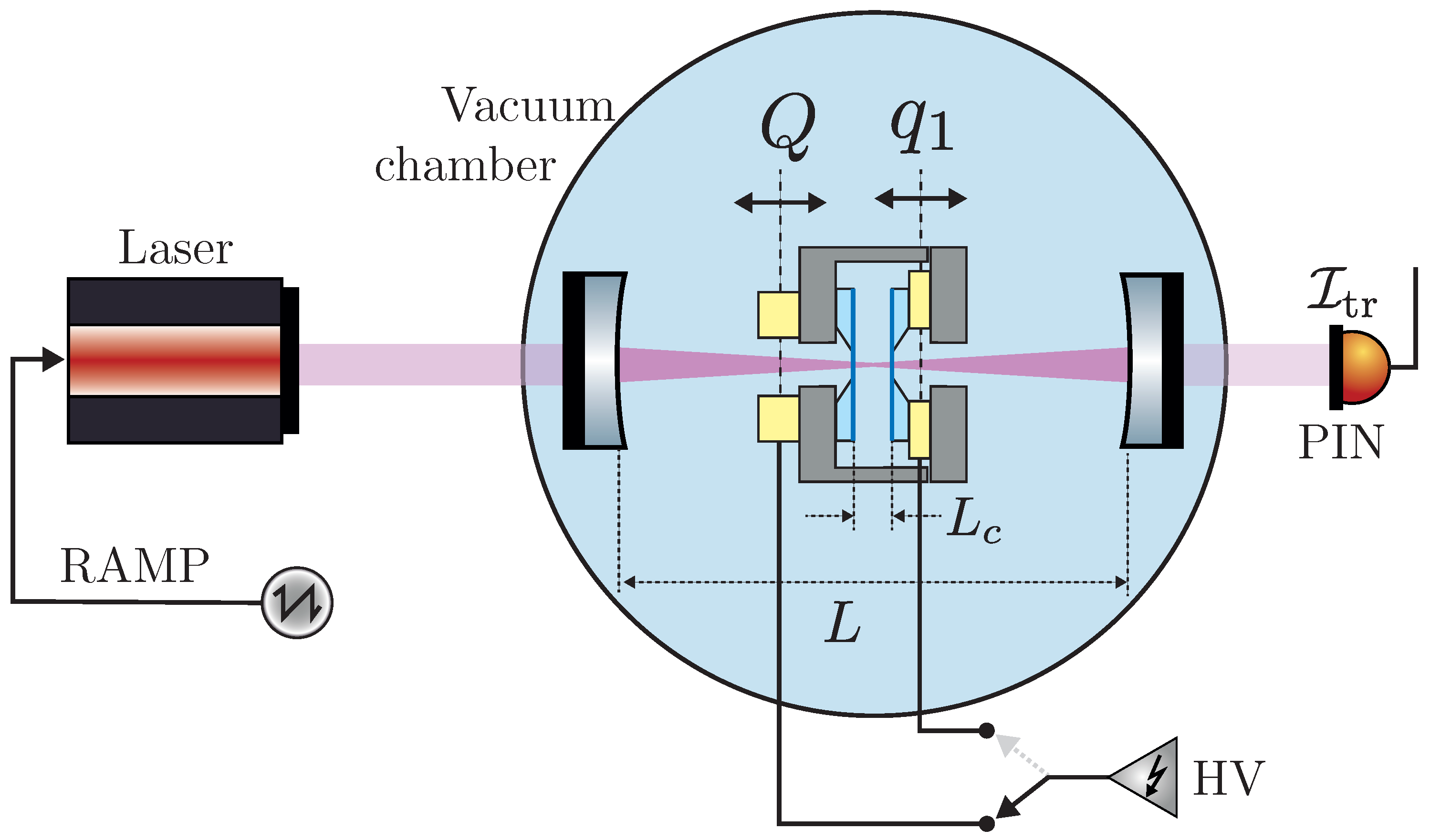
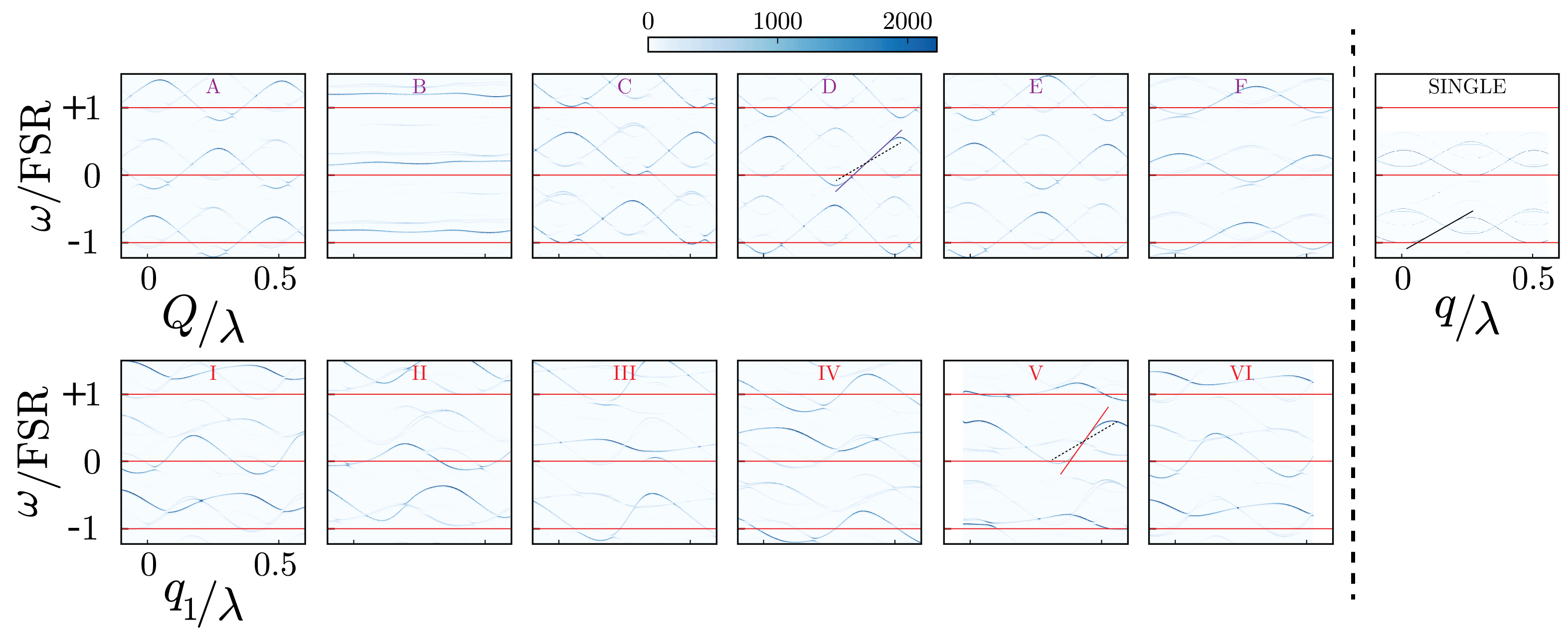
3. Conclusions
Acknowledgments
References
- Bhattacharya, M.; Meystre, P. Multiple membrane cavity optomechanics. Phys. Rev. A 2008, 78, 041801. [Google Scholar] [CrossRef]
- Hartmann, M.J.; Plenio, M.B. Steady State Entanglement in the Mechanical Vibrations of Two Dielectric Membranes. Phys. Rev. Lett. 2008, 101, 200503. [Google Scholar] [CrossRef]
- Xuereb, A.; Genes, C.; Dantan, A. Strong Coupling and Long-Range Collective Interactions in Optomechanical Arrays. Phys. Rev. Lett. 2012, 109, 223601. [Google Scholar] [CrossRef] [PubMed]
- Xuereb, A.; Genes, C.; Dantan, A. Collectively enhanced optomechanical coupling in periodic arrays of scatterers. Phys. Rev. A 2013, 88, 053803. [Google Scholar] [CrossRef]
- Li, J.; Xuereb, A.; Malossi, N.; Vitali, D. Cavity mode frequencies and strong optomechanical coupling in two-membrane cavity optomechanics. J. Opt. 2016, 18, 084001. [Google Scholar] [CrossRef]
- Nair, B.; Xuereb, A.; Dantan, A. Cavity optomechanics with arrays of thick dielectric membranes. Phys. Rev. A 2016, 94, 053812. [Google Scholar] [CrossRef]
- Li, J.; Li, G.; Zippilli, S.; Vitali, D.; Zhang, T. Enhanced entanglement of two different mechanical resonators via coherent feedback. Phys. Rev. A 2017, 95, 043819. [Google Scholar] [CrossRef]
- Piergentili, P.; Catalini, L.; Bawaj, M.; Zippilli, S.; Malossi, N.; Natali, R.; Vitali, D.; Di Giuseppe, G. Two-membrane cavity optomechanics. New J. Phys. 2018, 20, 101001. [Google Scholar] [CrossRef]
- Ludwig, M.; Marquardt, F. Quantum Many-Body Dynamics in Optomechanical Arrays. Phys. Rev. Lett. 2013, 111, 073603. [Google Scholar] [CrossRef] [PubMed]
- Weaver, M.J.; Buters, F.; Luna, F.; Eerkens, H.; Heeck, K.; de Man, S.; Bouwmeester, D. Coherent optomechanical state transfer between disparate mechanical resonators. Nat. Commun. 2017, 8, 824. [Google Scholar] [CrossRef] [PubMed]
- Rossi, M.; Kralj, N.; Zippilli, S.; Natali, R.; Borrielli, A.; Pandraud, G.; Serra, E.; Di Giuseppe, G.; Vitali, D. Enhancing Sideband Cooling by Feedback-Controlled Light. Phys. Rev. Lett. 2017, 119, 123603. [Google Scholar] [CrossRef] [PubMed]
- Kralj, N.; Rossi, M.; Zippilli, S.; Natali, R.; Borrielli, A.; Pandraud, G.; Serra, E.; Giuseppe, G.D.; Vitali, D. Enhancement of three-mode optomechanical interaction by feedback-controlled light. Quantum Sci. Technol. 2017, 2, 034014. [Google Scholar] [CrossRef]
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Piergentili, P.; Catalini, L.; Bawaj, M.; Zippili, S.; Malossi, N.; Natali, R.; Vitali, D.; Giuseppe, G.D. Multimode Cavity Optomechanics. Proceedings 2019, 12, 54. https://doi.org/10.3390/proceedings2019012054
Piergentili P, Catalini L, Bawaj M, Zippili S, Malossi N, Natali R, Vitali D, Giuseppe GD. Multimode Cavity Optomechanics. Proceedings. 2019; 12(1):54. https://doi.org/10.3390/proceedings2019012054
Chicago/Turabian StylePiergentili, Paolo, Letizia Catalini, Mateusz Bawaj, Stefano Zippili, Nicola Malossi, Riccardo Natali, David Vitali, and Giovanni Di Giuseppe. 2019. "Multimode Cavity Optomechanics" Proceedings 12, no. 1: 54. https://doi.org/10.3390/proceedings2019012054
APA StylePiergentili, P., Catalini, L., Bawaj, M., Zippili, S., Malossi, N., Natali, R., Vitali, D., & Giuseppe, G. D. (2019). Multimode Cavity Optomechanics. Proceedings, 12(1), 54. https://doi.org/10.3390/proceedings2019012054




