Ferromagnetic Josephson Junctions for High Performance Computation ^†

Abstract

Josephson junctions drive the operation of superconducting qubits and they are the key for the coupling and the interfacing of superconducting qubit components with other quantum platforms. They are the only means to introduce non linearity in a superconducting circuit and offer direct solutions to tune the properties of a superconducting qubit, thus enlarging the possible qubit layouts. Junctions performances and tunability can take advantage of using a large variety of barriers and their special functionalities. We mention pertinent results on the advances in understanding the properties of ferromagnetic junctions, which make possible the use of these devices either as memory elements and as core circuit elements.

1. Introduction

The control of a Josephson junction (JJ) is meant to be more and more important for the development of novel concepts and layouts of superconducting elements for a wide range of applications, ranging from digital circuits to different types of superconducting qubits. If on one hand Al junctions technology needs to do further steps to guarantee a huge number of junctions with required yield and reproducibility for further advances in the realization of a quantum computer, on the other hand it is reasonable to expect that hybrid solutions may offer some alternatives in the realization of complex quantum systems. For hybrid we mean either junctions employing barriers other than common oxides such as AlO${}_x$, or physical systems which can incorporate various quantum systems in the same architecture. This hybrid perspective is the motivation behind the integration of a transmon in a cavity and a spin ensemble [1], or the realization of a gatemon [2,3]. An hybrid system for novel functionalities wants to take advantage of the various quantum components, while a hybrid junction wants to use more efficient knobs and controls, and this is not always possible in traditional Al and Nb technologies. This is operatively possible because some of these circuits, as for instance the transmon, do not necessarily require to use junctions with very high quality factors.

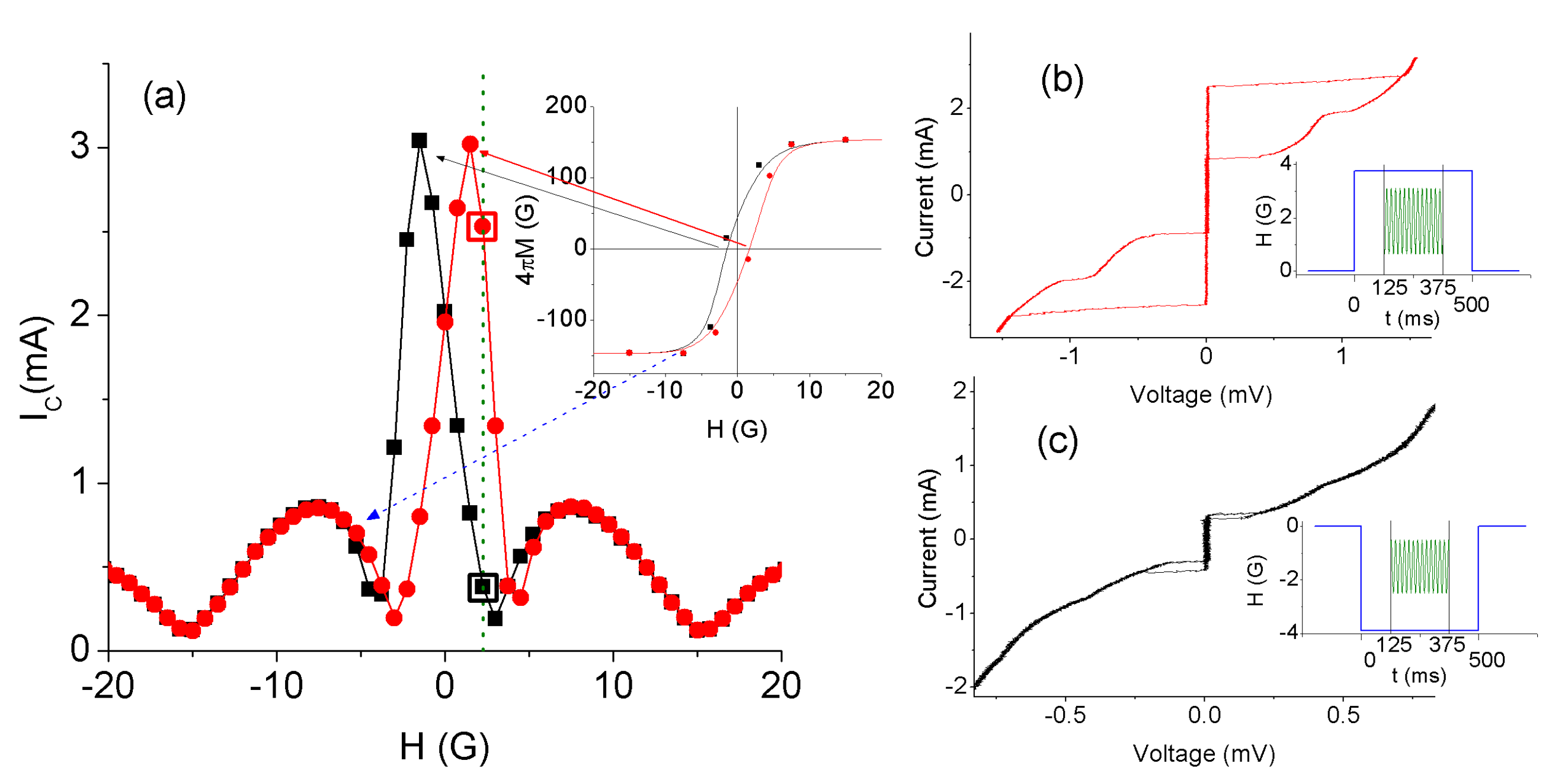
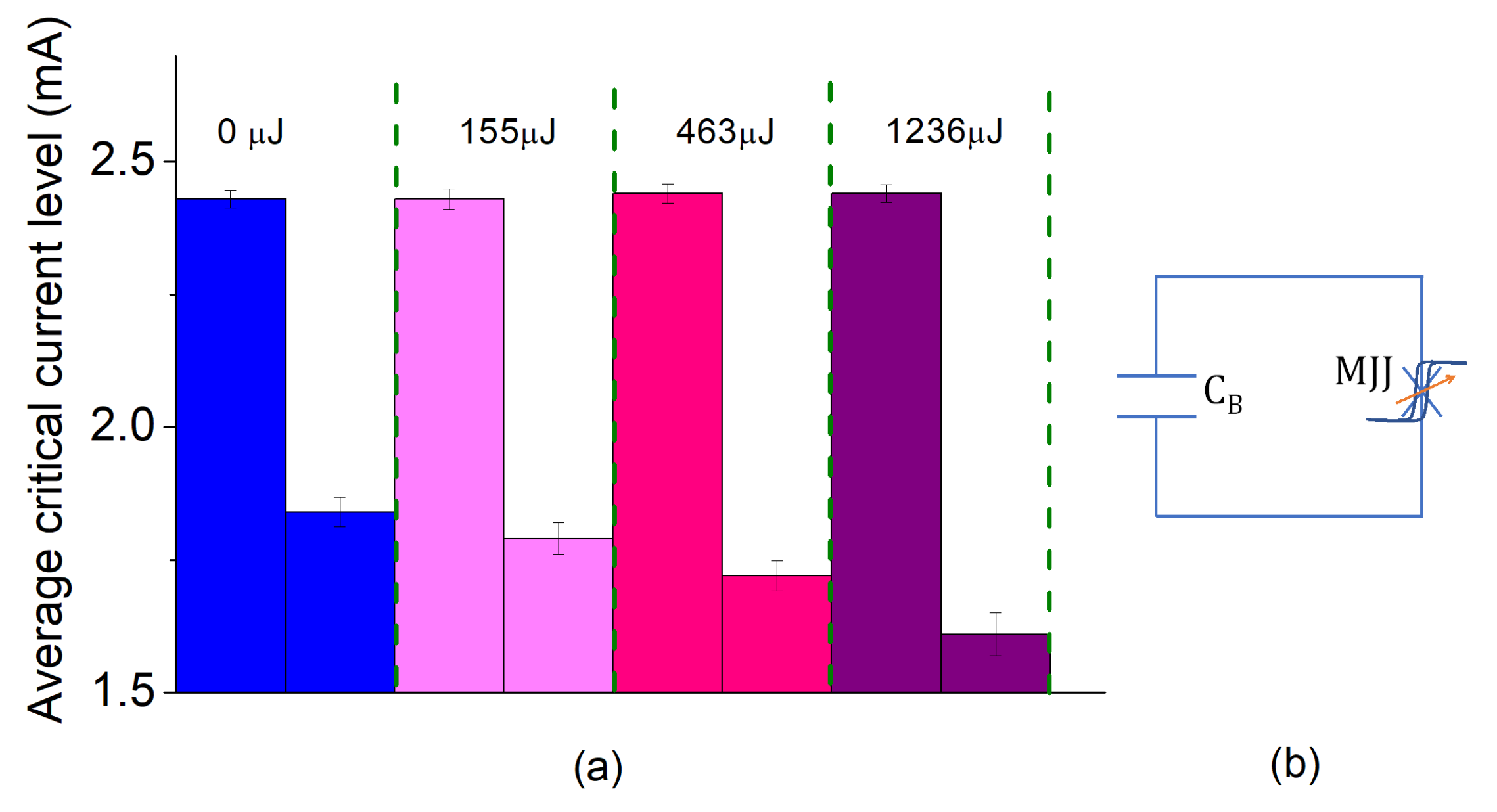
Keeping in mind that a ’different’ junction can offer novel functionalities or is more easily prone to be controlled by alternative and possibly less noisy knobs, or to be coupled to other platforms, we will describe some interesting properties of ferromagnetic SFS (Superconductor-Ferromagnet-Superconductor) junctions which pave the way to their use in real quantum circuits. In addition to the well established notion of using SFS junctions as energy-efficient Random Access Memories (RAM) to be employed for hybrid quantum/classic high performance computation, we report on the demonstration of how an RF train can change the state of the memory suggesting an RF drive for SFS circuits in some specific conditions. The notions developed for these types of junctions could be in the long run used as a new tool to control the state of a transmon, more easily connected to a qubit control driven by Single Flux Quantum (SFQ) logic [4].

2. Memory Properties of Ferromagnetic Junctions

In the last few years, there have been several approaches to the use of the memory properties of ferromagnetic JJs. Most recent achievements employ spin valves [5] or pseudo-spin valves [6] as junction barriers to control the phase or the critical current magnitude switch between two well-defined states. Another interesting approach uses $\phi$-junctions as memory elements, defining the two logic states as the escape from the two different wells of the degenerate washboard potential, which is characteristic for such devices [7].

The possibility to use ferromagnetic JJs as RAMs was demonstrated by Bol’ginov et al. [8]. In these Nb-PdFe-Nb trilayers, the two classical logic states correspond to two different critical current levels due to the hysteretic behavior of ferromagnetic materials. Such materials have a multivalued dependence of the magnetization M upon the external magnetic field H, which is reflected on the critical current dependence of the junction on the external magnetic field (Figure 1a). The use of positive and negative external magnetic field pulses allows to switch between the two critical current states (Figure 1b,c). The readout is performed by applying a reading current $I_{read}$ such that the condition $I_1<I_{read}<I_2$ is satisfied, where $I_1$ and $I_2$ are the two critical current levels, and by measuring the voltage across the junction. If the device is in the state with $I_C=I_1$, then the output is a finite voltage, while if it is in the state with $I_C=I_2$ no voltage is detected across the junction.

The junctions used to demonstrate the magnetic switch have a metallic barrier, so their dissipation is high when compared with standard SIS tunnel junctions. While for SIS junctions typical values of $I_C{R}_N$ are as high as 1 mV and the junctions are underdamped, typical values of $I_C{R}_N$ in metallic junctions are in the range of tens of nV and the junctions fall in the overdamped regime with quality factor $Q<<1$. In terms of compatibility with existing superconducting electronics, especially with SFQ circuits, this represents a major disadvantage, as in this case the voltage levels are typically of the order of millivolts. Recently, dissipation in spin valve JJs with Ho/Co barriers has been fully classified within the existing models to describe a JJ [9].

A significant step towards a full compatibility between superconducting magnetic RAM (MRAM) and SFQ circuits, and in general towards the use of low dissipation memory devices with high voltage levels, has been reported by Larkin et al. in 2012 [10], by using a modification of the SFS junction characterized in [8], with a more complex barrier. This includes an insulating Al/AlO${}_x$ layer, a thin superconducting Nb interlayer, and a ferromagnetic PdFe layer. Such stacked barrier allows to obtain voltage levels comparable with the ones typical of SFQ circuits, keeping the switching properties intact. Junctions using ferromagnetic insulating barriers as GdN [11], completely falling in the underdamped regime and even showing macroscopic quantum tunneling effects [12] and indication of spin-triplet supercurrents [13], have also shown properties in principle compatible with memory applications.

Recent experiments on SIsFS junctions have demonstrated that the switching mechanism can be enhanced by using external RF fields [14,15]. This result is of relevance because it indicates an additional tool to manipulate the memory state more efficiently. The effect of RF fields on the magnetization loops of ferromagnetic materials has been extensively studied in several other systems [14,16], however, this represents the first attempt on magnetic JJs. We have found that the current level separation enhancement depends on the magnetic field pulse amplitude, on the total energy of the RF train and on the working temperature. The dependence on the magnetic field pulse amplitude is due to the magnetization curve of the ferromagnet: if the pulses are close to the saturation field, then any change induced by the RF field is covered by the saturation, while if the pulse amplitude is too close to the working point, the enhancement is comparable to the current level fluctuations and so it is not significant. The largest effect is observed for intermediate pulse amplitudes, where the slope of the $M\left(H\right)$ curve allows to appreciate the change in the magnetization induced by the microwaves (see Figure 1a, inset). By investigating the enhancement for different power levels and duration of the RF train, we see that the relevant parameter is the total energy associated with the microwaves, rather than the power level or the duration alone. This indicates that the external RF field induces fluctuations in the ferromagnet that are analogous to thermal fluctuations. Finally, we analyzed the thermal behavior of the current level separation enhancement, and we found it compatible with existing literature on PdFe magnetic structure [17]: we observe a larger effect in specific temperature ranges, where one of the two mechanisms that cause ferromagnetism in PdFe can be switched off by the fluctuations induced by the microwaves. This latter result confirms the assumption that the fluctuations induced in PdFe by RF fields are analogous to thermal fluctuations.

The use of RF fields to control the critical current of magnetic Josephson junctions adds to the well-established capabilities to use ferromagnetic junctions for phase qubits [18,19,20].

3. Conclusions and Perspectives

The tunability of the critical current in different types of ferromagnetic junctions leading to their memory capabilities make them possibly useful in the long range not only for the integration in SFQ circuits, but also for the development of specific types of qubits. Apart from the phase qubit [18,19,20], a transmon could benefit of the notions developed for ferromagnetic JJs. The ratio $E_J/E_C$ would be no longer tuned by a dc-SQUID, but using a single magnetic JJ (MJJ), via magnetic pulses or RF trains. The memory properties of ferromagnetic Josephson junctions enable them to retain their state (and so their Josephson energy) when the external field is removed, once they are initialized before all the qubit operations, down to typical qubit operational temperatures. By changing the control input, it is possible to obtain different critical current levels and so different $E_J/E_C$ ratios. In Figure 2a, we show the different critical current levels obtained using RF trains with different nominal energies. The problem of coherence time and dissipation is obvioulsy complex for all types of qubits and not under control [21,22,23]. The architecture qualitatively sketched in Figure 2b in parallel with the capacitive shunting connection $C_B$ typical of a transmon qubit, could reduce noise and decoherence associated with the presence of a static magnetic field and corresponding control lines at low temperatures.