Diffusion of Conserved Charges in Relativistic Heavy Ion Collisions †
Abstract
:1. Introduction
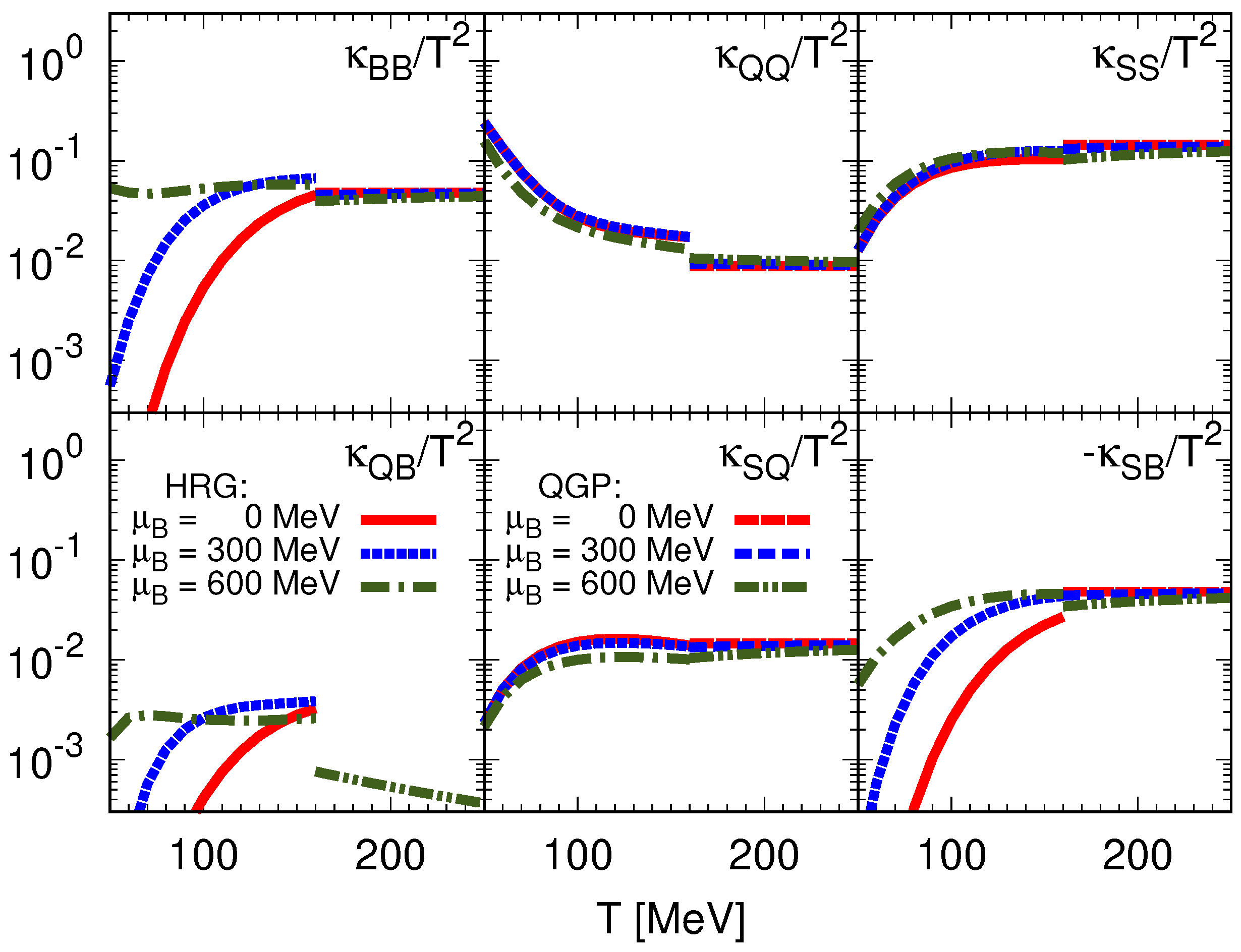
2. Results and Discussion
3. Conclusions
Funding
Acknowledgments
Conflicts of Interest
References
- Aggarwal, M.M.; Ahammed, Z.; Alakhverdyants, A.V.; Alekseev, I.; Anderson, B.D.; Arkhipkin, D.; Averichev, G.S.; Balewski, J.; Barnby, L.S.; Baumgart, S.; et al. (STAR Collaboration). An Experimental Exploration of the QCD Phase Diagram: The Search for the Critical Point and the Onset of De-confinement. 2010. [Google Scholar]
- Mohanty, B. for STAR Collaboration. STAR experiment results from the beam energy scan program at RHIC. J. Phys. 2011, G38, 124023. [Google Scholar] [CrossRef]
- Mitchell, J.T. for PHENIX Collaboration. The RHIC Beam Energy Scan Program: Results from the PHENIX Experiment. Nucl. Phys. 2013, A904–A905, 903c–906c. [Google Scholar] [CrossRef]
- Aarts, G.; Allton, C.; Amato, A.; Giudice, P.; Hands, S.; Skullerud, J.-I. Electrical conductivity and charge diffusion in thermal QCD from the lattice. JHEP 2015, 2, 186. [Google Scholar] [CrossRef]
- Brandt, B.B.; Francis, A.; Jaeger, B.; Meyer, H.B. Charge transport and vector meson dissociation across the thermal phase transition in lattice QCD with two light quark flavors. Phys. Rev. 2016, D93, 054510. [Google Scholar] [CrossRef]
- Ding, H.T.; Kaczmarek, O.; Meyer, F. Thermal dilepton rates and electrical conductivity of the QGP from the lattice. Phys. Rev. 2016, D94, 034504. [Google Scholar] [CrossRef]
- Greif, M.; Bouras, I. , Xu, Z.; Greiner, C. Electric conductivity of the quark-gluon plasma investigated using a perturbative QCD based parton cascade. Phys. Rev. 2014, D90, 094014. [Google Scholar]
- Puglisi, A.; Plumari, S.; Greco, V. Electric Conductivity from the solution of the Relativistic Boltzmann Equation. Phys. Rev. 2014, D90, 114009. [Google Scholar] [CrossRef]
- Arnold, P.; Moore, G.D.; Yaffe, L.G. Transport coefficients in high temperature gauge theories: (I) Leading-log results. JHEP 2000, 11, 001. [Google Scholar] [CrossRef]
- Rougemont, R.; Noronha, J.; Noronha-Hostler, J. Suppression of baryon diffusion and transport in a baryon rich strongly coupled quark-gluon plasma. Phys. Rev. Lett. 2015, 115, 202301. [Google Scholar] [CrossRef]
- Greif, M.; Greiner, C.; Denicol, G.S. Electric Conductivity of a hot hadron gas from a kinetic approach. Phys. Rev. 2016, D93, 096012. [Google Scholar] [CrossRef]
- Rougemont, R.; Critelli, R.; Noronha-Hostler, J.; Noronha, J.; Ratti, C. Dynamical vs. equilibrium properties of the QCD phase transition: a holographic perspective. Phys. Rev. 2017, D96, 014032. [Google Scholar]
- Monnai, A. Dissipative Hydrodynamic Effects on Baryon Stopping. Phys. Rev. 2012, C86, 014908. [Google Scholar] [CrossRef]
- Odyniec, G. The RHIC Beam Energy Scan program in STAR and what’s next …. J. Phys. Conf. Ser. 2013, 455, 012037. [Google Scholar] [CrossRef]
- Adamczyk, L.; Adkins, J.K.; Agakishiev, G.; Aggarwal, M.M.; Ahammed, Z.; Ajitanand, N.N.; Alekseev, I.; Anderson, D.M.; Aoyama, R.; Aparin, A.; et al. (STAR Collaboration). Bulk Properties of the Medium Produced in Relativistic Heavy-Ion Collisions from the Beam Energy Scan Program. Phys. Rev. 2017, C96, 044904. [Google Scholar]
- Chapman, S.; Cowling, T.G.; Burnett, D. The Mathematical Theory of Non-Uniform Gases: An Account of the Kinetic Theory of Viscosity, Thermal Conduction and Diffusion in Gases; Cambridge University Press: Cambridge, UK, 1990. [Google Scholar]
- Denicol, G.S. Kinetic foundations of relativistic dissipative fluid dynamics. J. Phys. 2014, G41. [Google Scholar] [CrossRef]
- Greif, M.; Fotakis, J.A.; Denicol, G.S.; Greiner, C. Diffusion of conserved charges in relativistic heavy ion collisions. Phys. Rev. Lett. 2018, 120, 242301. [Google Scholar] [CrossRef]
- Fotakis, J.A.; et al. in preparation.
- Olive, K.A.; Agashe, K.; Amsler, C.; Antonelli, M.; Arguin, J.-F.; Asner, D.M.; Baer, H.; Band, H.R.; Barnett, R.M.; Basaglia, T.; et al. (Particle Data Group). Review of Particle Physics. Chin. Phys. 2014, C38, 090001. [Google Scholar] [CrossRef]
- Buss, O.; Gaitanos, T.; Gallmeister, K.; van Hees, H.; Kaskulov, M.; Lalakulich, O.; Larionov, A.B.; Leitner, T.; Weil, J.; Mosel, U. Transport-theoretical Description of Nuclear Reactions. Phys. Rept. 2012, 512, 1–124. [Google Scholar] [CrossRef]
- Bass, S.A.; Belkacem, M.; Bleicher, M.; Brandstetter, M.; Bravina, L.; Ernst, C.; Gerland, L.; Hofmann, M.; Hofmann, S.; Konopka, J.; et al. Microscopic Models for Ultrarelativistic Heavy Ion Collisions. Prog. Part. Nucl. Phys. 1998, 41, 255–369. [Google Scholar] [CrossRef]
- Bleicher, M.; Zabrodin, E.; Spieles, C.; Bass, S.A.; Ernst, C.; Soff, S.; Bravina, L.; Belkacem, M.; Weber, H.; Stöcker, H.; Greiner, W. Relativistic hadron–hadron collisions in the ultra-relativisticquantum molecular dynamics model. J. Phys. G: Nucl. Part. Phys. 1999, 25, 1859. [Google Scholar] [CrossRef]
- Xu, Z.; Greiner, C. Shear Viscosity in a Gluon Gas. Phys. Rev. Lett. 2008, 100, 172301. [Google Scholar] [CrossRef] [PubMed]
- Bouras, I.; Molnar, E.; Niemi, H.; Xu, Z.; El, A.; Fochler, O.; Greiner, C.; Rischke, D.H. Relativistic shock waves in viscous gluon matter. Phys. Rev. Lett. 2009, 103, 032301. [Google Scholar] [CrossRef] [PubMed]
- Onsager, L. Reciprocal Relations in Irreversible Processes. I. Phys. Rev. 1931, 37, 405–426. [Google Scholar] [CrossRef]
- Onsager, L. Reciprocal Relations in Irreversible Processes. II. Phys. Rev. 1931, 38, 2265–2279. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fotakis, J.A.; Greif, M.; Denicol, G.S.; Greiner, C. Diffusion of Conserved Charges in Relativistic Heavy Ion Collisions. Proceedings 2019, 10, 31. https://doi.org/10.3390/proceedings2019010031
Fotakis JA, Greif M, Denicol GS, Greiner C. Diffusion of Conserved Charges in Relativistic Heavy Ion Collisions. Proceedings. 2019; 10(1):31. https://doi.org/10.3390/proceedings2019010031
Chicago/Turabian StyleFotakis, Jan A., Moritz Greif, Gabriel S. Denicol, and Carsten Greiner. 2019. "Diffusion of Conserved Charges in Relativistic Heavy Ion Collisions" Proceedings 10, no. 1: 31. https://doi.org/10.3390/proceedings2019010031
APA StyleFotakis, J. A., Greif, M., Denicol, G. S., & Greiner, C. (2019). Diffusion of Conserved Charges in Relativistic Heavy Ion Collisions. Proceedings, 10(1), 31. https://doi.org/10.3390/proceedings2019010031




