Monitoring of Monosodium Urate Crystallization for the Detection of Crystal Arthropathies in Human Joints †
Abstract
:1. Introduction
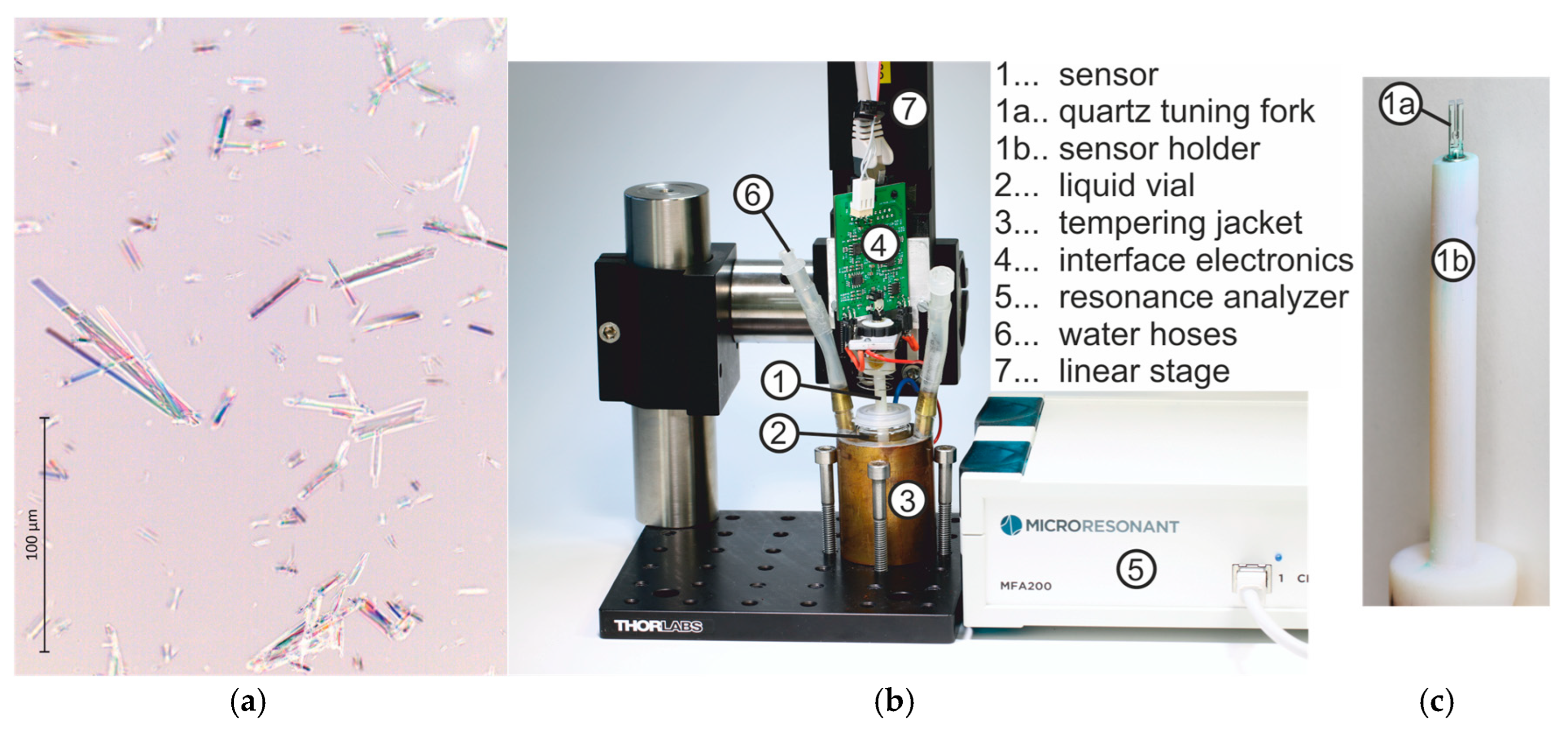
2. Measurement Setup
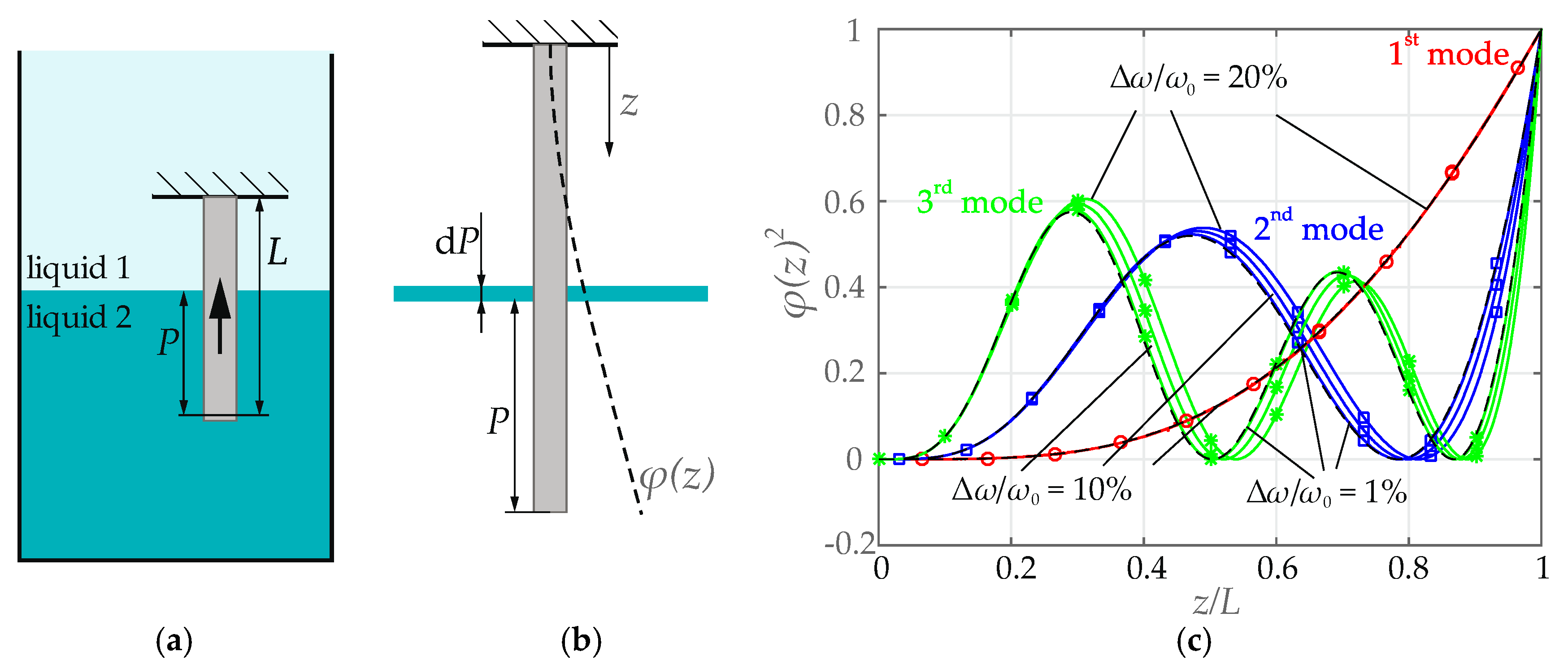
3. Methods
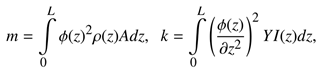
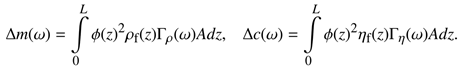
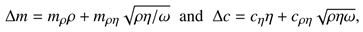
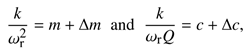
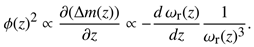
4. Results
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Martillo, M.A.; Nazzal, L.; Crittenden, D.B. The crystallization of monosodium urate. Curr. Rrheumatol. Rep. 2014, 16, 400. [Google Scholar] [CrossRef] [PubMed]
- Voinova, M.V.; Jonson, M.; Kasemo, B. Missing mass effect in biosensor’s QCM applications. Biosens. Bioelectron. 2002, 17, 835–841. [Google Scholar] [CrossRef]
- Niedermayer, A.O.; Voglhuber-Brunnmaier, T.; Sell, J.K.; Jakoby, B. Methods for the robust measurement of the resonant frequency and quality factor of significantly damped resonating devices. Meas. Sci. Technol. 2014, 23, 085107. [Google Scholar] [CrossRef]
- Voglhuber-Brunnmaier, T.; Niedermayer, O.A.; Beigelbeck, R.; Jakoby, B. Resonance parameter estimation from spectral data: Cramér–Rao lower bound and stable algorithms with application to liquid sensors. Meas. Sci. Technol. 2014, 25, 105303. [Google Scholar] [CrossRef]
- Voglhuber-Brunnmaier, T.; Heinisch, M.; Niedermayer, A.O.; Abdallah, A.; Reigelbeck, R.; Jakoby, B. Optimal parameter estimation method for different types of resonant liquid sensors. Procedia Eng. 2014, 87, 1581–1584. [Google Scholar] [CrossRef]
- Mardes, E.W.J. Viscosity and Suspensions and the Einstein Equation. Nature 1940, 145, 970. [Google Scholar] [CrossRef]
- Perrin, C.M.; Dobish, M.A.; Van Keuren, E.; Swift, J.A. Monosodium urate monohydrate crystallization. CrystEngComm 2011, 13, 1111. [Google Scholar] [CrossRef]
- Sader, J.E. Frequency response of cantilever beams immersed in viscous fluids with applications to the atomic force microscope. J. Appl. Phys. 1998, 84, 64–76. [Google Scholar] [CrossRef]
- Heinisch, M.; Voglhuber-Brunnmaier, T.; Reichel, E.K.; Dufour, I.; Jakoby, B. Reduced order models for resonant viscosity and mass density sensors. Sens. Actuators A Phys. 2014, 220, 76–84. [Google Scholar] [CrossRef]
- Timoshenko, S.P.; Woinowsky-Krieger, S. Theory of Plates and Shells; McGraw-hill: New York, NY, USA, 1959. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Voglhuber-Brunnmaier, T.; Reichel, E.K.; Sell, J.K.; Jakoby, B. Monitoring of Monosodium Urate Crystallization for the Detection of Crystal Arthropathies in Human Joints. Proceedings 2017, 1, 544. https://doi.org/10.3390/proceedings1040544
Voglhuber-Brunnmaier T, Reichel EK, Sell JK, Jakoby B. Monitoring of Monosodium Urate Crystallization for the Detection of Crystal Arthropathies in Human Joints. Proceedings. 2017; 1(4):544. https://doi.org/10.3390/proceedings1040544
Chicago/Turabian StyleVoglhuber-Brunnmaier, Thomas, Erwin K. Reichel, Johannes K. Sell, and Bernhard Jakoby. 2017. "Monitoring of Monosodium Urate Crystallization for the Detection of Crystal Arthropathies in Human Joints" Proceedings 1, no. 4: 544. https://doi.org/10.3390/proceedings1040544
APA StyleVoglhuber-Brunnmaier, T., Reichel, E. K., Sell, J. K., & Jakoby, B. (2017). Monitoring of Monosodium Urate Crystallization for the Detection of Crystal Arthropathies in Human Joints. Proceedings, 1(4), 544. https://doi.org/10.3390/proceedings1040544




