A Combined Temperature and Stress Sensor in 0.18 μm CMOS Technology ^†

Abstract

This paper presents a solution for on-chip temperature and mechanical stress measurement in CMOS integrated circuits. Thereby both temperature and stress sensors are realized as resistive Wheatstone bridges. By design, both sensors show outputs affected by non-linearities and parasitic cross-sensitivities. The novelty presented in this work is to combine both non-ideal sensor outputs by applying a two-dimensional Newton-Raphson method to extract the actual values of temperature and mechanical stress which were obtained with errors of less than 0.5 K and 0.5 MPa.

1. Introduction

The accurate on-chip measurement of temperature free from the parasitic impact of package mechanical stress is of utmost importance for integrated sensors in order to provide a temperature-compensated output signal. Moreover, in Hall sensor microsystems the information of both temperature and mechanical stress is required for the compensation of thermal and stress-related cross-sensitivities [1]. However, CMOS temperature sensors are known to suffer from mechanical stress, often referred to as package shift [2]. Similarly, mechanical stress sensors are known to show a parasitic temperature dependence [3].

In contrast to previous attempts [2,4], this work does not try to minimize parasitic effects via optimization of the sensor designs. Quite to the contrary, the purpose of this work is to extract the accurate values of temperature and mechanical stress from the combination of both erroneous sensor outputs, removing non-linearities as well as cross-sensitivities. Therefore our solution is useful not only for the measurement of temperature and mechanical stress but may be applicable in general to a variety of multisensor systems.

2. Materials and Methods

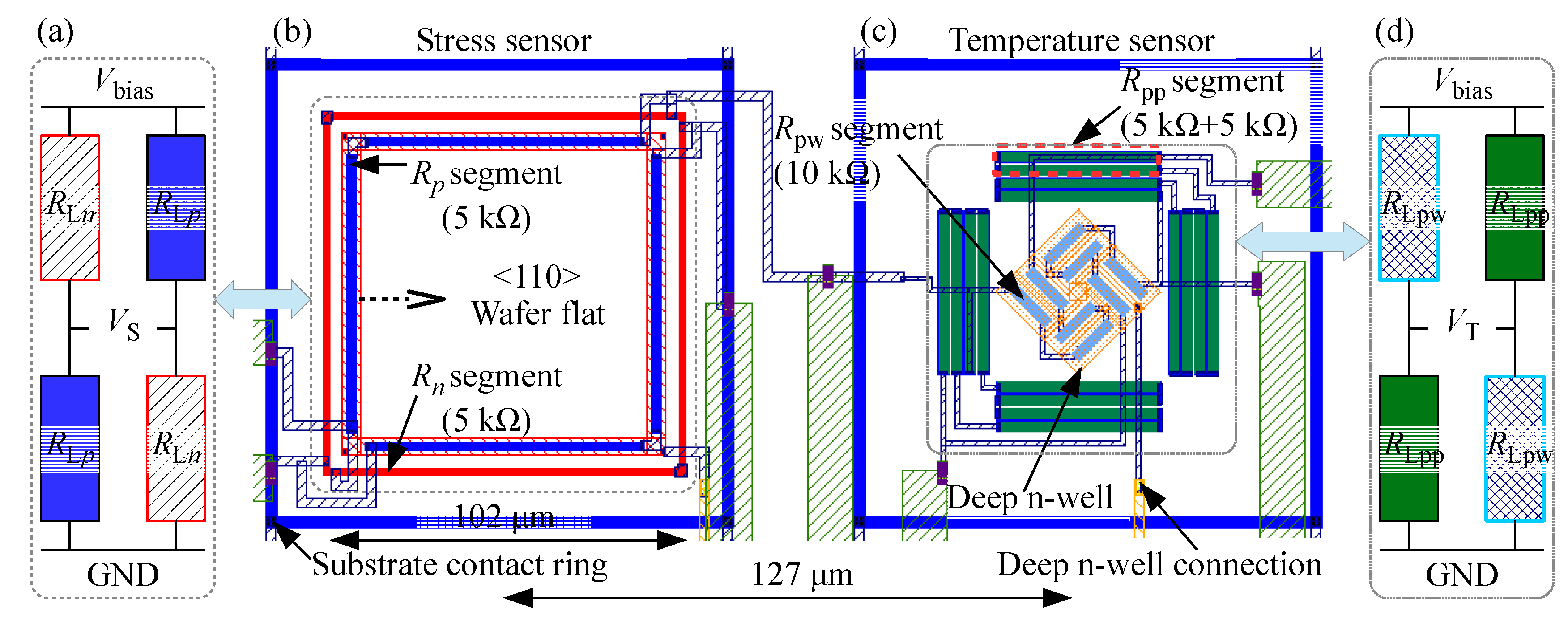
2.1. Sensor Design and Implementation

The stress and temperature sensors consist of different CMOS in-plane resistors connected to Wheatstone bridges, as illustrated in the schematic diagrams of Figure 1a,d, respectively. The piezoresistive stress sensor combines highly doped n-type (R_n) and p-type (R_p) resistors, whereas for the temperature sensor p-well (R_pw) and p-type polysilicon (R_pp) resistors are used. For the sake of isotropy wrt. in-plane normal stresses, the two sensors are made of L-shaped resistors (R_L), as detailed in [3,5]. The layouts of the sensors are shown in Figure 1b,c. They have sizes of 102 μm and 61 μm, respectively.

2.2. Sensor Response

The sensors provide a differential, ratiometric output voltage. Due to the combination of different resistor materials in the same bridge, an offset voltage appears, i.e., the output voltage at the reference stress 0 MPa and reference temperature 25 °C. This parasitic output signal is subtracted. The resulting offset-free signals V_σ and V_T as a function of in-plane stress Δσ_ip and temperature ΔT are described by [3,5]

V_σ/V_bias = ε_σΔσ_ip + γ_σΔT + δ_σΔT²,

(1)

V_T/V_bias = ε_TΔσ_ip + γ_TΔT + δ_TΔT²,

(2)

where ε_σ, γ_σ, δ_σ, ε_T, γ_T, and δ_T denote the stress-related sensitivity and the first and second order temperature coefficients of the stress and temperature sensors, respectively. V_bias, Δσ_ip, and ΔT denote the supply voltage and the deviations of in-plane mechanical stress and temperature, respectively, from the reference values. The coefficients ε_i, γ_i, and δ_i of (1) and (2) are obtained by calibration.

2.3. Extraction of Temperature and Mechanical Stress

Both sensor outputs depend on temperature and mechanical stress. Additionally, the thermal response is expected to show a quadratic term. Nevertheless, the accurate temperature and mechanical stress magnitude can be retrieved from the two erroneous sensor signals (1) and (2). In our case an iterative approach via a two-dimensional Newton-Raphson (2DNR) method was used, i.e.,

x_n+1 = x_n − [J]⁻¹[F(x_n) − y],

(3)

where x_n+1 = [Δσ_ip,n+1, ΔT_n+1], x_n= [Δσ_ip,n, ΔT_n] denote two successive iterations of the extracted stress and temperature vector, J, F = [V_σ,calc, V_T,calc], and y = [V_σ,meas, V_T,meas] denote the Jacobian matrix of partial derivatives of (1) and (2), the vector of calculated stress and temperature function values obtained from (1) and (2), and the vector of measured sensor outputs. The elements of J are derived straightforwardly from the polynomial expressions (1) and (2), i.e., ∂V_σ/∂Δσ_ip = V_biasε_σ and ∂V_σ/∂ΔT = V_bias(γ_σ + 2δ_σΔT) and similarly for (2). The iteration of (3) is repeated until the residual difference |x_n+1 − x_n| is below a given threshold, e.g., 0.1 K and 0.1 MPa.

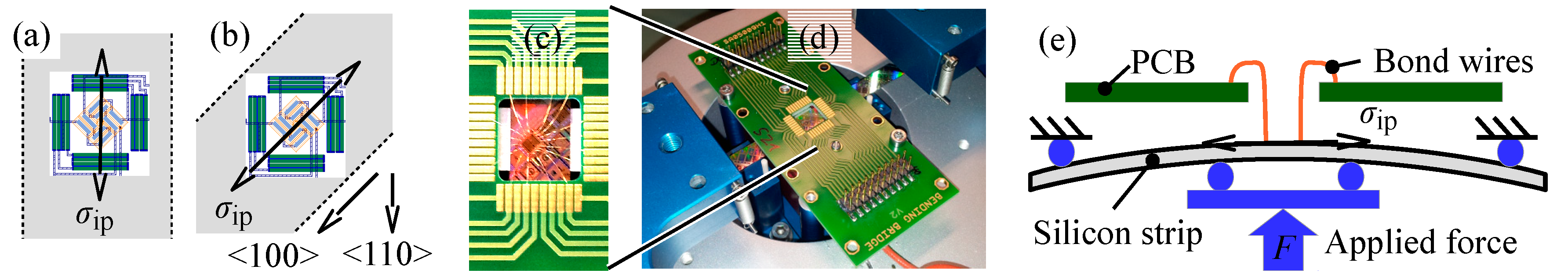
2.4. Experimental Setup

Samples were fabricated in standard 0.18 μm CMOS technology. Strips were cut from the wafer along the <110> (S#1) and <100> (S#2) crystal directions, as shown in Figure 2a,b, respectively. The strips containing the sensors were assembled to substrate PCBs and thereafter mounted in the four-point bending bridge, see Figure 2c,d. Via bending, the applied force gives rise to in-plane mechanical stress on the strip surface, as illustrated in Figure 2e. Placing the entire setup into a climate chamber allows to independently vary the temperature between 10 °C and 60 °C and the mechanical stress from 0 MPa to 75 MPa.

3. Results

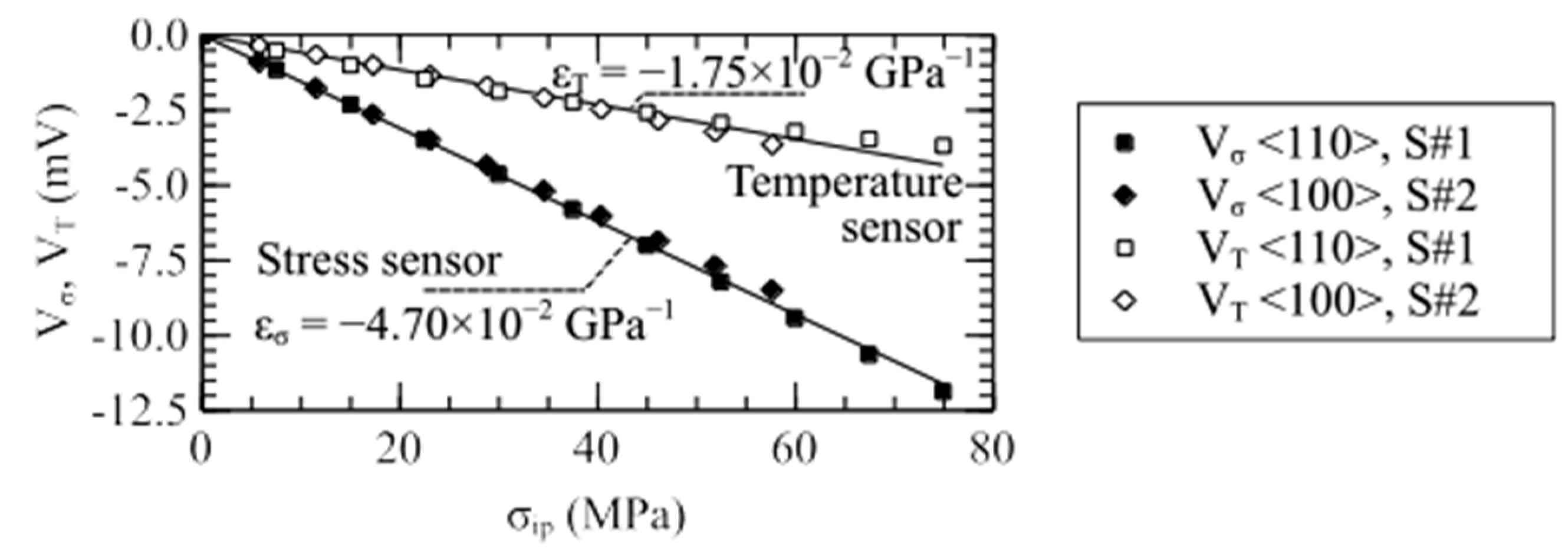
3.1. Stress Response

The stress-related responses of the stress and temperature sensors were measured at V_bias = 3.3 V. The results are shown in Figure 3. The corresponding sensitivities were extracted to be ε_σ = −4.70 × 10⁻² GPa⁻¹ and ε_T = −1.75 × 10⁻² GPa⁻¹, respectively, by averaging S#1 and S#2. The difference between the sensitivities of S#1 and S#2 was below 2%, indicating the sensors isotropic response wrt. in-plane stresses.

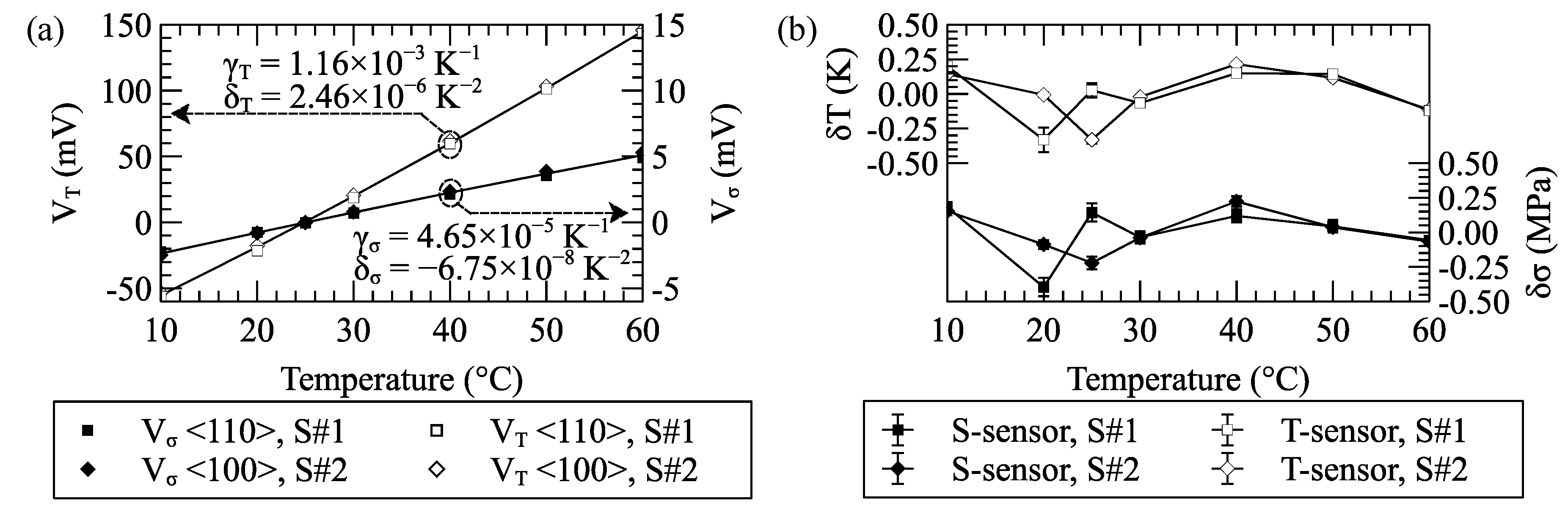
3.2. Thermal Response

The thermal responses of V_σ and V_T were measured and subsequently approximated by the second-order functions (1) and (2). The corresponding coefficients for the stress sensor were extracted as γ_σ = 4.65 × 10⁻⁵ K⁻¹ and δ_σ = −6.75 × 10⁻⁸ K⁻², by averaging S#1 and S#2, and γ_T = 1.16 × 10⁻³ K⁻¹ and δ_T = 2.46 × 10⁻⁶ K⁻², for the temperature sensor. The results are shown in Figure 4a. The differences of γ_σ and γ_T between S#1 and S#2 from the same wafer were observed to be below 10% and 1%, respectively.

3.3. Extraction of Temperature and Mechanical Stress, Residual Error

The 2DNR method (3) was applied to pairs of measured sensor output voltages. The obtained values of temperature and mechanical stress were found to match the externally applied test conditions, with differences below 0.5 K and 0.5 MPa, respectively, as shown in Figure 4b.

4. Discussion

A method to extract the values of temperature and mechanical stress in CMOS ICs was demonstrated using simple resistive bridge sensors. Thereby the proposed method successfully removes parasitic cross-sensitivities and non-linearities. In contrast to [2,4] no effort for transducer optimization, i.e., linearization and elimination of parasitic effects was necessary, as this regularization is achieved numerically.