Amorphous SiC/c-ZnO-Based Lamb Mode Sensor for Liquid Environments †
Abstract
:1. Introduction
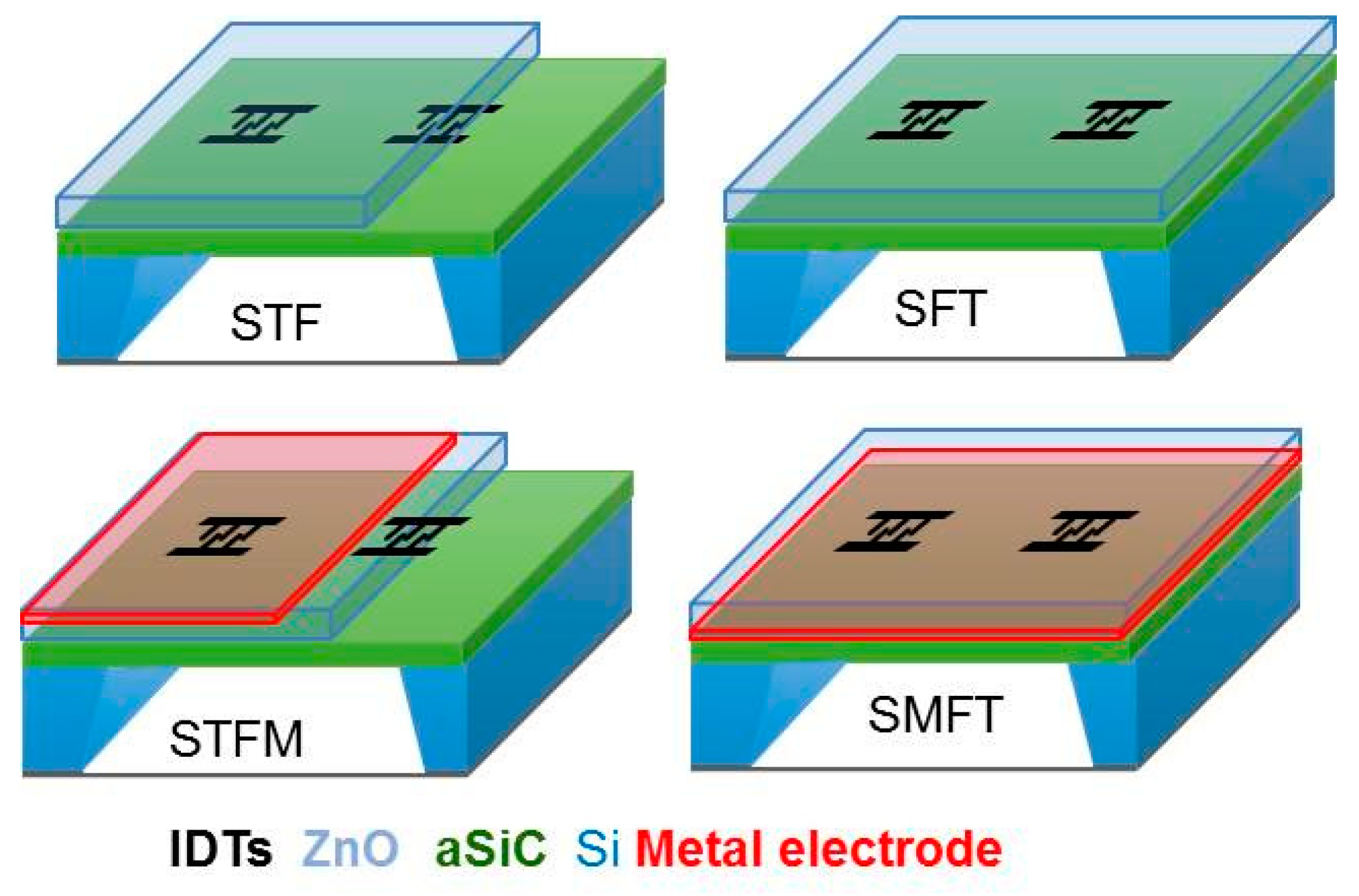
2. S0 Lamb Mode in a-SiC and a-SiC/ZnO Plates
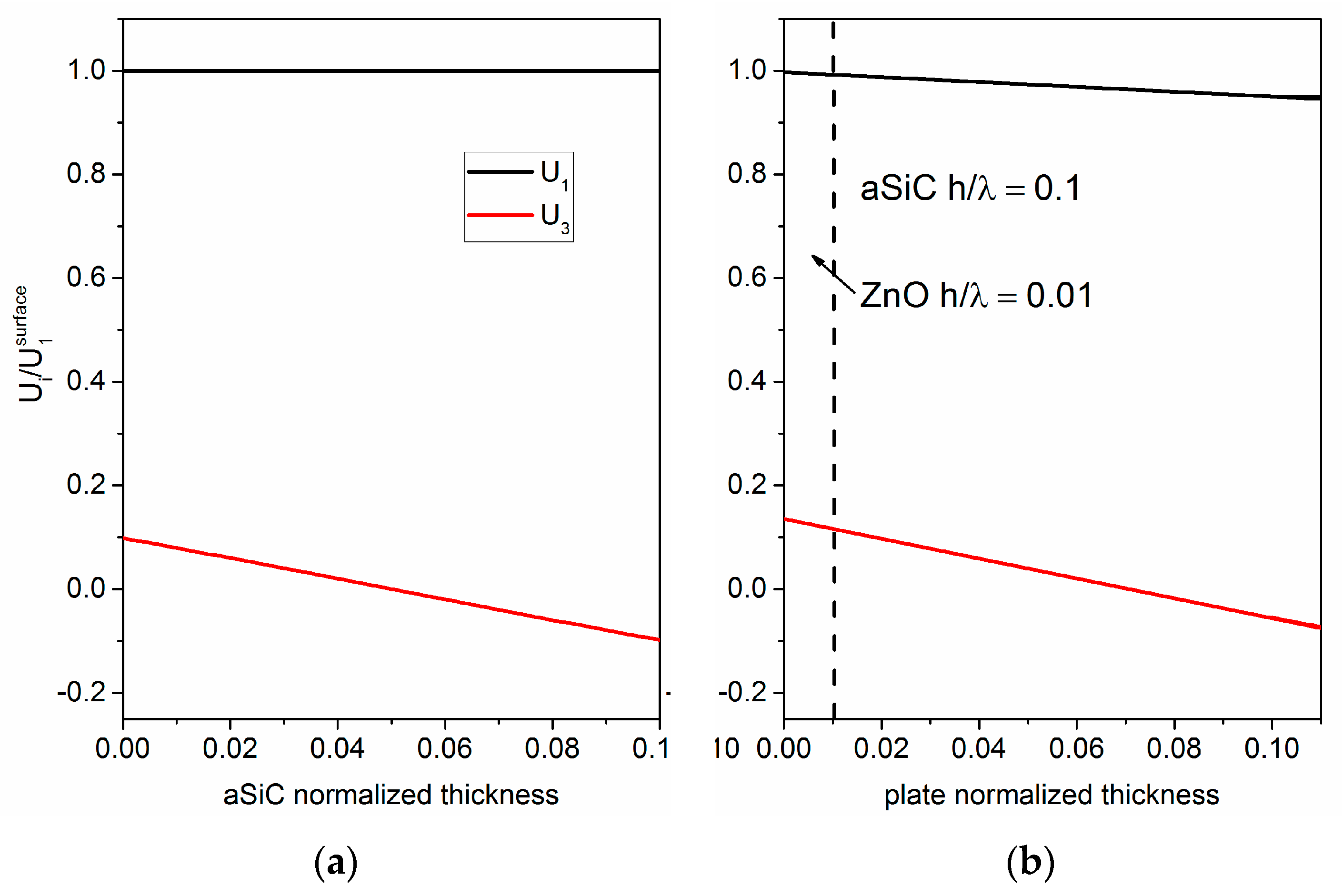
2.1. Acoustic Field Profile
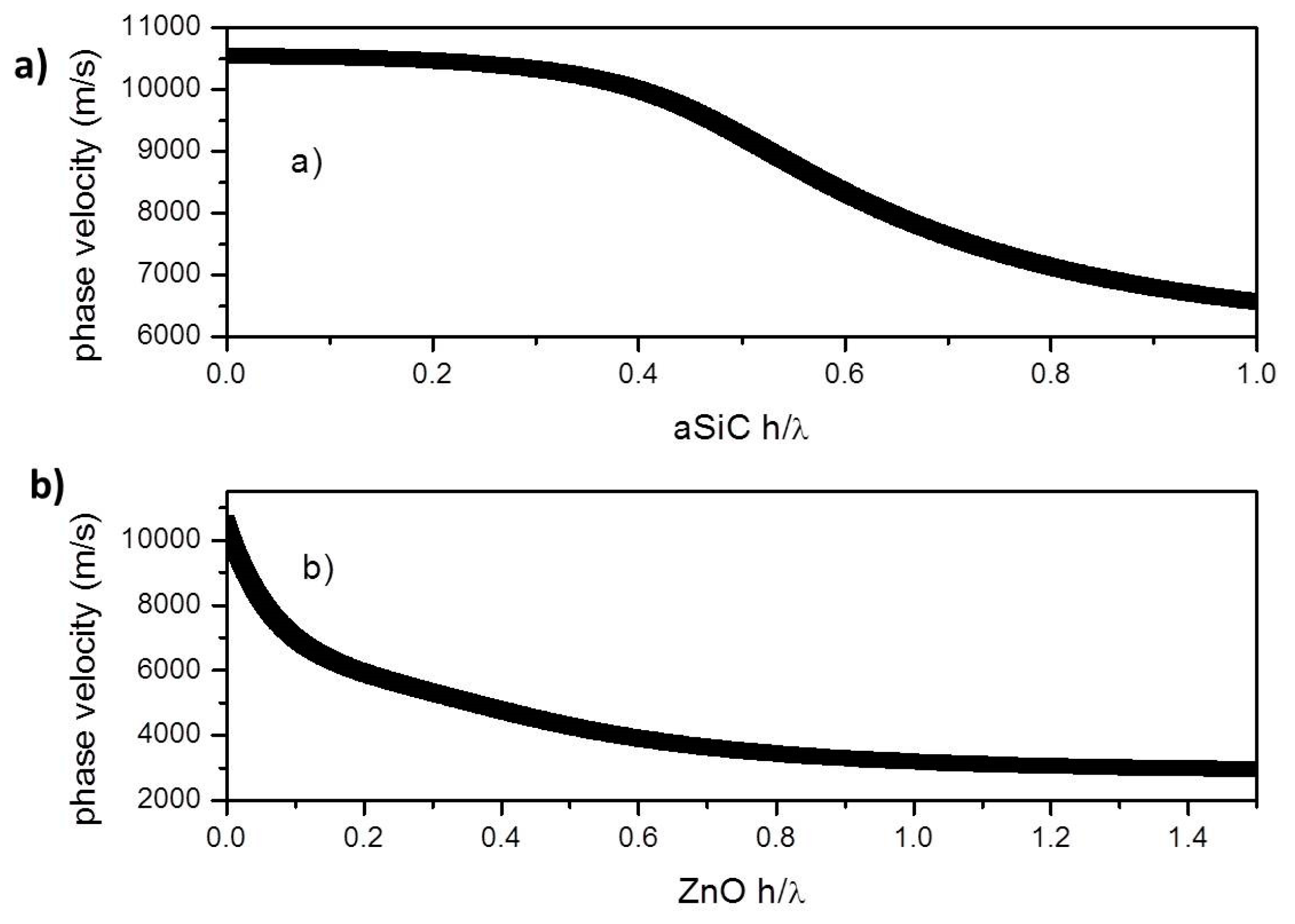
2.2. Phase Velocity Dispersion Curves
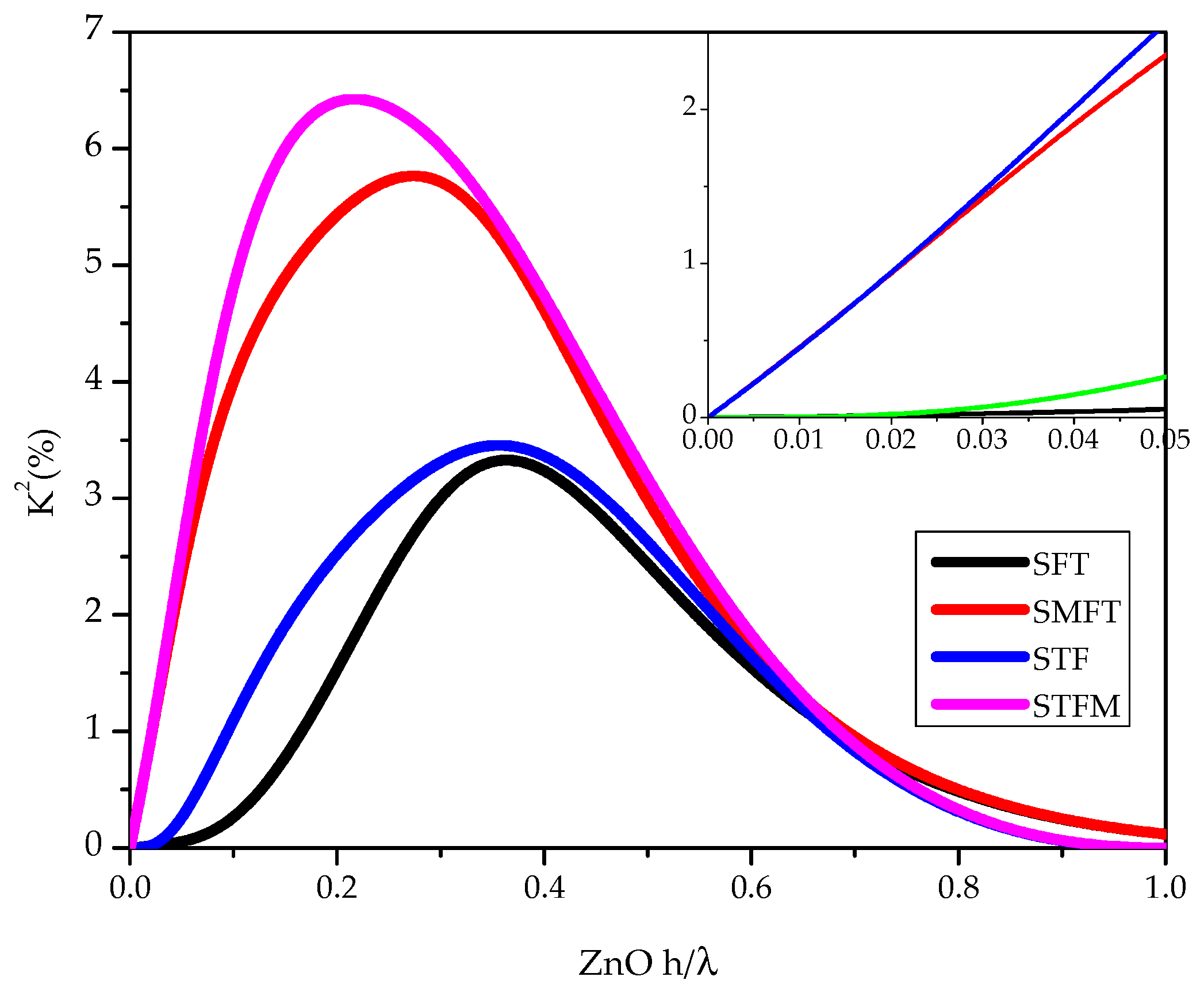
2.3. The Coupling Coefficient Dispersion Curves
3. S0 Lamb Wave Sensor
3.1. Gravimetric Sensor
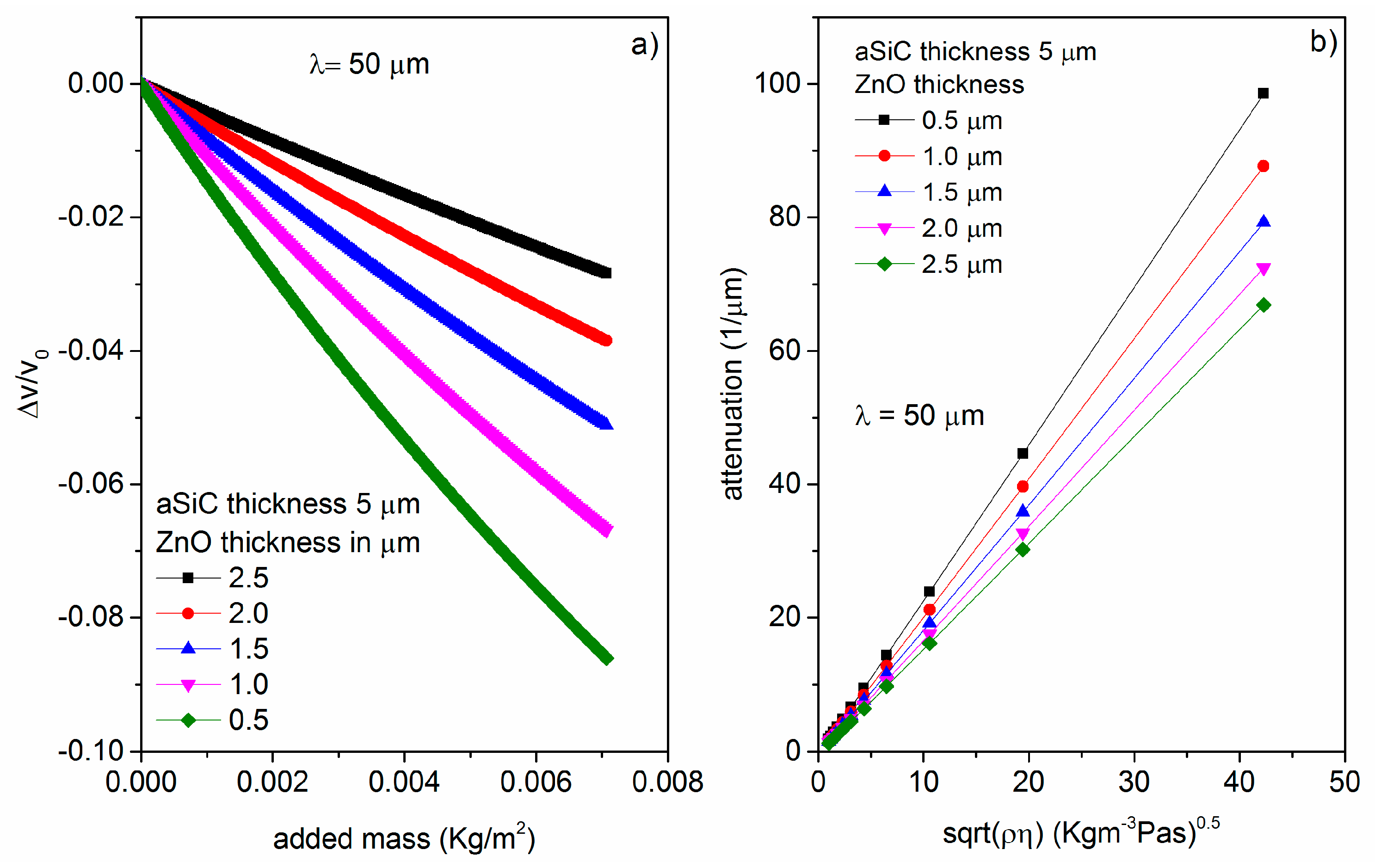
3.2. Viscosity Sensor
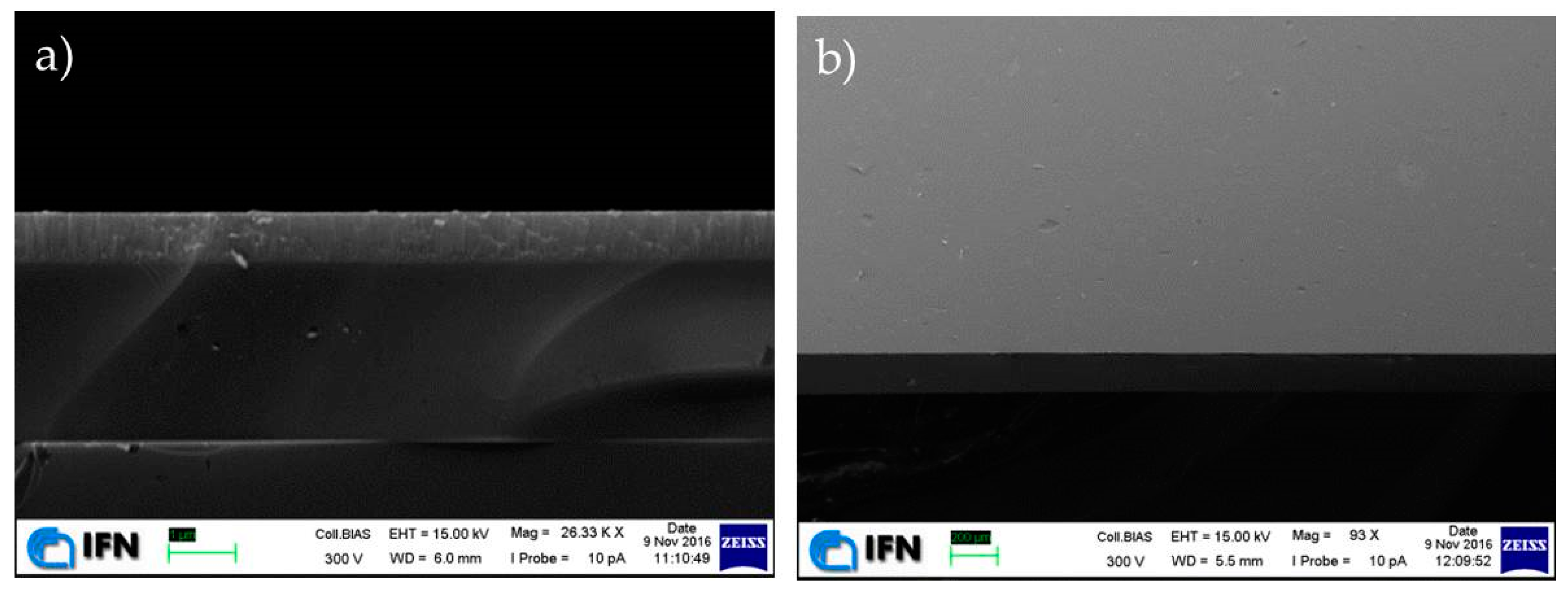
4. A-SiC and ZnO Layers Deposition
5. Conclusions
Author Contributions
Acknowledgments
Conflicts of Interest
References
- Caliendo, C. Theoretical investigation of high velocity, temperature compensated Rayleigh waves along AlN/SiC substrates for high sensitivity mass sensors. Appl. Phys. Lett. 2012, 100. [Google Scholar] [CrossRef]
- Hellwege, K.-H.; Hellwege, A.M. Landolt-Börnstein: Numerical Data and Functional Relationships in Science and Technology; New Series, Group III; Springer: Berlin, Germany, 1979; Volume 11. [Google Scholar]
- Vashishta, P.; Kalia, R.K.; Nakano, A. Interaction potential for silicon carbide: A molecular dynamics study of elastic constants and vibrational density of states for crystalline and amorphous silicon carbide. J. Appl. Phys. 2007, 101, 103515. [Google Scholar] [CrossRef]
- Nayfeh, A.H.; Nagy, P.B. Excess attenuation of leaky Lamb waves due to viscous fluid loading. J. Acoust. Soc. Am. 1997, 101. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2016 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Caliendo, C.; Hamidullah, M.; Laidoudi, F. Amorphous SiC/c-ZnO-Based Lamb Mode Sensor for Liquid Environments. Proceedings 2017, 1, 21. https://doi.org/10.3390/ecsa-3-C004
Caliendo C, Hamidullah M, Laidoudi F. Amorphous SiC/c-ZnO-Based Lamb Mode Sensor for Liquid Environments. Proceedings. 2017; 1(2):21. https://doi.org/10.3390/ecsa-3-C004
Chicago/Turabian StyleCaliendo, Cinzia, Muhammad Hamidullah, and Farouk Laidoudi. 2017. "Amorphous SiC/c-ZnO-Based Lamb Mode Sensor for Liquid Environments" Proceedings 1, no. 2: 21. https://doi.org/10.3390/ecsa-3-C004
APA StyleCaliendo, C., Hamidullah, M., & Laidoudi, F. (2017). Amorphous SiC/c-ZnO-Based Lamb Mode Sensor for Liquid Environments. Proceedings, 1(2), 21. https://doi.org/10.3390/ecsa-3-C004





