A Stable Forward Modeling Approach in Heterogeneous Attenuating Media Using Reapplied Hilbert Transform
Abstract
1. Introduction
2. Materials and Methods
3. Numerical Examples
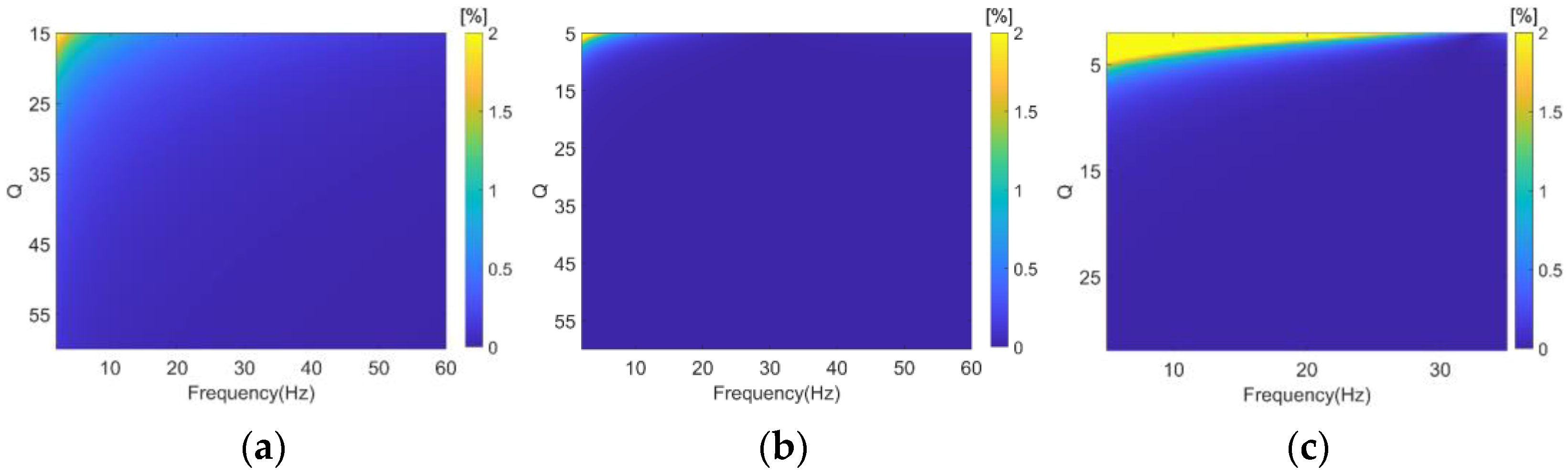
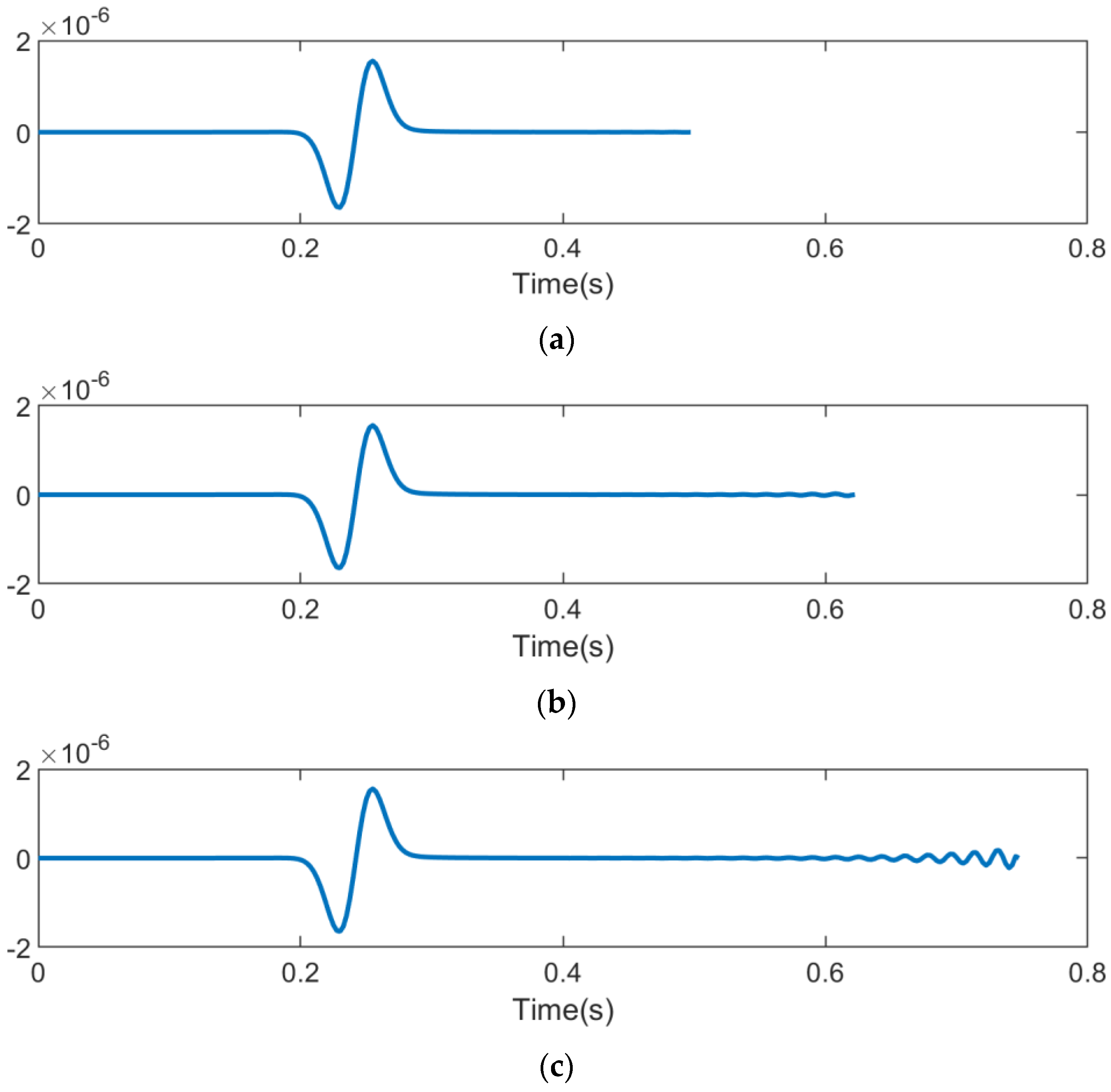
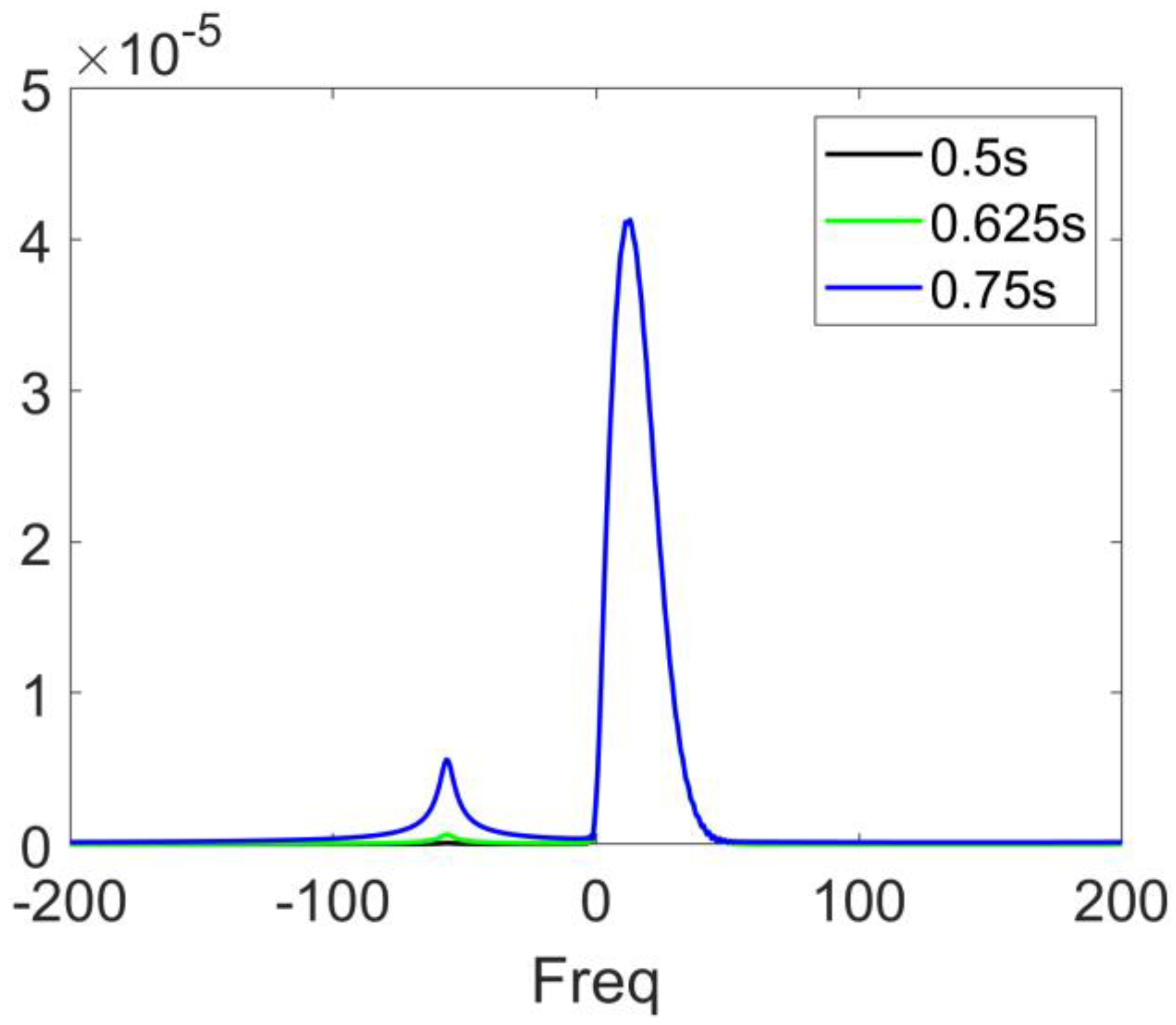
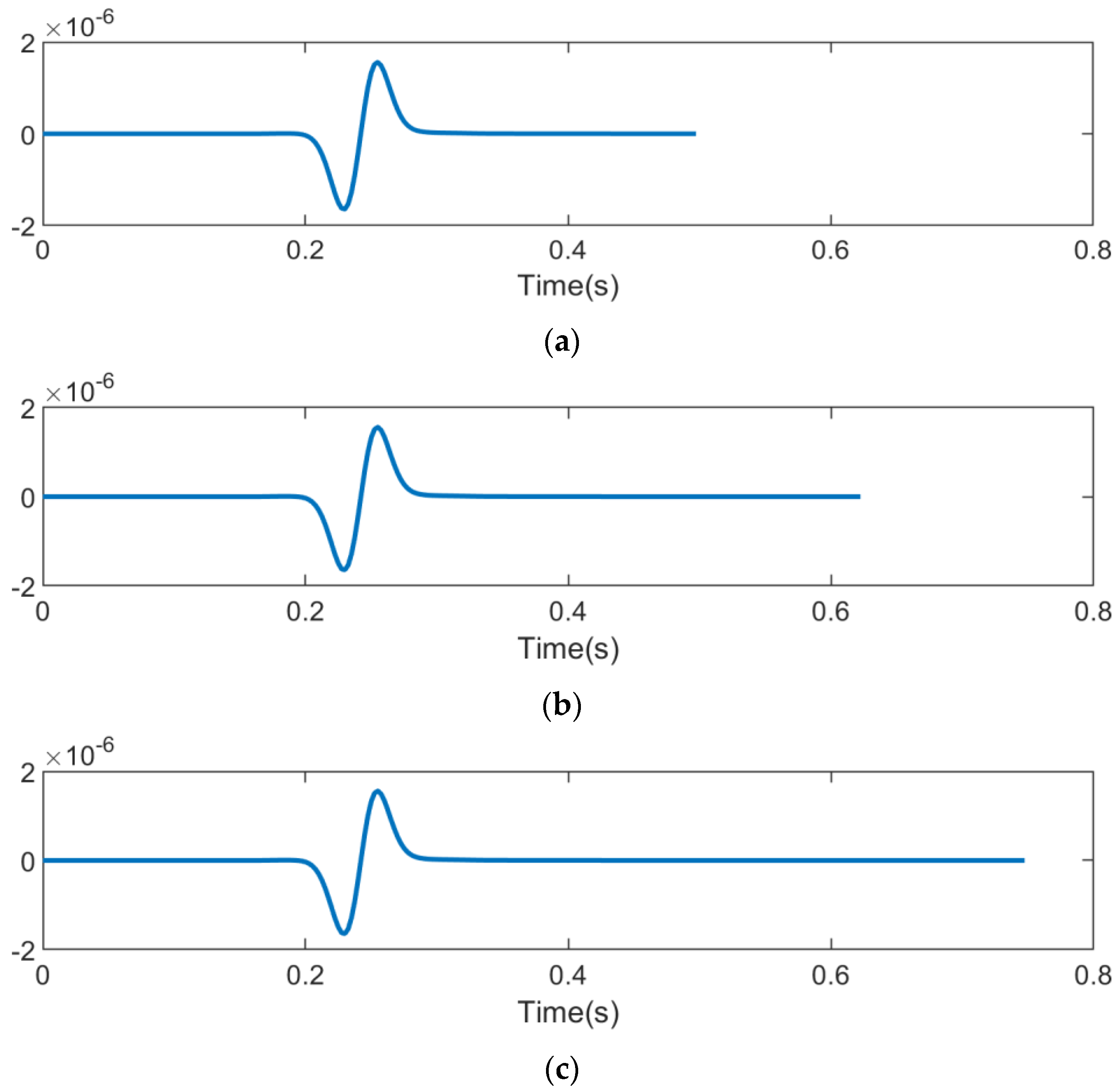
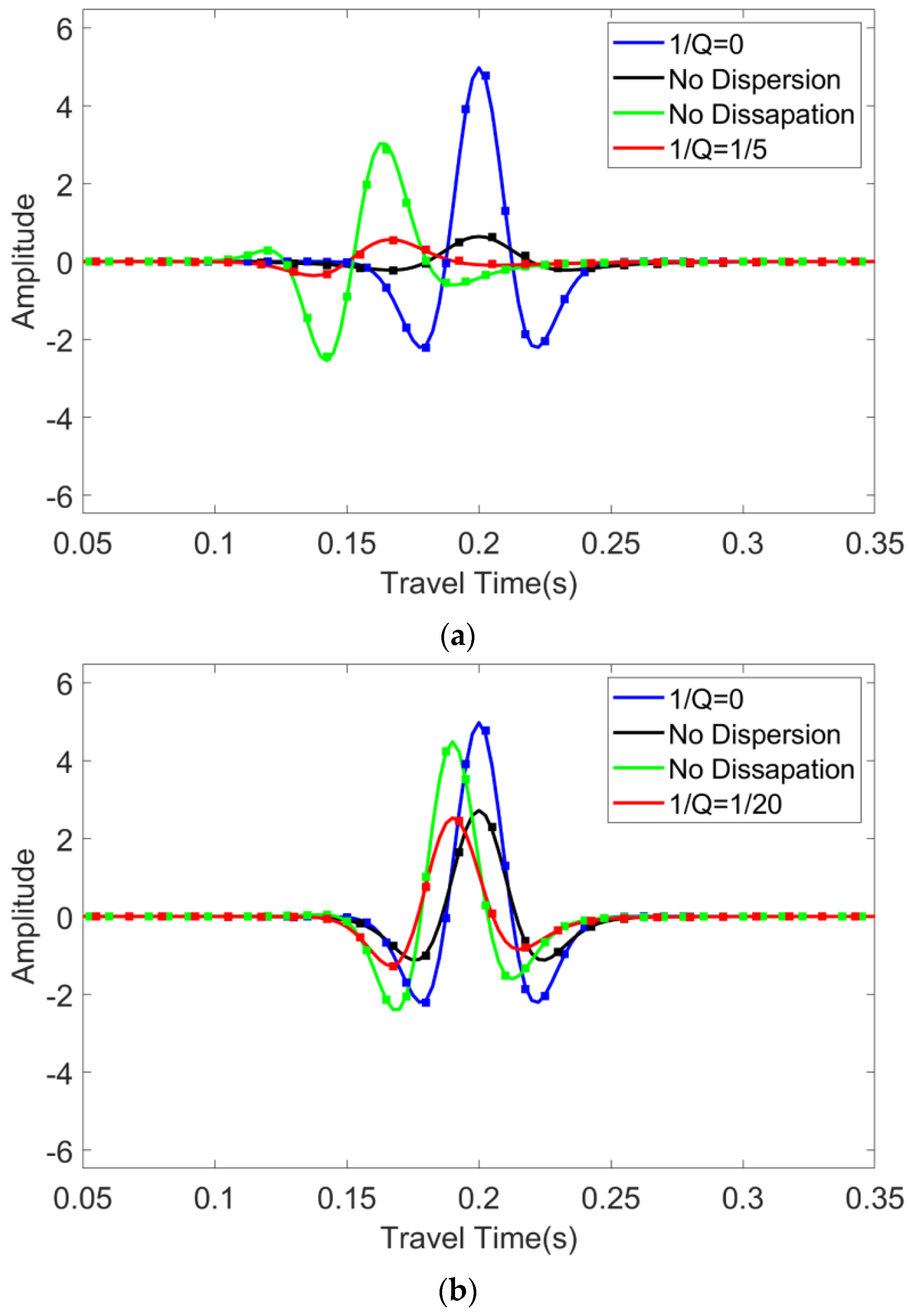
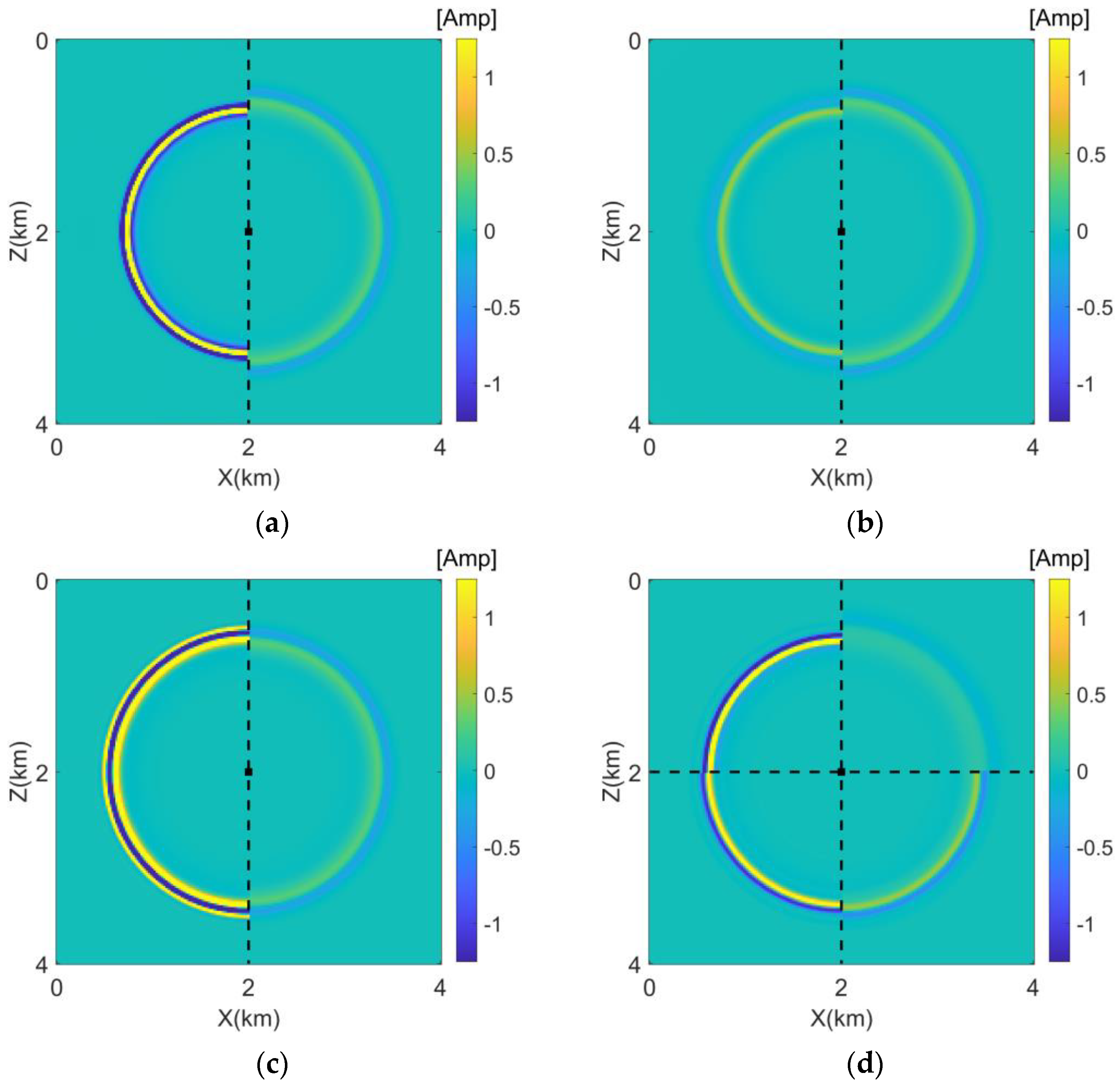
3.1. Homogeneous Model
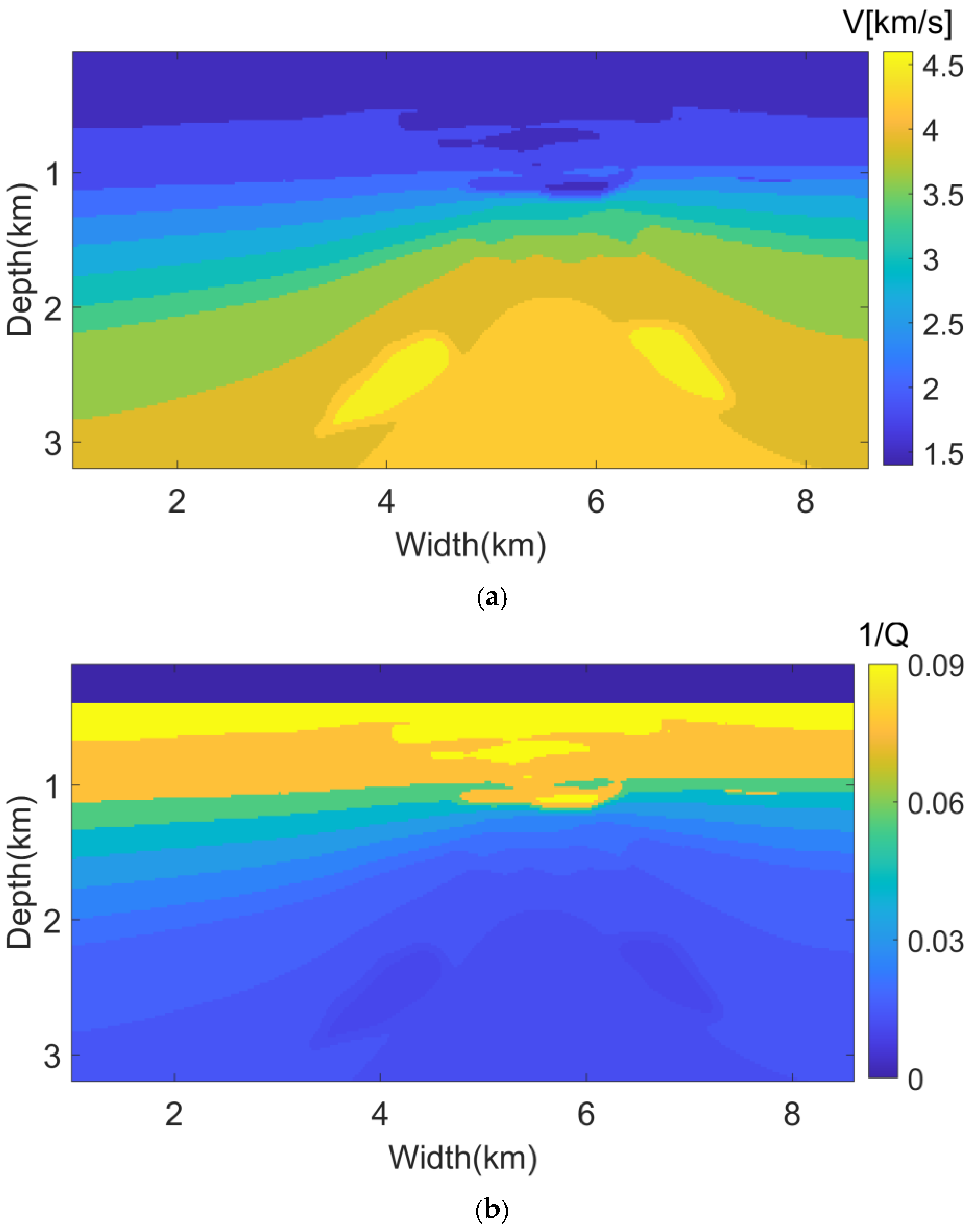
3.2. Chimney Model
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Futterman, W. Dispersion body waves. J. Geophys. Res. 1962, 67, 5279–5291. [Google Scholar] [CrossRef]
- Kjartansson, E. Constant Q-wave propagation and attenuation. J. Geophys. Res. 1979, 84, 4737–4748. [Google Scholar] [CrossRef]
- Aki, K.; Richards, P. Quantitative Seismology, 2nd ed.; University Science Books: Melville, NY, USA, 2002. [Google Scholar]
- Carcione, J.M. Wave Fields in Real Media: Theory and Numerical Simulation of Wave Propagation in Anisotropic, Anelastic, Porous and Electromagnetic Media; Elsevier: Amsterdam, The Netherlands, 2007. [Google Scholar]
- Zhang, Y.; Chen, T.; Zhu, H.; Liu, Y. A stable Q-compensated reverse-time migration method using a modified fractional viscoacoustic wave equation. Geophysics 2024, 89, S15–S29. [Google Scholar] [CrossRef]
- Xu, J.; Wang, R.; Zhang, Y. Research progress of seismic attenuation models. Prog. Geophys. 2024, 39, 525–541. [Google Scholar]
- Hale, D. An inverse Q-filter. Stanf. Explor. Proj. Rep. 1981, 28, 231–244. [Google Scholar]
- Hargreaves, N. Similarity and the inverse Q filter: Some simple algorithms for inverse Q filtering. Geophysics 1992, 57, 944–947. [Google Scholar] [CrossRef]
- Virieux, J.; Operto, S. An overview of full-waveform inversion in exploration geophysics. Geophysics 2009, 74, WCC1–WCC26. [Google Scholar] [CrossRef]
- Carcione, J.; Kosloff, D.; Kosloff, R. Wave propagation simulation in a linear viscoacoustic medium. Geophys. J. Int. 1988, 93, 393–401. [Google Scholar] [CrossRef]
- Robertsson, J.; Blanch, J.; Symes, W. Viscoelastic finite- difference modeling. Geophysics 1994, 59, 1444–1456. [Google Scholar] [CrossRef]
- Blanch, J.; Robertsson, J.; Symes, W. Modeling of a constant Q: Methodology and algorithm for an efficient and optimally inexpensive viscoelastic technique. Geophysics 1995, 60, 176–184. [Google Scholar] [CrossRef]
- Emmerich, H.; Korn, M. Incorporation of attenuation into time- domain computations of seismic wave fields. Geophysics 1987, 52, 1252–1264. [Google Scholar] [CrossRef]
- Zhu, T.; Carcione, J.; Harris, J. Approximating constant-Q seismic propagation in the time domain. Geophys. Prospect. 2013, 61, 931–940. [Google Scholar] [CrossRef]
- Carcione, J.; Cavallini, F.; Mainardi, F.; Hanyga, A. Time-domain modeling of constant-Q seismic waves using fractional derivatives. Pure Appl. Geophys. 2002, 159, 1719–1736. [Google Scholar] [CrossRef]
- Zhu, T.; Carcione, J. Theory and modelling of constant-Q P- and S-waves using fractional spatial derivatives. Geophys. J. Int. 2014, 196, 1787–1795. [Google Scholar] [CrossRef]
- Zhu, T.; Harris, J. Modeling acoustic wave propagation in heterogeneous attenuating media using decoupled fractional Laplacians. Geophysics 2014, 79, T105–T116. [Google Scholar] [CrossRef]
- Yao, J.; Zhu, T.; Hussain, F. Locally solving fractional Laplacian viscoacoustic wave equation using Hermite distributed approximating functional method. Geophysics 2017, 82, T59–T67. [Google Scholar] [CrossRef]
- Li, Q.; Zhou, H.; Zhang, Q. Efficient reverse time migration based on fractional Laplacian viscoacoustic wave equation. Geophys. J. Int. 2016, 204, 488–504. [Google Scholar] [CrossRef]
- Aki, K.; Richards, P. Quantitative Seismology, 1st ed.; University Science Books: Melville, NY, USA, 1980. [Google Scholar]
- Operto, S.; Virieux, J.; Amestoy, P.; L’Excellent, J.; Giraud, L.; Ali, H. 3D finite-difference frequency-domain modeling of vis-coacoustic wave propagation using a massively parallel direct solver: A feasibility study. Geophysics 2007, 72, SM195–SM211. [Google Scholar] [CrossRef]
- Mu, X.; Huang, J.; Wen, L.; Zhuang, S. Modeling viscoacoustic wave propagation using a new spatial variable order fractional Laplacian wave equation. Geophysics 2021, 86, T487–T507. [Google Scholar] [CrossRef]
- Carcione, J. Theory and modeling of constant-Q P- and S-waves using fractional time derivatives. Geophysics 2009, 74, T1–T11. [Google Scholar] [CrossRef]
- Zhu, T. Numerical simulation of seismic wave propagation in viscoelastic-anisotropic media using frequency-independent Q wave equation. Geophysics 2017, 82, WA1–WA10. [Google Scholar] [CrossRef]
- Chen, H.; Zhou, H.; Rao, Y. Constant-Q wave propagation and compensation by pseudo-spectral time-domain methods. Comput. Geosci. 2021, 155, 104861. [Google Scholar] [CrossRef]
- Fathalian, A.; Trad, D.; Innanen, K. An approach for attenuation-compensating multidimensional constant-Q viscoacoustic reverse time migration. Geophysics 2020, 85, S33–S46. [Google Scholar] [CrossRef]
- Chen, H.; Zhou, H.; Rao, Y. An implicit stabilization strategy for Q-compensated reverse time migration. Geophysics 2020, 85, S169–S183. [Google Scholar] [CrossRef]
- Sun, J.; Zhu, T. Strategies for stable attenuation compensation in reverse-time migration. Geophys. Prospect. 2018, 66, 498–511. [Google Scholar] [CrossRef]
- Xing, G.; Zhu, T. Modeling frequency-independent Q viscoacoustic wave propagation in heterogeneous media. J. Geophys. Res. Solid Earth 2019, 124, 11568–11584. [Google Scholar] [CrossRef]
- Liu, H.; Luo, Y. An analytic signal-based accurate time-domain vis-coacoustic wave equation from the constant-Q theory. Geophysics 2021, 86, T117–T126. [Google Scholar] [CrossRef]
- Carcione, J. A generalization of the Fourier pseudospectral method. Geophysics 2010, 75, A53–A56. [Google Scholar] [CrossRef]
- Liu, H.; Luo, Y. Comparing four numerical stencils for elastic wave simulation. In SEG Technical Program Expanded Abstracts; Society of Exploration Geophysicists (SEG): Houston, TX, USA, 2019; pp. 3745–3749. [Google Scholar] [CrossRef]
- Liu, H.; Zhang, H. Reducing computation cost by Lax-Wendroff methods with fourth-order temporal accuracy. Geophysics 2019, 84, T109–T119. [Google Scholar] [CrossRef]


| Equation Type | Wavenumber Parameter | Velocity Parameter |
|---|---|---|
| Acoustic | ||
| Dissipation only | ||
| Dispersion only | ||
| Dispersion and dissipation |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Deng, S.; Shi, S.; Liu, H. A Stable Forward Modeling Approach in Heterogeneous Attenuating Media Using Reapplied Hilbert Transform. Fractal Fract. 2024, 8, 434. https://doi.org/10.3390/fractalfract8070434
Deng S, Shi S, Liu H. A Stable Forward Modeling Approach in Heterogeneous Attenuating Media Using Reapplied Hilbert Transform. Fractal and Fractional. 2024; 8(7):434. https://doi.org/10.3390/fractalfract8070434
Chicago/Turabian StyleDeng, Songmei, Shaolin Shi, and Hongwei Liu. 2024. "A Stable Forward Modeling Approach in Heterogeneous Attenuating Media Using Reapplied Hilbert Transform" Fractal and Fractional 8, no. 7: 434. https://doi.org/10.3390/fractalfract8070434
APA StyleDeng, S., Shi, S., & Liu, H. (2024). A Stable Forward Modeling Approach in Heterogeneous Attenuating Media Using Reapplied Hilbert Transform. Fractal and Fractional, 8(7), 434. https://doi.org/10.3390/fractalfract8070434





