A Class of Fifth-Order Chebyshev–Halley-Type Iterative Methods and Its Stability Analysis

Abstract

In this paper, a family of fifth-order Chebyshev–Halley-type iterative methods with one parameter is presented. The convergence order of the new iterative method is analyzed. By obtaining rational operators associated with iterative methods, the stability of the iterative method is studied by using fractal theory. In addition, some strange fixed points and critical points are obtained. By using the parameter space related to the critical points, some parameters with good stability are obtained. The dynamic plane corresponding to these parameters is plotted, visualizing the stability characteristics. Finally, the fractal diagrams of several iterative methods on different polynomials are compared. Both numerical results and fractal graphs show that the new iterative method has good convergence and stability when $\alpha =\frac{1}{2}$.

1. Introduction

With the rapid development of science and technology, scientific calculation is becoming more and more important. Scientific computing has been widely used in all walks of life, such as the analysis of meteorological data images and the design of aircraft, automobiles and ships, and high-tech research cannot be separated from scientific computing. Therefore, it is often necessary to find the roots of the nonlinear equation $f(x)=0$. For most nonlinear equations, it is difficult for us to find the exact root, so it becomes particularly important to find the approximate root of the equation. Among the methods for solving approximate roots of nonlinear equations, Newton’s iterative method [1], proposed by Newton in the 17th century, is widely used. The iterative format is as follows:

$$x_{n+1}=x_n-\frac{f(x_n)}{f^{\prime }(x_n)}.$$

(1)

Based on Newton’s method, some iterative methods with high computational efficiency and good stability are proposed, for example, the Steffensen iterative method [2], Jarratt-type methods [3], the Ostrowski method [4] and the Chebyshev–Halley iteration method [5]. The Chebyshev–Halley method’s iterative expression is as follows:

$$x_{n+1}=x_n-(1+\frac{L_f(x_n)}{2(1-\alpha L_f(x_n))})\frac{f(x_n)}{f^{\prime }(x_n)},$$

(2)

where $L_f(x)=\frac{f(x)f^{″}(x)}{{f^{\prime }(x)}^2}.$

Some interesting methods have been proposed in recent years in order to improve the computational accuracy and reduce the computational cost of the iterative method. For example, the Legendre spectral collocation method (LSCM) is applied for the solution of the fractional Bratu equation [6], and Nachaoui’s iterative alternating method for solving the Cauchy problem [7]. The Hybrid Jarratt–Butterfly Optimization Algorithm [8] and Hybrid Newton–Sperm Swarm Optimization Algorithm [9] are used to solve nonlinear equations.

Recently, on the basis of the Chebyshev–Halley method, Kou [10] obtained a fifth-order Chebyshev–Halley-type iterative method by using the inverse function method. In addition, Li [11], Chun [12], Kou [13] and Kim [14] proposed some high order Chebyshev–Halley-type iterative methods. In this paper, we proposed a new fifth-order Chebyshev–Halley-type iterative method and analyzed its stability.

In order to intuitively judge the stability of the iterative methods, some authors obtained some preliminary results by using the fractal theory [15,16,17,18,19,20,21,22,23]. Cordero [15] analyzed the stability of third-order Chebyshev–Halley method. In the drawing of the parameter plane and the dynamic plane, Chicharro [16] gives a detailed explanation.

Next, we will present some concepts related to fractal theory [24,25].

Polynomial $M(z)=a_0+a_{1}z+a_2{z}^2+\dots +a_n{z}^n$, where $n\ge 2$, and $M:\mathbb{C}\to \mathbb{C}$. Let $M^k$ be the k-repetition of the function $M\circ \dots \circ M$. $M^k(w)$ is the $kth$ iteration of w. If $M(w)=w$, then w is said to be the fixed point of M; if there is an integer p greater than or equal to 1 that makes $M^p(w)=w$, then w is said to be the periodic point of M; let us call $w,M(w),\dots ,M^p(w)$ the orbital of period p. For $M^{\prime }(w)=\beta$, if $\beta =0$, then point w is superattractive; if $0<|\beta |<1$, then the point w is the attraction point; if $|\beta |=1$, then w is the saddle point; if $|\beta |>1$, then point w is repulsive. The Julia set $J(M)$ of M can be defined as the closure of the set of repulsive periodic points of M, and the remainder of the Julia set is called the Fatou set or the stable set is denoted $F(M)$.

The purpose of this paper is to propose a new iterative method with one parameter and analyze the stability of the strange fixed points associated with the method by using fractal theory. Parameter planes and dynamic planes are used to select the appropriate parameters. The stability and convergence of the iterative method will be analyzed by drawing the fractal diagram of the iterative method.

The structure of this paper is as follows: In Section 2, the convergence order of the Chebyshev–Halley-type iterative method is analyzed. In Section 3, we mapped the iterative method to a rational operator via a Möbius conjugacy map and analyzed its fixed points and critical points. Through the parameter planes related to the critical points, some special parameters are selected and the relevant dynamic planes are drawn. In Section 4, the numerical results of the new method and other methods are compared. In Section 5, fractal diagrams of different iteration methods are drawn. It is proved that the new method has the best stability and convergence when $\alpha =\frac{1}{2}$.

2. Convergence of the New Family

In this section, we propose a two-step fifth-order iterative method based on the Chebyshev–Halley method. The expression of the method is as follows

$$\left\{\begin{array}{c}{y}_n=z_n-[1+\frac{1}{2}\frac{L_f(z_n)}{1-\alpha L_f(z_n)}]\frac{f(z_n)}{f^{\prime }(z_n)},\\ \\ z_{n+1}=y_n-(H_0+H_1{L}_f(z_n))\frac{f(y_n)}{f^{\prime }(z_n)},\end{array}\right.$$

(3)

where

$$L_f(z)=\frac{f(z)f^{″}(z)}{{f^{\prime }(z)}^2},$$

(4)

parameter $\alpha$ is a complex paramater. Then, we further analyze the convergence order of iterative method (3) and give a proof.

Theorem 1. 

Let $\epsilon \in I_f\subset D$ be a simple zero of a real single-valued function $f:D\subset R\to R$ possessing a certain number of continuous derivatives in the neighborhood of $\epsilon \in I_f$, where $I_f$ is an open interval. When iterative method (3) satisfies the condition $H_0=H_1=1$, the iterative method is convergent in the fifth order, and the error expression is as follows:

$$e_{n+1}=(2(6-6\alpha )c_2^4+(-12+6\alpha )c_2^2{c}_3+3c_3^2)e_n^5+O(e_n^6),$$

(5)

which holds.

Proof. 

Let $c_n=\frac{f^{(n)}(\epsilon )}{n!f^{\prime }(\epsilon )}$, $e_n=z_n-\epsilon ,e_y=y_n-\epsilon$, and $e_{n+1}=z_{n+1}-\epsilon .$ Expanding f by Taylor’s series about $\epsilon$, we find

$$f(z_n)=f^{\prime }(\epsilon )(e_n+c_2{e_n}^2+c_3{e_n}^3+c_4{e_n}^4+c_5{e_n}^5+c_6{e_n}^6+O(e_n^7)),$$

(6)

$$f^{\prime }(z_n)=f^{\prime }(\epsilon )(1+2c_2{e}_n+3c_3{e_n}^2+4c_4{e_n}^3+5c_5{e_n}^4+6c_6{e_n}^5+O(e_n^6)),$$

(7)

$$f^{″}(z_n)=f^{\prime }(\epsilon )(2c_2+6c_3{e}_n+12c_4{e_n}^2+20c_5{e_n}^3+30c_6{e_n}^4+O(e_n^5)),$$

(8)

Putting (6)–(8) into (4), we obtain

$$\begin{array}{cc}\hfill L_f(z_n)=& 2c_2{e}_n+(-6c_2^2+6c_3)e_n^2+4(4c_2^3-7c_2{c}_3+3c_4)e_n^3-10(4c_2^4-10c_2^2{c}_3+3c_3^2\hfill \\ \hfill & +5c_2{c}_4)e_n^4+6(16c_2^5-52c_2^3{c}_3+33c_2{c}_3^2+28c_2^2{c}_4-17c_3{c}_4-13c_2{c}_5+5c_6)e_n^5\hfill \\ \hfill & -14(16c_2^6-64c_2^4{c}_3-9c_3^3+36c_2^3{c}_4+6c_4^2+9c_2^2(7c_3^2-2c_5)\hfill \\ \hfill & +11c_3{c}_5+c_2(-46c_3{c}_4+8c_6))e_n^6+O({e_n}^7),\hfill \end{array}$$

(9)

and then,

$$\begin{array}{cc}\hfill e_y=& (2{c_2}^2-2\alpha {c_2}^2-c_3){e_n}^3+(-9{c_2}^3+14\alpha {c_2}^3-4{\alpha }^2{c_2}^3+12c_2{c}_3-12\alpha c_2{c}_3-3c_4){e_n}^4\hfill \\ \hfill & +((30-66\alpha +40{\alpha }^2-8{\alpha }^3)c_2^4-9(7-12\alpha +4{\alpha }^2)c_2^2{c}_3-3(-5+6\alpha )c_3^2\hfill \\ \hfill & -24(-1+\alpha )c_2{c}_4-6c_5)e_n^5+(-2(44-129\alpha +124{\alpha }^2-52{\alpha }^3+8{\alpha }^4)c_2^5\hfill \\ \hfill & +(251-618\alpha +428{\alpha }^2-96{\alpha }^3)c_2^3{c}_3-2(56-101\alpha +36{\alpha }^2)c_2^2{c}_4\hfill \\ \hfill & +(55-72\alpha )c_3{c}_4-2c_2((68-135\alpha +54{\alpha }^2)c_3^2+20(-1+\alpha )c_5)\hfill \\ \hfill & -10c_6)e_n^6+O({e_n}^7),\hfill \end{array}$$

(10)

Hence, applying Taylor’s series again, we have

$$f(y_n)=f^{\prime }(\epsilon )(e_y+c_2{e_y}^2+c_3{e_y}^3+c_4{e_y}^4+c_5{e_y}^5+c_6{e_y}^6+O({e_y}^7)),$$

(11)

Now, using (9)–(11) we obtain

$$\begin{array}{cc}\hfill e_{n+1}=& (2(-1+\alpha )c_2^2+c_3)(-1+H_0)e_n^3+(3c_4(-1+H_0)+2c_2{c}_3(6+6\alpha (-1+H_0)-7H_0+H_1)\hfill \\ \hfill & +c_2^3(-9+4{\alpha }^2(-1+H_0)+13H_0-4H_1+\alpha (14-18H_0+4H_1)))e_n^4+(c_5(-6+5H_0)\hfill \\ \hfill & +6c_2{c}_4(4+4a(-1+H_0)-5H_0+H_1)+3c_3^2(5+6a(-1+H_0)-6H_0+2H_1)\hfill \\ \hfill & +c_2^2{c}_3(-63+36{\alpha }^2(-1+H_0)+97H_0-138a{H}_0-46H_1+36\alpha (3+H_1))\hfill \\ \hfill & +2c_2^4(15+4{\alpha }^3(-1+H_0)-28H_0+3\alpha (-11+17H_0-8H_1)+19H_1\hfill \\ \hfill & +4{\alpha }^2(5-6H_0+H_1)))e_n^5+O({e_n}^6).\hfill \end{array}$$

(12)

From the above expression, if the coefficients of $e_n^3$ and $e_n^4$ are zero, then the iterative method is a fifth-order convergence; that is, when $H_0=H_1=1$, the error expression becomes

$$e_{n+1}=(2(6-6\alpha )c_2^4+(-12+6\alpha )c_2^2{c}_3+3c_3^2)e_n^5+O(e_n^6),$$

(13)

Then it is obvious that method (3) is a fifth-order convergence. □

In this case, the format of the obtained fifth-order iterative method is

$$\left\{\begin{array}{c}{y}_n=z_n-[1+\frac{1}{2}\frac{L_f(z_n)}{1-\alpha L_f(z_n)}]\frac{f(z_n)}{f^{\prime }(z_n)},\\ \\ z_{n+1}=y_n-(1+L_f(z_n))\frac{f(y_n)}{f^{\prime }(z_n)},\end{array}\right.$$

(14)

where

$$L_f(z)=\frac{f(z)f^{″}(z)}{{f^{\prime }(z)}^2}.$$

(15)

3. Complex Dynamics Behavior

Complex dynamics mainly studies the dynamic properties of the rational function related to the iterative method. The stability and reliability of the dynamic characteristics of rational operators can be analyzed by studying the dynamic behavior of fixed points. The parameter space established from the critical point also allows us to understand the stability of different elements of the method, so that we can choose more suitable and reliable family members.

In this section, we study the complex dynamics of method (14). For this purpose, we construct a family correlated rational operator on a general nonlinear polynomial of low order, and analyze the stability of its fixed points and critical points. Then, we construct some parameter planes based on the free critical points. Some iterative methods corresponding to parameter values with good stability are selected to construct the corresponding dynamic plane.

3.1. Rational Operator

We will now analyze the dynamics of this method applied to quadratic polynomials. We know that the roots of polynomials can be transformed by affine mapping without any qualitative change in the dynamics. Therefore, by bringing the quadratic polynomial $p(z)=(z-a)(z-b)$ into the new two-step Chebyshev–Halley-type method, the corresponding rational function can be obtained:

$$H_p(z;\alpha ,a,b)=\frac{\kappa (z)}{\rho (z)},$$

(16)

where $\kappa (z)={(a-z)}^3{(-b+z)}^3(-b+2a(-1+a-z)+3z)(a+2b-2ab-3z+2az)(a^2+4ab+b^2-6(a+b)z+6z^2)+{(a+b-2z)}^{5}z{({(b-2z)}^2+2ab(1+z)-2az(2+z)+a^2(1-2b+2z))}^2+{(a+b-2z)}^4(-a+z)(-b+z)({(b-2z)}^2+2ab(1+z)-2az(2+z)+a^2(1-2b+2z))(b^2-5bz+5z^2+ab(3+2z)-az(5+2z)+a^2(1-2b+2z))$ and $\rho (z)=({(a+b-2z)}^5{({(b-2z)}^2+2ab(1+z)-2az(2+z)+a^2(1-2b+2z))}^2)$, depending on the parameters $\alpha$, a and b. Next, we consider the conjugacy map

$$m(z)=\frac{z-a}{z-b},$$

(17)

with the following properties:

$$(i)m(\infty )=1,(ii)m(a)=0,(iii)m(b)=\infty .$$

(18)

It can be obtained that the fixed point operator

$$\begin{array}{cc}\hfill O_p(z;\alpha )& =(m\circ H_p\circ m^{-1})(z)\hfill \\ \hfill & =(z^5(12-12\alpha +(42-56\alpha +20{\alpha }^2)z+(48-56\alpha +16{\alpha }^2)z^2+(27-24\alpha +4{\alpha }^2)z^3\hfill \\ \hfill & +(8-4\alpha )z^4+z^5))/(1-4(-2+\alpha )z+(27-24\alpha +4{\alpha }^2)z^2+8(6-7\alpha +2{\alpha }^2)z^3\hfill \\ \hfill & +(42-56\alpha +20{\alpha }^2)z^4-12(-1+\alpha )z^5)\hfill \end{array}$$

(19)

where $O_p(z;\alpha )$ is only related to $\alpha$. In addition, we know that $H_p(z)$ and $O_p(z)$ are conjugate, the obtained $O_p(z)$ contains only parameter $\alpha$ and the form of $O_p(z)$ depends on the value of parameter $\alpha$, so we only need to study one parameter, which greatly simplifies our research below. Furthermore, by factorizing the numerator and denominator of $O_p(z;\alpha )$, we can observe that the expression for $O_p(z;\alpha )$ is further simplified when $\alpha$ takes different values, such as $-1,\frac{1}{2}$:

$$O_p(z;-1)=\frac{z^5(24+22z+8z^2+z^3)}{1+8z+22z^2+24z^3},$$

(20)

and

$$O_p(z;\frac{1}{2})=\frac{z^5(6+7z+4z^2+z^3)}{1+4z+7z^2+6z^3}.$$

(21)

3.2. Analysis and Stability of Fixed Points

We will calculate the fixed points of the operators $O_p(z;\alpha )$ mentioned earlier and analyze their stability. As we will see, the number of fixed points and their stability depend on the value of the parameter $\alpha$. According to the definition of fixed point $O_p(z)=z$, we obtain:

$$O_p(z)-z=\frac{z(z-1)\chi (z)}{\psi (z)},$$

(22)

where

$$\begin{array}{cc}\hfill \chi (z)=& 1+(9-4\alpha )z+(36-28\alpha +4{\alpha }^2)z^2+(84-84\alpha +20{\alpha }^2)z^3+(114-128\alpha +40{\alpha }^2)z^4\hfill \\ \hfill & +(84-84\alpha +20{\alpha }^2)z^5+(36-28\alpha +4{\alpha }^2)z^6+(9-4\alpha )z^7+z^8,\hfill \end{array}$$

(23)

and

$$\begin{array}{cc}\hfill \psi (z)=& 1-4(-2+\alpha )z+(27-24\alpha +4{\alpha }^2)z^2+8(6-7\alpha +2{\alpha }^2)z^3+(42-56\alpha +20{\alpha }^2)z^4\hfill \\ \hfill & -12(-1+\alpha )z^5.\hfill \end{array}$$

(24)

From $O_p(z)-z=0$, we can see that the fixed points are $z=0,z=\infty ,z=1$ and the root of the polynomial $\chi (z)=1+(9-4\alpha )z+(36-28\alpha +4{\alpha }^2)z^2+(84-84\alpha +20{\alpha }^2)z^3+(114-128\alpha +40{\alpha }^2)z^4+(84-84\alpha +20{\alpha }^2)z^5+(36-28\alpha +4{\alpha }^2)z^6+(9-4\alpha )z^7+z^8$. It is easy to see that $z=0,z=\infty$ are free points of the parameter $\alpha$. In addition, they are super-attractive fixed points. Next, we will analyze the case of strange fixed points.

Since the form of the expression of $O_p(z)$ depends on the value of the parameter $\alpha$, the following theorem is given for the number of strange fixed points under different parameter values.

Theorem 2. 

•

If $\alpha =\frac{1}{2}$, the polynomials $\chi (z)$ and $\psi (z)$ have common factors ${(z+1)}^2$, in which case the operator $O_p(z)$ has seven strange fixed points $z=1,z=-2.39258,z=-0.41796,z=-0.86623\pm 1.74372i,z=-0.228502\pm 0.459974i$.

•

If $\alpha =-1$, the operator $O_p(z)$ has six strange fixed points $z=1,z=-4.51786,z=-0.221344,z=-1.89002\pm 2.07137i,z=-0.240376\pm 0.263441i$.

•

If α satisfies $(\alpha -\frac{1}{2})(\alpha +1)\ne 0$, the operator $O_p(z)$ has nine strange fixed points: $z=1$ and eight roots of the polynomial $\chi (z)=0$.

Proof. 

•

Suppose that $z\in C$ are some values of $\chi (z)=0$ and $\psi (z)=0$; by concatenating the two polynomial equations, we can eliminate the parameter $\alpha$, at which time we obtain ${(1+z)}^5=0$, from which we know that $(1+z)$ can be a common factor of $\chi (z)$ and $\psi (z)$. So, if we put $z=-1$ into $\chi (z;-1)$ and $\psi (z;-1)$, we obtain $\alpha =\frac{1}{2}$. Now operator $O_p(z,\frac{1}{2})$ has seven strange fixed points.

•

The remaining cases are obviously as shown in the above theorem.

□

Next, we will analyze the stability of strange fixed points. To study that, first we need to figure out the first derivative of the operator $O_p(z;\alpha )$:

$$O_p^{\prime }(z;\alpha )=-\frac{-4(z^4){(1+z)}^4\lambda (z)\omega (z)}{{\mu (z)}^2},$$

(25)

where

$$\lambda (z)=1+2z-2\alpha z+z^2$$

(26)

$$\begin{array}{cc}\hfill \omega (z)=& -15+15\alpha +(-69+108\alpha -48{\alpha }^2)z+(-108+196\alpha -136{\alpha }^2+40{\alpha }^3)z^2\hfill \\ \hfill & +(-69+108\alpha -48{\alpha }^2)z^3+(-15+15\alpha )z^4,\hfill \end{array}$$

(27)

$$\begin{array}{cc}\hfill \mu (z)=& -1+4(-2+\alpha )z+(-27+24\alpha -4{\alpha }^2)z^2-8(6-7\alpha +2{\alpha }^2)z^3+(-42+56\alpha -20{\alpha }^2)z^4\hfill \\ \hfill & +12(-1+\alpha )z^5.\hfill \end{array}$$

(28)

Obviously, the stability of the fixed point also depends on the value of the parameter $\alpha$. The following content will give the stability of the fixed point obtained above.

Proposition 1. 

The strange fixed points we know are $z=1$ and the root of polynomial $\chi (z)=1+(9-4\alpha )z+(36-28\alpha +4{\alpha }^2)z^2+(84-84\alpha +20{\alpha }^2)z^3+(114-128\alpha +40{\alpha }^2)z^4+(84-84\alpha +20{\alpha }^2)z^5+(36-28\alpha +4{\alpha }^2)z^6+(9-4\alpha )z^7+z^8$. We define the root of $\chi (z)$ as ${\chi }_i(z),i=1,2,\dots ,8$ for α satisfying $(z-\frac{1}{2})(z+1)\ne 0$,

•

$z=1$, taking parameter values in the region $[1.7,2.3]\times [-0.3,0.3]$ of the complex plane is an attractor. And z = 1 is a superattractive fixed point for $a=2$; $z=1$ is a hyperbolic fixed point for $\alpha =2.04545\pm 0.25713i$ and $\alpha =1.5,\alpha =2.3$.

•

${\chi }_1(z),{\chi }_3(z),{\chi }_4(z),{\chi }_5(z),{\chi }_8(z)$ are repulsive, with independence of the value of parameter α.

•

${\chi }_2(z)$ is an attractor for values of α in small regions of the complex plane, inside the complex area $[-0.58,-0.56]\times [0.96,0.98]$.

•

${\chi }_{6,7}(z)$ is an attractor for values of α in small regions of the complex plane, inside the complex area $[1.5,2.5]\times [-0.5,0.5]$.

Figure 1 and Figure 2 show the stability of the strange fixed point of the operator $O_p(z;\alpha )$.

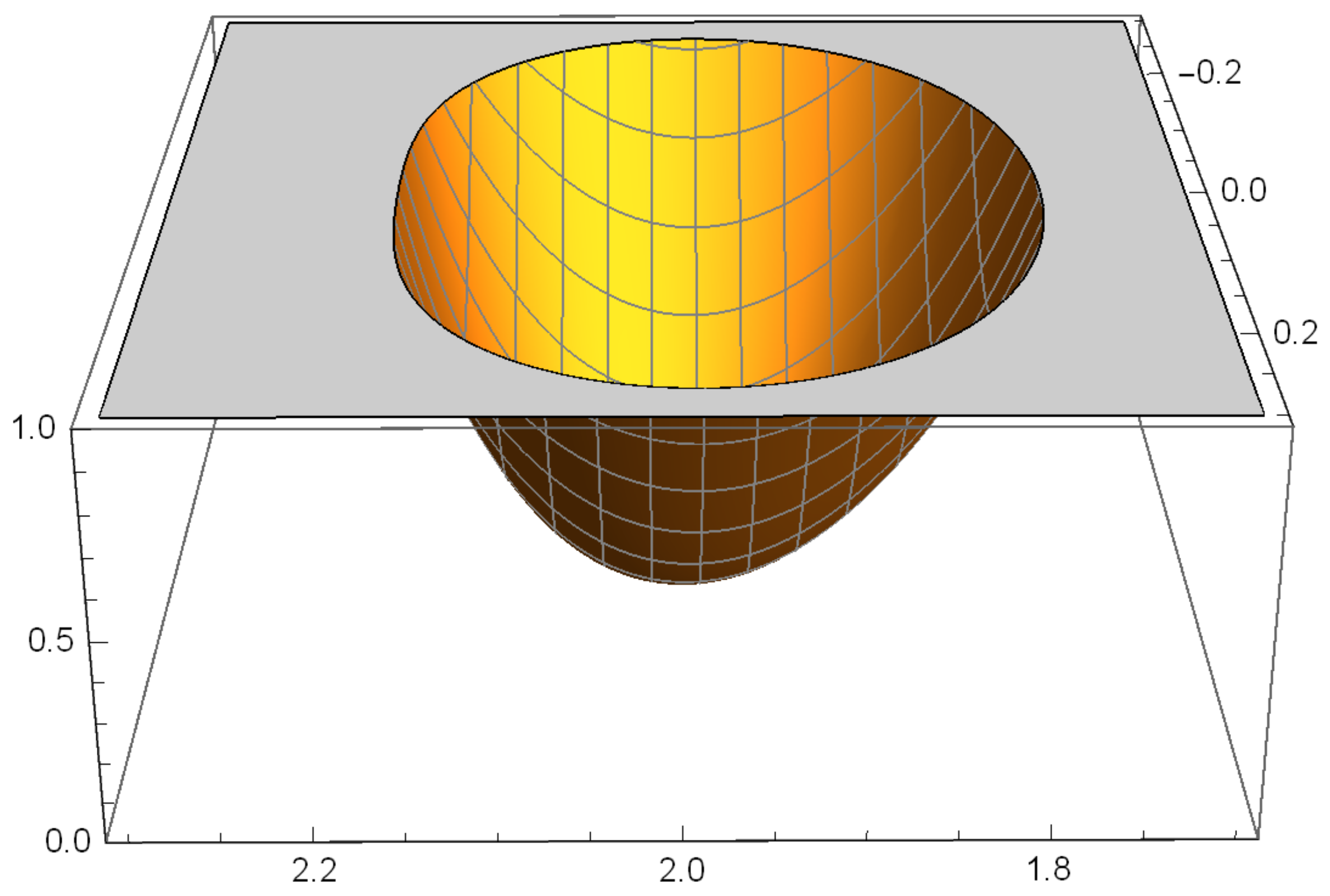
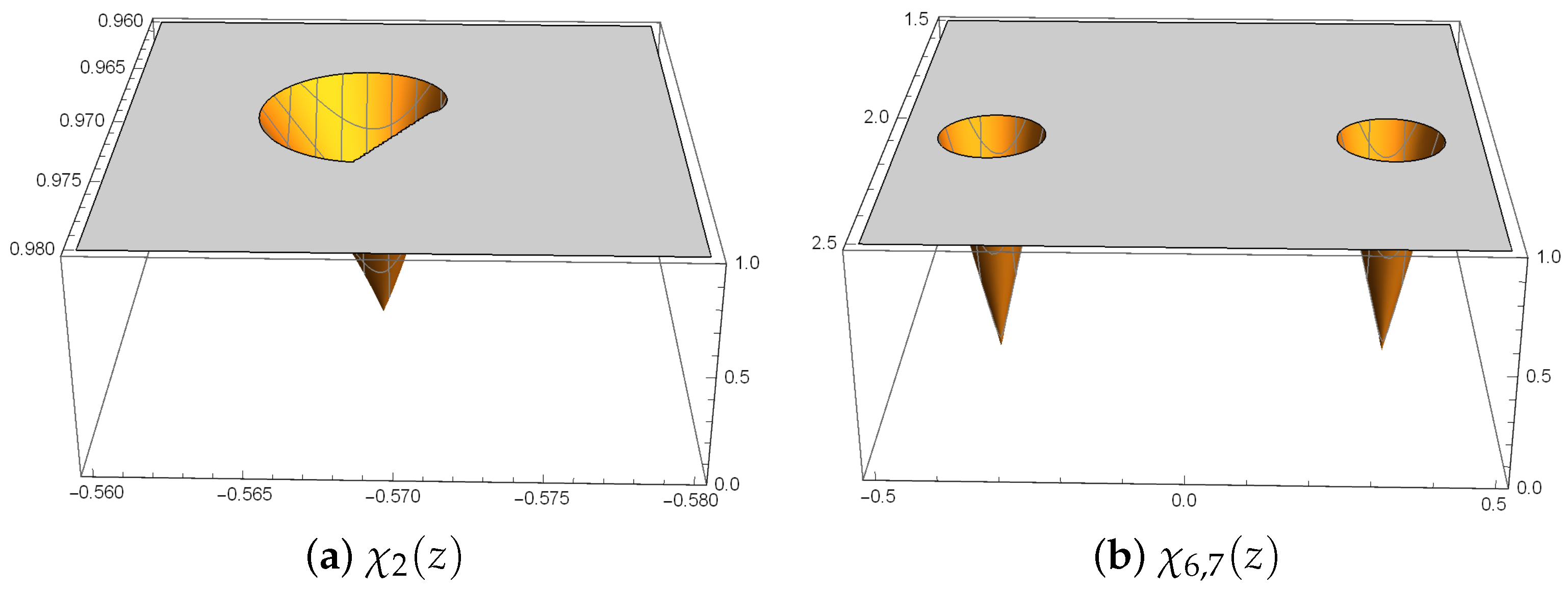
3.3. Critical Points of Operator $Op(z;\alpha )$

According to the definition of critical points, we can find the necessary critical points by taking the root of $O_p^{\prime }(z;\alpha )=0$. Thus, it is clear that 0, 1 are critical points of the operator $Op(z;\alpha )$, and that they depend on the roots of the quadratic polynomial $p(z)=(z-a)(z-b)$. In addition, the remaining critical points are called free critical points. The following theorem summarizes the number of free critical points when $\alpha$ takes different values.

Theorem 3. 

With $O_p^{\prime }(z;\alpha )=0$, we obtain $z=-1$ and the roots of $\lambda (z)=0,\omega (z)=0$ are the free critical points. So

•

If $\alpha =0,$$\omega (z)=3{(1+z)}^2(5+13z+5z^2)$, then $O_p(z;\alpha )$ has three different free critical points $z=-1$ (with multiplicity 8), $z=-2.13066,z=-0.469338$. If $\alpha =2$, $\omega (z)=15{(-1+z)}^2(1-z+z^2)$, then $O_p(z;\alpha )$ has four different free critical points $z=-1$ (with multiplicity 4), $z=1$(with multiplicity 4), $z=0.5\pm 0.866025i$.

•

If $\alpha =-1$, $\omega (z)=15(2+7z+2z^2)$, then $O_p(z;\alpha )$ has three different free critical points $z=-1$ (with multiplicity 4), $z=-3.18614,z=-0.313859$.

•

If $\alpha =\frac{1}{2}$, $\omega (z)=3(5+8z+5z^2)$, $O_p(z;\alpha )$ has five different free critical points $z=-1$ (with multiplicity 2), $z=0.5\pm 0.866025i,z=-0.8\pm 0.6i$. If $\alpha =-\frac{1}{2}$, there are six different free critical points $z=-1,z=-3,z=-2.61803,z=-0.381966,z=-2.21525,z=-0.451416$.

•

If $\alpha =\frac{3}{2}$, there are three different free critical points $z=-1$ (with multiplicity 4), $z=\pm i,z=0.5\pm 0.866025i$; and with $\alpha =\frac{23}{10}$, there are five different free critical points $z=-1$ (with multiplicity 4), $z=0.469338,z=2.13066,z=0.910769\pm 0.412916i$.

•

For $\alpha (\alpha -2)(\alpha \pm \frac{1}{2})(\alpha -\frac{3}{2})(\alpha -\frac{23}{10})(\alpha +1)\ne 0$, there are seven different free critical points

$c{r}_1=-1;$

$c{r}_2=-1+\alpha -\sqrt{-2\alpha +{\alpha }^2};$

$c{r}_3=-1+\alpha +\sqrt{-2\alpha +{\alpha }^2};$

$c{r}_4=\frac{1}{60}(\Delta -\sqrt{6}\sqrt{\frac{1}{{\tau }^3}(\Gamma -\Lambda -\Theta +{\rm Y})};$

$c{r}_5=\frac{1}{60}(\Delta +\sqrt{6}\sqrt{\frac{1}{{\tau }^3}(\Gamma -\Lambda -\Theta +{\rm Y})};$

$c{r}_6=\frac{1}{60}(\Delta -\sqrt{6}\sqrt{\frac{1}{{\tau }^3}(\Gamma +\Lambda +\Theta +{\rm Y})};$

$c{r}_7=\frac{1}{60}(\Delta +\sqrt{6}\sqrt{\frac{1}{{\tau }^3}(\Gamma +\Lambda +\Theta +{\rm Y})};$

where $\tau =\alpha -1;\xi =\sqrt{27-88\alpha +56{\alpha }^2+64{\alpha }^3-32{\alpha }^4};\Delta =\frac{69-108\alpha +48{\alpha }^2-\sqrt{3}\xi }{\tau };\Gamma =(4172{\alpha }^3-2064{\alpha }^4+368{\alpha }^5;\Lambda =23\sqrt{9+\sqrt{3}\tau \xi };\Theta =4{\alpha }^2(951+4\sqrt{3}\tau \xi );{\rm Y}=\alpha (1535+36\sqrt{3}\tau \xi ).$ Moreover, it can be proved that all free critical points are not independent, as $c{r}_2=\frac{1}{c{r}_3};c{r}_4=\frac{1}{c{r}_5};c{r}_6=\frac{1}{c{r}_7}.$

Proof. 

For $1,2$, we already know $\lambda (z)=1+(2-2\alpha )z+z^2$; then let $\lambda (z)=0$; we can obtain the expression of the critical points $c{r}_i=-1+a\pm \sqrt{-2\alpha +{\alpha }^2},i=1,2$, and $c{r}_1=\frac{1}{c{r}_2}$. So we can start with a special case, which is $c{r}_1=c{r}_2$. At this time, $c{r}_1=c{r}_2=\pm 1$; that is ${\alpha }^2-2\alpha =0$; solving the equation, we obtain $\alpha =0$, $\alpha =2$. Therefore, when $c{r}_1=c{r}_2=-1$, $O_p(z;\alpha )$ has three different free critical points. When $c{r}_1=c{r}_2=1$, $O_p(z;\alpha )$ has four different free critical points.

And for $3,4$, suppose that $z\in C$ are some values of $\omega (z)=0$ and $\mu (z)=0$; by concatenating the two polynomial equations, we can eliminate the parameter $\alpha$, at which time we obtain ${((1+z)(1+3z))}^4{(1+11z+45z^2+71z^3-23z^4-189z^5-153z^6+69z^7+138z^8+30z^9)}^2=0$, from which we know that $z+1$ and $1+3z$ can be the common factor of $\omega (z)$ and $\mu (z)$. Therefore, we put $z=-1$ into $\omega (z)$ and $\mu (z)$; we obtain $\alpha =\frac{1}{2}$; this time, $\frac{\omega (z)}{\mu (z)}=-\frac{3(5+8z+5z^2)}{2{(1+z)}^2{(1+4z+7z^2+6z^3)}^2}$; there are five different critical points. Therefore, we also obtain $\alpha =\frac{3}{2}$ and $\alpha =\frac{23}{10}$; this time, $O^{\prime }p(z)=-\frac{30z^4{(1+z)}^4(1+z^2)(1-z+z^2)}{{(1+3z+3z^2+3z^3+6z^4)}^2}$ and $O^{\prime }p(z)=-\frac{30z^4{(1+z)}^4(5-13z+5z^2)(325-592z+325z^2)}{{(-25+5z+181z^2+85z^3-390z^4)}^2}$; there are five different critical points. Then, we put $z=\frac{1}{3}$ into $\omega (z)$ and $\mu (z)$; we obtain $\alpha =-\frac{1}{2}$; this time, $O^{\prime }p(z)=(10z^4{(1+z)}^4(1+3z+z^2)(9+27z+17z^2+3z^3))/({(1+3z)}^3{(1+4z+7z^2+2z^3)}^2)$; there are six different critical points. □

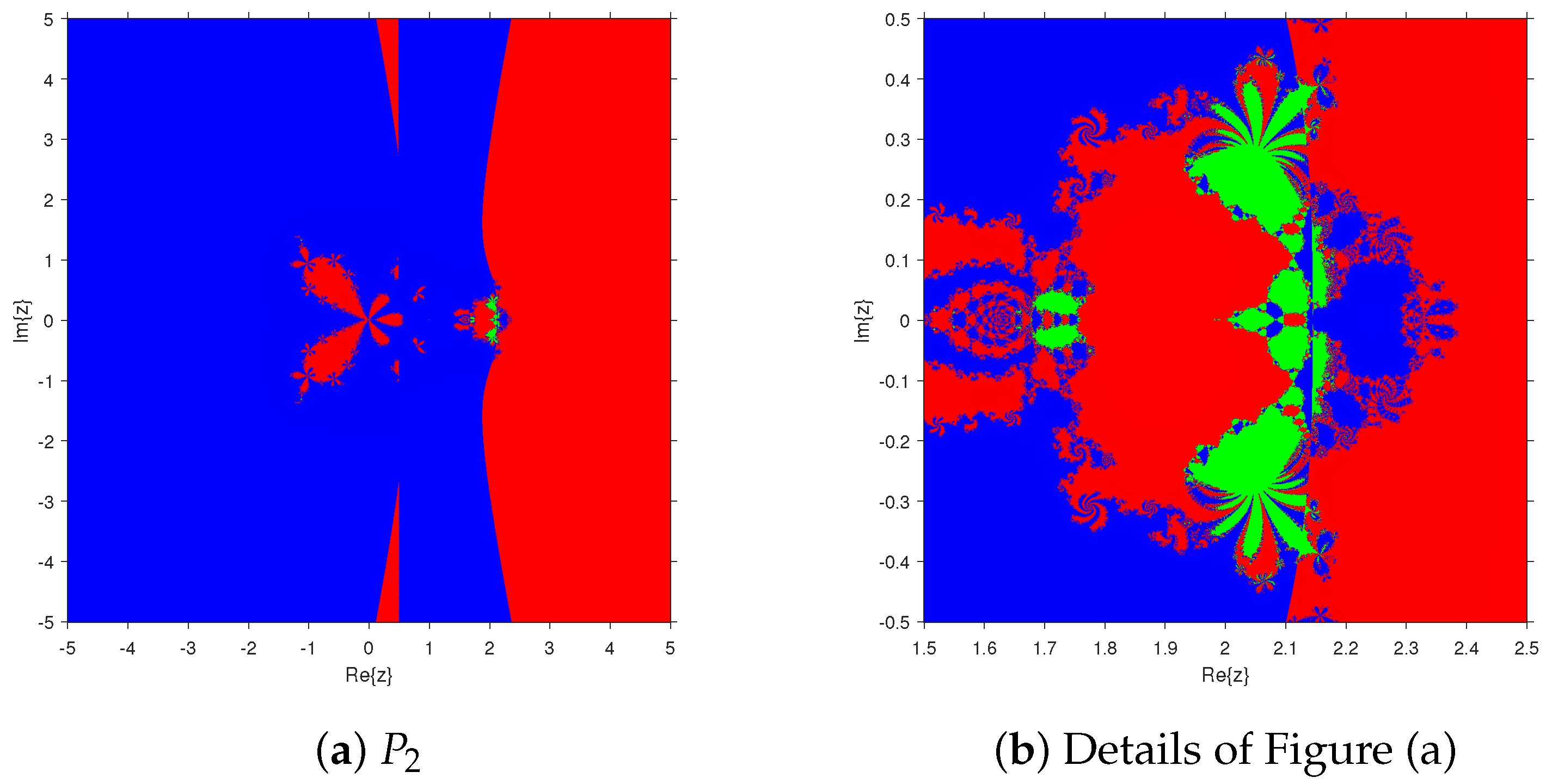
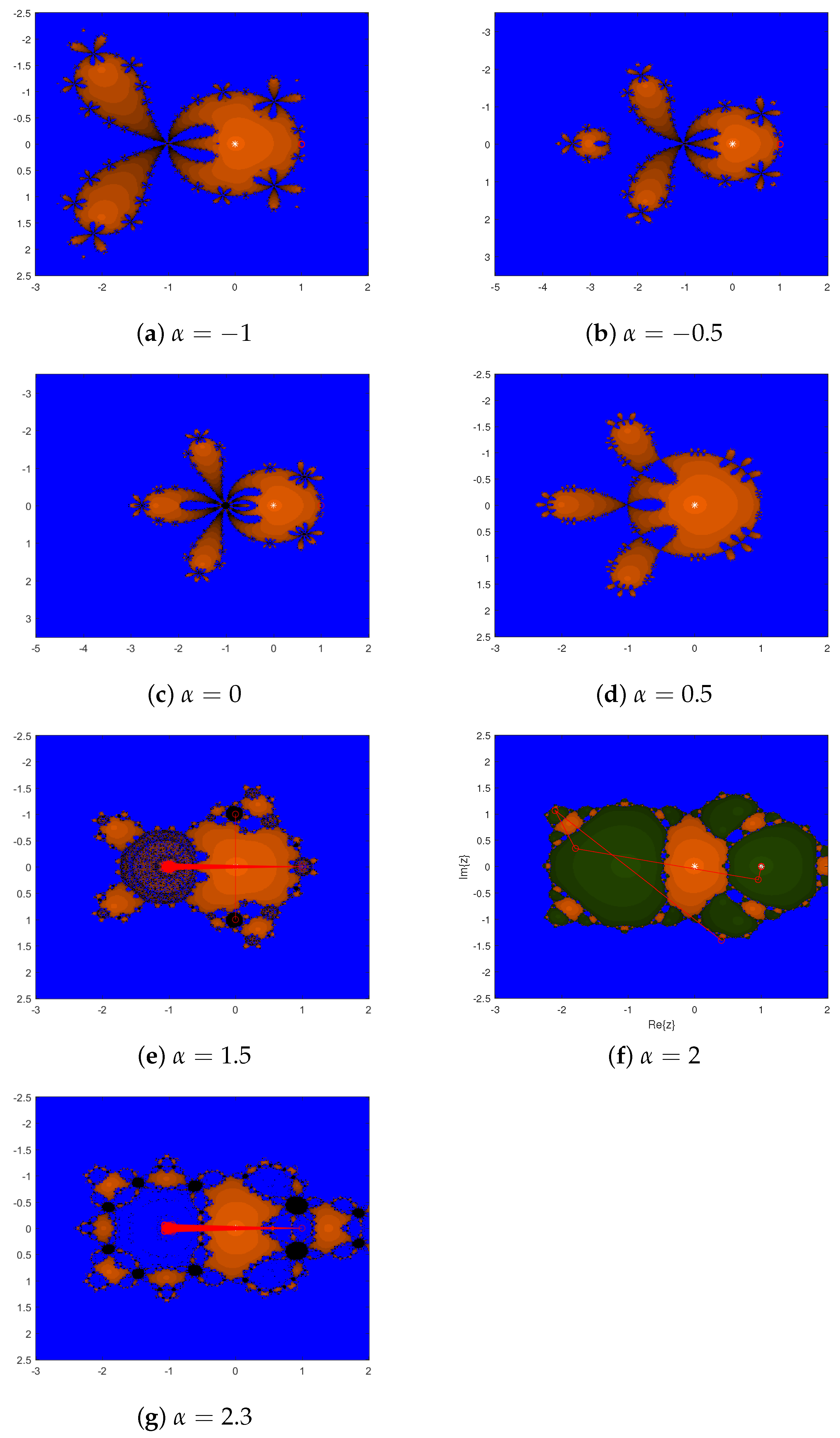
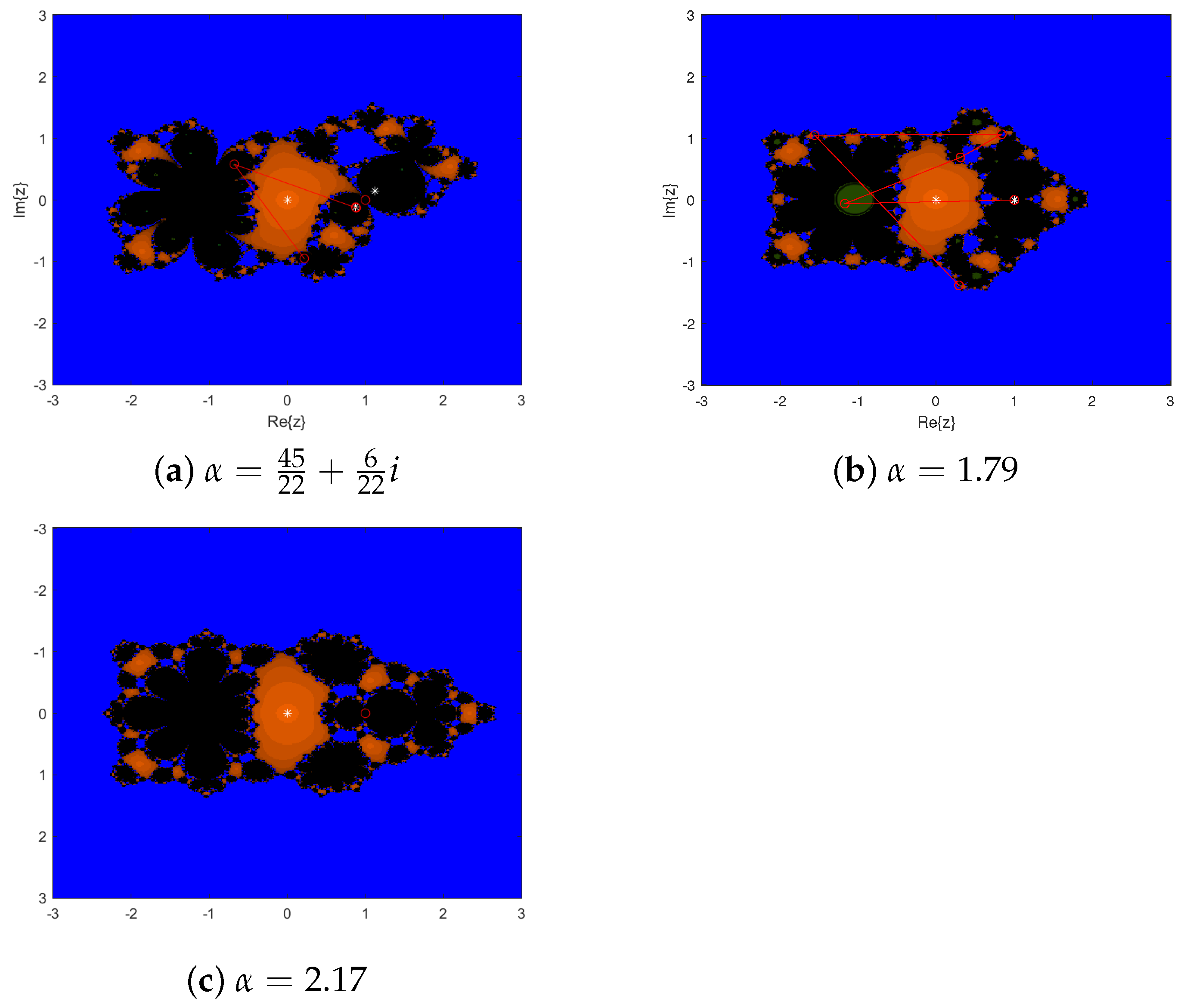
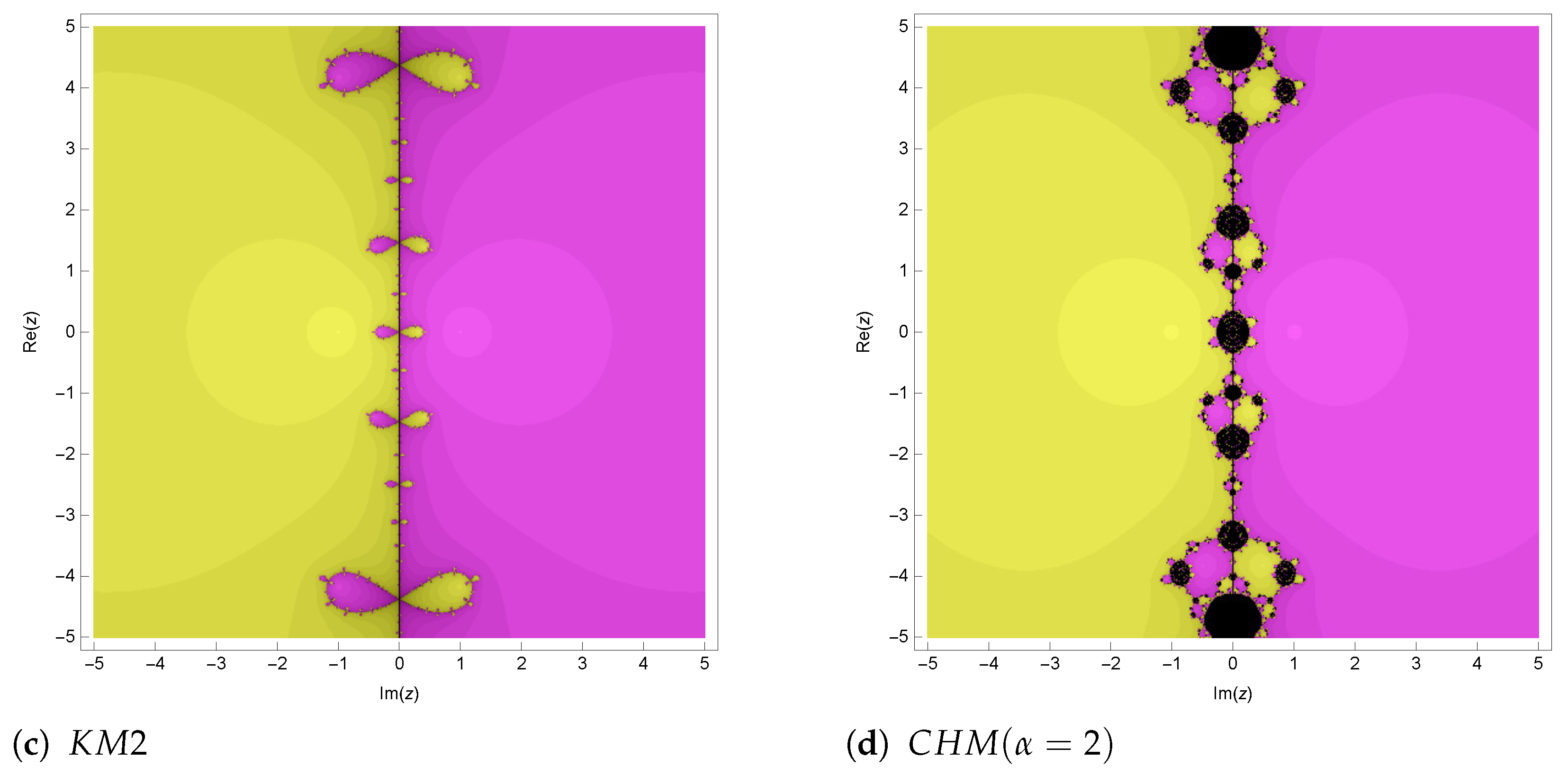
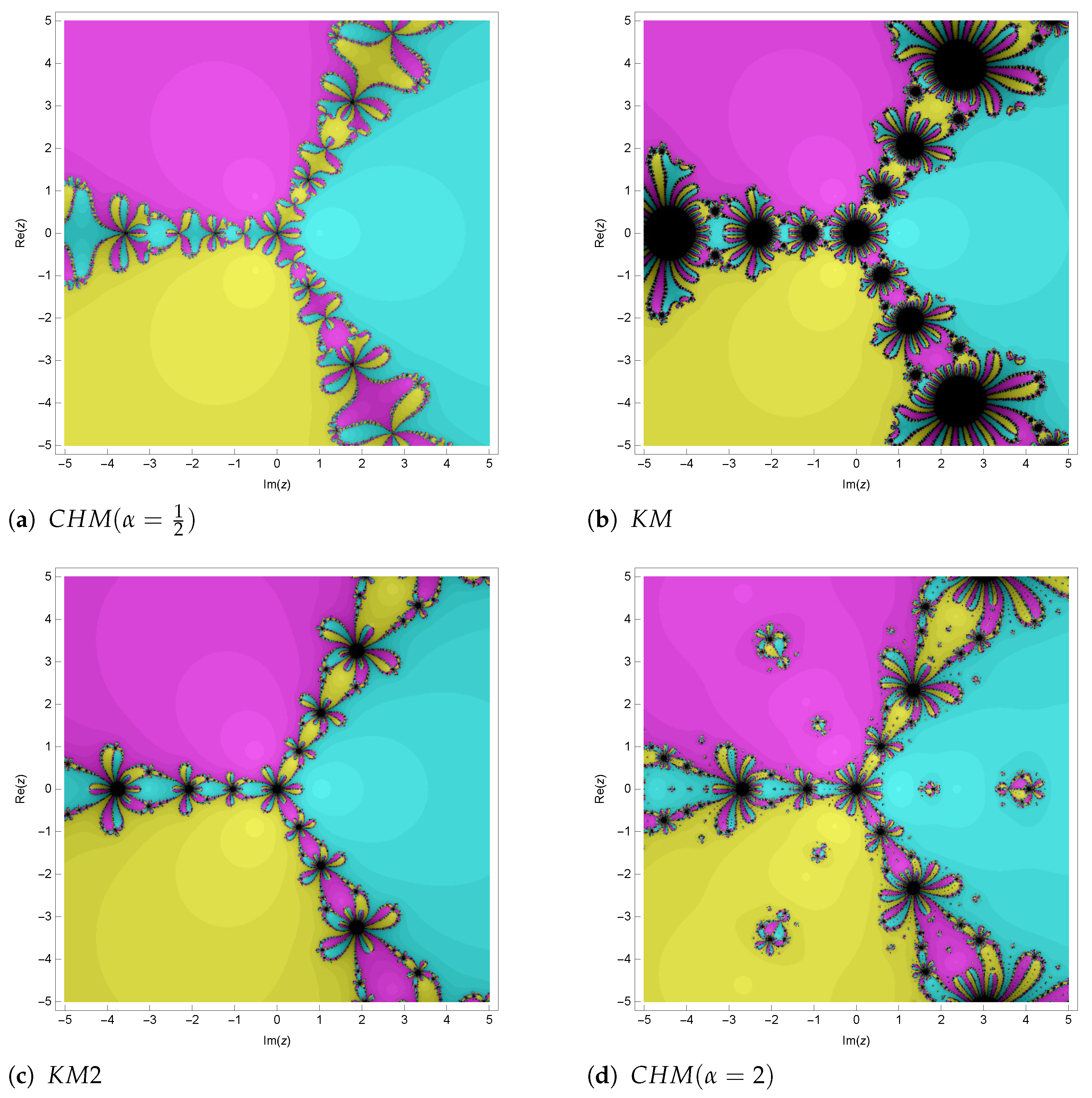
3.4. Parameter Spaces and Dynamical Planes

In the above analysis, we can clearly understand that the dynamic behavior of the corresponding rational operators will also be different in the case of different parameters. In the following, we will plot the dynamic plane D and the parameter plane P to understand the dynamic behavior of the iterative method in this paper at a glance. On the basis of drawing the parameter plane, every value of $\alpha$ belonging to the same connected component of the parameter space gives rise to subsets of schemes of method (14) with similar dynamical behavior. In this way, we can find some regions with good stability in the parameter plane, so we can obtain the stable members of the family of iterative method (14).

$P=\alpha \in \mathbb{C}$: an orbit of a free critical point $cr$ tends to a number ${\delta }_p\in \tilde{C}$ under the action of $O_p(z;\alpha )$.

$D=z\in \mathbb{C}$: an orbit of $z(\alpha )$ for a given $\alpha \in P$ tends to a number ${\delta }_d\in \tilde{C}$ under the action of $O_p(z;\alpha )$.

3.4.1. Parameter Spaces

From Theorem 3, we know that in general, we have at most four independent free critical points. Moreover, we know that $z=-1$ is the preimage of the fixed point $z=1$, and the parameter plane corresponding to this critical point is not significant. Thus, we can obtain three different parameter planes with complementary information.

When we consider the free critical point $c{r}_2$ (or $c{r}_3$) as the initial point of the iterative method for the family associated with each complex value, if the method converges to zero, we color this point in the complex plane red, if the method converges to zero, we color this point in the complex plane blue, in other cases they are green. Figure 3 shows the parameter plane when the initial point is $c{r}_2$. This figure has been generated for values of $\alpha$ in $[-500,500]\times [-500,500]$, with a mesh of $1000\times 1000$ points and 50 iterations per point. Figure 3b shows the situation in greater detail in Figure 3a. If the parameter plane is drawn with $c{r}_{4,5}$ and $c{r}_{6,7}$ as the initial points, $P_2$ and $P_3$ can be obtained, as shown in Figure 4 and Figure 5, respectively. In Figure 3 and Figure 4, we can see red areas that converge to 0, blue areas that converge to infinity and green areas that converge to nothing. In Figure 5, only red and blue appear. Therefore, the iterative method with $c{r}_{6,7}$ as the initial points has better stability. In addition, all the iterative methods corresponding to the green parameter values are unstable, so we should avoid the green parameter values as much as possible in the final parameter selection, and choose the red or blue parameter values.

3.4.2. Dynamical Planes

The dynamic planes of the new iterative method (14) are also dependent on the parameter $\alpha$, where each region is drawn in a different color. If initial points converge to 0 and infinity, they are orange and blue, respectively. If initial points converge to the fixed point $z=1$, they are green; if initial points converge to black, they do not converge to any roots. Other than that, we set the track to red. These dynamic planes were generated with a mesh of $400\times 400$ points and up to 20 iterations per point.

In Figure 6, the parameter planes corresponding to some special parameter values are plotted. When $\alpha =\frac{1}{2}$, there are only blue and orange regions in Figure 6d, this means that the initial points only converge to 0 and infinity; this parameter value is ideal. When $\alpha =2$, $z=1$ is a superattractive fixed point, and the green area appears in Figure 6f, this means that this additional fixed point is convergent. When $\alpha =-1,\alpha =-\frac{1}{2},\alpha =0,\alpha =\frac{3}{2},\alpha =\frac{23}{10}$, some black areas appear in Figure 6; the initial values in the black area do not converge to any root, which means that these parameter values are not ideal.

When $\alpha =\frac{45}{22}+\frac{4\sqrt{2}}{22}i$ and $\alpha =\frac{25}{14},\alpha =\frac{13}{6}$, $z=1$ is a hyperbolic point, and the corresponding dynamic planes are shown in Figure 7. In Figure 7, we can observe that black regions appear in each graph, indicating that they all have regions that do not converge to any root at this time. So the value of this parameter is not what we want it to be. A similar situation appears in Figure 8.

Based on the above, we can find that when $\alpha =-1,\alpha =-\frac{1}{2},\alpha =\frac{1}{2}$, the dynamic planes are relatively simple; when the iterative method takes these parameter values, the iterative methods are relatively stable.

4. Numerical Experiments

Here, we perform several numerical tests in order to check the theoretical convergence and stability results of the CHM($\alpha$) (14) family obtained in previous sections. Our method CHM($alpha=\frac{1}{2}$) (14) was compared with Kou’s method (KM) and Li’s method (LIM) [11] to solve some nonlinear equations.

Kou’s method [10]

$$\left\{\begin{array}{c}{y}_n=z_n-[1+\frac{1}{2}\frac{L_f(z_n)}{1-{\beta }_1{L}_f(z_n)}]\frac{f(z_n)}{f^{\prime }(z_n)},\\ \\ z_{n+1}=y_n-(1+\frac{M_f(z_n,y_n)}{(1-\gamma M_f(z_n,y_n))})\frac{f(y_n)}{f^{\prime }(z_n)},\end{array}\right.$$

(29)

where $L_f=\frac{f(z)f^{″}(z)}{f^{\prime }{(z)}^2},M_f(z_n,y_n)=\frac{f^{″}(z_n)(f(z_n)-f(y_n))}{f^{\prime }{(z_n)}^2}$, ${\beta }_1=-1$ and $\gamma =0$.

Li’s method [11]

$$\left\{\begin{array}{c}{y}_n=x_n-\frac{f(x_n)}{f^{\prime }(x_n)},\\ z_n=x_n-\frac{f(y_n)}{f(x_n)-2{\beta }_{2}f(y_n)}\frac{f(x_n)}{f^{\prime }(x_n)},\\ x_{n+1}=z_n-\frac{f(z_n)}{f^{\prime }(x_n)+f^{″}(x_n)(z_n-x_n)},\end{array}\right.$$

(30)

where ${\beta }_2=3$.

In

Table 1. Numerical results of different iterative methods.

${\mathit{f}}_{\mathit{i}}(\mathit{z})$	Method	${\mathit{z}}_0$	$|{\mathit{z}}_1-{\mathit{z}}_0|$	$|{\mathit{z}}_2-{\mathit{z}}_1|$	$|{\mathit{z}}_3-{\mathit{z}}_2|$	$|{\mathit{z}}_4-{\mathit{z}}_3|$	ACOC	Time
$f_1(z)$	CHM	0.2	0.05753	2.8768 $\times {10}^{-9}$	1.1312 $\times {10}^{-45}$	1.0635 $\times {10}^{-227}$	5.0	0.368283
	KM	0.2	0.05753	4.9599 $\times {10}^{-9}$	2.6574 $\times {10}^{-44}$	1.1731 $\times {10}^{-220}$	5.0	0.504487
	LIM	0.2	0.05753	4.2069 $\times {10}^{-9}$	7.8807 $\times {10}^{-45}$	1.8179 $\times {10}^{-223}$	5.0	0.685724
	CHM	0.3	0.04247	9.2906 $\times {10}^{-10}$	3.9736 $\times {10}^{-48}$	5.687 $\times {10}^{-240}$	5.0	0.465012
	KM	0.3	0.04247	1.3287 $\times {10}^{-9}$	3.6658 $\times {10}^{-47}$	5.8604 $\times {10}^{-235}$	5.0	0.700821
	LIM	0.3	0.04247	7.5548 $\times {10}^{-10}$	1.4718 $\times {10}^{-48}$	4.1309 $\times {10}^{-242}$	5.0	0.617231
$f_2(z)$	CHM	1.3	0.06523	3.2362 $\times {10}^{-7}$	8.0437 $\times {10}^{-34}$	7.6303 $\times {10}^{-167}$	5.0	0.495794
	KM	1.3	0.065231	9.2698 $\times {10}^{-7}$	4.6694 $\times {10}^{-31}$	1.5142 $\times {10}^{-152}$	5.0	0.553354
	LIM	1.3	0.06523	1.6599 $\times {10}^{-7}$	2.2011 $\times {10}^{-35}$	9.0229 $\times {10}^{-175}$	5.0	0.550163
	CHM	1.4	0.03477	1.0435 $\times {10}^{-8}$	2.8031 $\times {10}^{-41}$	3.9216 $\times {10}^{-204}$	5.0	0.360747
	KM	1.4	0.03477	3.2389 $\times {10}^{-8}$	2.4315 $\times {10}^{-38}$	5.7982 $\times {10}^{-189}$	5.0	0.417446
	LIM	1.4	0.03477	1.0054 $\times {10}^{-8}$	1.7939 $\times {10}^{-41}$	3.245 $\times {10}^{-205}$	5.0	0.498558
$f_3(z)$	CHM	1.5	0.025994	4.5845 $\times {10}^{-8}$	6.9123 $\times {10}^{-37}$	5.3858 $\times {10}^{-181}$	5.0	0.480012
	KM	1.5	0.025994	1.7726 $\times {10}^{-7}$	2.4137 $\times {10}^{-33}$	1.13 $\times {10}^{-162}$	5.0	0.481797
	LIM	1.5	0.025994	5.9101 $\times {10}^{-8}$	4.1989 $\times {10}^{-36}$	7.6005 $\times {10}^{-177}$	5.0	0.796286
	CHM	1.6	0.074001	5.3941 $\times {10}^{-6}$	1.5586 $\times {10}^{-26}$	3.139 $\times {10}^{-129}$	4.9999995	0.524147
	KM	1.6	0.074032	0.000025903	1.6086 $\times {10}^{-22}$	1.4855 $\times {10}^{-108}$	5.0000019	0.484185
	LIM	1.6	0.073982	0.000023806	4.4536 $\times {10}^{-23}$	1.0203 $\times {10}^{-111}$	5.0000038	1.117498
$f_4(z)$	CHM	1.4	0.0044916	3.356 $\times {10}^{-12}$	7.6642 $\times {10}^{-58}$	4.761 $\times {10}^{-286}$	5.0	0.384553
	KM	1.4	0.0044916	8.3785 $\times {10}^{-12}$	1.8583 $\times {10}^{-55}$	9.9733 $\times {10}^{-274}$	5.0	0.363025
	LIM	1.4	0.0044916	1.1576 $\times {10}^{-12}$	1.3428 $\times {10}^{-60}$	2.8203 $\times {10}^{-300}$	5.0	0.514793
	CHM	1.5	0.095499	9.5656 $\times {10}^{-6}$	1.4419 $\times {10}^{-25}$	1.1219 $\times {10}^{-124}$	4.9999991	0.676156
	KM	1.5	0.095533	0.000024869	4.2817 $\times {10}^{-23}$	6.4769 $\times {10}^{-112}$	5.0000024	0.744585
	LIM	1.5	0.0955	8.5631 $\times {10}^{-6}$	2.9745 $\times {10}^{-26}$	1.5044 $\times {10}^{-128}$	5.0000008	1.075170
$f_5(z)$	CHM	−0.4	0.042854	5.0183 $\times {10}^{-8}$	1.1955 $\times {10}^{-37}$	9.174 $\times {10}^{-186}$	5.0	0.544822
	KM	−0.4	0.042855	3.6043 $\times {10}^{-7}$	1.5472 $\times {10}^{-32}$	2.2556 $\times {10}^{-159}$	5.0	0.572932
	LIM	−0.4	0.042854	3.1056 $\times {10}^{-7}$	4.9815 $\times {10}^{-33}$	5.2894 $\times {10}^{-162}$	5.0	0.730796
	CHM	−0.5	0.057145	2.5432 $\times {10}^{-7}$	3.9964 $\times {10}^{-34}$	3.8292 $\times {10}^{-168}$	5.0	0.763921
	KM	−0.5	0.057147	1.6222 $\times {10}^{-6}$	2.8576 $\times {10}^{-29}$	4.847 $\times {10}^{-143}$	5.0	0.585273
	LIM	−0.5	0.057145	8.2965 $\times {10}^{-7}$	6.7774 $\times {10}^{-31}$	2.4655 $\times {10}^{-151}$	4.9999999	0.753026
$f_6(z)$	CHM	0.1	0.055438	0.011285	2.2307 $\times {10}^{-7}$	4.8967 $\times {10}^{-31}$	5.0293972	0.793084
	KM	0.1	0.091476	0.024762	0.000010038	1.8075 $\times {10}^{-22}$	4.9362619	0.837225
	LIM	0.1	0.067567	0.00084373	3.1209 $\times {10}^{-15}$	9.2141 $\times {10}^{-74}$	5.1198554	0.933355
	CHM	0.2	0.033261	0.000015741	8.5645 $\times {10}^{-22}$	4.0847 $\times {10}^{-103}$	4.9999885	0.861654
	KM	0.2	0.033315	0.000038138	1.4321 $\times {10}^{-19}$	1.0684 $\times {10}^{-91}$	5.000026	1.014709
	LIM	0.2	0.03326	0.000016127	4.8457 $\times {10}^{-25}$	8.3149 $\times {10}^{-123}$	5.007914	1.187511

,

Table 2. Numerical results for stable parameter values.

${\mathit{f}}_{\mathit{i}}(\mathit{z})$	$\mathit{\alpha }$	${\mathit{z}}_0$	$|{\mathit{z}}_1-{\mathit{z}}_0|$	$|{\mathit{z}}_2-{\mathit{z}}_1|$	$|{\mathit{z}}_3-{\mathit{z}}_2|$	$|{\mathit{z}}_4-{\mathit{z}}_3|$	ACOC	Time
$f_1(z)$	−1	0.3	0.04247	5.1534 $\times {10}^{-10}$	9.5535 $\times {10}^{-50}$	2.0917 $\times {10}^{-248}$	5.0	0.533905
	$-\frac{1}{2}$	0.3	0.04247	6.542 $\times {10}^{-10}$	4.3928 $\times {10}^{-49}$	5.9965 $\times {10}^{-245}$	5.0	0.353646
	$\frac{1}{2}$	0.3	0.04247	9.2906 $\times {10}^{-10}$	3.9736 $\times {10}^{-48}$	5.687 $\times {10}^{-240}$	5.0	0.457915
	−1	1	0.73694	0.0055298	1.4278 $\times {10}^{-14}$	1.5599 $\times {10}^{-72}$	5.0018483	1.203424
	$-\frac{1}{2}$	1	0.73644	0.00603	3.0361 $\times {10}^{-14}$	9.4566 $\times {10}^{-71}$	5.0014638	0.654055
	$\frac{1}{2}$	1	0.7352	0.0072671	1.1962 $\times {10}^{-13}$	1.4062 $\times {10}^{-67}$	5.0011154	0.574249
$f_2(z)$	-1	1.4	0.03477	4.8832 $\times {10}^{-8}$	3.1527 $\times {10}^{-37}$	3.5361 $\times {10}^{-183}$	5.0	0.455763
	$-\frac{1}{2}$	1.4	0.03477	3.6526 $\times {10}^{-8}$	5.4119 $\times {10}^{-38}$	3.8648 $\times {10}^{-187}$	5.0	0.420997
	$\frac{1}{2}$	1.4	0.03477	1.0435 $\times {10}^{-8}$	2.8031 $\times {10}^{-41}$	3.9216 $\times {10}^{-204}$	5.0	0.736641
	−1	2	0.62288	0.011886	2.5419 $\times {10}^{-10}$	1.2049 $\times {10}^{-48}$	4.9967226	0.476672
	$-\frac{1}{2}$	2	0.62437	0.010403	9.7005 $\times {10}^{-11}$	7.1504 $\times {10}^{-51}$	4.9975948	0.429954
	$\frac{1}{2}$	2	0.62983	0.0049369	6.5529 $\times {10}^{-13}$	2.738 $\times {10}^{-62}$	4.9993795	0.482312
$f_3(z)$	−1	1.5	0.025994	3.1251 $\times {10}^{-7}$	6.1457 $\times {10}^{-32}$	1.8076 $\times {10}^{-155}$	5.0000001	0.505113
	$-\frac{1}{2}$	1.5	0.025994	2.1731 $\times {10}^{-7}$	7.2122 $\times {10}^{-33}$	2.9043 $\times {10}^{-160}$	5.0	0.600533
	$\frac{1}{2}$	1.5	0.025994	4.5845 $\times {10}^{-8}$	6.9123 $\times {10}^{-37}$	5.3858 $\times {10}^{-181}$	5.0	0.412318
	−1	2	0.44736	0.026644	2.1889 $\times {10}^{-7}$	1.036 $\times {10}^{-32}$	4.9799307	0.540150
	$-\frac{1}{2}$	2	0.44988	0.024127	1.0121 $\times {10}^{-7}$	1.5806 $\times {10}^{-34}$	4.9851187	0.472520
	$\frac{1}{2}$	2	0.46174	0.01227	8.9598 $\times {10}^{-10}$	1.9708 $\times {10}^{-45}$	4.9964929	0.584135
$f_4(z)$	−1	1.4	0.0044916	1.5364 $\times {10}^{-11}$	6.9365 $\times {10}^{-54}$	1.301 $\times {10}^{-265}$	5.0	0.763457
	$-\frac{1}{2}$	1.4	0.0044916	1.1328 $\times {10}^{-11}$	1.1194 $\times {10}^{-54}$	1.0548 $\times {10}^{-269}$	5.0	0.778445
	$\frac{1}{2}$	1.4	0.0044916	3.356 $\times {10}^{-12}$	7.6642 $\times {10}^{-58}$	4.761 $\times {10}^{-286}$	5.0	0.457384
	−1	1	nc	nc	nc	nc	nc	nc
	$-\frac{1}{2}$	1	nc	nc	nc	nc	nc	nc
	$\frac{1}{2}$	1	0.27406	0.13031	0.00012133	4.7358 $\times {10}^{-20}$	5.083647	0.822961
$f_5(z)$	−1	-0.4	0.042854	3.8978 $\times {10}^{-7}$	2.9643 $\times {10}^{-32}$	7.541 $\times {10}^{-158}$	5.0	0.550584
	$-\frac{1}{2}$	-0.4	0.042854	2.8444 $\times {10}^{-7}$	4.3227 $\times {10}^{-33}$	3.5043 $\times {10}^{-162}$	5.0	0.573988
	$\frac{1}{2}$	-0.4	0.042854	5.0183 $\times {10}^{-8}$	1.1955 $\times {10}^{-37}$	9.174 $\times {10}^{-186}$	5.0	0.352655
	−1	0	0.43258	0.010278	3.5974 $\times {10}^{-10}$	1.9851 $\times {10}^{-47}$	4.9971435	0.502055
	$-\frac{1}{2}$	0	0.43396	0.0088947	1.2485 $\times {10}^{-10}$	7.0433 $\times {10}^{-50}$	4.9980779	0.498097
	$\frac{1}{2}$	0	0.43998	0.0028767	7.3611 $\times {10}^{-14}$	8.1186 $\times {10}^{-67}$	4.9997831	0.479472
$f_6(z)$	−1	0.1	nc	nc	nc	nc	nc	nc
	$-\frac{1}{2}$	0.1	nc	nc	nc	nc	nc	nc
	$\frac{1}{2}$	0.1	0.055438	0.011285	2.2307 $\times {10}^{-7}$	4.8967 $\times {10}^{-31}$	5.0293972	0.793084
	−1	0.2	0.033233	0.000043824	5.7218 $\times {10}^{-19}$	2.1749 $\times {10}^{-88}$	4.9999423	0.974917
	$-\frac{1}{2}$	0.2	0.03324	0.000036187	1.6483 $\times {10}^{-19}$	3.2357 $\times {10}^{-91}$	4.9999586	1.016791
	$\frac{1}{2}$	0.2	0.033261	0.000015741	8.5645 $\times {10}^{-22}$	4.0847 $\times {10}^{-103}$	4.9999885	0.861654

and

Table 3. Numerical results for unstable parameter values.

${\mathit{f}}_{\mathit{i}}(\mathit{z})$	$\mathit{\alpha }$	${\mathit{z}}_0$	$|{\mathit{z}}_1-{\mathit{z}}_0|$	$|{\mathit{z}}_2-{\mathit{z}}_1|$	$|{\mathit{z}}_3-{\mathit{z}}_2|$	$|{\mathit{z}}_4-{\mathit{z}}_3|$	ACOC	Time
$f_1(z)$	1.5	0.3	0.04247	1.2001 $\times {10}^{-9}$	1.9459 $\times {10}^{-47}$	2.1807 $\times {10}^{-236}$	5.0	0.636435
	2	0.3	0.04247	1.3343 $\times {10}^{-9}$	3.7441 $\times {10}^{-47}$	6.5139 $\times {10}^{-235}$	5.0	0.622020
	2.17	0.3	0.04247	1.3797 $\times {10}^{-9}$	4.6023 $\times {10}^{-47}$	1.9009 $\times {10}^{-234}$	5.0	0.516650
	1.5	1	0.7335	0.0089677	4.6371 $\times {10}^{-13}$	1.6756 $\times {10}^{-64}$	5.0009595	0.632580
	2	1	0.73239	0.010082	9.4161 $\times {10}^{-13}$	6.5534 $\times {10}^{-63}$	5.0009115	0.541331
	2.17	1	0.73196	0.010515	1.2077 $\times {10}^{-12}$	2.3649 $\times {10}^{-62}$	5.0008979	0.526807
$f_2(z)$	1.5	1.4	0.03477	1.7826 $\times {10}^{-8}$	6.8271 $\times {10}^{-40}$	5.6248 $\times {10}^{-197}$	5.0	0.383288
	2	1.4	0.03477	3.2854 $\times {10}^{-8}$	2.6113 $\times {10}^{-38}$	8.2829 $\times {10}^{-189}$	5.0	0.387603
	2.17	1.4	0.03477	3.8109 $\times {10}^{-8}$	6.3115 $\times {10}^{-38}$	7.8636 $\times {10}^{-187}$	5.0	0.388549
	1.5	2	0.64754	0.012775	1.3287 $\times {10}^{-10}$	1.5705 $\times {10}^{-50}$	5.0015962	0.371706
	2	2	0.68237	0.047599	1.8055 $\times {10}^{-7}$	1.3087 $\times {10}^{-34}$	5.0064011	0.401961
	2.17	2	0.71315	0.078385	2.6199 $\times {10}^{-6}$	9.6917 $\times {10}^{-29}$	5.0116522	0.390029
$f_3(z)$	1.5	1.5	0.025994	1.0419 $\times {10}^{-7}$	9.8946 $\times {10}^{-35}$	7.6415 $\times {10}^{-170}$	5.0	0.509447
	2	1.5	0.025994	1.7237 $\times {10}^{-7}$	2.0986 $\times {10}^{-33}$	5.6139 $\times {10}^{-163}$	5.0	0.545617
	2.17	1.5	0.025994	1.9461 $\times {10}^{-7}$	4.3943 $\times {10}^{-33}$	2.5795 $\times {10}^{-161}$	5.0	0.473363
	1.5	2	0.56001	0.086052	0.000051492	2.9164 $\times {10}^{-21}$	5.0408977	0.488337
	2	2	nc	nc	nc	nc	nc	nc
	2.17	2	nc	nc	nc	nc	nc	nc
$f_4(z)$	1.5	1.4	0.0044916	4.4841 $\times {10}^{-12}$	4.3515 $\times {10}^{-57}$	3.7449 $\times {10}^{-282}$	5.0	0.709110
	2	1.4	0.0044916	8.3557 $\times {10}^{-12}$	1.8331 $\times {10}^{-55}$	9.3145 $\times {10}^{-274}$	5.0	0.705258
	2.17	1.4	0.0044916	9.6647 $\times {10}^{-12}$	4.3973 $\times {10}^{-55}$	8.5733 $\times {10}^{-272}$	5.0	0.699666
	1.5	1	0.64393	0.23998	0.00054848	1.1945 $\times {10}^{-16}$	4.7943402	0.688360
	2	1	0.72825	0.32888	0.0051229	1.6162 $\times {10}^{-11}$	4.7031415	0.879573
	2.17	1	0.75033	0.35501	0.0091719	3.4807 $\times {10}^{-10}$	4.6736711	0.745030
$f_5(z)$	1.5	-0.4	0.042855	2.2154 $\times {10}^{-7}$	8.3805 $\times {10}^{-34}$	6.4923 $\times {10}^{-166}$	5.0	0.490509
	2	-0.4	0.042855	3.7423 $\times {10}^{-7}$	1.867 $\times {10}^{-32}$	5.7706 $\times {10}^{-159}$	5.0	0.499436
	2.17	-0.4	0.042855	4.2904 $\times {10}^{-7}$	4.179 $\times {10}^{-32}$	3.6639 $\times {10}^{-157}$	5.0	0.737970
	1.5	0	0.4731	0.03025	4.0617 $\times {10}^{-8}$	1.7361 $\times {10}^{-37}$	5.0015384	0.459926
	2	0	0.69565	0.25573	0.0029359	5.553 $\times {10}^{-13}$	5.0118838	0.470911
	2.17	0	nc	nc	nc	nc	nc	nc
$f_6(z)$	1.5	0.1	0.042855	0.012147	1.727 $\times {10}^{-7}$	1.3621 $\times {10}^{-31}$	4.9725788	1.238945
	2	0.1	0.084431	0.01771	2.215 $\times {10}^{-6}$	9.4549 $\times {10}^{-26}$	4.9629725	1.001502
	2.17	0.1	0.085903	0.019183	3.8987 $\times {10}^{-6}$	1.869 $\times {10}^{-24}$	4.9618955	1.055451
	1.5	0.2	0.033293	0.000016401	1.0528 $\times {10}^{-21}$	1.1468 $\times {10}^{-102}$	5.0000115	1.223707
	2	0.2	0.033317	0.000040334	1.8947 $\times {10}^{-19}$	4.3301 $\times {10}^{-91}$	5.0000249	1.158187
	2.17	0.2	0.033327	0.000050322	6.7019 $\times {10}^{-19}$	2.8053 $\times {10}^{-88}$	5.0000295	1.150161

, Time is the computing time of the machine, $z_0$ is the initial value and ACOC [26] is the approximated order of convergence.

•

In the numerical experiment, we will use the following five nonlinear equations, which appear in [13,14], respectively. Their expressions and approximate roots are given below.

$f_1(z)=z^2-e^z-3z+2,\epsilon \approx 0.25753028543986076$

$f_2(z)=z^3+4z^2-10,\epsilon \approx 1.3652300134140968$

$f_3(z)=z^4-lg(z)-5,\epsilon \approx 1.5259939537536892$

$f_4(z)=si{n}^{2}z-z^2+1,\epsilon \approx 1.4044916482153412$

$f_5(z)=(z+2)e^z-1,\epsilon \approx -0.44285440100238858$

•

In addition, let us consider a physical problem. An object of mass m is dropped from a height h onto a real spring whose elastic force is $Fe=-(k_{1}x+k_2{x}^{1.5})$, where z is the compression of the spring; calculate the maximum compression of the spring:

$$mgh+mgz-\frac{1}{2}{k}_1{z}^2-\frac{2}{5}{k}_2{z}^{2.5}=0,\epsilon \approx 0.16672356243778485$$

Let the gravity be $g=9.81 \mathrm{m}/{\mathrm{s}}^2$, the proportionality constant $k_1=40,000 \mathrm{g}/{\mathrm{s}}^2$, $k_2=40 \mathrm{g}/({\mathrm{s}}^2{\mathrm{m}}^{0.5})$, mass of the object $m=95 \mathrm{g}$ and height $h=0.43 \mathrm{m}$. Thus, we obtain the test function

$f_6(z)=\frac{801,477}{2000}+\frac{18,639}{20}z-20,000z^2-16z^{2.5},\epsilon \approx 0.16672356243778485$.

Table 1 shows that our method has high computational accuracy. In Table 2, we obtained some numerical results for nonlinear equations by taking some stable parameter values $\alpha =-1,\alpha =-\frac{1}{2},\alpha =\frac{1}{2}$. Table 3 shows the numerical results for some of the unstable parameters $\alpha =1.5,\alpha =2,\alpha =2.17$. From Table 2 and Table 3, we can find that the iterative method corresponding to these stable values obtains better results, which is exactly in line with the results analyzed in the third part. In Table 1, Table 2 and Table 3, compared with other methods, our method has a much shorter computation time.

5. Comparison of Fractal Diagram for Different Methods

In this section, the fractal diagram of different methods for different polynomials $F_1(z)=z^2-1$ and $F_2(z)=z^3-1$ are compared. The iterative methods being compared are CHM($\alpha =\frac{1}{2}$), CHM($\alpha =2$), KM and KM2.

The KM2 [27] method

$$\left\{\begin{array}{c}{y}_n=z_n-\theta \frac{f(z_n)}{f^{\prime }(z_n)},\\ \\ z_{n+1}=z_n-\frac{f(y_n)+({\theta }^2+\theta -1)f(z_n)}{{\theta }^2{f}^{\prime }(z_n)},\end{array}\right.$$

(31)

where $\theta =\frac{1}{2}$.

Figure 9 and Figure 10, respectively, show the attractive basin of the above three methods on the polynomial $F_1(z)=z^2-1$ and $F_2(x)=z^3-1$. We set up a grid of $500\times 500$ points to draw the image in area $\mathbb{D}=[-5,5]\times [-5,5]$, and we set the maximum number of iterations to 25. The red, yellow and blue areas in these figures represent convergence to the roots of these polynomials, while non-convergence to the highest number of iterations is plotted as black areas. In addition, the number of iterations is shown as a lighter or darker color (the fewer iterations, the brighter the color).

From Figure 9, we can see that the divergence points of CHM($\alpha =\frac{1}{2}$) and KM2 are less than KM and CHM($\alpha =2$). In Figure 10, for solving $z^3-1$, the convergence effect of CHM($\alpha =\frac{1}{2}$) is better than the other methods. Therefore, when $\alpha =\frac{1}{2}$, the convergence and stability of iterative method CHM are superior to other methods, this result is in agreement with the numerical results above.

6. Conclusions

In this paper, a family of two-step Chebyshev–Halley-type iterative methods for solving nonlinear equations is presented. Its convergence order is the fifth order to be analyzed. And it increases the order of convergence of the Chebyshev–Halley method. By analyzing the dynamic behavior, the relevant strange fixed points and free critical points are discovered. Then, the stability of strange fixed points is studied and some results are obtained. In addition, by observing the parameter plane associated with the free critical point, some special parameter values are selected. It is ideal to select parameter values in the blue and red regions of the parameter plane. The dynamic planes corresponding to these special parameters are plotted. Based on the above research, a parameter $\alpha =\frac{1}{2}$ with good stability is selected. The numerical experiment results are consistent with the results obtained from the dynamic analysis. The study show that it is effective to research the stability of the iterative method by using fractal theory.