Fractal Analysis of Coal Pore Structure Based on Computed Tomography and Fluid Intrusions
Abstract
1. Introduction
2. Coal Samples and Methods
3. Experimental Results and Analysis
3.1. Low-Temperature N2 Adsorption Results
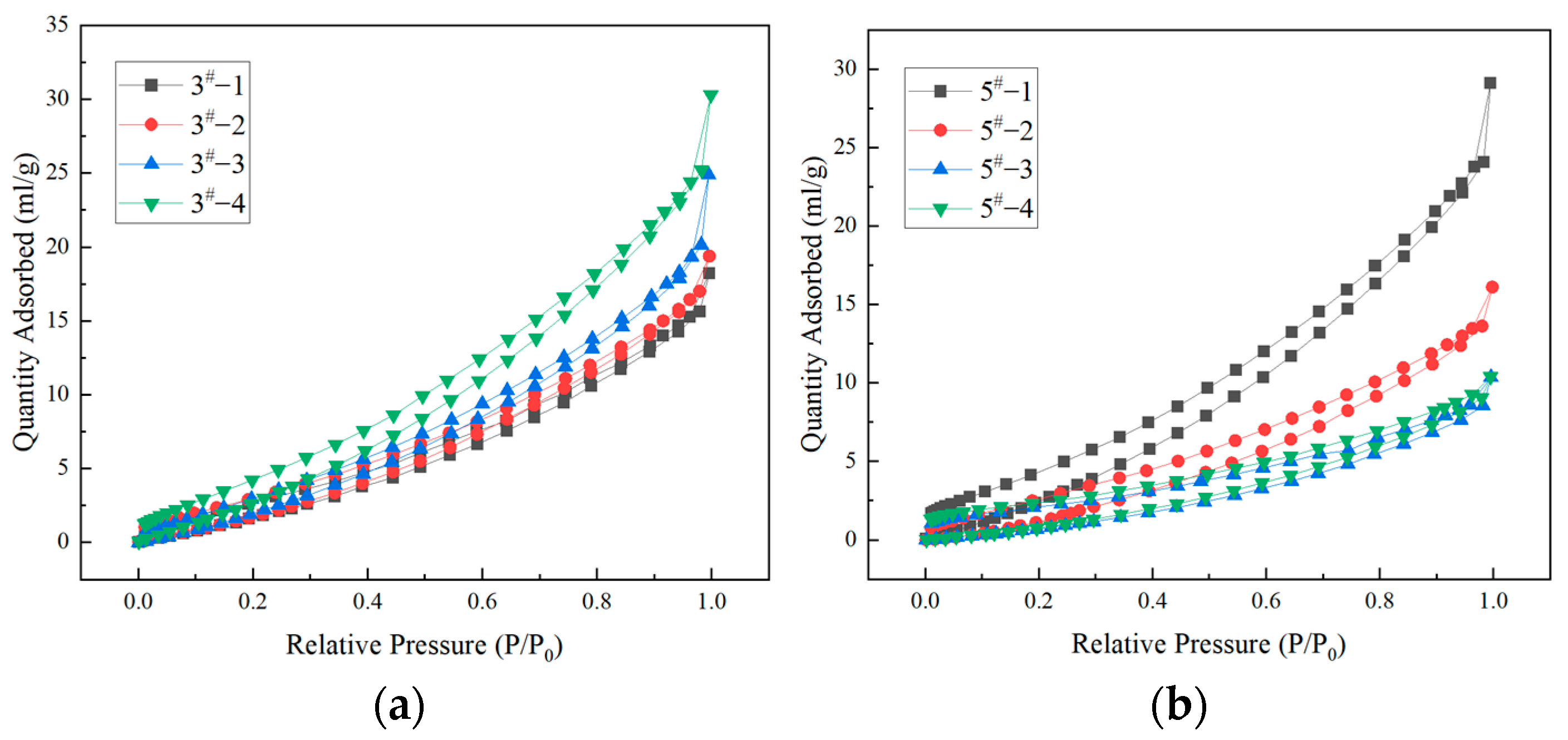
3.1.1. Adsorption Isotherm
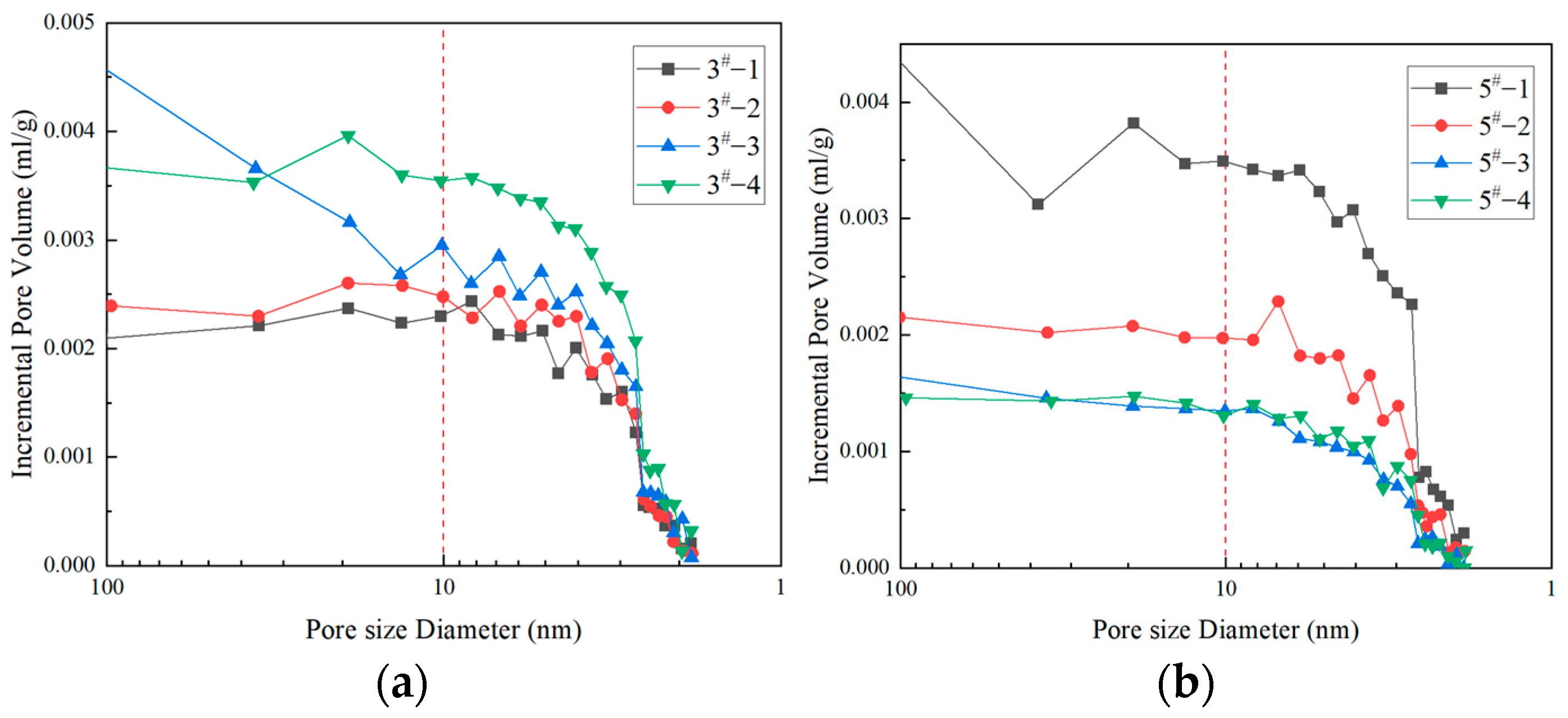
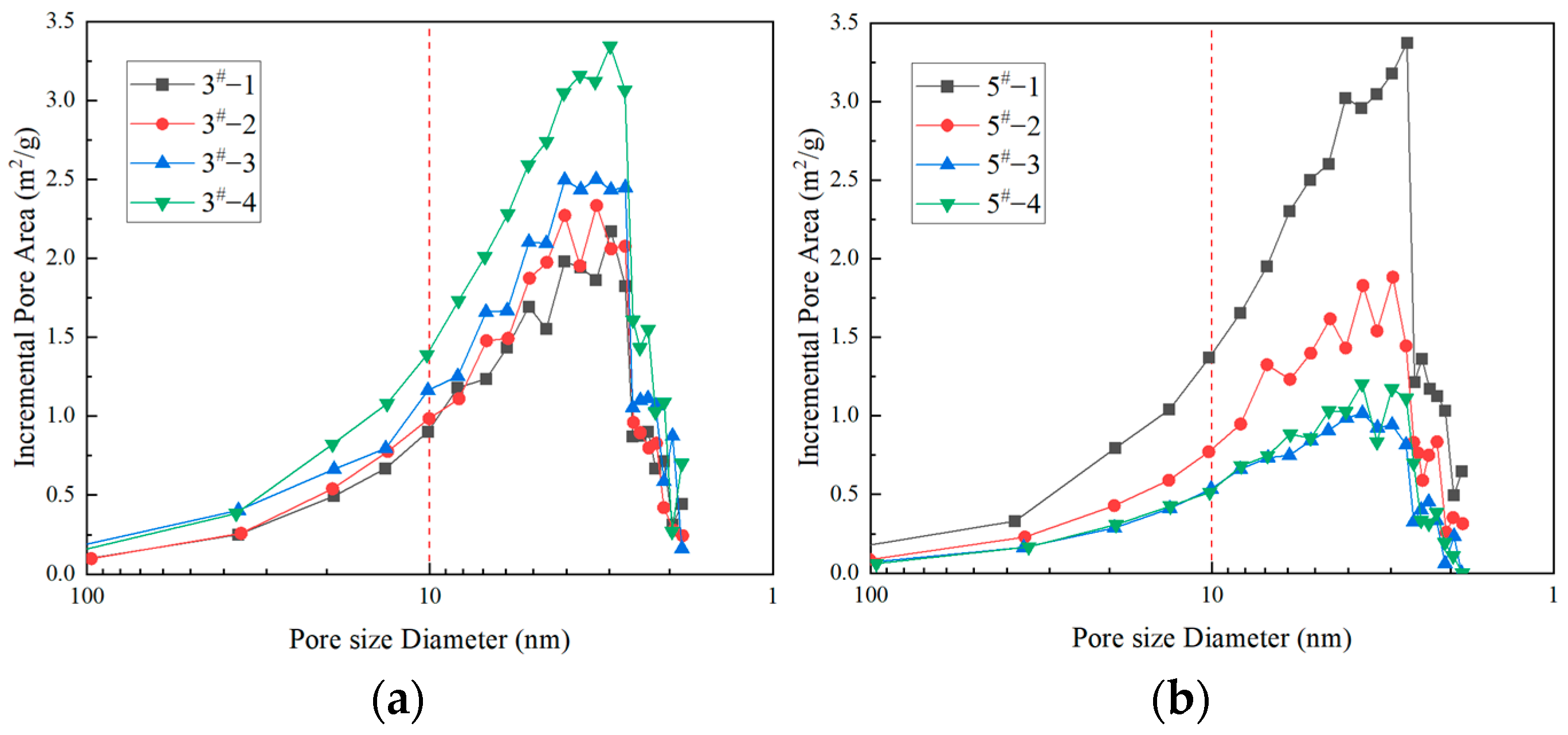
3.1.2. Pore Structure Characteristics of Small Pores and Micropores
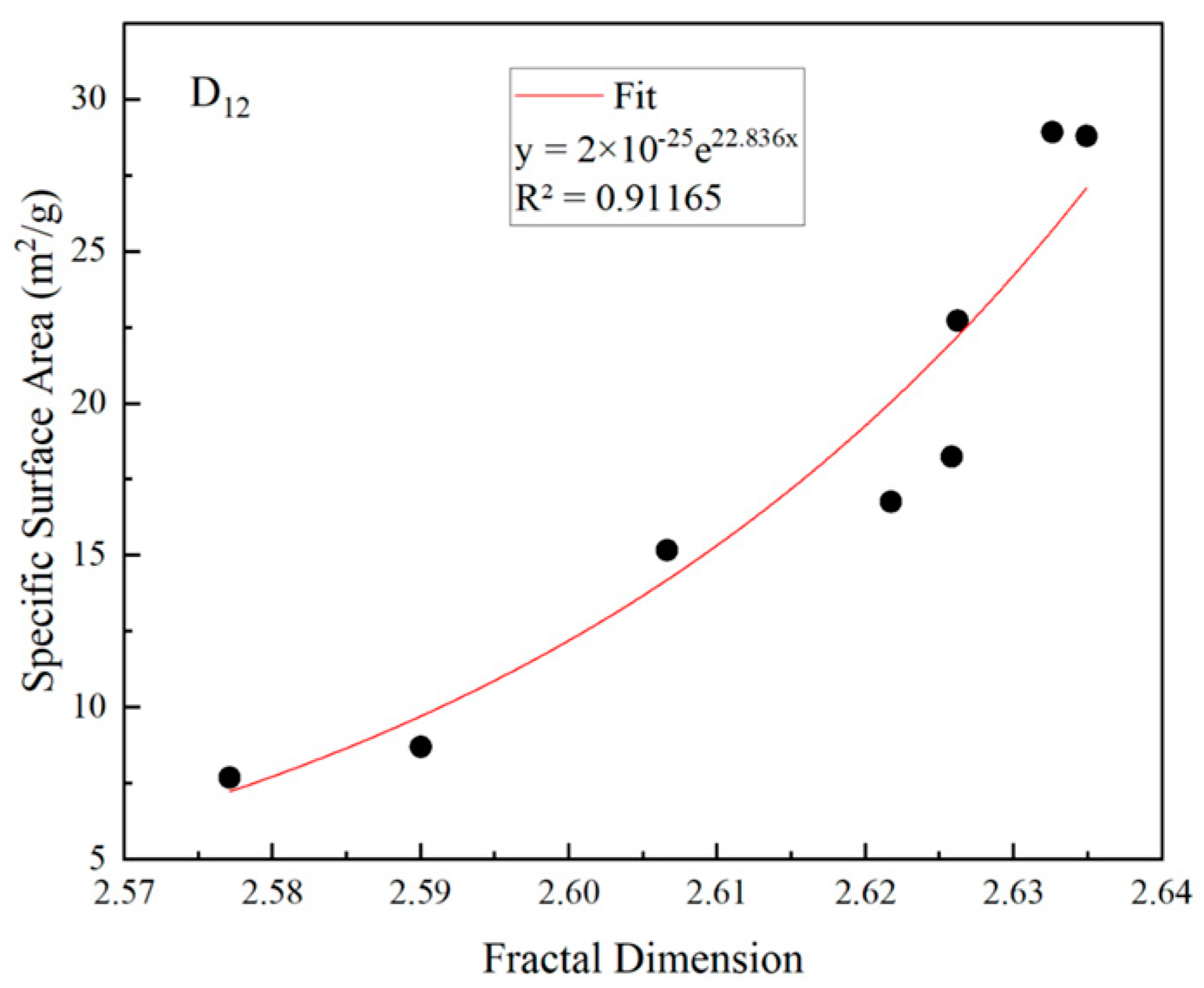
3.1.3. Fractal Analysis of Small Pores and Micropores
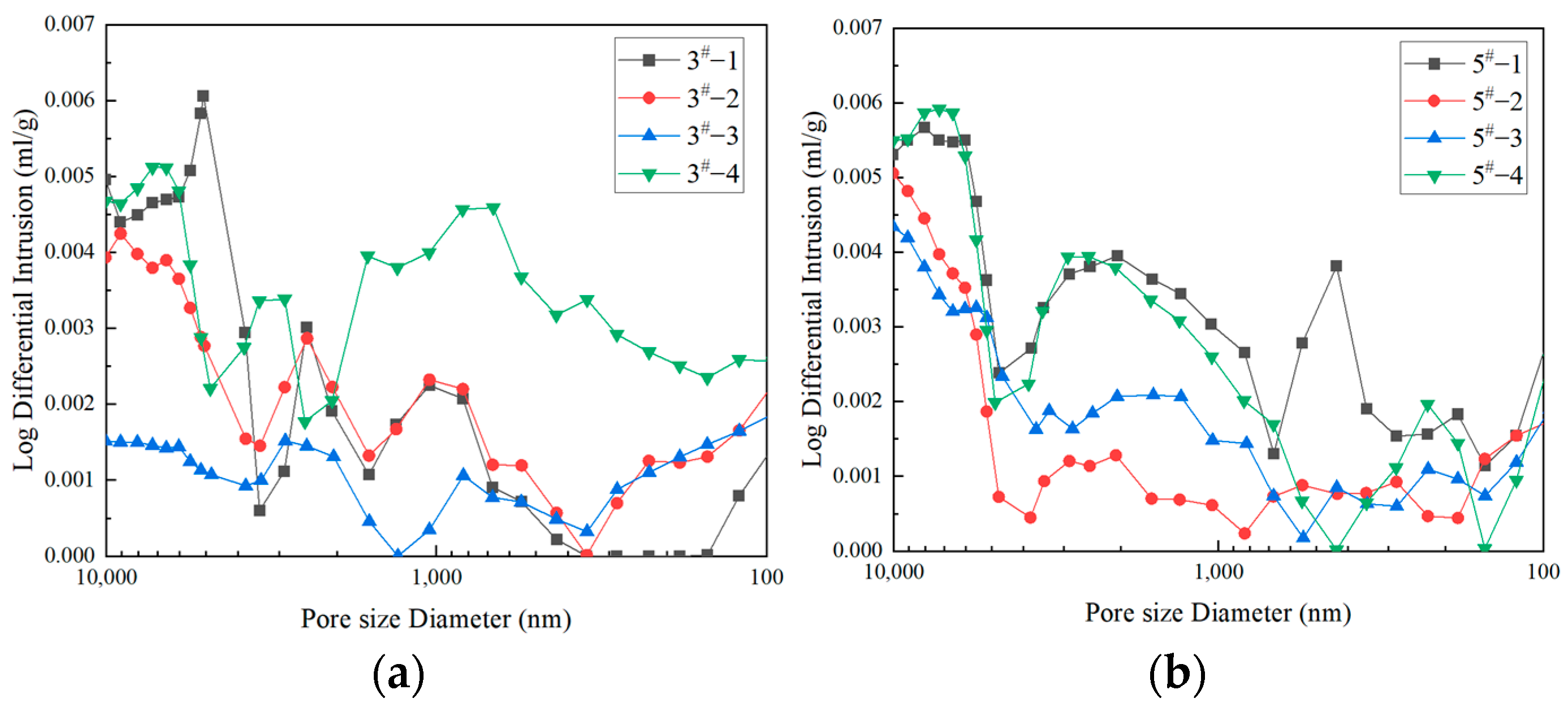
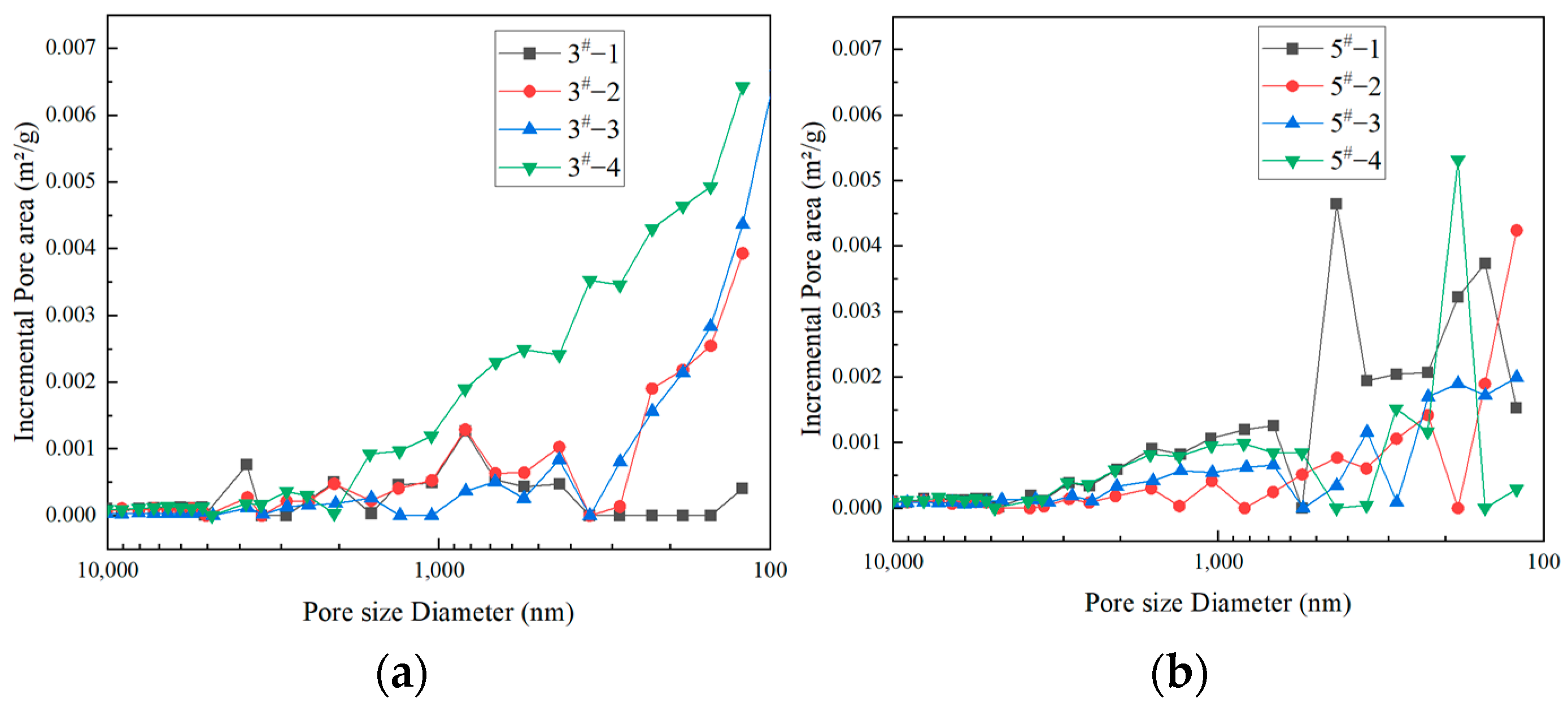
3.2. Mercury Pressure Method Results
3.2.1. Inlet and Outlet Mercury Curves
3.2.2. Large Pore and Mesopore Structure Characteristics
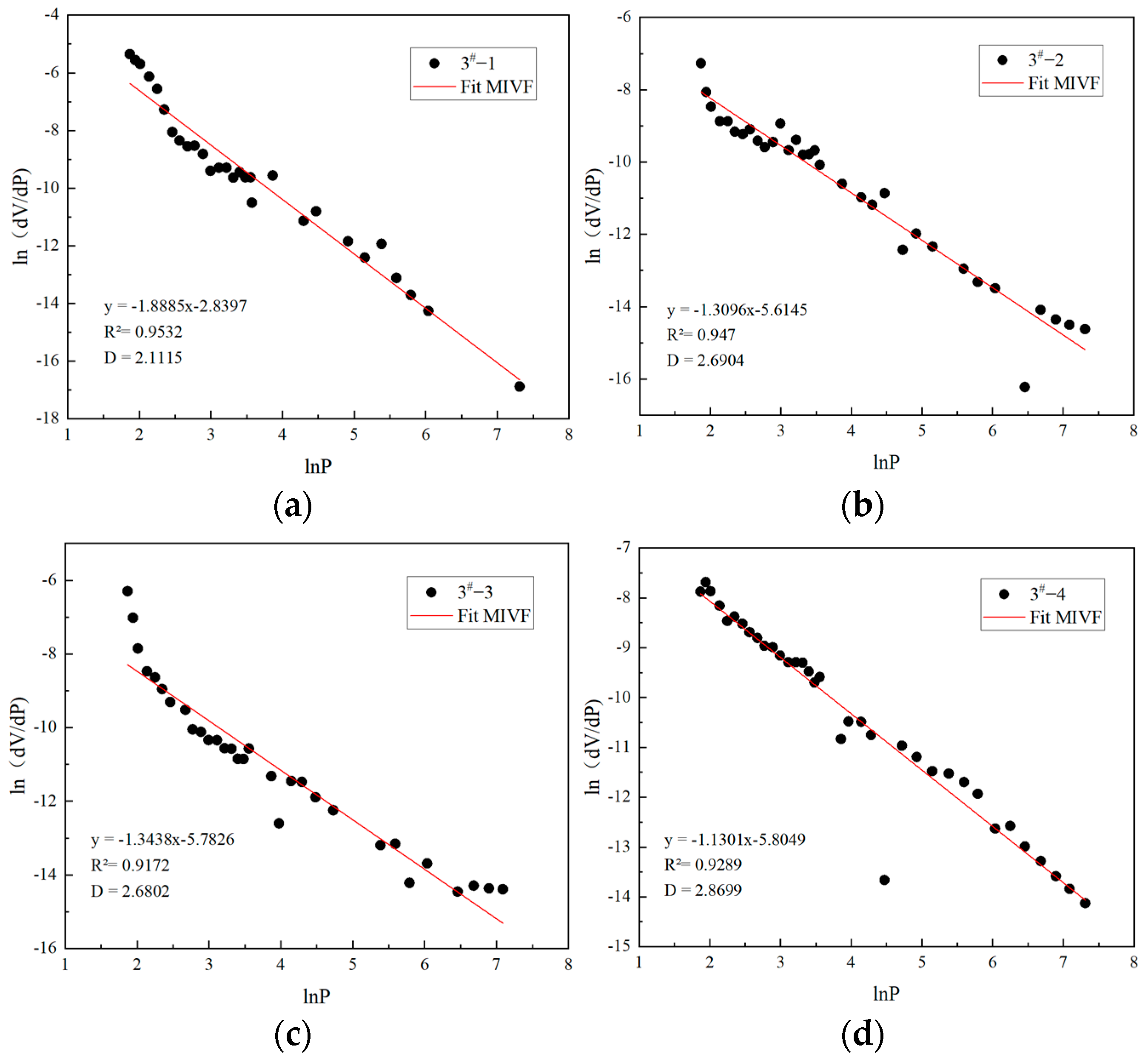
3.2.3. Fractal Analysis of Large Pores and Mesopores
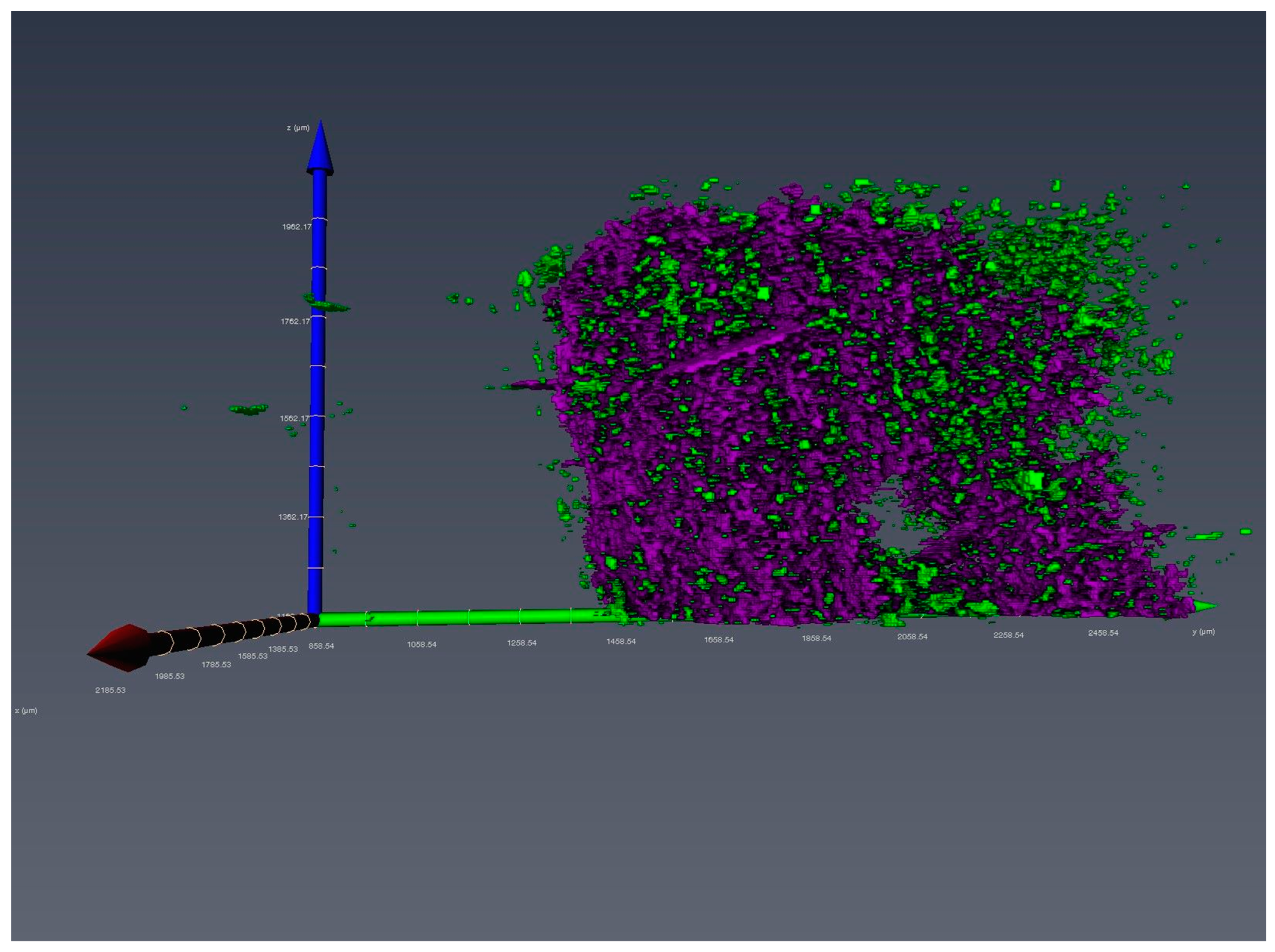
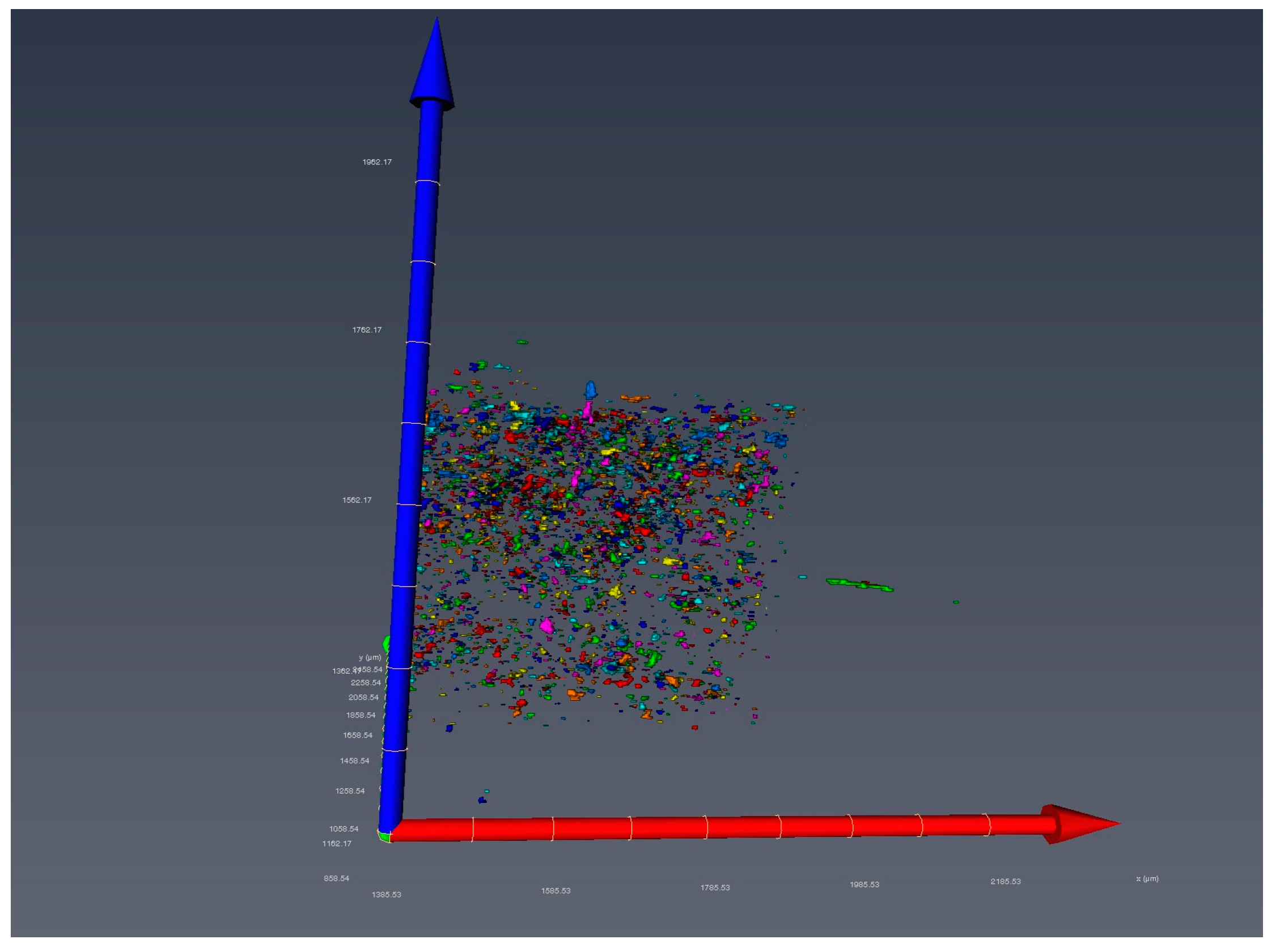
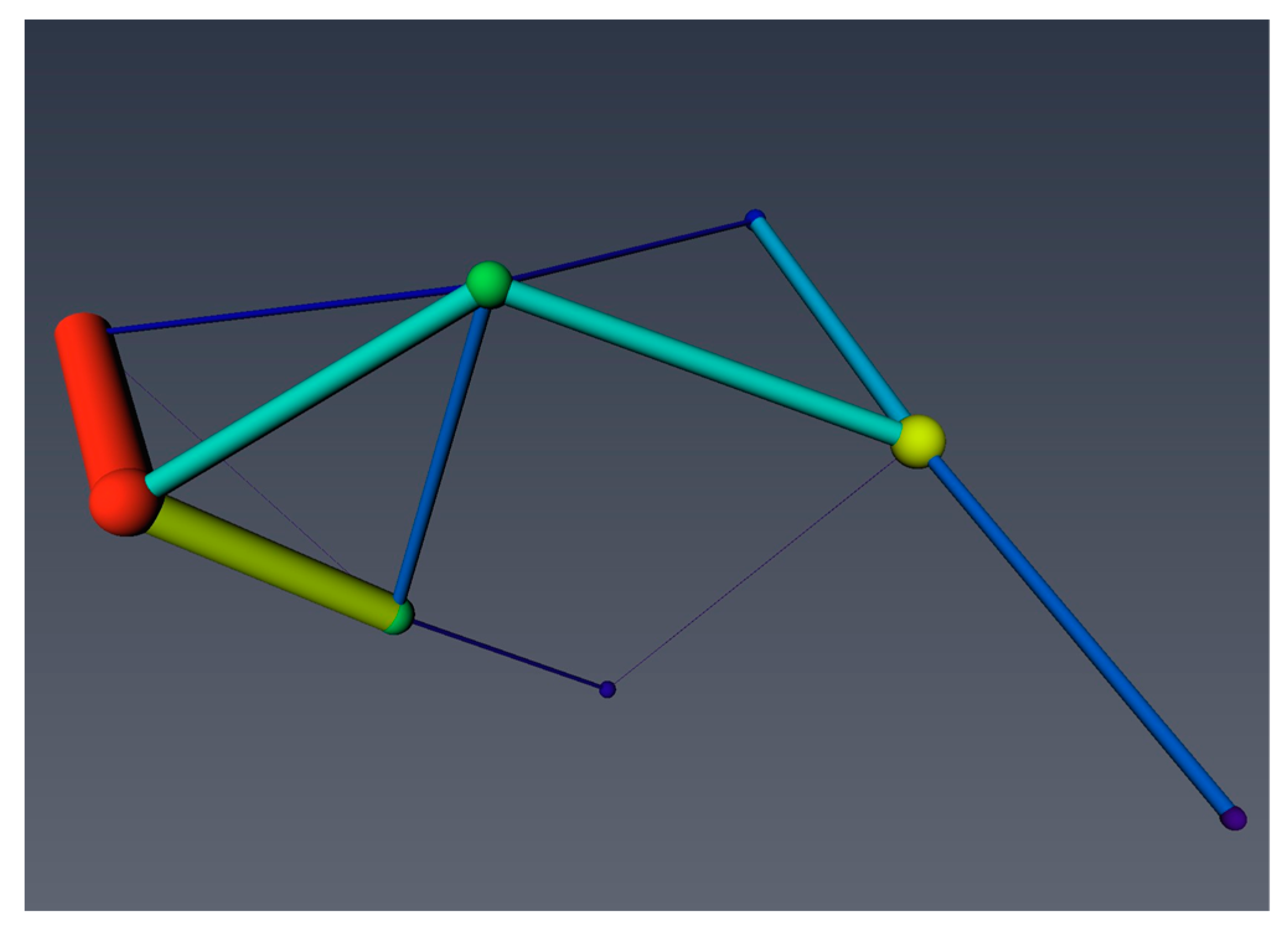
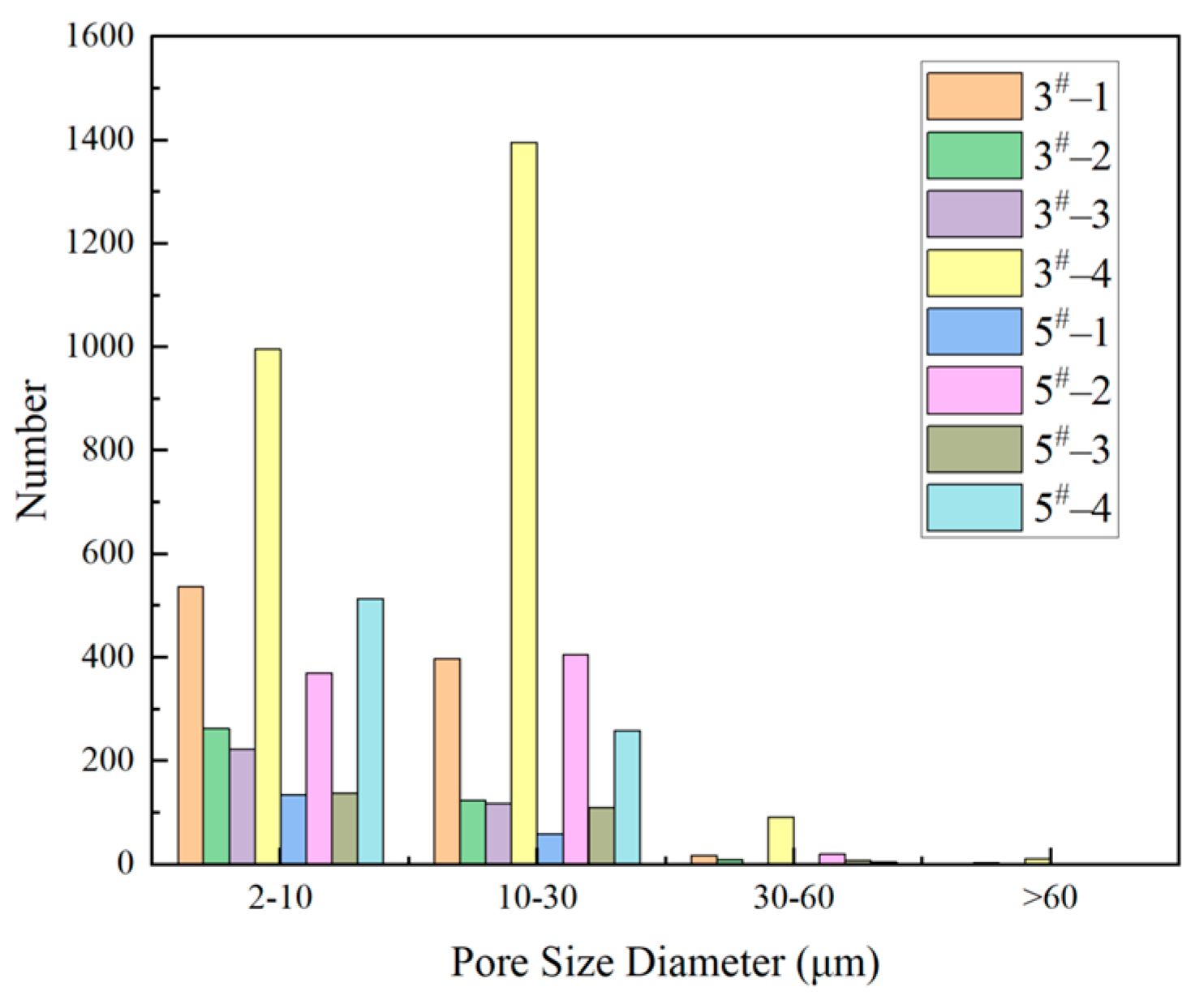
3.3. Coal Pore Structure from X-ray Micro-CT
4. Conclusions
- (1)
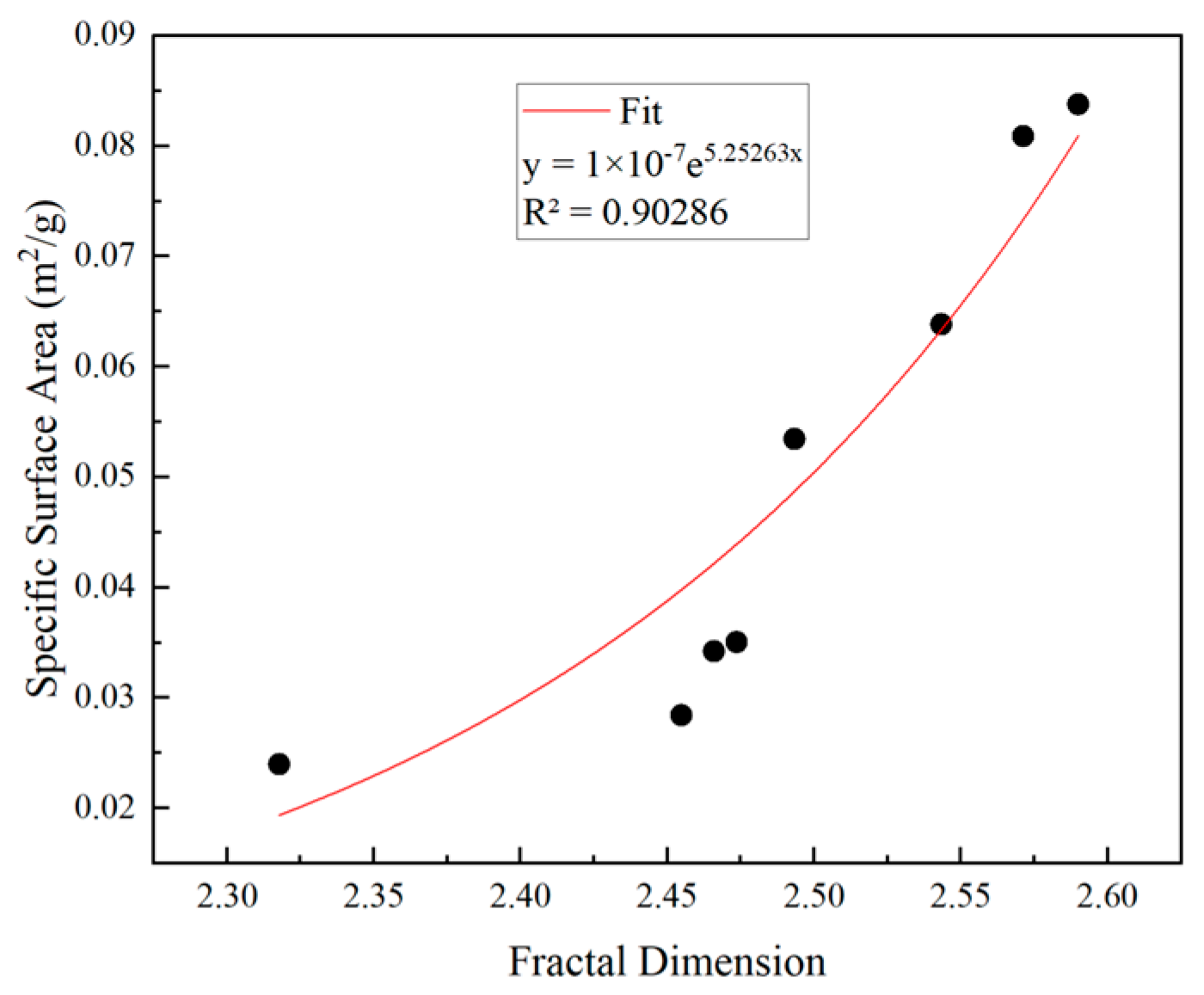
- The fractal size of each pore segment in the coal samples increased exponentially with increasing specific surface area: the relationship for small pores and micropores measured using low-temperature liquid N2 adsorption was y = 2 × 10−25e22.836x; the relationship for large pores and mesopores measured using the high-pressure mercury pressure method was y = 3 × 10−4e1.73431x; the relationship for large pores measured using X-ray micro-CT scanning was y = 1 × 10−7e5.25263x. The fitted values were all >0.9, indicating an extremely strong correlation.
- (2)
- The adsorption isotherms of all coal samples belonged to Type III. The adsorption curves increased with increasing P/P0, and there was no obvious inflection point in any of the curves. The desorption and adsorption curves decreased smoothly, without a steep change interval, indicating that the small micropores in the coal samples were mainly composed of impermeable pores closed at one end. The micropores developed in the coal samples provided sufficient channels for gas adsorption and transport. The fractal dimension in the range of small pores and micropores showed an exponential growth relationship with the specific surface area.
- (3)
- The inlet and outlet mercury curves for the samples were bullhorn shaped and similar. All coal samples produced hysteresis rings, although these were not significant, indicating that the closed pores in the large and medium pore range were more developed. The pore volume of large and medium pores in the coal samples was mainly attributable to large pores, whereas medium pores were less developed. The fractal dimension of large pores and mesopores was 2.11–2.87, and the fit was >0.9, indicating obvious fractal characteristics. The D of large and medium pores showed an exponential growth relationship with the specific surface area, and the fit was >0.97, indicating a very strong correlation.
- (4)
- The pores of the coal samples within the CT study were mainly concentrated in the range of 4–30 μm in diameter. The percentage of isolated pores was low in all samples, indicating good connectivity in this pore size range. The fractal dimension of the pore size of coal samples of >2 μm exponentially increased with increasing specific surface area overall, which was consistent with the conclusion drawn from the data obtained using the fluid intrusion method.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Du, F.; Wang, K.; Zhang, X.; Xin, C.P.; Shu, L.Y.; Wang, G.D. Experimental Study of Coal–Gas Outburst: Insights from Coal–Rock Structure, Gas Pressure and Adsorptivity. Nat. Resour. Res. 2020, 29, 2481–2493. [Google Scholar] [CrossRef]
- Yang, Y.P.; Wen, Z.H.; Si, L.L.; Xu, X.Y. Experimental study on variation law of electrical parameters and temperature rise effect of coal under DC electric field. Sci. Rep. 2021, 11, 7138. [Google Scholar] [CrossRef]
- Si, L.L.; Zhang, H.T.; Wei, J.P.; Li, B.; Han, H.K. Modeling and experiment for effective diffusion coefficient of gas in water-saturated coal. Fuel 2021, 284, 118887. [Google Scholar] [CrossRef]
- Zhang, H.J.; Zhang, L.; Wang, D.; Hou, J.L. Gas emission characteristics of tectonic coal and microscopic explanation of pore structure. J. China Coal Soc. 2018, 43, 3404–3410. [Google Scholar]
- Tim, A.M. Coalbed methane: A review. Int. J. Coal Geol. 2012, 101, 36–81. [Google Scholar]
- Qin, Y.; Tim, A.M.; Shen, J.; Yang, Z.B.; Shen, Y.L.; Wang, G. Resources and geology of coalbed methane in China: A review. Int. Geol. Rev. 2018, 60, 777–812. [Google Scholar] [CrossRef]
- Wen, Z.H.; Yang, Y.P.; Wang, Q.; Yao, B.H. Mechanism and characteristics of CH4/CO2/H2O adsorption in lignite molecules. Geofluids 2021, 2021, 5535321. [Google Scholar] [CrossRef]
- Yue, J.W.; Wang, Z.F.; Chen, J.S.; Zheng, M.H.; Wang, Q.; Lou, X.F. Investigation of pore structure characteristics and adsorption characteristics of coals with different destruction types. Adsorp. Sci. Technol. 2019, 37, 623–648. [Google Scholar] [CrossRef][Green Version]
- Du, F.; Wang, K. Unstable failure of gas-bearing coal-rock combination bodies: Insights from physical experiments and numerical simulations. Process. Saf. Environ. Prot. 2019, 129, 264–279. [Google Scholar] [CrossRef]
- Wang, P.F.; Jiang, Z.X.; Chen, L.; Yin, L.S.; Li, Z.; Zhang, C.; Tang, X.L.; Wang, G.Z. Pore structure characterization for the Longmaxi and Niutitang shales in the Upper Yangtze Platform, South China: Evidence from focused ion beam–He ion microscopy, nano-computerized tomography and gas adsorption analysis. Mar. Pet. Geol. 2016, 77, 1323–1337. [Google Scholar] [CrossRef]
- Zhou, G.; Ma, Y.L.; Fan, T.; Wang, G. Preparation and characteristics of a multifunctional dust suppressant with agglomeration and wettability performance used in coal mine. Chem. Eng. Res. Des. 2018, 132, 729–742. [Google Scholar] [CrossRef]
- Ma, Y.L.; Zhou, G.; Ding, J.F.; Li, S.L.; Wang, G. Preparation and characterization of an agglomeration-cementing agent for dust suppression in open pit coal mining. Cellulose 2018, 25, 4011–4029. [Google Scholar] [CrossRef]
- Sun, M.D.; Yu, B.S.; Hu, Q.H.; Chen, S.; Xia, W.; Ye, R.C. Nanoscale pore characteristics of the Lower Cambrian Niutitang Formation Shale: A case study from Well Yuke #1 in the Southeast of Chongqing, China. Int. J. Coal Geol. 2016, 154, 16–29. [Google Scholar]
- Jouini, M.S.; Bouchaala, F.; Riahi, M.K.; Sassi, M.; Abderrahmane, H.; Hjouj, F. Multifractal Analysis of Reservoir Rock Samples Using 3D X-Ray Micro Computed Tomography Images. IEEE Access 2022, 10, 67898–67909. [Google Scholar] [CrossRef]
- Li, X.C.; Kang, Y.L.; Haghighi, M. Investigation of pore size distributions of coals with different structures by nuclear magnetic resonance (NMR) and mercury intrusion porosimetry (MIP). Measurement 2018, 116, 122–128. [Google Scholar] [CrossRef]
- Li, P.P.; Zhang, X.D.; Zhang, S. Structures and fractal characteristics of pores in low volatile bituminous deformed coals by low-temperature N2 adsorption after different solvents treatments. Fuel 2018, 224, 661–675. [Google Scholar] [CrossRef]
- Wang, S. Analysis of rock pore structural characteristic by nuclear magnetic resonance. Xinjiang Pet. Geol. 2009, 30, 768–771. [Google Scholar]
- Zhao, Y.X.; Sun, Y.F.; Liu, S.M.; Wang, K.; Jiang, Y.D. Pore structure characterization of coal by NMR cryoporometry. Fuel 2017, 190, 359–369. [Google Scholar] [CrossRef]
- Wang, Z.Y.; Cheng, Y.P.; Zhang, K.Z.; Hao, C.M.; Wang, L.; Li, W.; Hu, B. Characteristics of microscopic pore structure and fractal dimension of bituminous coal by cyclic gas adsorption/desorption: An experimental study. Fuel 2018, 32, 495–505. [Google Scholar] [CrossRef]
- Lin, H.Y.; Tian, S.X.; Jiao, A.J.; Cao, Z.Y.; Song, K.; Zou, Y.H. Pore Characteristics and Fractal Dimension Analysis of Tectonic Coal and Primary-Structure Coal: A Case Study of Sanjia Coal Mine in Northern Guizhou. ACS Omega 2022, 7, 27300–27311. [Google Scholar] [CrossRef]
- Bale, H.D.; Schmidt, P.W. Small-Angle X-ray-Scattering Investigation of Submicroscopic Porosity with Fractal Properties. Phys. Rev. Lett. 1984, 53, 596–599. [Google Scholar] [CrossRef]
- Han, W.B.; Zhou, G.; Gao, D.H.; Zhang, Z.X.; Wei, Z.Y.; Wang, H.T.; Yang, H.Q. Experimental analysis of the pore structure and fractal characteristics of different metamorphic coal based on mercury intrusion nitrogen adsorption porosimetry. Powder Technol. 2020, 362, 386–398. [Google Scholar] [CrossRef]
- Niu, Q.H.; Pan, J.N.; Jin, Y.; Wang, H.C.; Li, M.; Ji, Z.M.; Wang, K.; Wang, Z.Z. Fractal study of adsorption-pores in pulverized coals with various metamorphism degrees using N2 adsorption, X-ray scattering and image analysis methods. J. Pet. Sci. Eng. 2019, 176, 584–593. [Google Scholar] [CrossRef]
- Jiang, W.; Zhou, Y.; Wu, C.F.; Du, M.Y. Fractal characteristics and theirs influence on methane adsorption in high-rank coals with NMR. Front. Earth Sci. 2022, 10, 1047557. [Google Scholar] [CrossRef]
- Li, Z.Q.; Shen, X.; Qi, Z.Y.; Hu, R.L. Study on the pore structure and fractal characteristics of marine and continental shale based on mercury porosimetry, N2 adsorption and NMR methods. J. Nat. Gas. Sci. Eng. 2018, 53, 12–21. [Google Scholar] [CrossRef]
- Naveen, P.; Asif, M.; Ojha, K. Integrated fractal description of nanopore structure and its effect on CH4 adsorption on Jharia coals, India. Fuel 2018, 232, 190–204. [Google Scholar] [CrossRef]
- Zhu, J.F.; Liu, J.Z.; Yang, Y.M.; Cheng, J.; Zhou, J.H.; Cen, K.F. Fractal characteristics of pore structures in 13 coal specimens: Relationship among fractal dimension, pore structure parameter, and slurry ability of coal. Fuel Process. Technol. 2016, 149, 256–267. [Google Scholar] [CrossRef]
- Zhang, S.H.; Tang, S.H.; Tang, D.Z.; Huang, W.H.; Pan, Z.J. Determining fractal dimensions of coal pores by FHH model: Problems and effects. J. Pet. Sci. Eng. 2014, 21, 929–939. [Google Scholar] [CrossRef]
- Yan, M.; Luo, H.X.; Yang, T.; Yan, D.J.; Wei, J.N.; Lin, H.F.; Li, S.G. Experimental Study on Fractal Characteristics of Surface Roughness of Briquettes and their Effect on Wettability of Coal Samples. Nat. Resour. Res. 2023, 32, 1235–1249. [Google Scholar] [CrossRef]
- Ni, G.H.; Li, S.; Rahman, S.; Xun, M.; Wang, H.; Xu, Y.H.; Xie, H.C. Effect of nitric acid on the pore structure and fractal characteristics of coal based on the low-temperature nitrogen adsorption method. Powder Technol. 2020, 367, 506–516. [Google Scholar] [CrossRef]
- Wen, Z.H.; Jia, B.T.; Wang, J.W.; Ren, J.G.; Wang, Q. Dendric Fractal Characteristics of Pores in Anthracite and Their Influences on Gas Adsorption Characteristics. Geofluids 2022, 2022, 1465777. [Google Scholar] [CrossRef]
- Zhou, Q.; Qin, B.T.; Wang, J.; Wang, H.T.; Wang, F. Experimental investigation on the changes of the wettability and surface characteristics of coal dust with different fractal dimensions. Colloids Surf. A Physicochem. Eng. Asp. 2018, 551, 148–157. [Google Scholar] [CrossRef]
- Hodot, B.B. Coal and Gas Protrusion; Song, S.Z.; Wang, Y.A., Translators; China Industry Press: Beijing, China, 1966; pp. 27–30. [Google Scholar]
- Sing, K.S.W.; Everett, D.H.; Haul, R.A.W.; Moscou, L.; Pierotti, R.A.; Rouquerol, J.; Siemieniewska, T. Reporting physisorption data for gas/solid systems with special reference to the determination of surface area and porosity (Recommendations 1984). Pure Appl. Chem. 1985, 57, 603–619. [Google Scholar] [CrossRef]
- Dubinin, M.M. The potential theory of adsorption of gases and vapors for adsorbents with energetically nonuniform surfaces. Chem. Rev. 1960, 60, 235–241. [Google Scholar] [CrossRef]
- Gan, H.; Nandi, S.P.; Walker, P.L. Nature of porosities in the American coals. Fuel 1972, 51, 272–277. [Google Scholar] [CrossRef]
- Thommes, M.; Kaneko, K.; Neimark, A.V.; Olivier, J.P.; Reinoso, F.R.; Rouquerol, J.; Sing, K.S.W. Physisorption of gases, with special reference to the evaluation of surface area and pore size distribution (IUPAC Technical Report). Pure Appl. Chem. 2015, 87, 1051–1069. [Google Scholar] [CrossRef][Green Version]
- Liu, R.L.; Cheng, W.M.; Yu, Y.B.; Xu, Q.F.; Jiang, A.W.; Lv, T. An impacting factors analysis of miners’ unsafe acts based on HFACS-CM and SEM. Process. Saf. Environ. Prot. 2019, 122, 221–231. [Google Scholar] [CrossRef]
- Wang, Z.Y.; Cheng, Y.P.; Qi, Y.X.; Wang, R.P.; Wang, L.; Jiang, J.Y. Experimental study of pore structure and fractal characteristics of pulverized intact coal and tectonic coal by low temperature nitrogen adsorption. Powder Technol. 2019, 350, 15–25. [Google Scholar] [CrossRef]
- Lin, Y.B.; Qin, Y.; Ma, D.M.; Duan, Z.H. Pore structure, adsorptivity and influencing factors of high-volatile bituminous coal rich in inertinite. Fuel 2021, 293, 120418. [Google Scholar] [CrossRef]
- Hui, W.; Wang, Y.S.; Ren, D.Z.; Jin, H. Effects of pore structures on the movable fluid saturation in tight sandstones: A He8 formation example in Sulige Gasfield, Ordos Basin, China. J. Pet. Sci. Eng. 2020, 192, 107295. [Google Scholar] [CrossRef]
- Conner, W.C.; Lane, A.M.; Hoffman, A.J. Measurement of the morphology of high surface area solids: Hysteresis in mercury porosimetry. J. Colloid Interface Sci. 1984, 100, 185–193. [Google Scholar]
- Guo, H.J.; Yuan, L.; Cheng, Y.P.; Wang, K.; Xu, C. Experimental investigation on coal pore and fracture characteristics based on fractal theory. Powder Technol. 2019, 346, 341–349. [Google Scholar] [CrossRef]
- Chibowski, E.; Hołysz, L. On the use of Washburn’s equation for contact angle determination. J. Adhes. Sci. Technol. 1997, 11, 1289–1301. [Google Scholar] [CrossRef]
- Friesen, W.I.; Mikula, R.J. Fractal dimensions of coal particles. J. Colloid Interf. Sci. 1987, 120, 263–271. [Google Scholar] [CrossRef]



| Classification Scheme | Large Pores (nm) | Mesopores (nm) | Small Pores (nm) | Micropores (nm) |
|---|---|---|---|---|
| B.B. Hodot (1961) [33] | >1000 | 100–1000 | 10–100 | <10 |
| International Union of Pure and Applied Chemistry (IUPAC) [34] | >50 | - | 2–50 | <2 |
| Dubinin (1966) [35] | >20 | - | 2–20 | <2 |
| Gan et al. (1972) [36] | >30 | 1.2–30 | - | <1.2 |
| Coal Sample | Pore Volume (mL/g) | |
|---|---|---|
| Small Pores (10–100 nm) | Micropores (<10 nm) | |
| 3#-1 | 8.905 × 10−3 | 2.374 × 10−2 |
| 3#-2 | 9.871 × 10−3 | 2.559 × 10−2 |
| 3#-3 | 1.416 × 10−2 | 2.959 × 10−2 |
| 3#-4 | 1.477 × 10−2 | 3.795 × 10−2 |
| 5#-1 | 1.494 × 10−2 | 3.677 × 10−2 |
| 5#-2 | 8.229 × 10−3 | 2.112 × 10−2 |
| 5#-3 | 5.869 × 10−3 | 1.219 × 10−2 |
| 5#-4 | 5.780 × 10−3 | 1.322 × 10−2 |
| Coal Sample | Fractal Dimension | BET Multipoint Specific Surface Area (m2/g) | |
|---|---|---|---|
| D11 | D12 | ||
| 3#-1 | 1.1489 | 2.6217 | 16.7758 |
| 3#-2 | 1.2136 | 2.6258 | 18.2541 |
| 3#-3 | 1.0257 | 2.6262 | 22.7340 |
| 3#-4 | 1.2144 | 2.6349 | 28.8033 |
| 5#-1 | 1.1394 | 2.6326 | 28.9357 |
| 5#-2 | 0.5549 | 2.6066 | 15.1786 |
| 5#-3 | 1.0270 | 2.5771 | 7.70090 |
| 5#-4 | 0.9720 | 2.5900 | 8.71280 |
| Coal Sample | Specific Surface Area (m2/g) | Porosity (%) | ||
|---|---|---|---|---|
| Total Pores (<30,000 nm) | Large Pores (1000–30,000 nm) | Mesopores (100–1000 nm) | ||
| 3#-1 | 12.86705073 | 0.005097158 | 0.003117883 | 14.7828 |
| 3#-2 | 15.06439553 | 0.003307208 | 0.014297674 | 10.8212 |
| 3#-3 | 13.63198928 | 0.001443009 | 0.013683093 | 10.7816 |
| 3#-4 | 15.30721409 | 0.005480325 | 0.03635567 | 18.0309 |
| 5#-1 | 17.00766847 | 0.006037652 | 0.021649133 | 12.7001 |
| 5#-2 | 12.85019095 | 0.002589737 | 0.010751821 | 14.5666 |
| 5#-3 | 14.36497538 | 0.004481844 | 0.01019947 | 21.7671 |
| 5#-4 | 17.79941884 | 0.005771029 | 0.011007771 | 13.7139 |
| Coal Sample | Porosity | Intensity Range for Interactive Thresholds | ||
|---|---|---|---|---|
| Total Pores | Connected Pores | Isolated Pores | ||
| 3#-1 | 0.69971764 | 0.698573 | 0.001145 | 0–20575 |
| 3#-2 | 0.74401748 | 0.741861 | 0.002157 | 0–8285 |
| 3#-3 | 0.80857825 | 0.807941 | 0.000638 | 0–8900 |
| 3#-4 | 0.30400881 | 0.300162 | 0.003847 | 0–9109 |
| 5#-1 | 0.84482800 | 0.844460 | 0.000369 | 0–8280 |
| 5#-2 | 0.50021219 | 0.497618 | 0.002594 | 0–5800 |
| 5#-3 | 0.62289700 | 0.621812 | 0.006962 | 0–9650 |
| 5#-4 | 0.58887321 | 0.586356 | 0.002518 | 0–7096 |
| Coal Sample | Fractal Dimension | Specific Surface Area (m2/g) |
|---|---|---|
| 3#-1 | 2.46580148 | 0.034228548 |
| 3#-2 | 2.31770778 | 0.024005108 |
| 3#-3 | 2.47354388 | 0.035068851 |
| 3#-4 | 2.57114244 | 0.080894694 |
| 5#-1 | 2.45474815 | 0.028446458 |
| 5#-2 | 2.58994627 | 0.083772153 |
| 5#-3 | 2.49323893 | 0.053474970 |
| 5#-4 | 2.5432539 | 0.063848071 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, X.; Ma, R.; Wu, J.; Sun, J. Fractal Analysis of Coal Pore Structure Based on Computed Tomography and Fluid Intrusions. Fractal Fract. 2023, 7, 439. https://doi.org/10.3390/fractalfract7060439
Chen X, Ma R, Wu J, Sun J. Fractal Analysis of Coal Pore Structure Based on Computed Tomography and Fluid Intrusions. Fractal and Fractional. 2023; 7(6):439. https://doi.org/10.3390/fractalfract7060439
Chicago/Turabian StyleChen, Xuexi, Ruiyue Ma, Jinsui Wu, and Jihong Sun. 2023. "Fractal Analysis of Coal Pore Structure Based on Computed Tomography and Fluid Intrusions" Fractal and Fractional 7, no. 6: 439. https://doi.org/10.3390/fractalfract7060439
APA StyleChen, X., Ma, R., Wu, J., & Sun, J. (2023). Fractal Analysis of Coal Pore Structure Based on Computed Tomography and Fluid Intrusions. Fractal and Fractional, 7(6), 439. https://doi.org/10.3390/fractalfract7060439





