On the Roots of a Family of Polynomials
Abstract
1. Introduction
- We introduce the family of polynomials obtained by composing the elementary polynomials and .
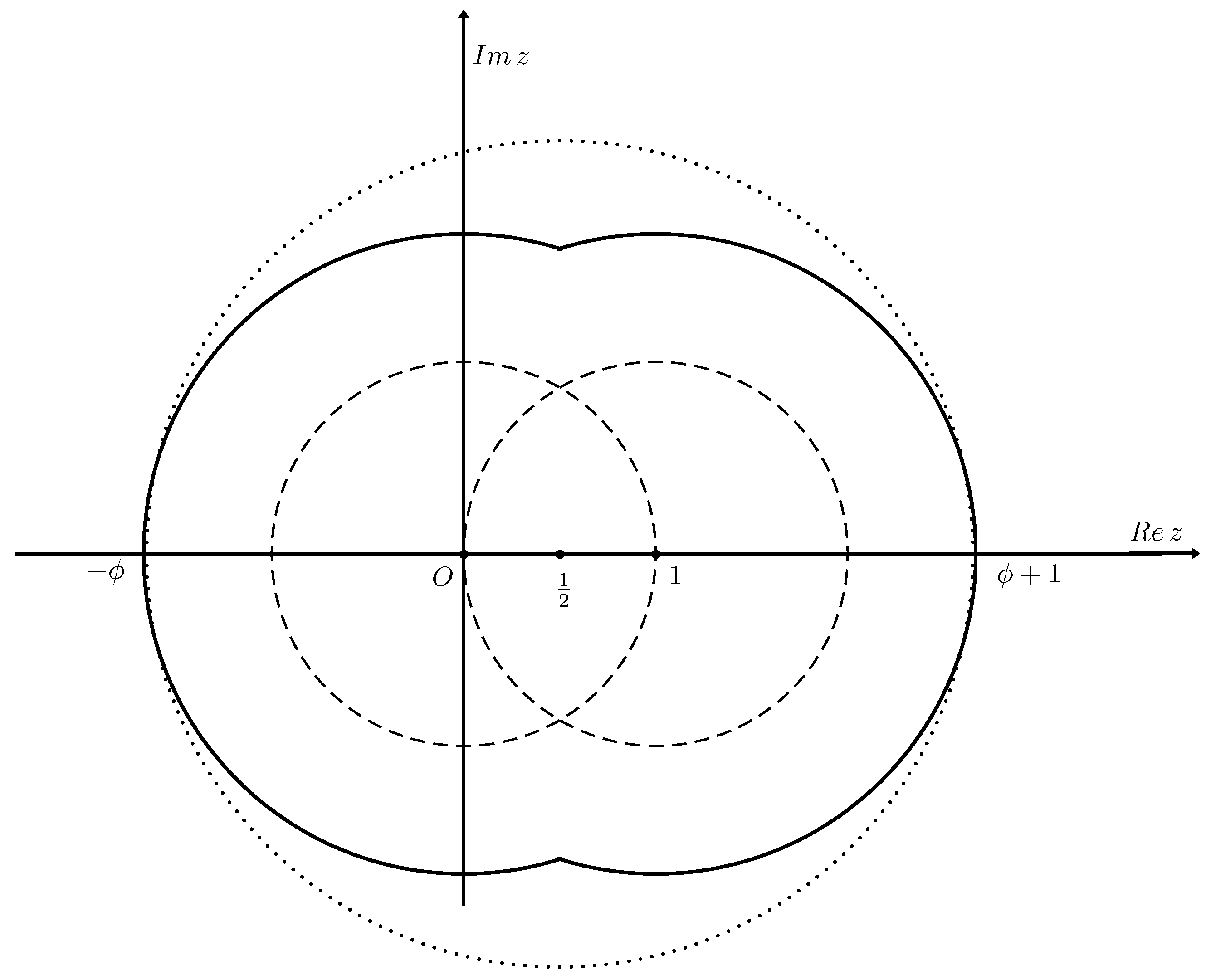
- We prove that the set of roots of this infinite family of polynomials is bounded by the circles and . A direct consequence is that the roots of the reliability polynomials of series-parallel composition networks are bounded.
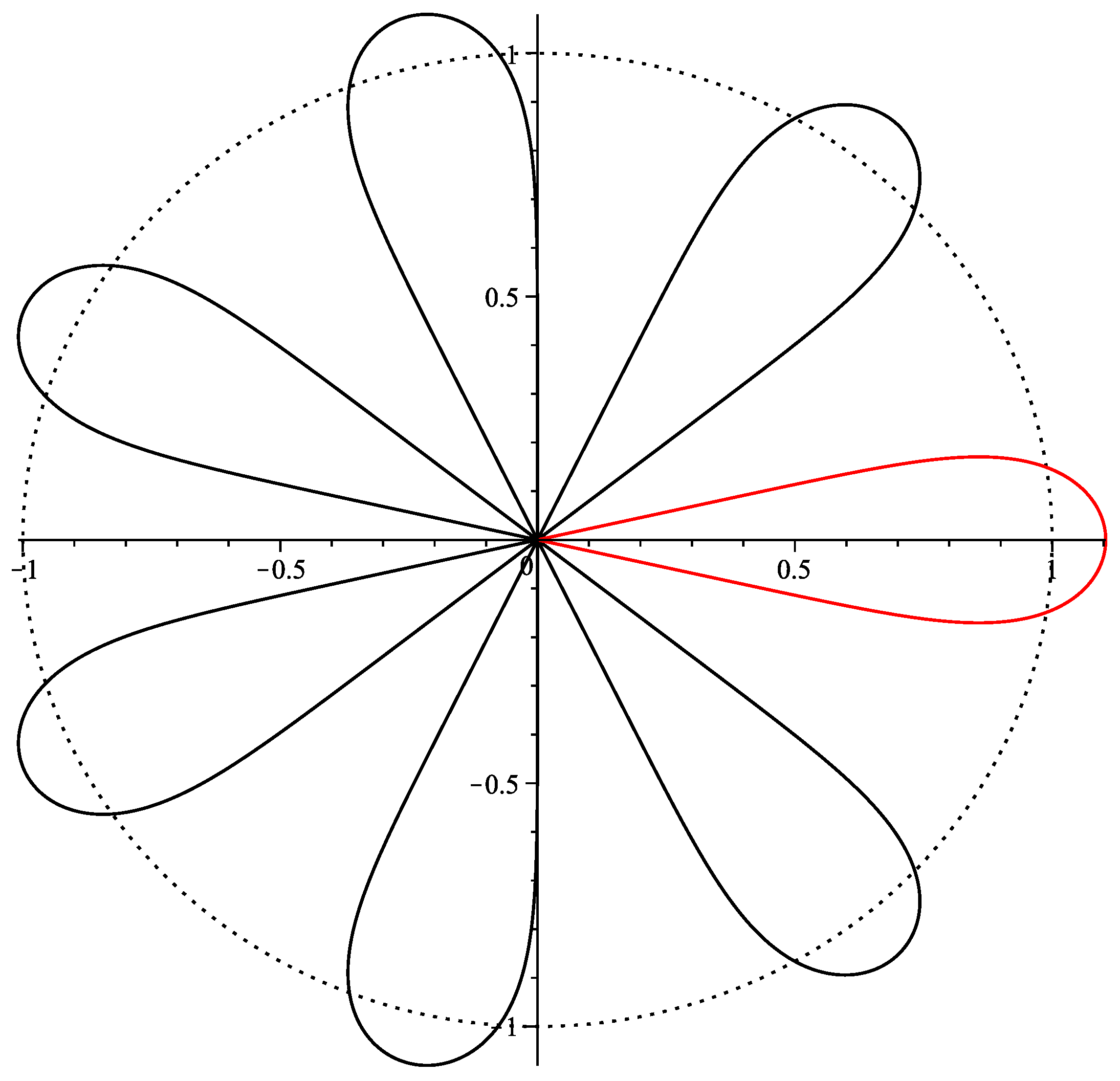
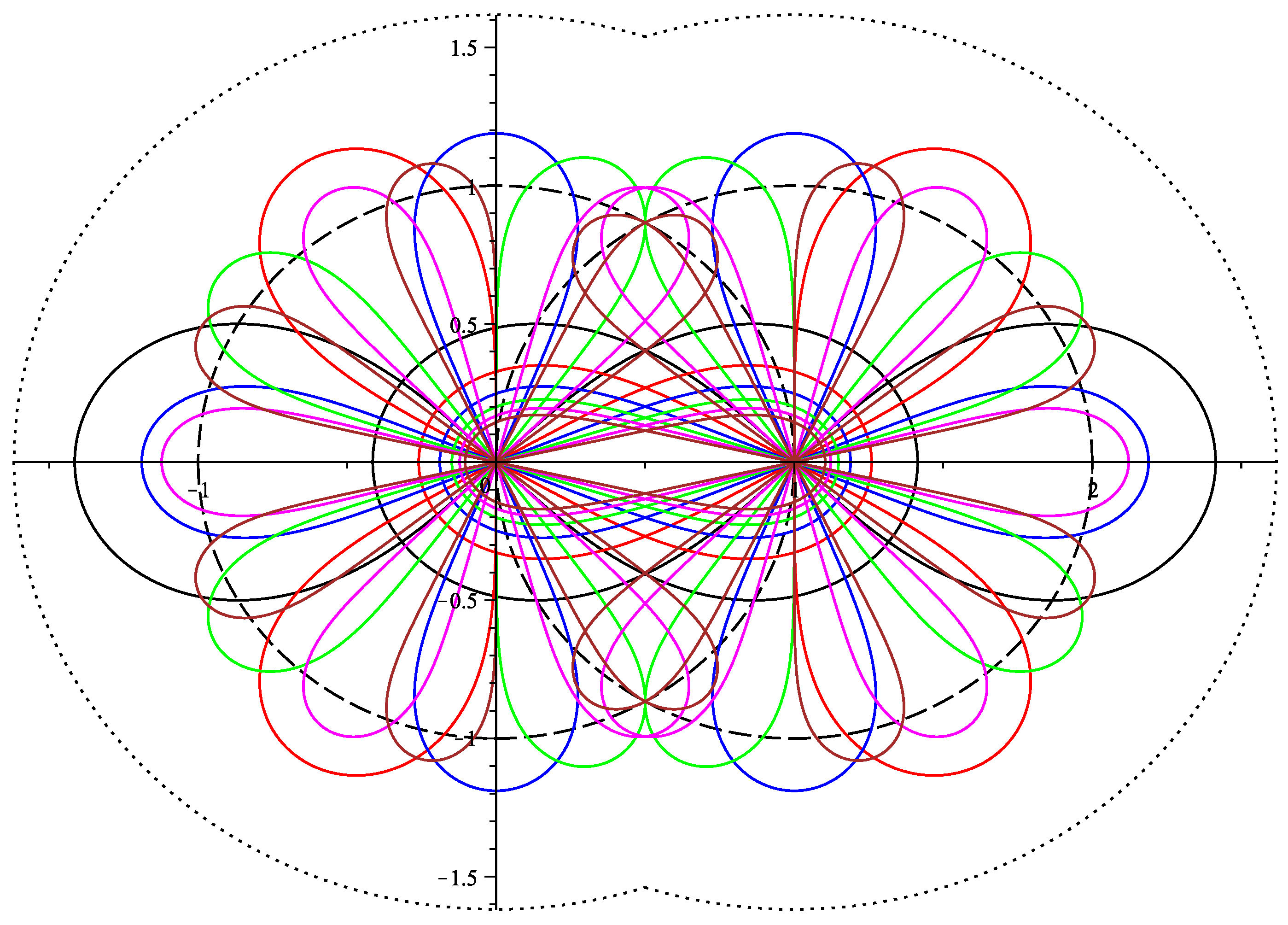
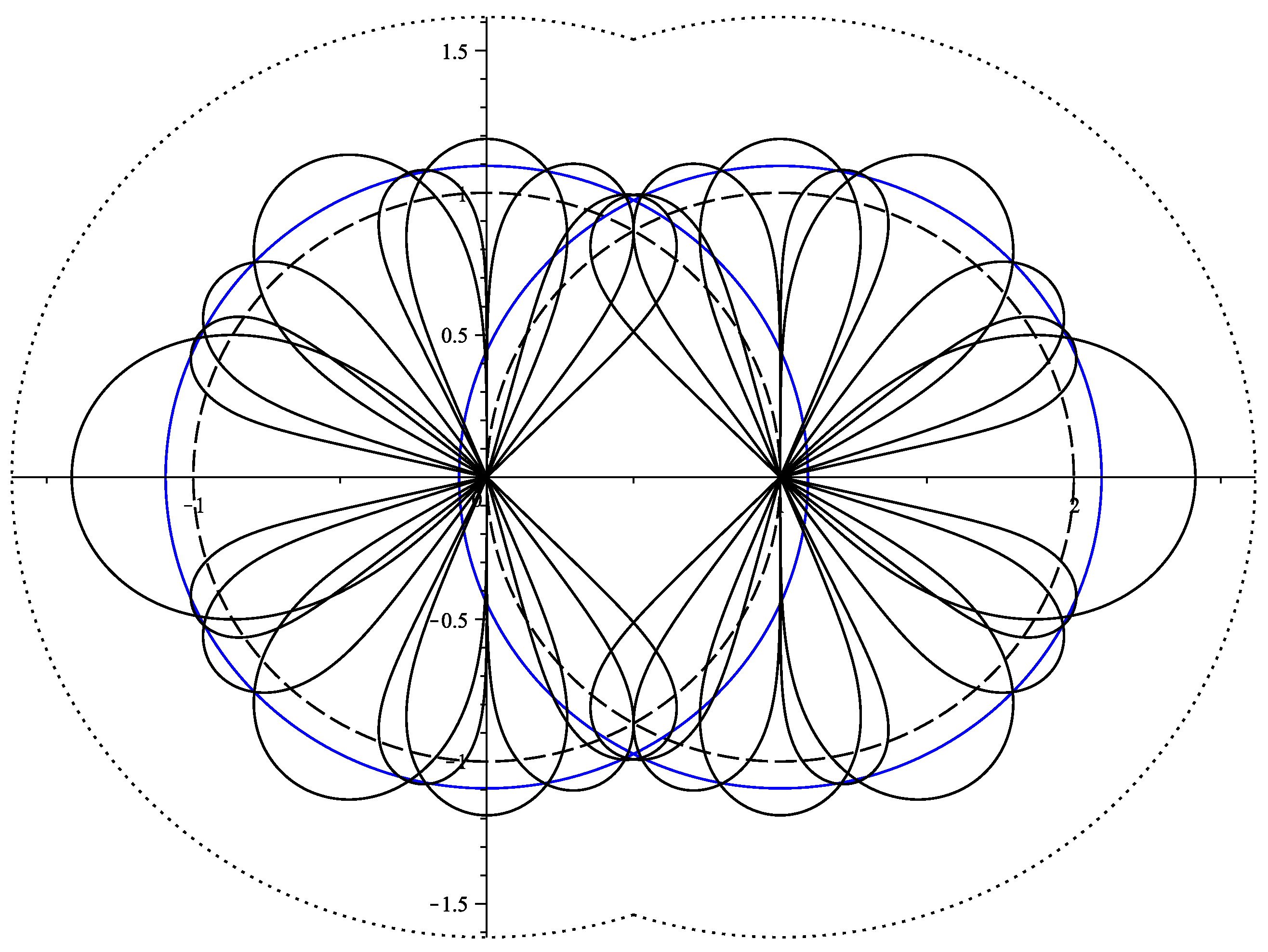
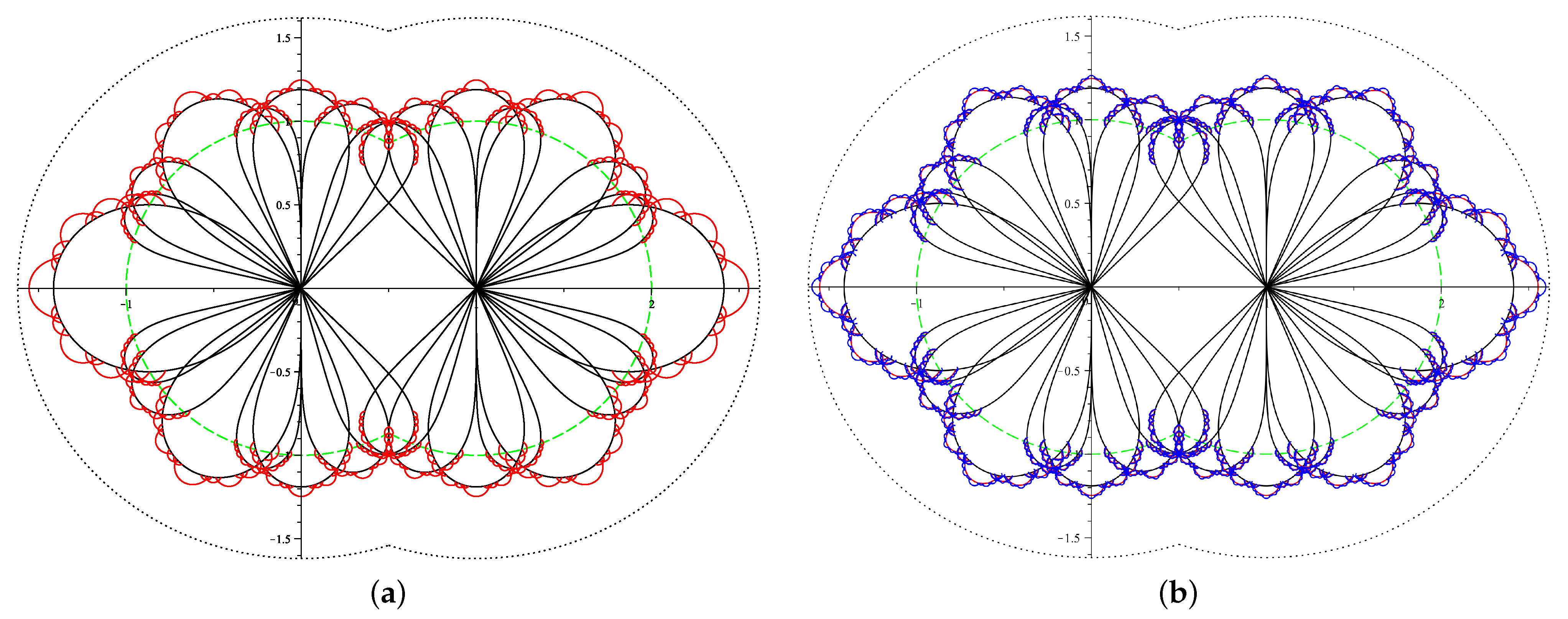
- Starting from the result proved by Brown and deGagné [21,23], we show that the closure of the set of roots of the polynomials in contains the domain bounded by the lemniscates with n poles centered at 0 and at 1; hence, it contains two disks of radius slightly greater than 1, centered at 0 and at 1.
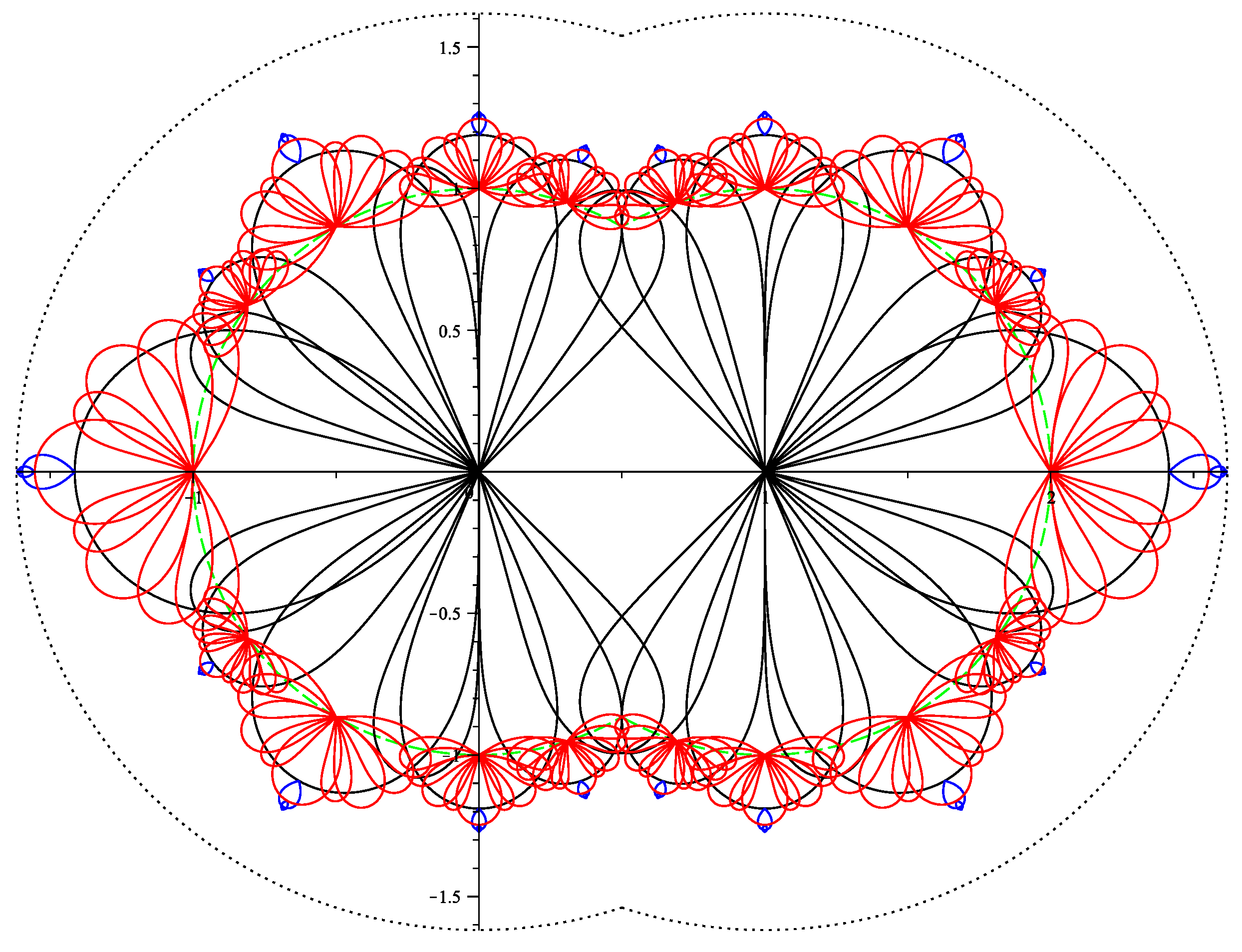
- We find 16 complex limit points of the set of roots apart from and , the two limit points on the real axis noted in [21].
2. Preliminaries
- (i)
- (the sets and are symmetric to each other with respect to );
- (ii)
- .
- (iii)
- and , .
- (i)
- If k is even then , and f is strictly increasing on .
- (ii)
- If k is odd then , and f is strictly decreasing on .
3. Bounds for the Set of Roots
4. The Closure of the Set of Roots
5. Limit Points
6. Discussion and Conclusions
- What is the maximum radius of the two disks contained into the closure of the set of roots?
- How can one find other limit points, apart from the ones presented in the last section of the paper?
- The real roots are proved to be in , and the points and are proved to be limit points. Is the set of real roots dense in ?
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cauchy, A.L. Exercises de Mathématique; Année 4. De Bure Frères: Paris, France, 1829. [Google Scholar]
- Gardner, R.; Govil, N.K. The Eneström–Kakeya Theorem and some of its generalizations. In Current Topics in Pure and Computational Complex Analysis; Joshi, S., Dorff, M., Lahiri, I., Eds.; Springer: New Delhi, India, 2014; pp. 171–200. [Google Scholar]
- Marden, M. Geometry of Polynomials, 2nd ed.; American Mathematical Society: Providence, RI, USA, 1966. [Google Scholar]
- Anderson, N.; Saff, E.B.; Varga, R.S. On the Enestróm-Kakeya theorem and its sharpness. Linear Algebra Appl. 1979, 28, 5–16. [Google Scholar] [CrossRef]
- Rahman, Q.I.; Schemeisser, G. Analytic Theory of Polynomials; London Mathematical Society Monographs (New Series 26); Oxford University Press: Oxford, UK, 2002. [Google Scholar]
- Moore, E.F.; Shannon, C.E. Reliable circuits using less reliable relays—Part I. J. Frankl. Inst. 1956, 262, 191–208. [Google Scholar] [CrossRef]
- Colbourn, C.J. The Combinatorics of Network Reliability; Oxford University Press: New York, NY, USA, 1987. [Google Scholar]
- Colbourn, C.J. Combinatorial aspects of network reliability. Ann. Oper. Res. 1991, 33, 1–15. [Google Scholar] [CrossRef]
- Valiant, C. The complexity of enumeration and reliability problems. SIAM J. Comput. 1979, 8, 410–421. [Google Scholar] [CrossRef]
- Beiu, V.; Drăgoi, V.F.; Beiu, R.M. Why reliability for computing needs rethinking. In Proceedings of the 2020 International Conference on Rebooting Computing (ICRC), Atlanta, GA, USA, 1–3 December 2020; IEEE: Piscataway, NJ, USA, 2020; pp. 16–25. [Google Scholar]
- Cristescu, G.; Drăgoi, V.F. Efficient approximation of two-terminal networks reliability polynomials using cubic splines. IEEE Trans. Reliab. 2021, 70, 1193–1203. [Google Scholar] [CrossRef]
- Jianu, M.; Ciuiu, D.; Dăuş, L.; Jianu, M. Markov chain method for computing the reliability of hammock networks. Probab. Eng. Inf. Sci. 2022, 36, 276–293. [Google Scholar] [CrossRef]
- Cowell, S.R.; Hoară, S.; Beiu, V. Experimenting with beta distributions for approximating hammocks’ reliability. In Intelligent Methods in Computing, Communications and Control, Proceedings of the 8th International Conference on Computers Communications and Control (ICCCC 2020), Oradea, Romania, 11–15 May 2020; Springer: Cham, Switzerland, 2021; pp. 70–81. [Google Scholar]
- Jianu, M.; Dăuş, L.; Hoară, S.H.; Beiu, V. Using Delta-Wye transformations for estimating networks’ reliability. In Intelligent Methods Systems and Applications in Computing, Communications and Control, Proceedings of the 9th International Conference on Computers Communications and Control (ICCCC 2022), Oradea, Romania, 16–20 May 2022; Springer: Cham, Switzerland, 2023; pp. 415–426. [Google Scholar]
- Dăuş, L.; Jianu, M. Full Hermite interpolation of the reliability of a hammock network. Appl. Anal. Discret. Math. 2020, 14, 198–220. [Google Scholar] [CrossRef]
- Cristescu, G.; Drăgoi, V.F.; Hoară, S.H. Generalized convexity properties and shape-based approximation in networks reliability. Mathematics 2021, 9, 3182. [Google Scholar] [CrossRef]
- Dăuş, L.; Jianu, M. The shape of the reliability polynomial of a hammock network. In Intelligent Methods in Computing, Communications and Control, Proceedings of the 8th International Conference on Computers Communications and Control (ICCCC 2020), Oradea, Romania, 11–15 May 2020; Springer: Cham, Switzerland, 2021; pp. 93–105. [Google Scholar]
- Graves, C.; Milan, D. Reliability polynomials having arbitrarily many inflection points. Networks 2014, 64, 1–5. [Google Scholar] [CrossRef]
- Mol, L. On Connectedness and Graph Polynomials. Ph.D. Thesis, Dalhousie University, Halifax, NS, Canada, 2016. [Google Scholar]
- Brown, J.I.; Colbourn, C.J. Roots of the reliability polynomial. SIAM J. Discr. Math. 1992, 5, 571–585. [Google Scholar] [CrossRef]
- Brown, J.I.; DeGagné, C.D.C. Roots of two-terminal reliability polynomials. Networks 2021, 78, 153–163. [Google Scholar] [CrossRef]
- Dăuş, L.; Drăgoi, V.F.; Jianu, M.; Bucerzan, D.; Beiu, V. On the roots of certain reliability polynomials. In Intelligent Methods Systems and Applications in Computing, Communications and Control, Proceedings of the 9th International Conference on Computers Communications and Control (ICCCC 2022), Oradea, Romania, 16–20 May 2022; Springer: Cham, Switzerland, 2023; pp. 401–414. [Google Scholar]
- DeGagné, C.D.C. Network Reliability, Simplicial Complexes, and Polynomial Roots. Ph.D. Thesis, Dalhousie University, Halifax, NS, Canada, 2020. [Google Scholar]
- Royle, G.; Sokal, A.D. The Brown–Colbourn conjecture on zeros of reliability polynomials is false. J. Comb. Theory 2004, 91, 345–360. [Google Scholar] [CrossRef]
- Birkhoff, G.D. A determinantal formula for the number of ways of coloring a map. Ann. Math. 1912, 14, 42–46. [Google Scholar] [CrossRef]
- Cameron, P.J.; Morgan, K. Algebraic properties of chromatic roots. Electron. J. Comb. 2017, 24, 1–21. [Google Scholar] [CrossRef] [PubMed]
- Jackson, B. A zero-free interval for chromatic polynomials of graphs. Comb. Probab. Comput. 1993, 2, 325–336. [Google Scholar] [CrossRef]
- Sokal, A.D. Chromatic roots are dense in the whole complex plane. Comb. Probab. Comput. 2004, 13, 221–261. [Google Scholar] [CrossRef]
- Thomassen, C. The zero-free intervals for chromatic polynomials of graphs. Comb. Probab. Comput. 1997, 6, 497–506. [Google Scholar] [CrossRef]
- Gutman, I.; Harary, F. Generalizations of the matching polynomial. Util. Math. 1983, 24, 97–106. [Google Scholar]
- Brown, J.I.; Hickman, C.A.; Nowakowski, R.J. On the location of roots of independence polynomials. J. Algebr. Comb. 2004, 19, 273–282. [Google Scholar] [CrossRef]
- Brown, J.I.; Cameron, B. A note on purely imaginary independence roots. Discrete Math. 2020, 343, 112113. [Google Scholar] [CrossRef]
- Arocha, J.L.; Llano, B. Mean value for the matching and dominating polynomial. Discuss. Math. Graph Theory 2000, 20, 57–69. [Google Scholar] [CrossRef]
- Beaton, I.; Brown, J.I. On the real roots of domination polynomials. Contrib. Discret. Math. 2021, 16, 175–182. [Google Scholar]
- Beaton, I.; Brown, J.I.; Cox, D. Optimal domination polynomials. Graph. Combinator. 2020, 36, 1477–1487. [Google Scholar] [CrossRef]
- Beaton, I.; Brown, J.I. On the unimodality of domination polynomials. Graphs Comb. 2022, 38, 90. [Google Scholar] [CrossRef]
- Brown, J.I.; Tufts, J. On the roots of domination polynomials. Graphs Comb. 2014, 30, 527–547. [Google Scholar] [CrossRef]
- Drăgoi, V.F.; Beiu, V. Fast reliability ranking of matchstick minimal networks. Networks 2022, 79, 479–500. [Google Scholar] [CrossRef]
- Drăgoi, V.F.; Cowell, S.R.; Beiu, V. Ordering series and parallel compositions. In Proceedings of the 2018 IEEE 18th International Conference on Nanotechnology (IEEE-NANO), Cork, Ireland, 23–26 July 2018; IEEE: Piscataway, NJ, USA, 2019; pp. 1–4. [Google Scholar]
- Drăgoi, V.F.; Cowell, S.R.; Beiu, V.; Hoară, S.; Gaspar, P. How reliable are compositions of series and parallel networks compared with hammocks? Int. J. Comput. Commun. Control 2018, 13, 772–791. [Google Scholar] [CrossRef]
- Beardon, A. Iteration of Rational Functions; Springer: New York, NY, USA, 1991. [Google Scholar]
- Lawrence, J.D. A Catalog of Special Plane Curves; Dover: New York, NY, USA, 1972; p. 184. [Google Scholar]
- Lockwood, E.H. A Book of Curves; Cambridge University Press: London, UK, 1961; p. 175. [Google Scholar]
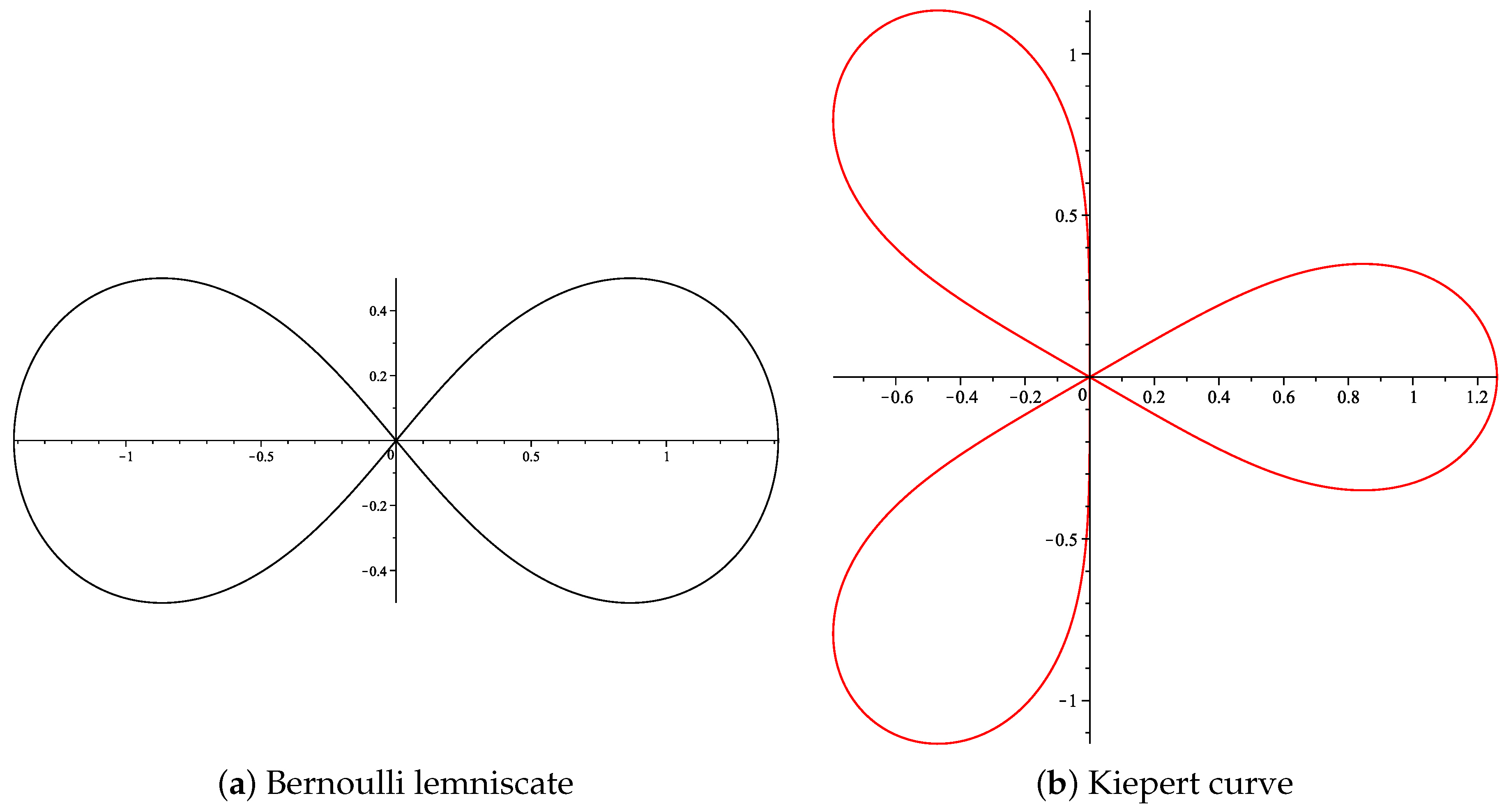
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jianu, M. On the Roots of a Family of Polynomials. Fractal Fract. 2023, 7, 339. https://doi.org/10.3390/fractalfract7040339
Jianu M. On the Roots of a Family of Polynomials. Fractal and Fractional. 2023; 7(4):339. https://doi.org/10.3390/fractalfract7040339
Chicago/Turabian StyleJianu, Marilena. 2023. "On the Roots of a Family of Polynomials" Fractal and Fractional 7, no. 4: 339. https://doi.org/10.3390/fractalfract7040339
APA StyleJianu, M. (2023). On the Roots of a Family of Polynomials. Fractal and Fractional, 7(4), 339. https://doi.org/10.3390/fractalfract7040339






