Mittag–Leffler Functions in Discrete Time
Abstract
1. Introduction
2. Preliminaries
- 1.
- , .
- 2.
- , .
3. -Discrete Mittag–Leffler Function
- 1.
- For , , .
- 2.
- For , , .
- 3.
- For and , , .
- 4.
- For and , , .
- 5.
- For and , is monotone increasing on .
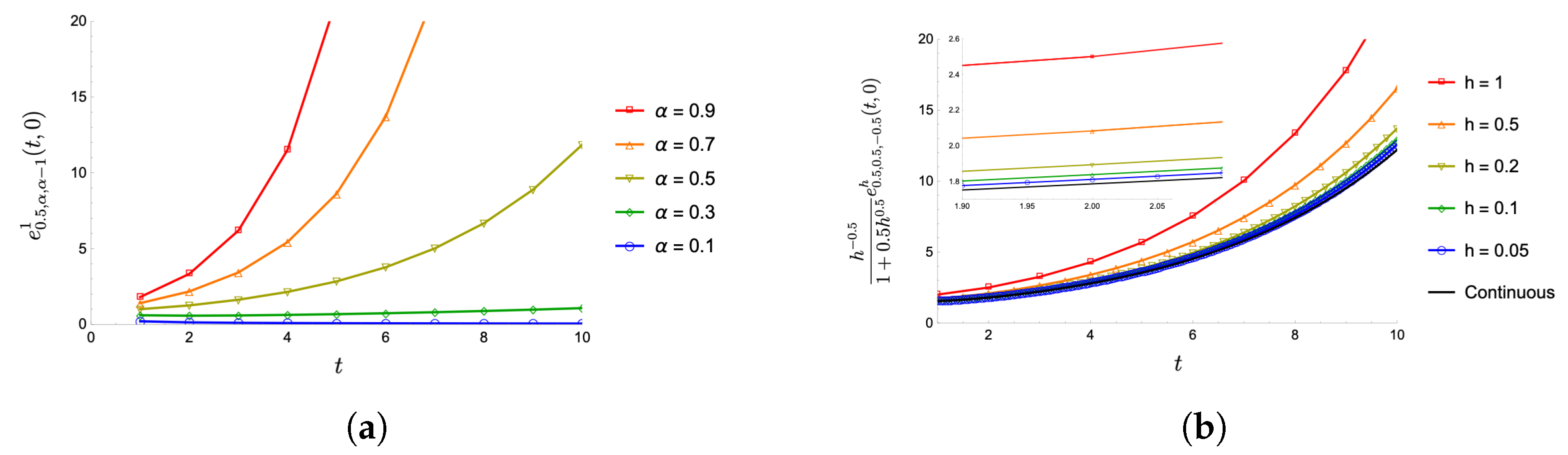
3.1. A Way to Compute
3.2. An Initial Value Problem
4. Matrix -Discrete Mittag–Leffler Function
- 1.
- .
- 2.
- , .
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Mittag–Leffler, G.M. Sur la nouvelle fonction eγ(x). C. R. Acad. Sci. Paris 1903, 137, 554–558. [Google Scholar]
- Adel, A.A.; Tamer, M.S.; Abdelhamid, A. Third-order differential subordination for meromorphic functions associated with generalized Mittag–Leffler function. Fractal Fract. 2023, 7, 175. [Google Scholar]
- Gorenflo, R.; Kilbas, A.A.; Mainardi, F. Mittag–Leffler Functions, Related Topics and Applications; Springer: Berlin/Heidelberg, Germany, 2014. [Google Scholar]
- Gorenflo, R.; Mainardi, F.; Rogosin, S. Mittag–Leffler function: Properties and applications. Handb. Fract. Calc. Appl. 2019, 1, 269–296. [Google Scholar]
- Haubold, H.J.; Mathai, A.M.; Saxena, R.K. Mittag–Leffler functions and their applications. J. Appl. Math. 2011, 2011, 298628. [Google Scholar] [CrossRef]
- Nagai, A. Discrete Mittag–Leffler function and its applications. Publ. Res. Inst. Math. Sci. Kyoto Univ. 2003, 1302, 1–20. [Google Scholar]
- Rogosin, S. The role of the Mittag–Leffler function in fractional modeling. Mathematics 2015, 3, 368–381. [Google Scholar] [CrossRef]
- Saenko, V.V. The calculation of the Mittag–Leffler function. Int. J. Comput. Math. 2022, 99, 1367–1394. [Google Scholar] [CrossRef]
- Saima, R.; Zakia, H.; Rehana, A.; Yu-Ming, C. New computation of unified bounds via a more general fractional operator using generalized Mittag–Leffler function in the kernel. Comput. Model. Eng. Sci. 2021, 126, 359–378. [Google Scholar]
- Garrappa, R. Numerical evaluation of two and three parameter Mittag–Leffler functions. SIAM J. Numer. Anal. 2015, 53, 1350–1369. [Google Scholar] [CrossRef]
- Garrappa, R.; Popolizio, M. Computing the matrix Mittag–Leffler function with applications to fractional calculus. J. Sci. Comput. 2018, 77, 129–153. [Google Scholar] [CrossRef]
- Hilfer, R.; Seybold, H.J. Computation of the generalized Mittag–Leffler function and its inverse in the complex plane. Integral Transform. Spec. Funct. 2006, 17, 637–652. [Google Scholar] [CrossRef]
- Li, A.; Wei, Y.; Li, Z.; Wang, Y. The numerical algorithms for discrete Mittag–Leffler functions approximation. Fract. Calc. Appl. Anal. 2019, 22, 95–112. [Google Scholar] [CrossRef]
- Naz, S.; Yu-Ming, C. A unified approach for novel estimates of inequalities via discrete fractional calculus techniques. Alex. Eng. J. 2022, 61, 847–854. [Google Scholar] [CrossRef]
- Popolizio, M. On the matrix Mittag–Leffler function: Theoretical properties and numerical computation. Mathematics 2019, 7, 1140. [Google Scholar] [CrossRef]
- Saima, R.; Yu-Ming, C.; Singh, J.; Kumar, D. A unifying computational framework for novel estimates involving discrete fractional calculus approaches. Alex. Eng. J. 2021, 60, 2677–2685. [Google Scholar]
- Seybold, H.; Hilfer, R. Numerical algorithm for calculating the generalized Mittag–Leffler function. SIAM J. Numer. Anal. 2008, 47, 69–88. [Google Scholar] [CrossRef]
- Wu, G.C.; Baleanu, D.; Zeng, S.D.; Luo, W.H. Mittag–Leffler function for discrete fractional modelling. J. King Saud Univ. Sci. 2016, 28, 99–102. [Google Scholar] [CrossRef]
- Atangana, A. Application of fractional calculus to epidemiology. In Fractional Dynamics; De Gruyter Open Poland: Warsaw, Poland, 2015; pp. 174–190. [Google Scholar]
- Atıcı, F.M.; Nguyen, N.; Dadashova, K.; Pedersen, S.E.; Koch, G. Pharmacokinetics and pharmacodynamics models of tumor growth and anticancer effects in discrete time. Comput. Math. Biophys. 2020, 8, 114–125. [Google Scholar] [CrossRef]
- Khan, A.; Bai, X.; Ilyas, M.; Rauf, A.; Xie, W.; Yan, P.; Zhang, B. Design and application of an interval estimator for nonlinear discrete-time SEIR epidemic models. Fractal Fract. 2022, 6, 213. [Google Scholar] [CrossRef]
- Kumar, S.; Pandey, R.K.; Kumar, K.; Kamal, S.; Dinh, T.N. Finite difference- collocation method for the generalized fractional diffusion equation. Fractal Fract. 2022, 6, 387. [Google Scholar] [CrossRef]
- Magin, R. Fractional Calculus in Bioengineering; Begell House Publishers Inc.: Danbury, CT, USA, 2004. [Google Scholar]
- Makhlouf, A.B.; Baleanu, D. Finite time stability of fractional order systems of neutral type. Fractal Fract. 2022, 6, 289. [Google Scholar] [CrossRef]
- Muresan, C.I.; Ostalczyk, P.; Ortigueira, M.D. Fractional calculus applications in modeling and design of control systems. J. Appl. Nonlinear Dyn. 2017, 6, 131–134. [Google Scholar] [CrossRef]
- Ostalczyk, P. Discrete Fractional Calculus: Applications in Control and Image Processing; World Scientific: Singapore, 2015. [Google Scholar]
- Podlubny, I. Fractional Differential Equations; Academic Press: New York, NY, USA, 1999. [Google Scholar]
- Samko, G.; Kilbas, A.A.; Marichev, O.I. Fractional Integrals and Derivatives: Theory and Applications; Gordon and Breach: Yverdon, Switzerland, 1993. [Google Scholar]
- Sopasakis, P.; Sarimveis, H.; Macheras, P.; Dokoumetzidis, A. Fractional calculus in pharmacokinetics. J. Pharmacokinet Pharmacodyn. 2018, 45, 107–125. [Google Scholar] [CrossRef]
- Podlubny, I. Matrix approach to discrete fractional calculus. Fract. Calc. Appl. Anal. 2000, 3, 359–386. [Google Scholar]
- Atıcı, F.M.; Chang, S.; Jonnalagadda, J. Grünwald-Letnikov fractional operators: From past to present. Fract. Differ. Calc. 2021, 11, 147–159. [Google Scholar] [CrossRef]
- Atıcı, F.M.; Dadashova, K.; Jonnalagadda, J. Linear fractional order h-difference equations. Int. J. Differ. Equ. (Special Issue Honor. Profr. Johnny Henderson) 2020, 15, 281–300. [Google Scholar]
- Atıcı, F.M.; Jonnalagadda, J.M. An eigenvalue problem in fractional h-discrete calculus. Fract. Calc. Appl. Anal. 2022, 25, 630–647. [Google Scholar] [CrossRef]
- Jonnalagadda, J.M.; Alzabut, J. Numerical computation of exponential functions in frame of nabla fractional calculus. Comput. Methods Differ. Equ. 2023, 11, 291–302. [Google Scholar] [CrossRef]
- Horn, R.A.; Johnson, C.R. Matrix Analysis, 2nd ed.; Cambridge University Press: Cambridge, MA, USA, 2013. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Atıcı, F.M.; Chang, S.; Jonnalagadda, J.M. Mittag–Leffler Functions in Discrete Time. Fractal Fract. 2023, 7, 254. https://doi.org/10.3390/fractalfract7030254
Atıcı FM, Chang S, Jonnalagadda JM. Mittag–Leffler Functions in Discrete Time. Fractal and Fractional. 2023; 7(3):254. https://doi.org/10.3390/fractalfract7030254
Chicago/Turabian StyleAtıcı, Ferhan M., Samuel Chang, and Jagan Mohan Jonnalagadda. 2023. "Mittag–Leffler Functions in Discrete Time" Fractal and Fractional 7, no. 3: 254. https://doi.org/10.3390/fractalfract7030254
APA StyleAtıcı, F. M., Chang, S., & Jonnalagadda, J. M. (2023). Mittag–Leffler Functions in Discrete Time. Fractal and Fractional, 7(3), 254. https://doi.org/10.3390/fractalfract7030254









