First-of-Its-Kind Frequency Enhancement Methodology Based on an Optimized Combination of FLC and TFOIDFF Controllers Evaluated on EVs, SMES, and UPFC-Integrated Smart Grid
Abstract
1. Introduction
1.1. Motivation and Incitement
1.2. Literature Review
1.3. Contribution and Paper Organization
- The suggestion of a control structure that combines the benefits of tilt, fuzzy logic, FOPID, and fractional filter regulators in a single controller known as FTFOIDFF that efficiently improves frequency stability in a hybrid two-area linked power system incorporated with severe RES penetrations.
- Utilization of a nature-inspired metaheuristic optimization technique that was recently developed (i.e., the prairie dog optimizer, or PDO) for the purpose of fine-tuning the recommended controller settings as well as the input scaling factors and membership functions for both FLC inputs and outputs in an effective manner.
- Validation of the beneficial influence of the integration of SMES, UPFC, and EVs in enhancing frequency performance during load perturbations and RESs penetrations.
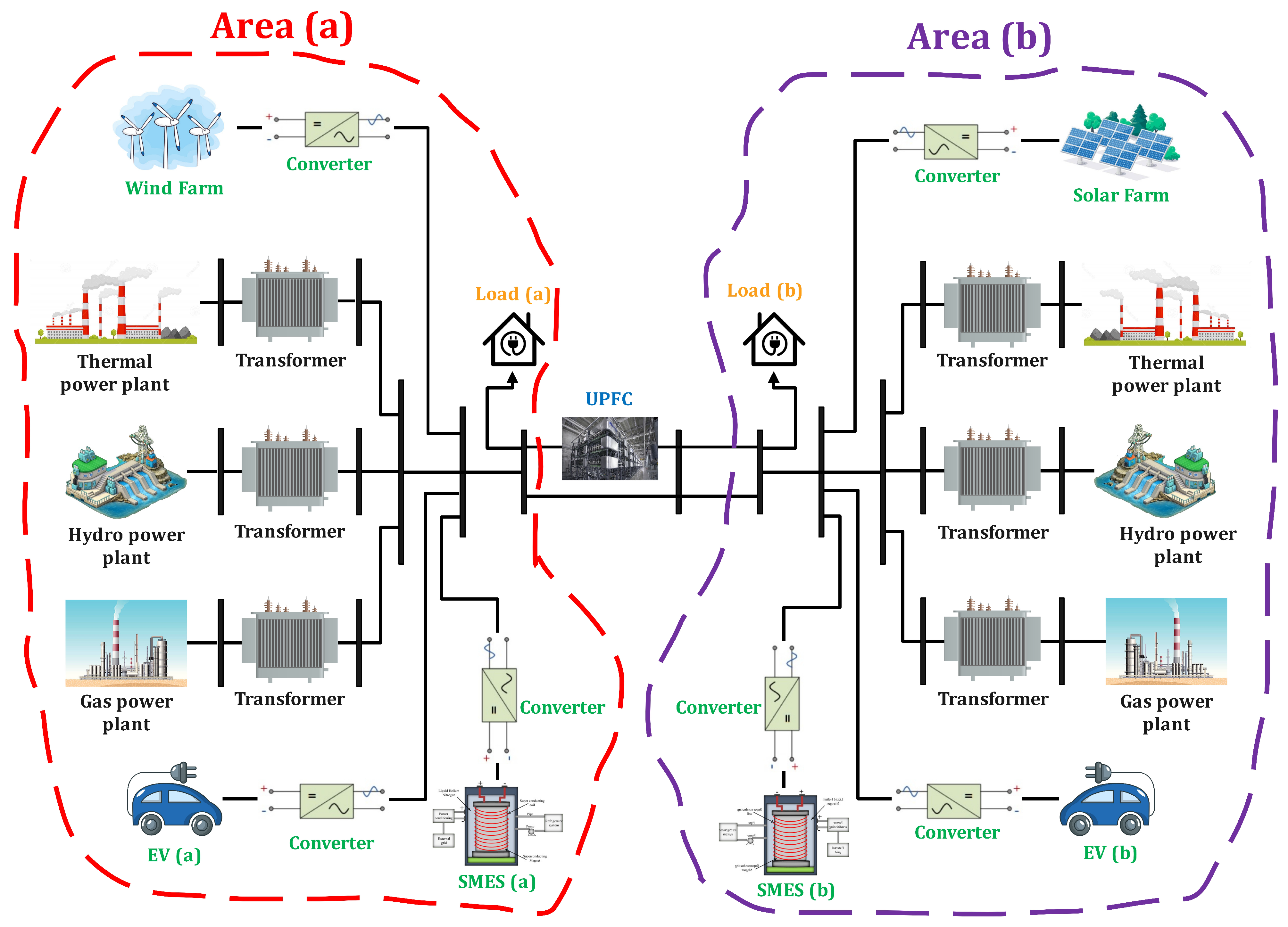
2. Modelling of the Investigated Hybrid Power System with SMES, UPFC and EVs
2.1. The Power System Structure
2.2. Mathematical Representation of UPFC
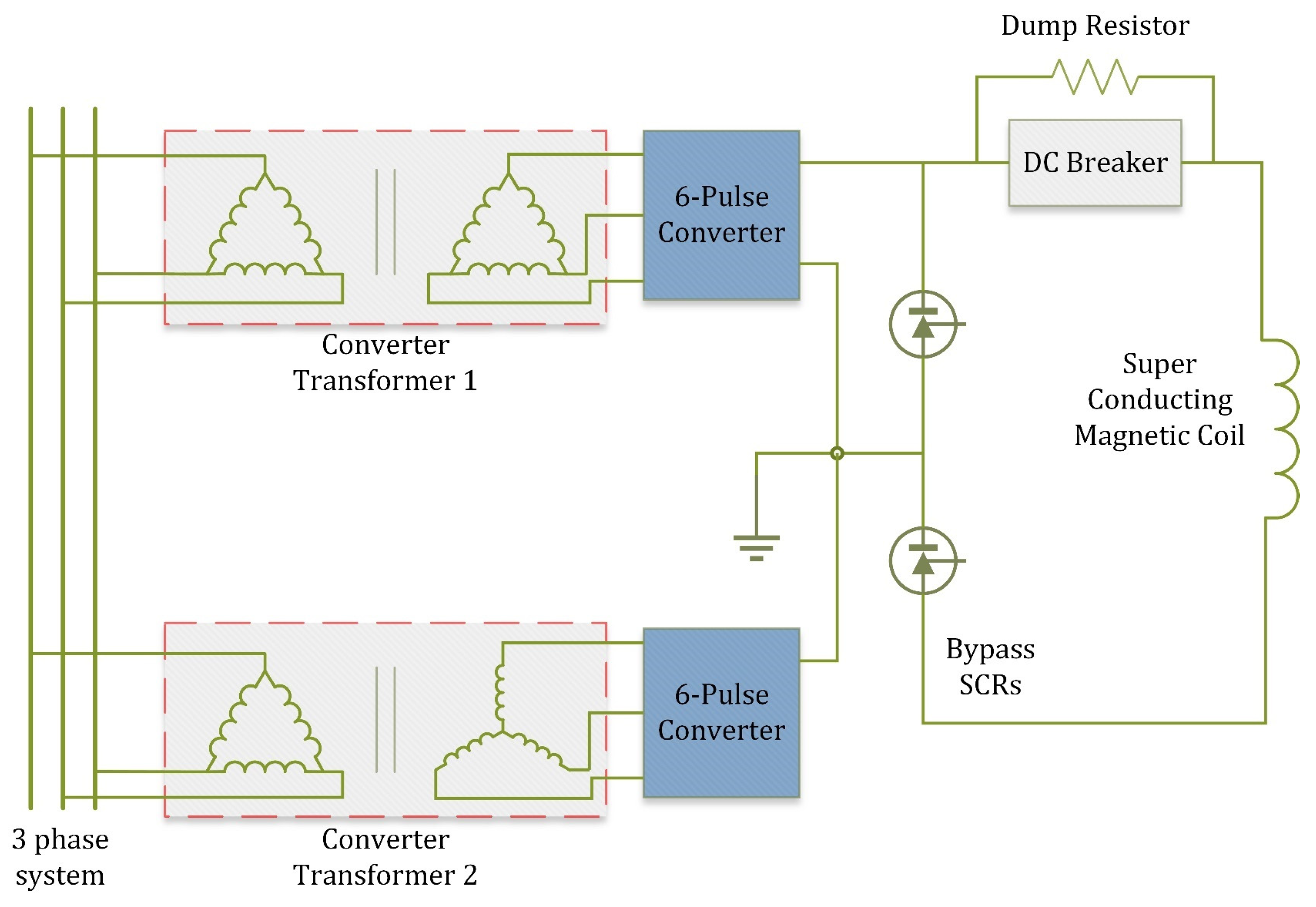
2.3. Mathematical Representation of SMES
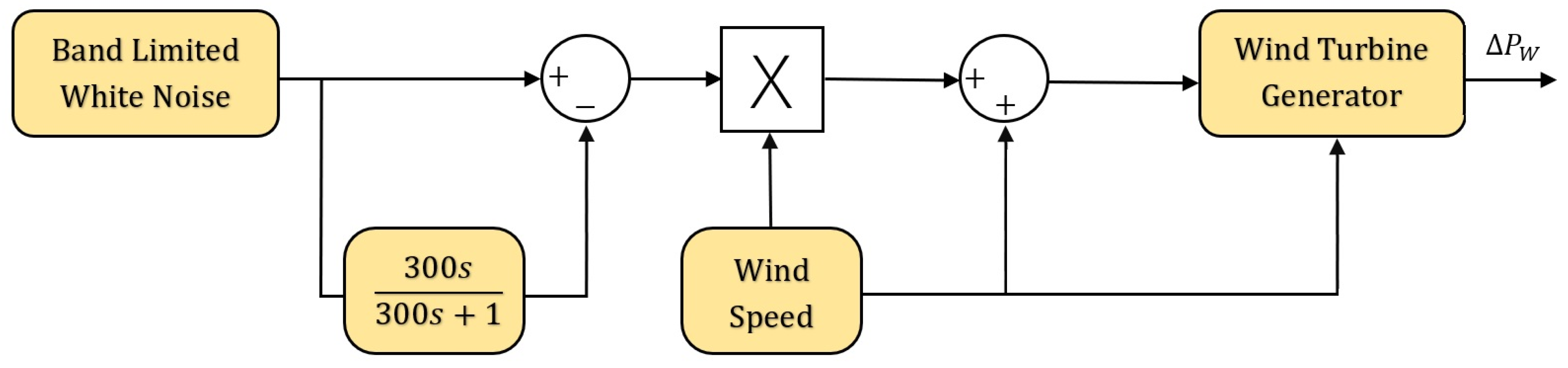
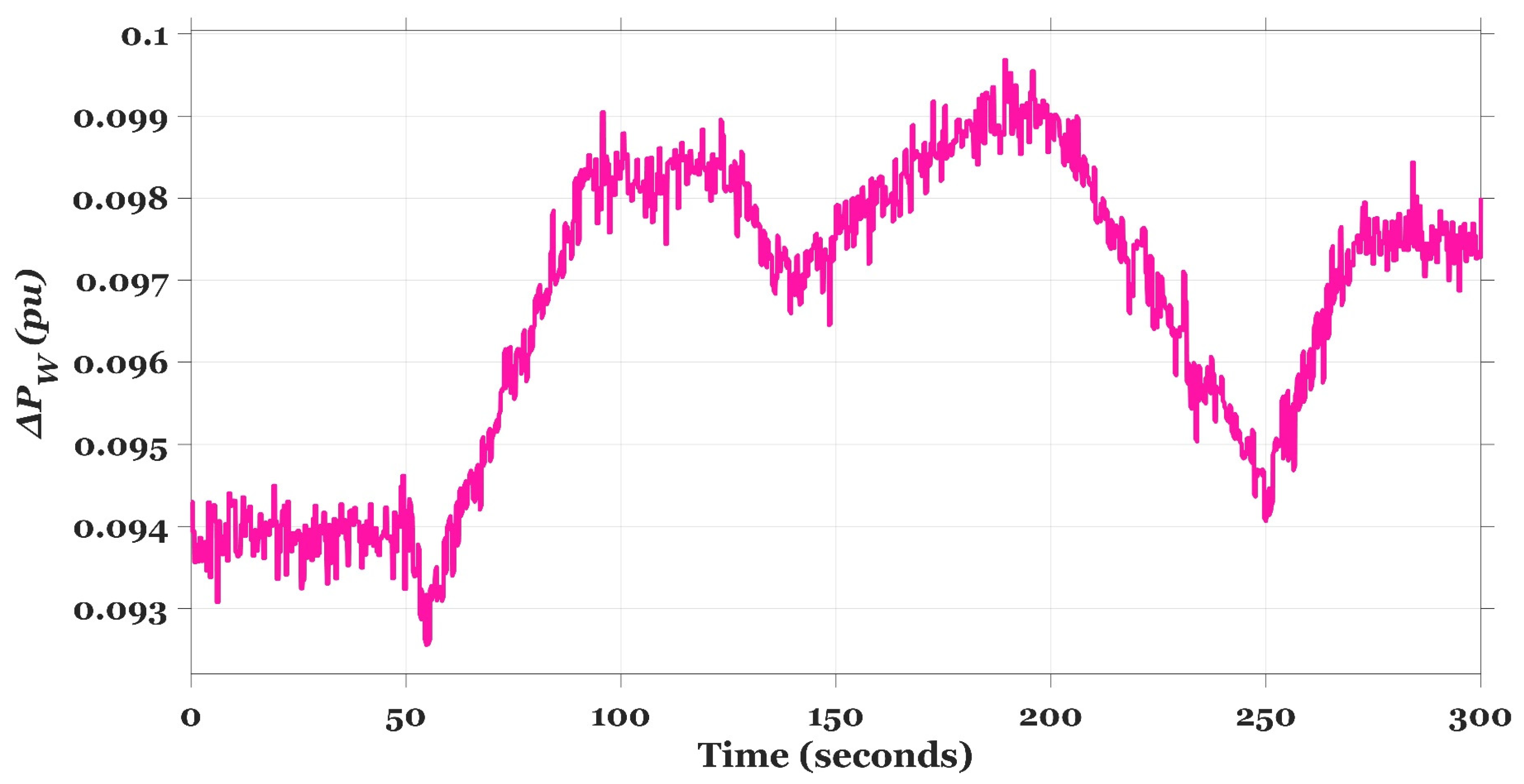
2.4. Mathematical Representation of the Wind Farm Unit
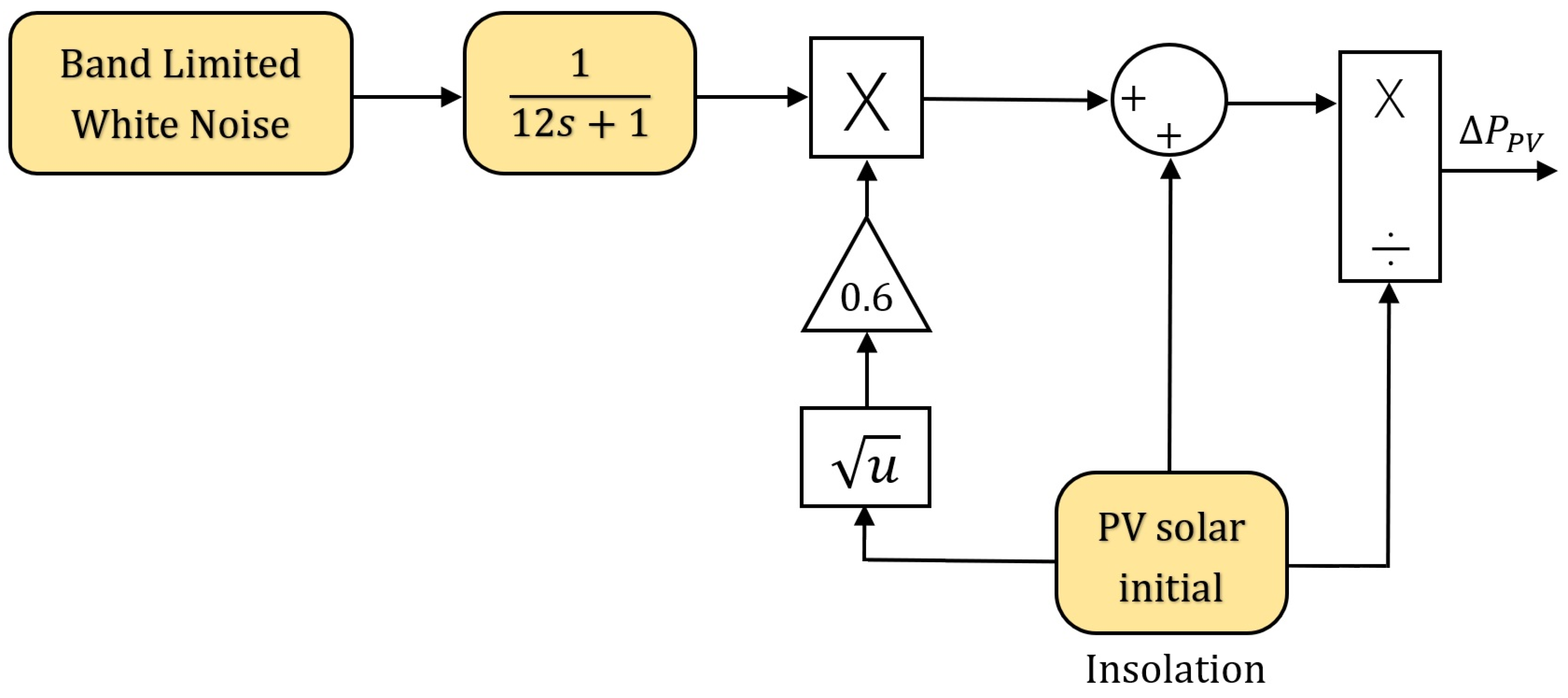
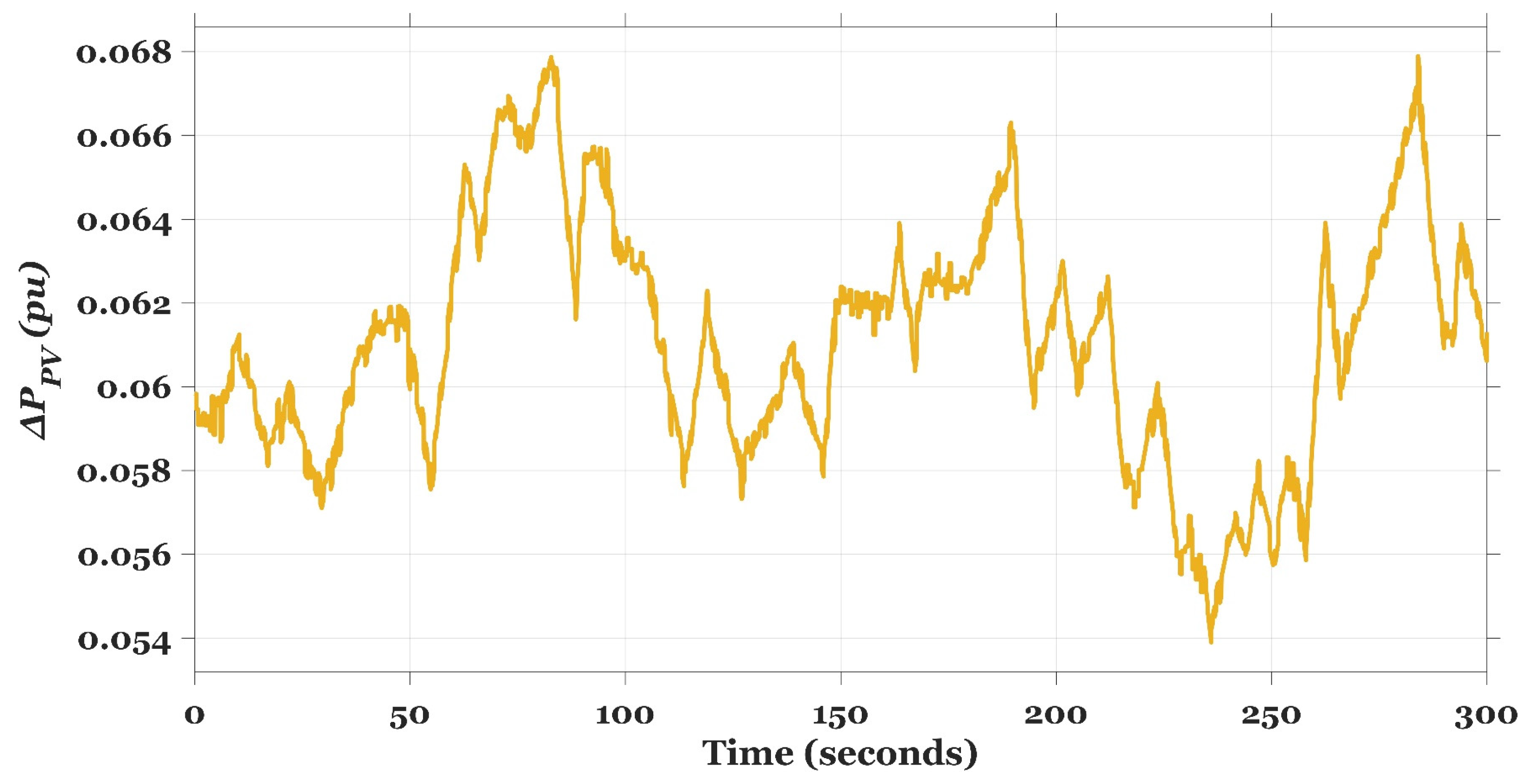
2.5. Mathematical Representation of the PV Unit
2.6. Mathematical Representation of the EVs Units
3. Control Strategy and Problem Presentation
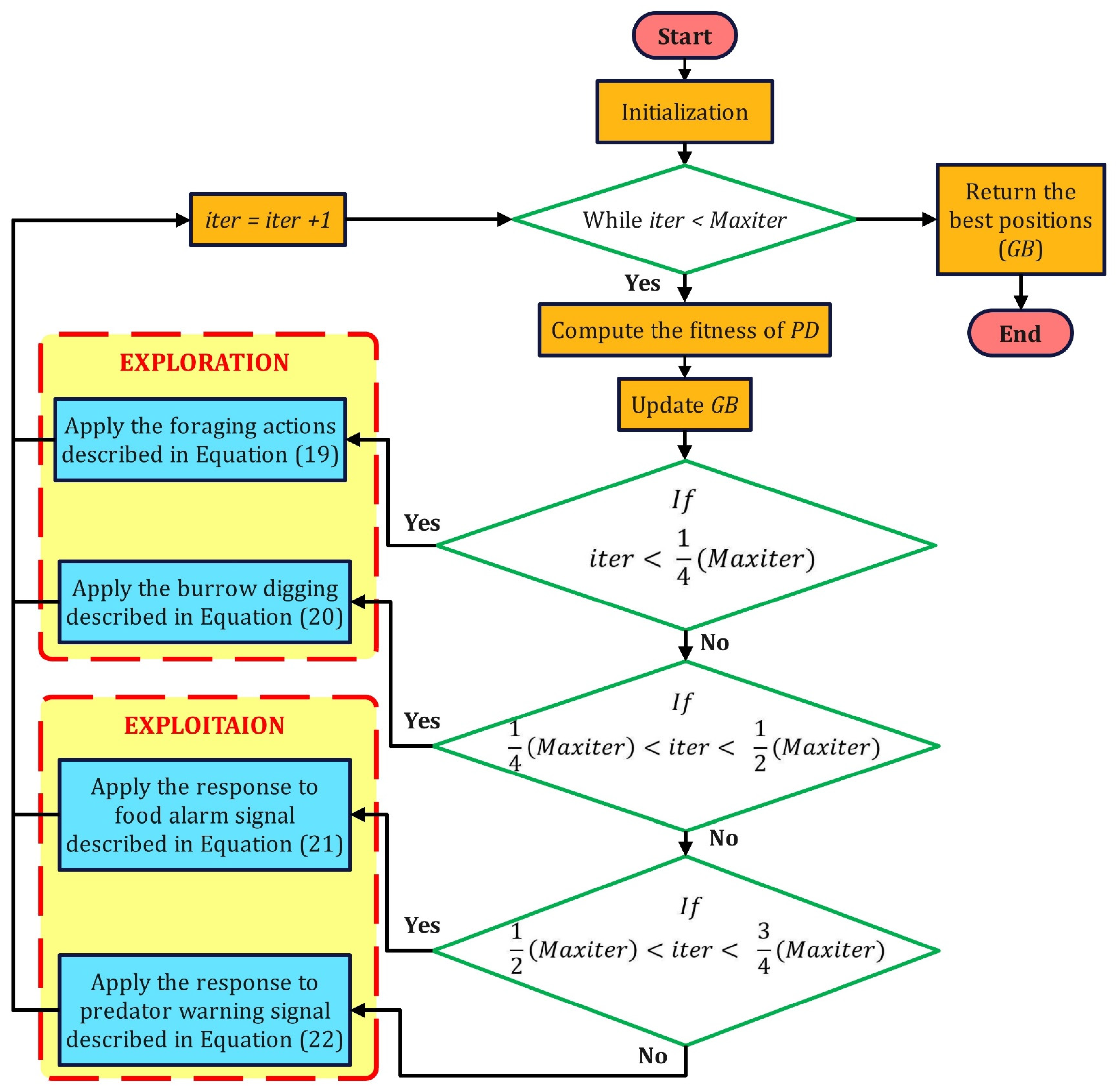
3.1. Prairie Dog Optimizer (PDO)
3.1.1. Initialization
3.1.2. The Estimation of Objective Function
3.1.3. Exploration Phase
3.1.4. Exploitation Phase
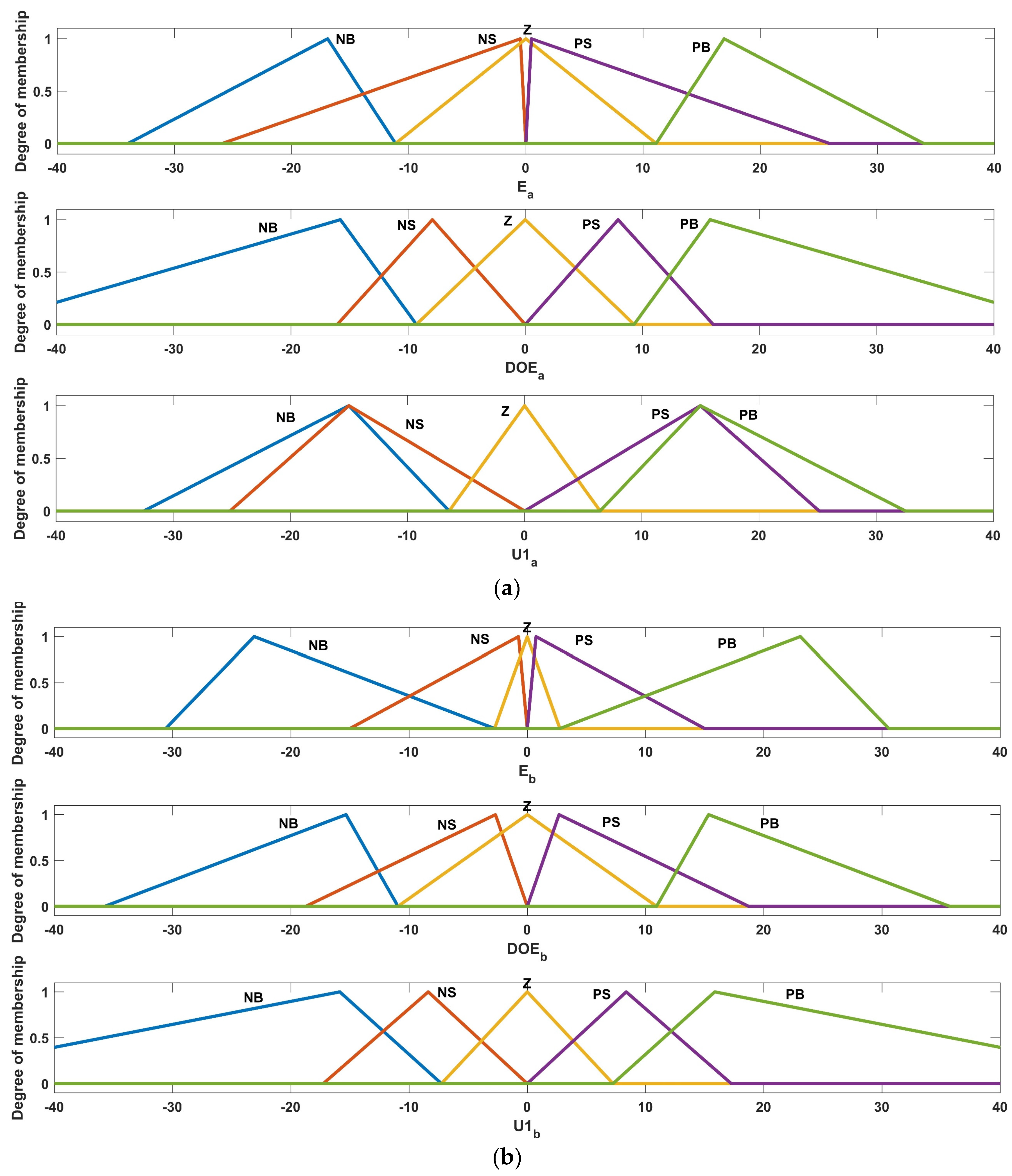
3.2. The Detailed Configuration of The Proposed FTFOIDFF Regulator
4. Results and Discussion
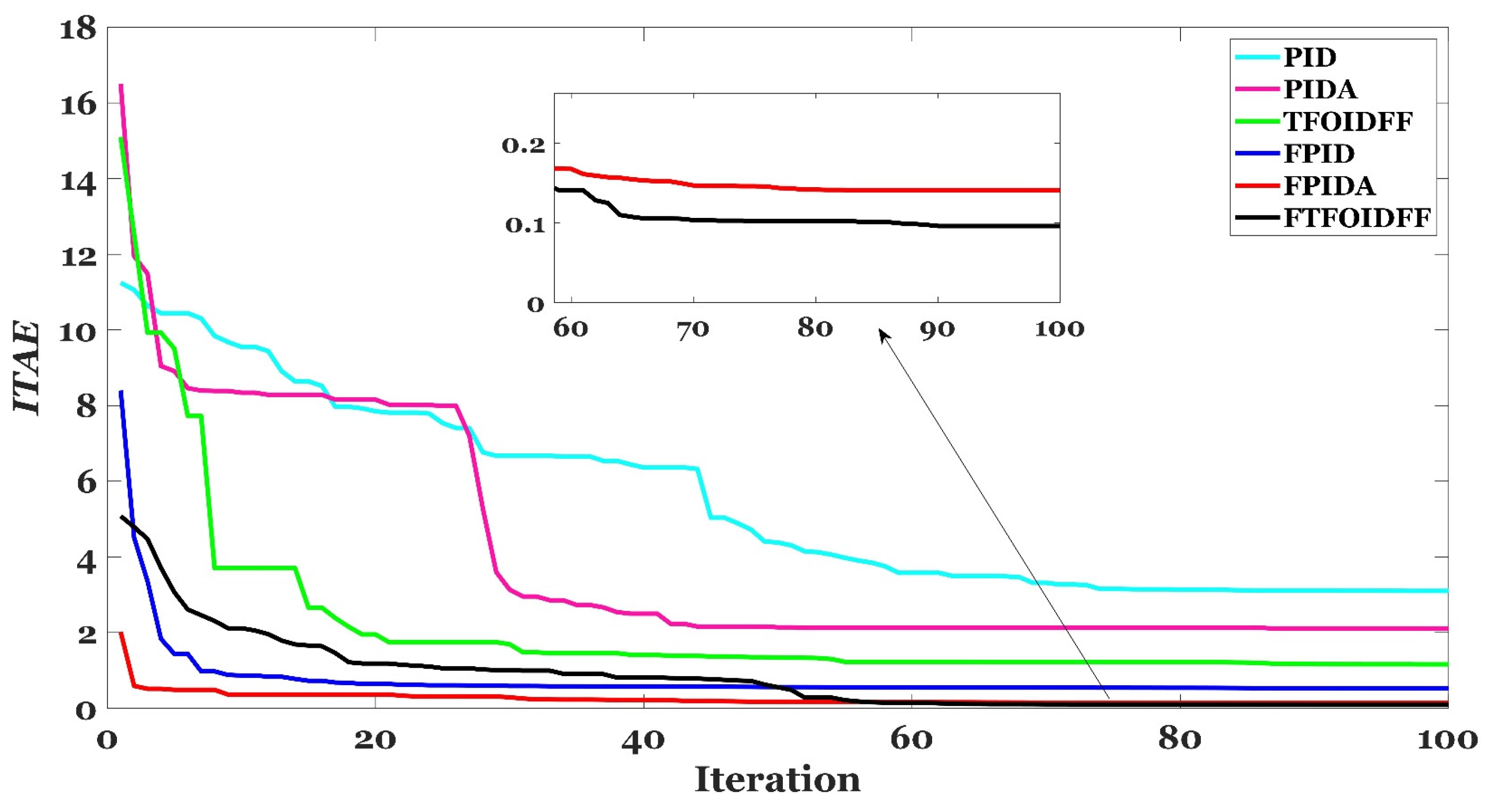
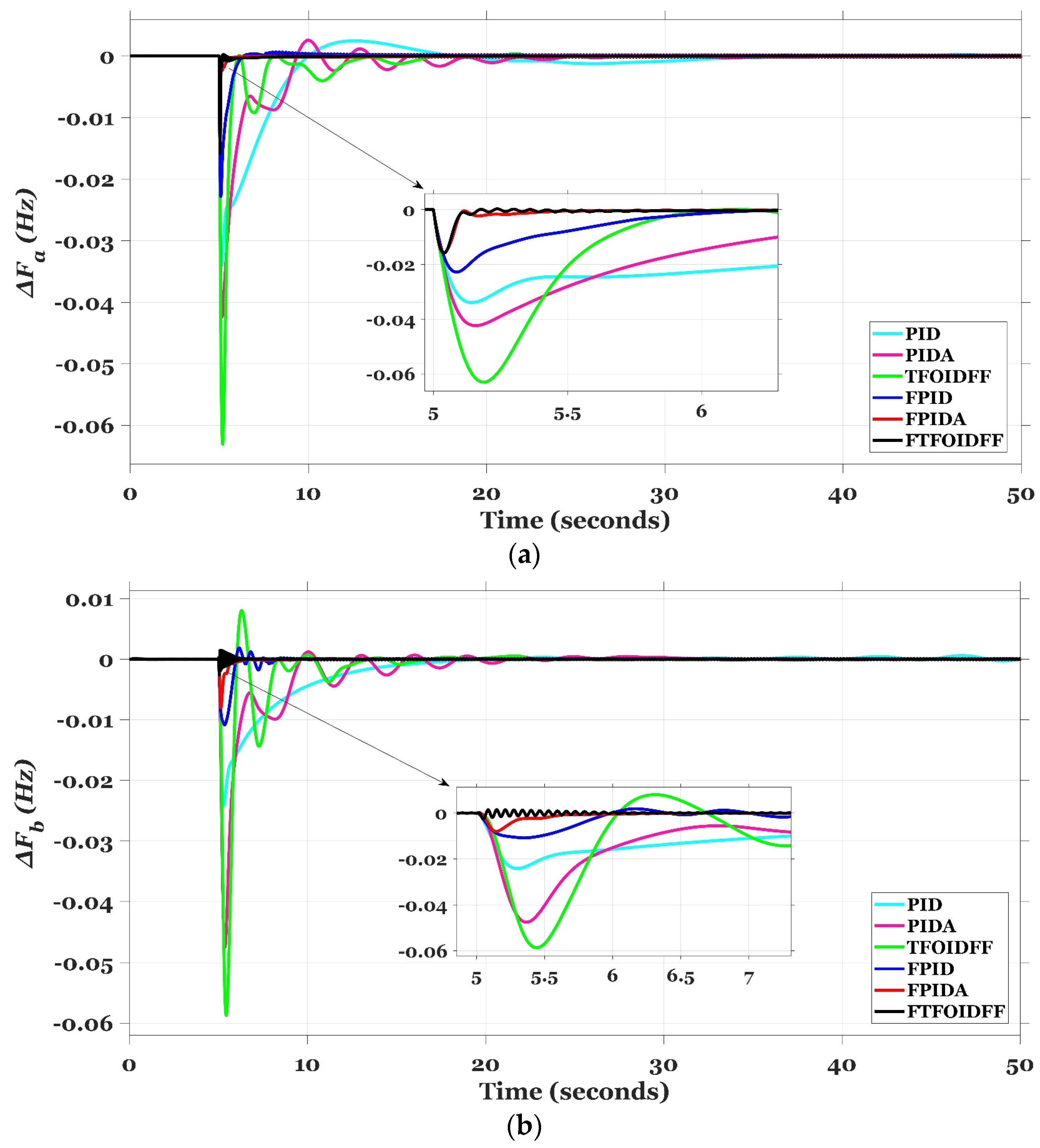
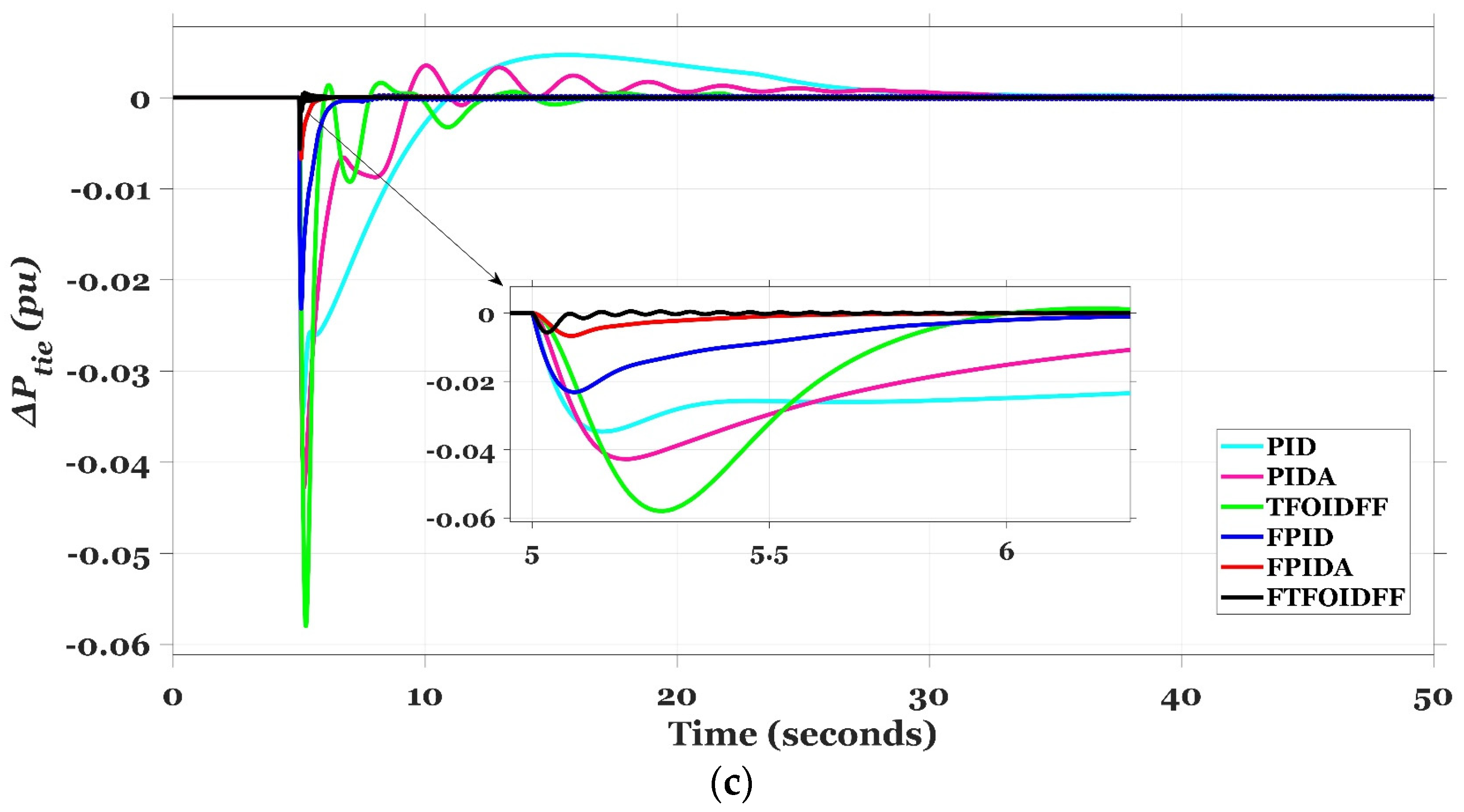
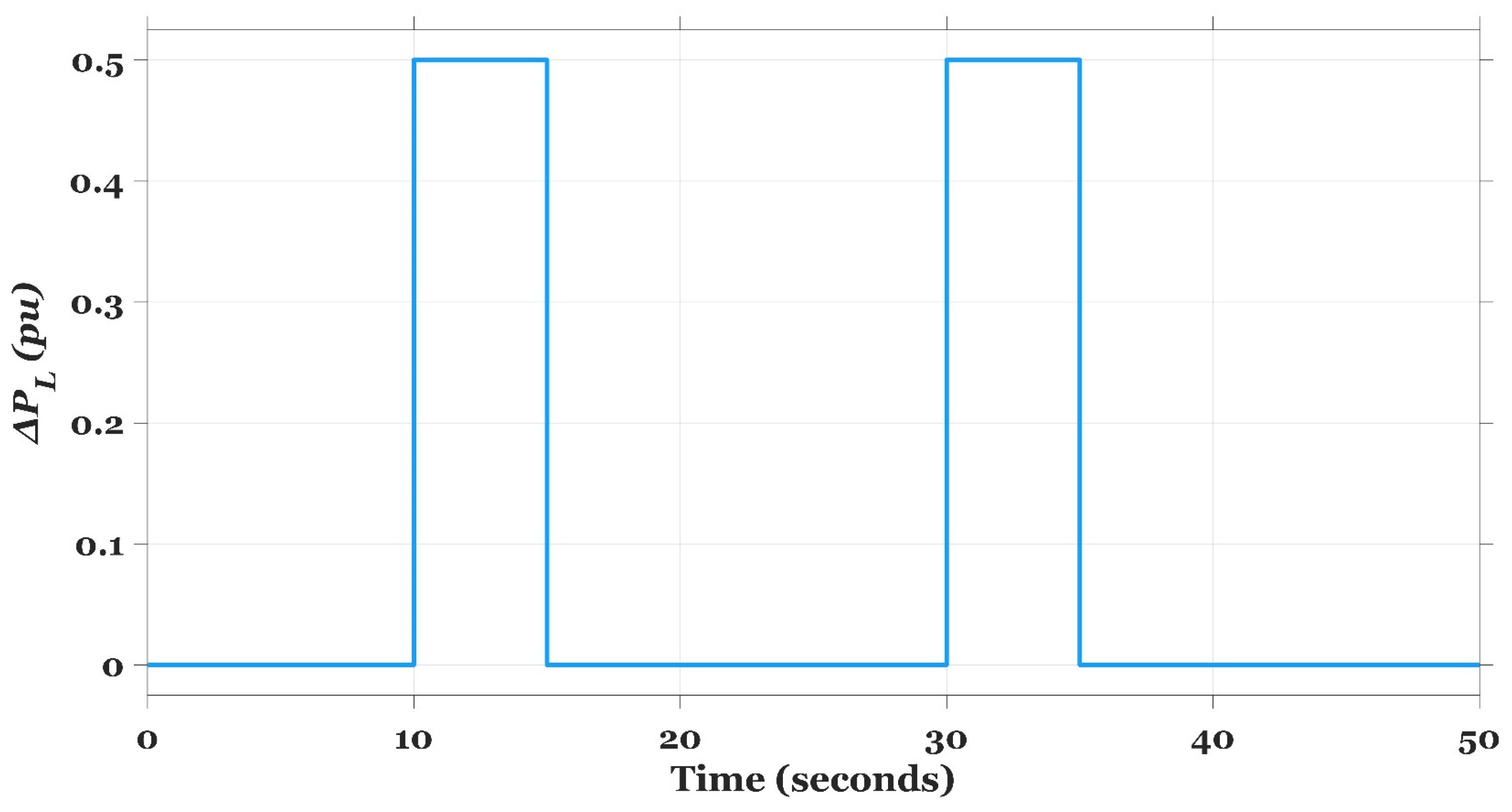
4.1. Case I: 10% Step Load Perturbation (SLP) at t = 5 s in Area (a)
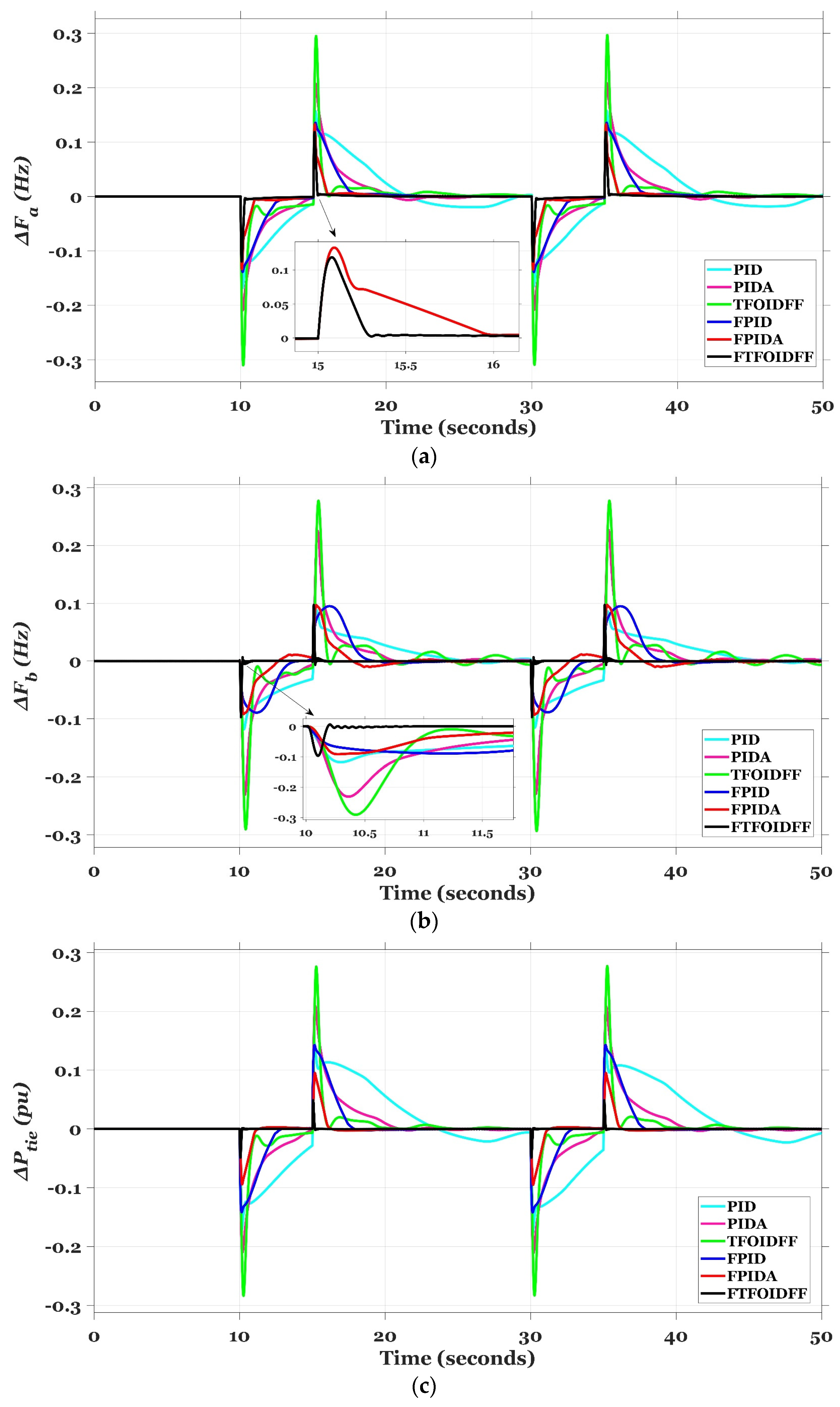
4.2. Case II: Multi-Step Load Perturbation (MSLP) in Area (a)
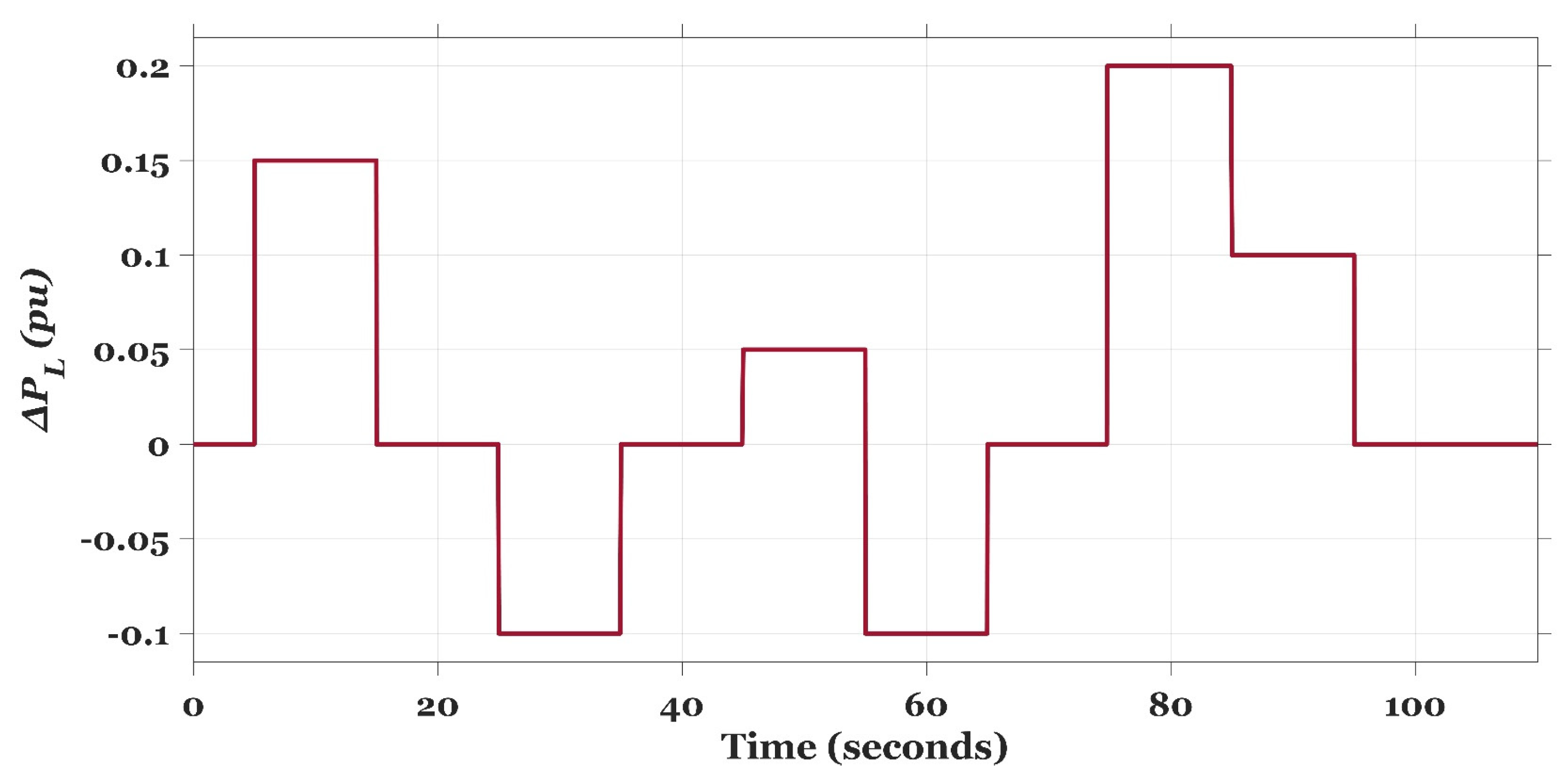
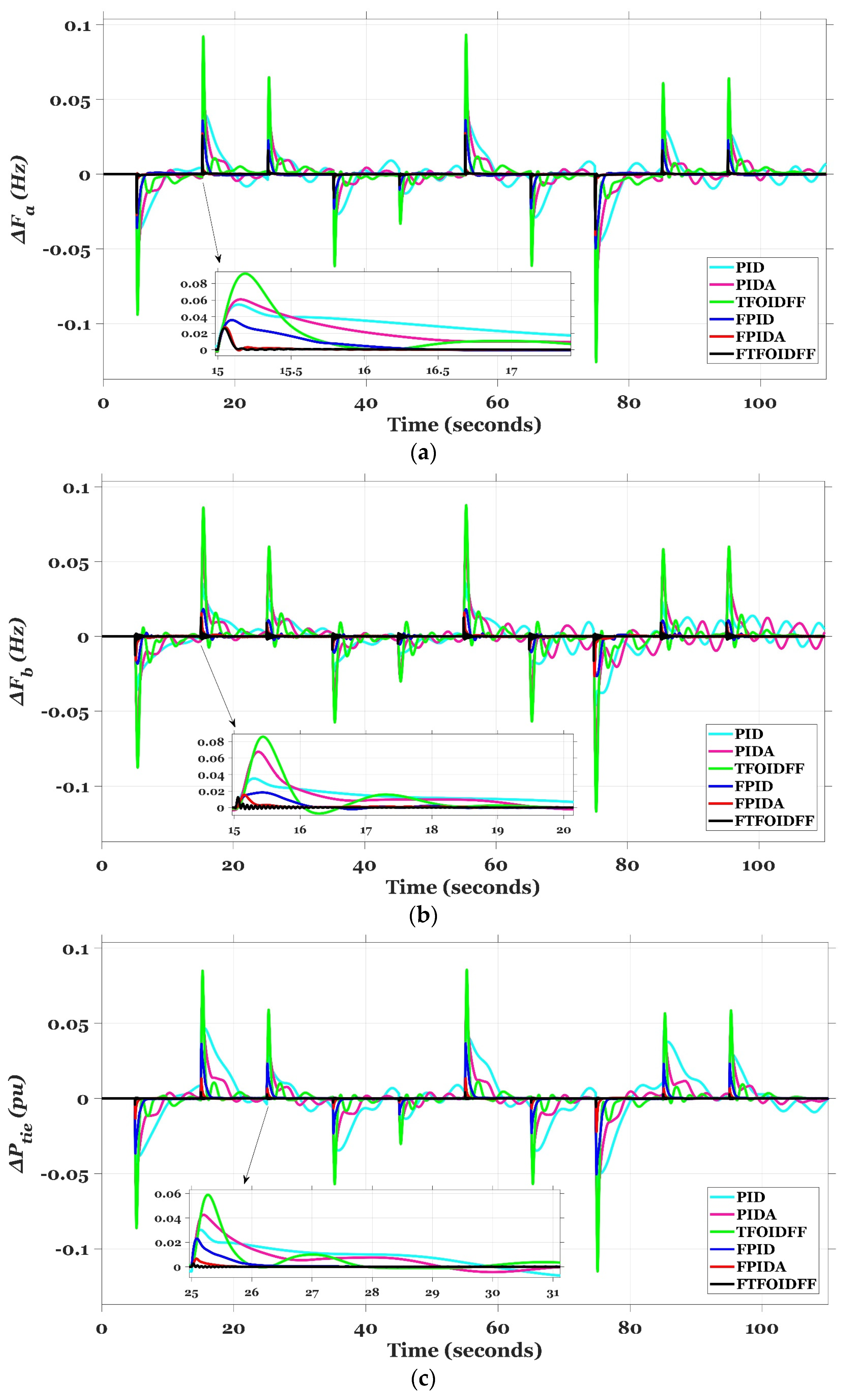
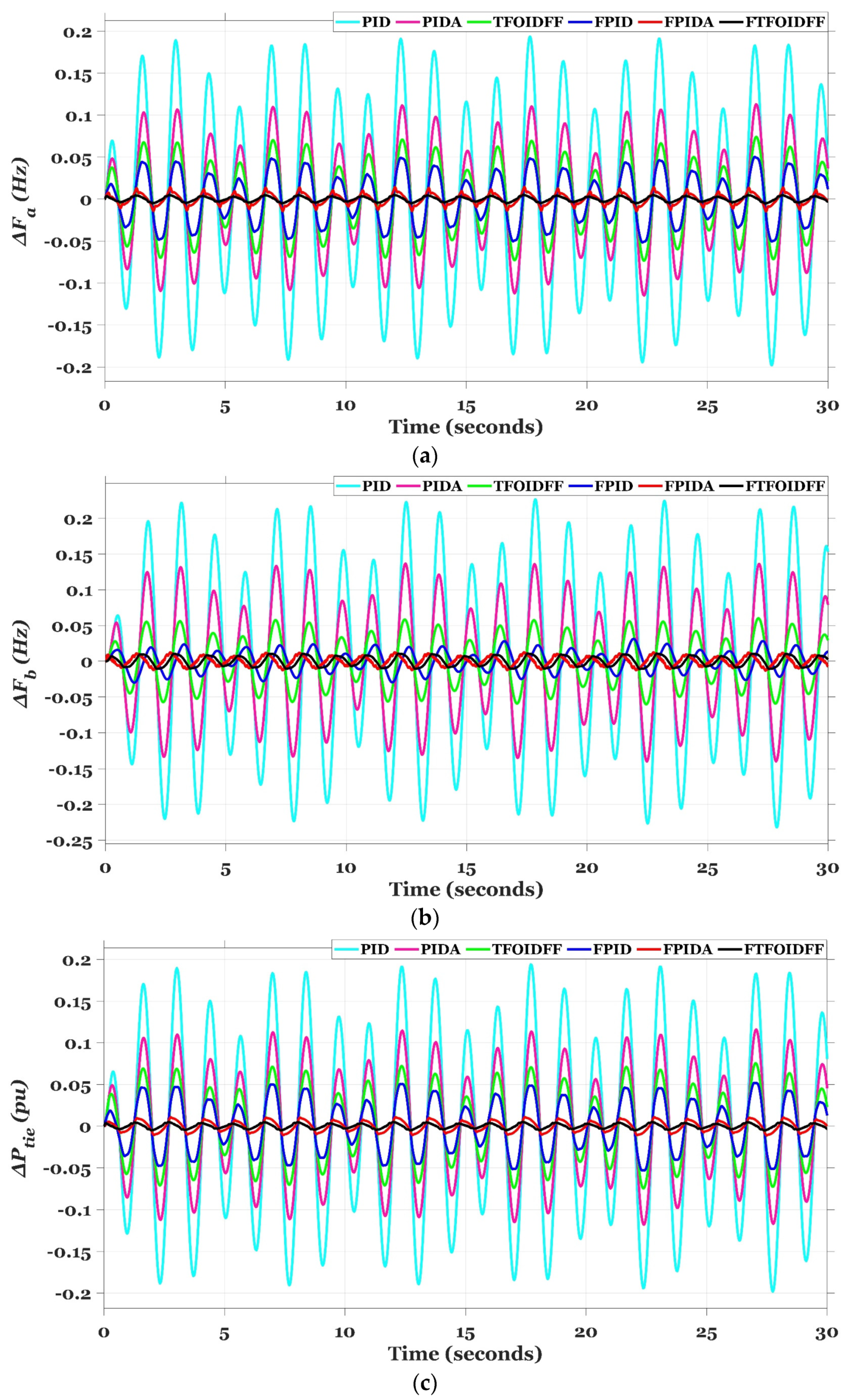
4.3. Case III: Random Load Perturbation (RLP) in Area (b)
4.4. Case IV: Pulse Load Perturbation (PLP) in Area (a)
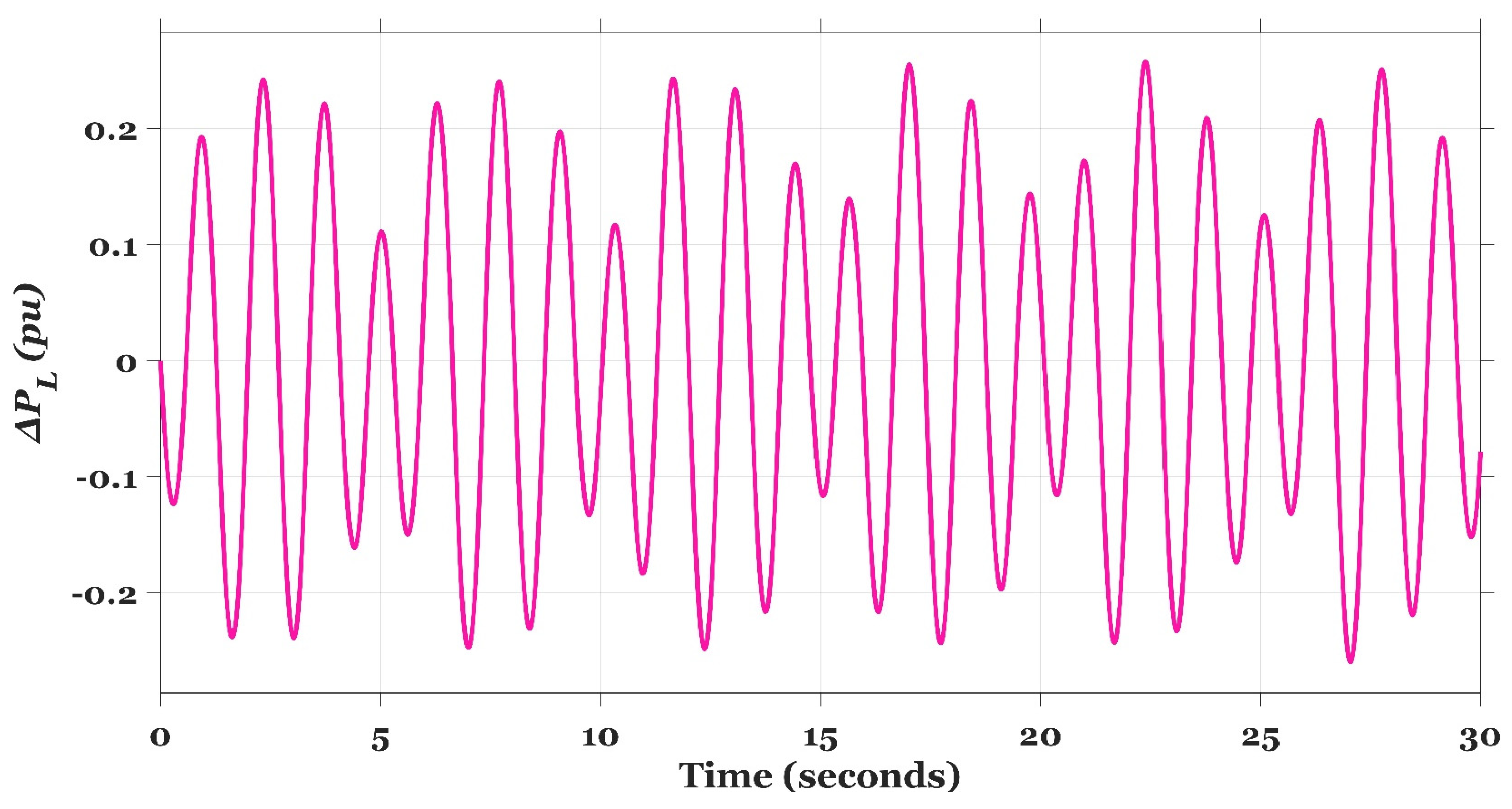
4.5. Case V: Random Sinusoidal Load Perturbation (RSLP) in Area (a)
4.6. Case VI: MSLP in Area (a) with 0.01 s Communication Time Delay (CTD)
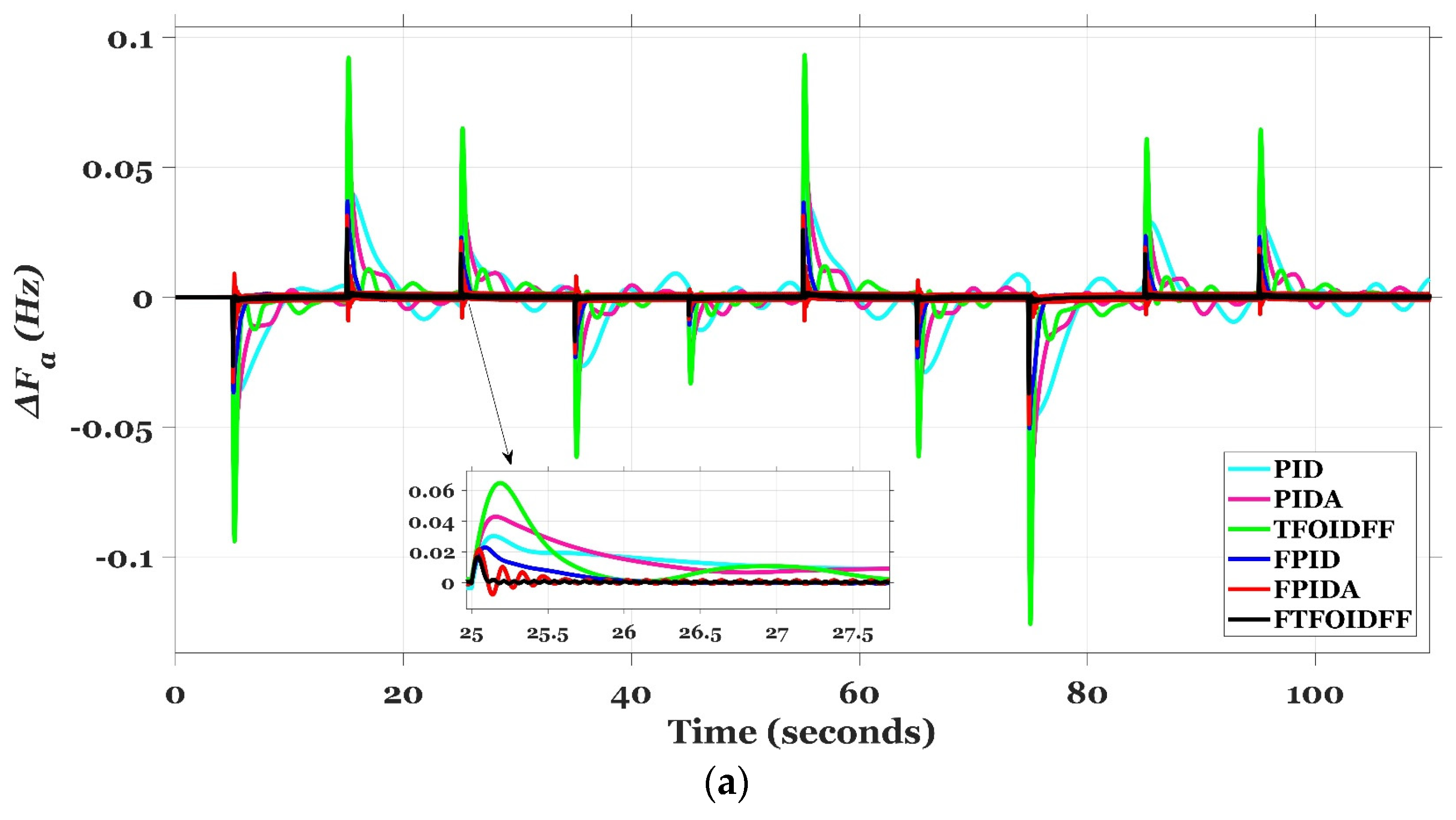
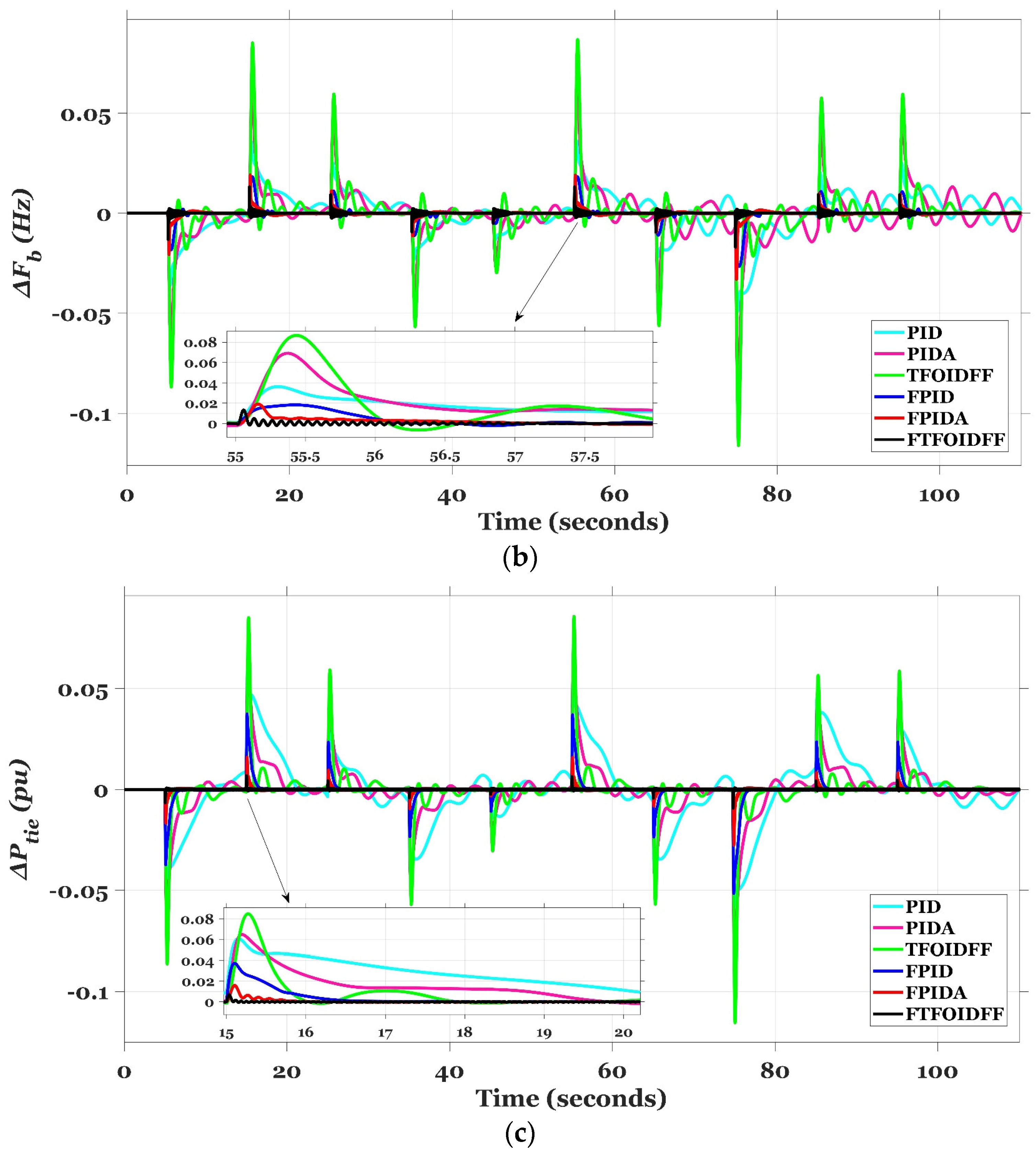
4.7. Case VII: Applying RESs Fluctuations in Both Areas
4.8. Case VIII: Applying RESs Fluctuations with MSLP in Area (b) and RSLP in Area (a)
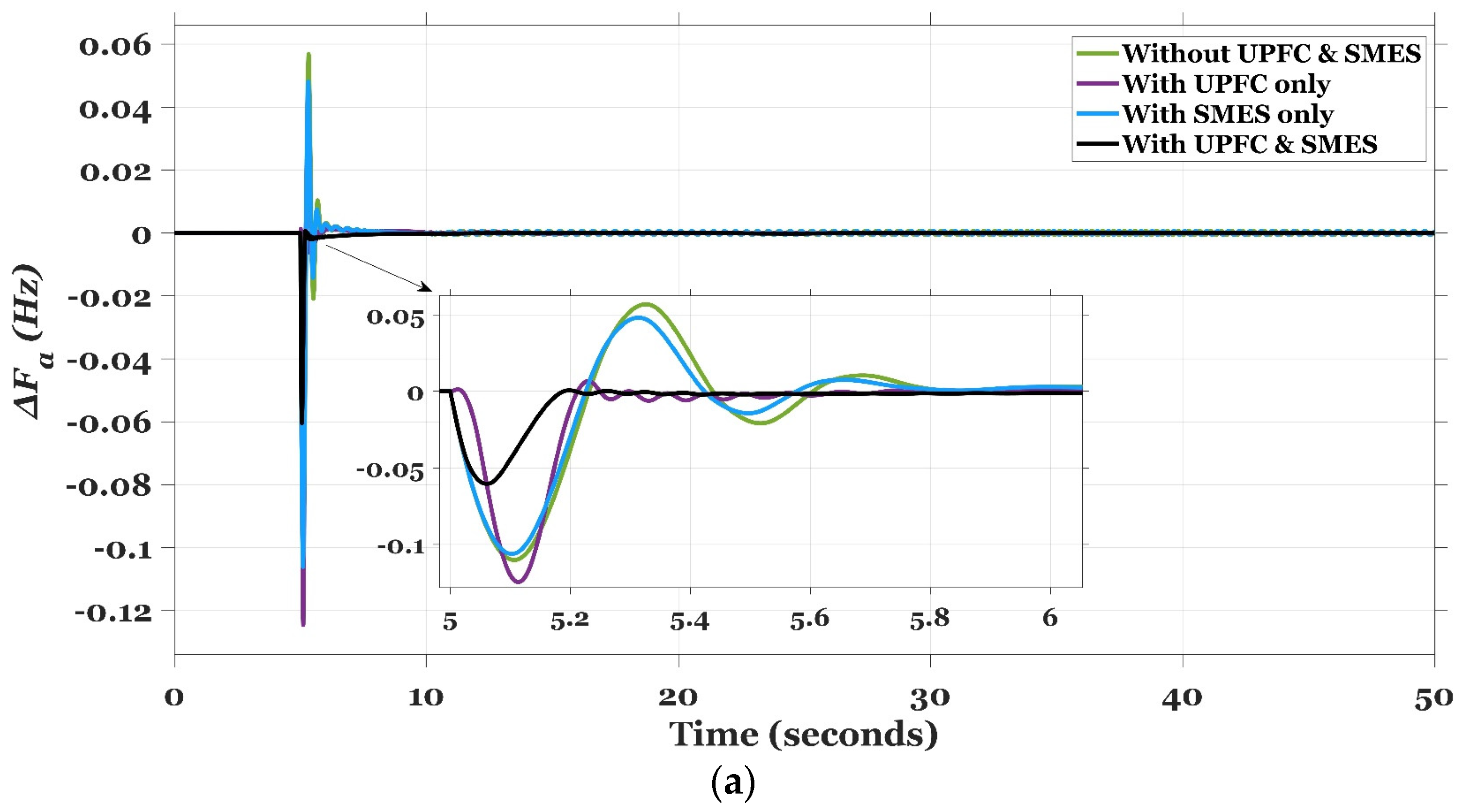
4.9. Case IX: UPFC and SMES Effect on the Studied System with 30% SLP in Area (a)
4.10. Case X: EVs Effect on the Studied System with MSLP in Area (a)
4.11. Case XI: Sensitivity Analysis
5. Conclusions
- The proposed control structure has efficiently improved frequency stability in a multi-area power system with severe RES penetrations.
- Integrating the benefits of tilt, fuzzy logic, FOPID, and fractional filter regulators in a single controller known as FTFOIDFF, which has superior performance over the other recent control structures.
- Application of a nature-inspired metaheuristic optimization technique that was recently developed (i.e., the Prairie Dog Optimizer, or PDO) for the purpose of fine-tuning not only the recommended controller settings but also the MFs of the FLC’s inputs and outputs in an effective manner.
- Validation of the positive effect of the integration of SMES, UPFC, and EVs in enhancing frequency performance during several harsh disturbances.
- The inclusion of conventional controllers for comparison with intelligent fuzzy-based controllers is unfair, as the incorporation of intelligent controllers, such as fuzzy logic or artificial neural networks, enhances the frequency response performance excessively.
- The use of simple structured models for EV, SMES, and UPFC will not reveal the full impact of these devices or the uncertainties that may be introduced into the systems as a result of their incorporation.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| FLC | Fuzzy Logic Control |
| FTFOIDFF | Fuzzy Tilted Fractional Order Integral Derivative with Fractional Filter |
| PDO | Prairie Dog Optimizer |
| SOA | Seagull Optimisation Algorithm |
| RUN | Runge Kutta optimizer |
| CGO | Chaos Game Optimizer |
| LFC | Load Frequency Control |
| CTD | Communication Time Delay |
| GRC | Generation Rate Constraint |
| UPFC | Unified Power Flow Controller |
| SMES | Superconducting Magnetic Energy Storage |
| EV | Electric Vehicle |
| PID | Proportional Integral Derivative |
| PIDA | Proportional Integral Derivative Acceleration |
| SLP | Step Load Perturbation |
| MSLP | Multi-Step Load Perturbation |
| RLP | Random Load Perturbation |
| RSLP | Random Sinusoidal Load Perturbation |
| PLP | Pulse Load Perturbation |
| AGC | Automatic Generation Control |
| RES | Renewable Energy Sources |
| PV | Photovoltaic |
| FACTS | Flexible Alternating Current Transmission Systems |
| FO | Fractional Order |
| PCS | Power Conversion System |
| PD | Prairie Dog |
| CT | Coterie |
| LB | Lower Boundary |
| UB | Upper Boundary |
| GB | Global Best |
| iter | Current Iteration |
| Maxiter | Maximum Iteration Number |
| MFs | Membership Functions |
| E | Error |
| DOE | Derivative of Error |
| NB | Negative Big |
| NS | Negative Small |
| Z | Zero |
| PS | Positive Small |
| PB | Positive Big |
| FIS | Fuzzy Interface System |
| First Control Law | |
| Total Control Law | |
| TFOIDFF Controller’s Transfer Function | |
| Simulation Time | |
| Tilt Gain | |
| Integral Gain | |
| Derivative Gain | |
| Tilt Fractional Order Power | |
| Fractional Order Integral Operator | |
| Fractional Order Derivative Operator | |
| Fractional Order Filter Operator | |
| Fractional Filter Coefficient | |
| Scaling Factor of the FLC inputs | |
| ACE | Area Control Error |
| ITAE | Integral Time Absolute Error |
| MOS | Maximum Overshoot |
| MUS | Maximum Undershoot |
| ST | Settling Time |
| ΔFa | The frequency deviation of Area (a) |
| ΔFb | The frequency deviation of Area (b) |
| ΔPtie | The tie-line power deviation |
Appendix A. The Nominal Values of the Power System’s Parameters
| Parameter | Nominal Value | Parameter Definition |
| 0.08 s | Governor time constant | |
| 0.3 s | Gain of reheater steam turbine | |
| 10.2 s | The time constant of reheater steam turbine | |
| 0.3 s | Steam turbine time constant | |
| 0.2 s | Hydroelectric turbine speed governor time constant | |
| 4.9 s | Hydro turbine speed governor reset time | |
| 28.749 s | Time constant of the transient droop | |
| 1.1 s | Average water string time in penstock | |
| 0.049 s | Gas turbine constant of valve positioner | |
| 1 | Valves’ gas turbine positioner constant | |
| 0.6 s | Gas turbine governor’s lead time constant | |
| 1.1 s | Gas turbine governor’s lag time constant | |
| 0.01 s | Combustion response time delay in a gas turbine | |
| 0.239 s | Gas turbine fuel time constant | |
| 0.2 s | Volume-time constant for gas turbine compressor discharge | |
| , | 68.965, 68.965 | Power system gains |
| , | 11.49, 11.49 s | Power system time constants |
| 0.0433 MW | Coefficient of synchronizing | |
| , | 1, 1 | Gains of SMES |
| , | 0.07 s | Time constants of SMES units |
| 0.003 s | Time constant of UPFC unit | |
| , | 1 | Gains of EVs |
| , | 0.28 s | Time constants of EVs |
| 0.431, 0.431 MW/Hz | Frequency bias coefficients | |
| 2.4 Hz/MW | Governor speed regulation constant for thermal, hydro, and gas units | |
| 0.5435, 0.3261, 0.1304 | Contribution factors of thermal, hydro, and gas units | |
| GRC with Hydro | -------- | (0.045 pu.MW/s) and (0.06 pu.MW/s. For both rising and decreasing rates), respectively |
| GRC with Thermal | -------- | The GRC (generation rate constraint) for the thermal unit is set (0.0017 pu.MW/s) For rising and decreasing rates |
References
- Elgerd, O.I. Electric Energy Systems Theory—An Introduction; Tata McGraw Hill: New Delhi, India, 2000. [Google Scholar]
- Kundur, P.S.; Malik, O. Power System Stability and Control, 2nd ed.; McGraw-Hill Education: Columbus, OH, USA, 2022. [Google Scholar]
- Bevrani, H. Robust Power System Frequency Control; Springer International Publishing: Cham, Switzerland, 2016. [Google Scholar]
- Parmar, K.P.S.; Majhi, S.; Kothari, D.P. Load frequency control of a realistic power system with multi-source power generation. Int. J. Electr. Power Energ. Syst. 2012, 42, 426–433. [Google Scholar] [CrossRef]
- Mohanty, B.; Panda, S.; Hota, P.K. Controller parameters tuning of differential evolution algorithm and its application to load frequency control of multi-source power system. Int. J. Electr. Power Energ. Syst. 2014, 54, 77–85. [Google Scholar] [CrossRef]
- Yildirim, B.; Gheisarnejad, M.; Khooban, M.H. A robust non-integer controller design for load frequency control in modern marine power grids. IEEE Trans. Emerg. Top. Comput. Intell. 2022, 6, 852–866. [Google Scholar] [CrossRef]
- Ahmed, E.M.; Mohamed, E.A.; Elmelegi, A.; Aly, M.; Elbaksawi, O. Optimum Modified Fractional Order Controller for Future Electric Vehicles and Renewable Energy-Based Interconnected Power Systems. IEEE Access 2021, 9, 29993–30010. [Google Scholar] [CrossRef]
- Sun, P.; Yun, T.; Chen, Z. Multi-objective robust optimization of multi-energy microgrid with waste treatment. Renew. Energy 2021, 178, 1198–1210. [Google Scholar] [CrossRef]
- Xiao, D.; Chen, H.; Wei, C.; Bai, X. Statistical Measure for Risk-seeking Stochastic Wind Power Offering Strategies in Electricity Markets. J. Mod. Power Syst. Clean Energy 2022, 10, 1437–1442. [Google Scholar] [CrossRef]
- Said, S.M.; Aly, M.; Hartmann, B.; Mohamed, E.A. Coordinated fuzzy logic-based virtual inertia controller and frequency relay scheme for reliable operation of low-inertia power system. IET Renew. Power Gener. 2021, 15, 1286–1300. [Google Scholar] [CrossRef]
- Liu, L.; Hu, Z.; Mujeeb, A. Automatic generation control considering uncertainties of the key parameters in the frequency response model. IEEE Trans. Power Syst. 2022, 37, 4605–4617. [Google Scholar] [CrossRef]
- Magdy, G.; Ali, H.; Xu, D. Effective Control of Smart Hybrid Power Systems: Cooperation of Robust LFC and Virtual Inertia Control Systems. CSEE J. Power Energy Syst. 2022, 8, 1583–1593. [Google Scholar] [CrossRef]
- Tripathy, S.C.; Kalantar, M.; Balasubramanian, R. Dynamics and stability of wind and diesel turbine generators with superconducting magnetic energy storage unit on an isolated power system. IEEE Trans. Energy Conv. 1991, 6, 579–585. [Google Scholar] [CrossRef]
- Abraham, R.J.; Das, D.; Patra, A. Automatic generation control of an interconnected hydrothermal power system considering superconducting magnetic energy storage. Int. J. Electr. Power Energ. Syst. 2007, 29, 571–579. [Google Scholar] [CrossRef]
- Banerjee, S.; Chatterjee, J.K.; Tripathy, S.C. Application of magnetic energy storage unit as load-frequency stabilizer. IEEE Trans. Energy Conv. 1990, 5, 46–51. [Google Scholar] [CrossRef]
- Abraham, R.J.; Das, D.; Patra, A. AGC of a hydrothermal system with SMES unit. In Proceedings of the 2006 IEEE GCC Conference (GCC), Manama, Bahrain, 20–22 March 2006; pp. 1–7. [Google Scholar]
- Padhan, S.; Sahu, R.K.; Panda, S. Automatic generation control with thyristor controlled series compensator including superconducting magnetic energy storage units. Ain Shams Eng. J. 2014, 5, 759–774. [Google Scholar] [CrossRef]
- Sudha, K.R.; Vijaya, S.R. Load frequency control of an interconnected reheat thermal system using type-2 fuzzy system including SMES units. Int. J. Electr. Power Energ. Syst. 2012, 43, 1383–1392. [Google Scholar] [CrossRef]
- Hingorani, N.G.; Gyugyi, L. Understanding FACTS: Concepts and Technology of Flexible AC Transmission System; IEEE Press: New York, NY, USA, 2000. [Google Scholar]
- Praghnesh, B.; Ranjit, R. Load frequency stabilization by coordinated control of Thyristor Controlled Phase Shifters and superconducting magnetic energy storage for three types of interconnected two-area power systems. Int. J. Electr. Power Energ. Syst. 2010, 32, 1111–1124. [Google Scholar]
- Praghnesh, B.; Ranjit, R. Comparative performance evaluation of SMES-SMES, TCPS-SMES and SSSC-SMES controllers in automatic generation control for a two-area hydro-hydro system. Int. J. Electr. Power Energ. Syst. 2011, 32, 1585–1597. [Google Scholar]
- Paliwal, N.; Srivastava, L.; Pandit, M. Application of grey wolf optimization algorithm for load frequency control in multi-source single area power system. Evol. Intell. 2022, 15, 563–584. [Google Scholar] [CrossRef]
- Aryan, P.; Ranjan, M.; Shankar, R. Deregulated LFC scheme using equilibrium optimized Type-2 fuzzy controller. WEENTECH Proc. Energy 2021, 8, 494–505. [Google Scholar] [CrossRef]
- Dreidy, M.; Mokhlis, H.; Mekhilef, S. Inertia response and frequency control techniques for renewable energy sources: A review. Renew. Sustain. Energy Rev. 2017, 69, 144–155. [Google Scholar] [CrossRef]
- Fernández-Guillamón, A.; Gómez-Lázaro, E.; Muljadi, E.; Molina-García, Á. Power systems with high renewable energy sources: A review of inertia and frequency control strategies over time. Renew. Sustain. Energy Rev. 2019, 115, 109369. [Google Scholar] [CrossRef]
- Singh, V.P.; Kishor, N.; Samuel, P. Improved load frequency control of power system using LMI based PID approach. J. Franklin Inst. 2017, 354, 6805–6830. [Google Scholar] [CrossRef]
- Jagatheesan, K.; Anand, B.; Samanta, S.; Dey, N.; Ashour, A.S.; Balas, V.E. Particle swarm optimisation-based parameters optimisation of PID controller for load frequency control of multi-area reheat thermal power systems. Int. J. Adv. Intell. Paradig. 2017, 9, 464. [Google Scholar] [CrossRef]
- Hasanien, H.M. Whale optimisation algorithm for automatic generation control of interconnected modern power systems including renewable energy sources. IET Gener. Transm. Distrib. 2018, 12, 607–614. [Google Scholar] [CrossRef]
- Mehta, P.; Bhatt, P.; Pandya, V. Optimized coordinated control of frequency and voltage for distributed generating system using Cuckoo Search Algorithm. Ain Shams Eng. J. 2018, 9, 1855–1864. [Google Scholar] [CrossRef]
- Sobhy, M.A.; Abdelaziz, A.Y.; Hasanien, H.M.; Ezzat, M. Marine predators algorithm for load frequency control of modern interconnected power systems including renewable energy sources and energy storage units. Ain Shams Eng. J. 2021, 12, 3843–3857. [Google Scholar] [CrossRef]
- Nayak, S.R.; Khadanga, R.K.; Arya, Y.; Panda, S.; Sahu, P.R. Influence of ultra-capacitor on AGC of five-area hybrid power system with multi-type generations utilizing sine cosine adopted dingo optimization algorithm. Electr. Power Syst. Res. 2023, 223, 109513. [Google Scholar] [CrossRef]
- Veerendar, T.; Kumar, D.; Sreeram, V. Maiden application of colliding bodies optimizer for load frequency control of two-area nonreheated thermal and hydrothermal power systems. Asian J. Control. 2023, 25, 3443–3455. [Google Scholar] [CrossRef]
- Murugesan, D.; Jagatheesan, K.; Shah, P.; Sekhar, R. Fractional order PIλDμ controller for microgrid power system using cohort intelligence optimization. Results Control. Optim. 2023, 11, 100218. [Google Scholar] [CrossRef]
- Andic, C.; Ozumcan, S.; Varan, M.; Ozturk, A. A novel Sea Horse Optimizer based load frequency controller for two-area power system with PV and thermal units. Preprints 2023, 2023040368. [Google Scholar] [CrossRef]
- Gheisarnejad, M. An effective hybrid harmony search and cuckoo optimization algorithm based fuzzy PID controller for load frequency control. Appl. Soft Comput. 2018, 65, 121–138. [Google Scholar] [CrossRef]
- Ahmed, M.; Magdy, G.; Khamies, M.; Kamel, S. Modified TID controller for load frequency control of a two-area interconnected diverse-unit power system. Int. J. Electr. Power Energy Syst. 2022, 135, 107528. [Google Scholar] [CrossRef]
- Zahariev, A.; Kiskinov, H. Asymptotic Stability of the Solutions of Neutral Linear Fractional System with Nonlinear Perturbation. Mathematics 2020, 8, 390. [Google Scholar] [CrossRef]
- Milev, M.; Zlatev, S. A Note about Stability of Fractional Retarded Linear Systems with Distributed Delays. Int. J. Pure Appl. Math. 2017, 115, 873–881. [Google Scholar] [CrossRef][Green Version]
- Kiskinov, H.; Milev, M.; Zahariev, A. About the Resolvent Kernel of Neutral Linear Fractional System with Distributed Delays. Mathematics 2022, 10, 4573. [Google Scholar] [CrossRef]
- Kumar Sahu, R.; Panda, S.; Biswal, A.; Chandra Sekhar, G.T. Design and analysis of tilt integral derivative controller with filter for load frequency control of multi-area interconnected power systems. ISA Trans. 2016, 61, 251–264. [Google Scholar] [CrossRef] [PubMed]
- Topno, P.N.; Chanana, S. Load frequency control of a two-area multi-source power system using a tilt integral derivative controller. J. Vib. Control 2018, 24, 110–125. [Google Scholar] [CrossRef]
- Shouran, M.; Anayi, F.; Packianather, M.; Habil, M. Load frequency control based on the Bees Algorithm for the Great Britain power system. Designs 2021, 5, 50. [Google Scholar] [CrossRef]
- Bayati, N.; Dadkhah, A.; Vahidi, B.; Sadeghi, S.H.H. Fopid design for load-frequency control using genetic algorithm. Sci. Int. 2015, 27, 3089–3094. [Google Scholar]
- Pan, I.; Das, S. Fractional order AGC for distributed energy resources using robust optimization. IEEE Trans. Smart Grid 2016, 7, 2175–2186. [Google Scholar] [CrossRef]
- Ramachandran, R.; Satheesh Kumar, J.; Madasamy, B.; Veerasamy, V. A hybrid MFO-GHNN tuned self-adaptive FOPID controller for ALFC of renewable energy integrated hybrid power system. IET Renew. Power Gener. 2021, 15, 1582–1595. [Google Scholar] [CrossRef]
- Sambariya, D.K.; Nagar, O.; Sharma, A.K. Application of FOPID design for LFC using flower pollination algorithm for three-area power system. Univers. J. Contr. Autom. 2020, 8, 212695735. [Google Scholar] [CrossRef][Green Version]
- Alharbi, M.; Ragab, M.; AboRas, K.M.; Kotb, H.; Dashtdar, M.; Shouran, M.; Elgamli, E. Innovative AVR-LFC design for a multi-area power system using hybrid fractional-order PI and PIDD2 controllers based on dandelion optimizer. Mathematics 2023, 11, 1387. [Google Scholar] [CrossRef]
- Latif, A.; Hussain, S.M.S.; Das, D.C.; Ustun, T.S. Optimum synthesis of a BOA optimized novel dual-stage PI-(1+ID) controller for frequency response of a microgrid. Energies 2020, 13, 3446. [Google Scholar] [CrossRef]
- Mohamed, E.A.; Ahmed, E.M.; Elmelegi, A.; Aly, M.; Elbaksawi, O.; Mohamed, A.-A.A. An optimized hybrid fractional order controller for frequency regulation in multi-area power systems. IEEE Access 2020, 8, 213899–213915. [Google Scholar] [CrossRef]
- Yogendra, A. A novel CFFOPI-FOPID controller for AGC performance enhancement of single and multi-area electric power systems. ISA Trans. 2020, 100, 126–135. [Google Scholar] [CrossRef]
- Yogendra, A. A new optimized fuzzy FOPI-FOPD controller for automatic generation control of electric power systems. J. Franklin Inst. 2019, 356, 5611–5629. [Google Scholar] [CrossRef]
- Gheisarnejad, M.; Khooban, M.H. Design an optimal fuzzy fractional proportional integral derivative controller with derivative filter for load frequency control in power systems. Trans. Inst. Meas. Control 2019, 41, 2563–2581. [Google Scholar] [CrossRef]
- Yogendra, A. Improvement in automatic generation control of two-area electric power systems via a new fuzzy aided optimal PIDN-FOI controller. ISA Trans. 2018, 80, 475–490. [Google Scholar] [CrossRef]
- Arya, Y.; Kumar, N.; Dahiya, P.; Sharma, G.; Çelik, E.; Dhundhara, S.; Sharma, M. Cascade-I λ D μ N controller design for AGC of thermal and hydro-thermal power systems integrated with renewable energy sources. IET Renew. Power Gener. 2021, 15, 504–520. [Google Scholar] [CrossRef]
- Magdy, G.; Shabib, G.; Elbaset, A.A.; Mitani, Y. Frequency stabilization of renewable power systems based on MPC with application to the Egyptian grid. IFAC-PapersOnLine 2018, 51, 280–285. [Google Scholar] [CrossRef]
- Zhang, C.; Wang, S.; Zhao, Q. Distributed economic MPC for LFC of multi-area power system with wind power plants in power market environment. Int. J. Electr. Power Energy Syst. 2020, 126, 106548. [Google Scholar] [CrossRef]
- Fathy, A.; Kassem, A.M. Antlion optimizer-ANFIS load frequency control for multi-interconnected plants comprising photovoltaic and wind turbine. ISA Trans. 2018, 87, 282–296. [Google Scholar] [CrossRef]
- Patowary, M.; Panda, G.; Naidu, B.R.; Deka, B.C. ANN-based adaptive current controller for on-grid DG system to meet frequency deviation and transient load challenges with hardware implementation. IET Renew. Power Gener. 2017, 12, 61–71. [Google Scholar] [CrossRef]
- Dombi, J.; Hussain, A. A new approach to fuzzy control using the distending function. J. Process. Control. 2019, 86, 16–29. [Google Scholar] [CrossRef]
- Valdez, F.; Castillo, O.; Peraza, C. Fuzzy logic in dynamic parameter adaptation of Harmony search optimization for benchmark functions and fuzzy controllers. Int. J. Fuzzy Syst. 2020, 22, 1198–1211. [Google Scholar] [CrossRef]
- Yakout, A.H.; Kotb, H.; Hasanien, H.M.; Aboras, K.M. Optimal fuzzy PIDF load frequency controller for hybrid microgrid system using marine predator algorithm. IEEE Access 2021, 9, 54220–54232. [Google Scholar] [CrossRef]
- Rajesh, K.S.; Dash, S.S. Load frequency control of autonomous power system using adaptive fuzzy based PID controller optimized on improved sine cosine algorithm. J. Ambient. Intell. Humaniz. Comput. 2018, 10, 2361–2373. [Google Scholar] [CrossRef]
- Mishra, D.; Sahu, P.C.; Prusty, R.C.; Panda, S. Fuzzy adaptive Fractional Order-PID controller for frequency control of an Islanded Microgrid under stochastic wind/solar uncertainties. Int. J. Ambient. Energy 2021, 43, 4602–4611. [Google Scholar] [CrossRef]
- Osinski, C.; Leandro, G.V.; Oliveira, G.H.d.C. A new hybrid load frequency control strategy combining fuzzy sets and differential evolution. J. Control. Autom. Electr. Syst. 2021, 32, 1627–1638. [Google Scholar] [CrossRef]
- Mohamed, E.A.; Aly, M.; Watanabe, M. New tilt fractional-order integral derivative with fractional filter (TFOIDFF) controller with artificial hummingbird optimizer for LFC in renewable energy power grids. Mathematics 2022, 10, 3006. [Google Scholar] [CrossRef]
- Yakout, A.H.; Attia, M.A.; Kotb, H. Marine predator algorithm based cascaded PIDA load frequency controller for electric power systems with wave energy conversion systems. Alex. Eng. J. 2021, 60, 4213–4222. [Google Scholar] [CrossRef]
- Pradhan, P.C.; Sahu, R.K.; Panda, S. Firefly algorithm optimized fuzzy PID controller for AGC of multi-area multi-source power systems with UPFC and SMES. Eng. Sci. Technol. Int. J. 2016, 19, 338–354. [Google Scholar] [CrossRef]
- AboRas, K.M.; Ragab, M.; Shouran, M.; Alghamdi, S.; Kotb, H. Voltage and frequency regulation in smart grids via a unique Fuzzy PIDD2 controller optimized by Gradient-Based Optimization algorithm. Energy Rep. 2023, 9, 1201–1235. [Google Scholar] [CrossRef]
- Elkasem, A.H.A.; Khamies, M.; Hassan, M.H.; Agwa, A.M.; Kamel, S. Optimal design of TD-TI controller for LFC considering renewables penetration by an improved chaos game optimizer. Fractal Fract. 2022, 6, 220. [Google Scholar] [CrossRef]
- Gyugyi, L. Unified power-flow control concept for flexible AC transmission systems. IEE Proc. 1992, 139, 323. [Google Scholar] [CrossRef]
- Khadanga, R.K.; Panda, S. Gravitational search algorithm for Unified Power Flow Controller based damping controller design. In Proceedings of the 2011 International Conference on Energy, Automation and Signal, Bhubaneswar, India, 28–30 December 2011. [Google Scholar]
- Kazemi, A.; Shadmesgaran, M.R. Extended supplementary Controller of UPFC to improve damping inter-area oscillations considering inertia coefficient. Int. J. Energy 2008, 2, 25–36. [Google Scholar]
- Ezugwu, A.E.; Agushaka, J.O.; Abualigah, L.; Mirjalili, S.; Gandomi, A.H. Prairie dog optimization algorithm. Neural Comput. Appl. 2022, 34, 20017–20065. [Google Scholar] [CrossRef]
- Yang, X.-S.; Deb, S. Cuckoo search via Lévy flights. In Proceedings of the 2009 World Congress on Nature & Biologically Inspired Computing (NaBIC), Coimbatore, India, 9–11 December 2009; pp. 210–214. [Google Scholar]
- Zhu, R.; Huang, C.; Deng, S.; Li, Y. Detection of false data injection attacks based on Kalman filter and controller design in power system LFC. J. Phys. Conf. Ser. 2021, 1861, 012120. [Google Scholar] [CrossRef]
- Wu, Z.; Tian, E.; Chen, H. Covert attack detection for LFC systems of electric vehicles: A dual time-varying coding method. IEEE ASME Trans. Mechatron. 2023, 28, 681–691. [Google Scholar] [CrossRef]


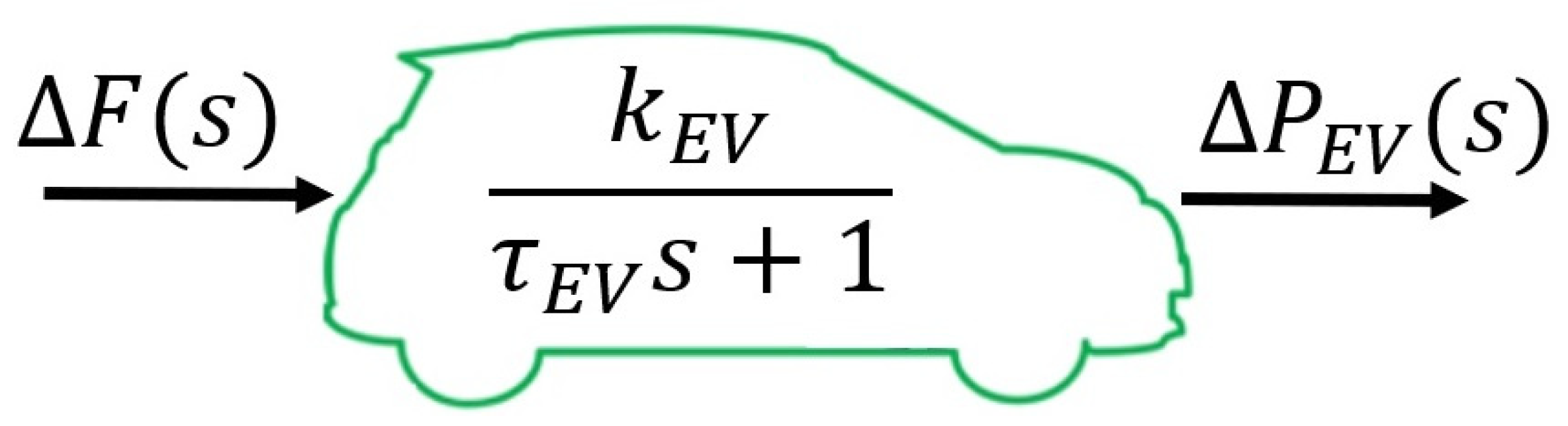










| Power Planet | Model | Transfer Function |
|---|---|---|
| Thermal | Governor | |
| Reheat | ||
| Turbine | ||
| Hydraulic | Governor | |
| Transient droop compensation | ||
| turbine | ||
| Gas | Valve positioner | |
| Speed governor | ||
| Fuel system and combustor | ||
| Compressor discharge | ||
| Others | Power system (a) | |
| Power system (b) | ||
| T-line | ||
| SMES (a) | ||
| SMES (b) | ||
| UPFC | ||
| EV (a) | ||
| EV (b) |
| E | DOE | ||||
|---|---|---|---|---|---|
| NB | SN | Z | SP | LP | |
| NB | NB | NB | NS | NS | Z |
| NS | NB | NS | NS | Z | PS |
| Z | NS | NS | Z | PS | PS |
| PS | NS | Z | PS | PS | PB |
| PB | Z | PS | PS | PB | PB |
| Controller | Thermal | Hydro | Gas | |||
|---|---|---|---|---|---|---|
| PID | Area (a) | Area (a) | Area (a) | |||
| Area (b) | Area (b) | Area (b) | ||||
| PIDA | Area (a) | Area (a) | Area (a) | |||
| Area (b) | Area (b) | Area (b) | ||||
| TFOIDFF | Area (a) | Area (a) | Area (a) | |||
| Area (b) | Area (b) | Area (b) | ||||
| FPID | Area (a) | Area (a) | Area (a) | |||
| Area (b) | Area (b) | Area (b) | ||||
| FPIDA | Area (a) | Area (a) | Area (a) | |||
| Area (b) | Area (b) | Area (b) | ||||
| FTFOIDFF | Area (a) | Area (a) | Area (a) | |||
| Area (b) | Area (b) | Area (b) | ||||
| Controller | ΔFa (Hz) | ΔFb (Hz) | ΔPtie (pu) | ITAE | ||||||
|---|---|---|---|---|---|---|---|---|---|---|
| MOS | MUS | ST | MOS | MUS | ST | MOS | MUS | ST | ||
| PID | 0.0025 | −0.034 | 30 | 0 | −0.024 | 13 | 0.0047 | −0.0346 | 30 | 3.312 |
| PIDA | 0.0026 | −0.0424 | 20 | 0.001 | −0.0475 | 17 | 0.0035 | −0.0427 | 28 | 2.103 |
| TFOIDFF | 0 | −0.063 | 13 | 0.008 | −0.0587 | 13 | 0.0016 | −0.0578 | 17 | 1.159 |
| FPID | 0 | −0.0228 | 8 | 0.002 | −0.0107 | 3.3 | 0 | −0.0228 | 3 | 0.5282 |
| FPIDA | 0 | −0.0158 | 0.4 | 0 | −0.008 | 1.5 | 0 | −0.0067 | 1 | 0.1409 |
| FTFOIDFF | 0 | −0.0158 | 0.18 | 0 | −0.0026 | 1 | 0 | −0.0056 | 0.7 | 0.0875 |
| Controller | ITAE | ITAEtot | ||
|---|---|---|---|---|
| ΔFa (Hz) | ΔFb (Hz) | ΔPtie (pu) | ||
| PID | 50.32 | 49.36 | 71.27 | 170.9 |
| PIDA | 35.35 | 48.48 | 39.47 | 123.3 |
| TFOIDFF | 28.73 | 34.73 | 24.71 | 88.17 |
| FPID | 8.039 | 6.663 | 8.06 | 22.76 |
| FPIDA | 1.804 | 2.866 | 1.491 | 6.162 |
| FTFOIDFF | 1.451 | 2.231 | 0.923 | 4.605 |
| Controller | ITAE | ITAEtot | ||
|---|---|---|---|---|
| ΔFa (Hz) | ΔFb (Hz) | ΔPtie (pu) | ||
| PID | 98.32 | 147.3 | 59.77 | 305.4 |
| PIDA | 94.31 | 155.4 | 47.36 | 297 |
| TFOIDFF | 90.72 | 117.2 | 39.85 | 247.8 |
| FPID | 11.62 | 27.77 | 5.077 | 44.46 |
| FPIDA | 6.283 | 20.09 | 2.724 | 29.09 |
| FTFOIDFF | 1.608 | 5.676 | 0.6926 | 7.976 |
| Controller | ITAE | ITAEtot | ||
|---|---|---|---|---|
| ΔFa (Hz) | ΔFb (Hz) | ΔPtie (pu) | ||
| PID | 45.48 | 30.13 | 58.21 | 133.8 |
| PIDA | 25.53 | 25.08 | 27.02 | 77.64 |
| TFOIDFF | 20.16 | 25.54 | 17.45 | 63.15 |
| FPID | 17.7 | 20.84 | 18.45 | 56.98 |
| FPIDA | 6.826 | 12.11 | 5.803 | 24.74 |
| FTFOIDFF | 2.776 | 1.101 | 0.5113 | 4.388 |
| Controller | ITAE | ITAEtot | ||
|---|---|---|---|---|
| ΔFa (Hz) | ΔFb (Hz) | ΔPtie (pu) | ||
| PID | 45.53 | 53.08 | 45.56 | 144.2 |
| PIDA | 26.19 | 31.95 | 26.89 | 85.03 |
| TFOIDFF | 16.82 | 13.75 | 17.14 | 47.71 |
| FPID | 11.75 | 5.644 | 12.08 | 29.48 |
| FPIDA | 2.485 | 2.734 | 2.406 | 7.626 |
| FTFOIDFF | 1.08 | 2.537 | 1.076 | 4.694 |
| Controller | ITAE | ITAEtot | ||
|---|---|---|---|---|
| ΔFa (Hz) | ΔFb (Hz) | ΔPtie (pu) | ||
| PID | 50.36 | 49.53 | 71.43 | 171.32 |
| PIDA | 35.55 | 49.72 | 39.79 | 125.1 |
| TFOIDFF | 28.93 | 35.9 | 25.22 | 90.05 |
| FPID | 8.065 | 6.751 | 8.225 | 23.04 |
| FPIDA | 6.222 | 4.129 | 2.413 | 12.76 |
| FTFOIDFF | 2.132 | 2.619 | 1.348 | 6.099 |
| Controller | ITAE | ITAEtot | ||
|---|---|---|---|---|
| ΔFa (Hz) | ΔFb (Hz) | ΔPtie (pu) | ||
| PID | 129.3 | 230.4 | 170.5 | 530.1 |
| PIDA | 47.43 | 245.6 | 25.69 | 318.7 |
| TFOIDFF | 56.51 | 258.4 | 30.04 | 344.9 |
| FPID | 5.713 | 6.861 | 5.619 | 18.19 |
| FPIDA | 2.554 | 7.118 | 0.7173 | 10.39 |
| FTFOIDFF | 0.777 | 3.823 | 0.6413 | 5.241 |
| Controller | ITAE | ITAEtot | ||
|---|---|---|---|---|
| ΔFa (Hz) | ΔFb (Hz) | ΔPtie (pu) | ||
| PID | 4517 | 5261 | 4501 | 14,280 |
| PIDA | 2626 | 3214 | 2671 | 8511 |
| TFOIDFF | 1704 | 1437 | 1708 | 4849 |
| FPID | 1164 | 583.7 | 1198 | 2945 |
| FPIDA | 249.6 | 281.5 | 240.2 | 771.3 |
| FTFOIDFF | 108.6 | 257.9 | 108.1 | 474.6 |
| Controller | Conditions | ΔFa (Hz) | ΔFb (Hz) | ΔPtie (pu) | ITAE | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| MOS | MUS | ST | MOS | MUS | ST | MOS | MUS | ST | |||
| FTFOIDFF | Without UPFC and SMES | 0.057 | −0.11 | 1.3 | 0.008 | −0.119 | 1 | 0.001 | −0.061 | 0.5 | 0.614 |
| With UPFC only | 0.007 | −0.125 | 0.5 | 0 | −0.026 | 2.3 | 0 | −0.011 | 3 | 0.377 | |
| With SMES only | 0.048 | −0.106 | 1.28 | 0.005 | −0.076 | 1 | 0 | −0.036 | 0.5 | 0.534 | |
| With both UPFC and SMES | 0 | −0.06 | 0.2 | 0 | −0.01 | 2 | 0 | −0.004 | 2.5 | 0.201 | |
| FTFOIDFF Optimized by PDO (Proposed) | ITAE | ITAEtot | ||
|---|---|---|---|---|
| ΔFa (Hz) | ΔFb (Hz) | ΔPtie (pu) | ||
| Without EVs | 1.946 | 3.349 | 1.367 | 6.662 |
| With EVs | 1.451 | 2.231 | 0.923 | 4.605 |
| Controller | Parameters Variation | % Variation | ΔFa (Hz) | ΔFb (Hz) | ΔPtie (pu) | ITAE | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| MOS | MUS | ST | MOS | MUS | ST | MOS | MUS | ST | ||||
| FTFOIDFF tuned by PDO (proposed) | Nominal | 0 | −0.0106 | 0.18 | 0 | −0.003 | 1 | 0 | −0.0056 | 0.7 | 0.0875 | |
| 0 | −0.0107 | 0.19 | 0 | −0.003 | 1.1 | 0 | −0.0057 | 0.7 | 0.0878 | |||
| 0 | −0.0106 | 0.17 | 0 | −0.003 | 1 | 0 | −0.0055 | 0.7 | 0.0873 | |||
| 0 | −0.0106 | 0.18 | 0 | −0.0032 | 1 | 0 | −0.0057 | 0.7 | 0.0875 | |||
| 0 | −0.0106 | 0.18 | 0 | −0.0029 | 1 | 0 | −0.0055 | 0.7 | 0.0875 | |||
| 0 | −0.0107 | 0.17 | 0 | −0.004 | 0.9 | 0 | −0.0057 | 0.5 | 0.0871 | |||
| 0.001 | −0.0105 | 0.2 | 0.0004 | −0.002 | 1.5 | 0.0002 | −0.0053 | 1 | 0.0889 | |||
| 0 | −0.0106 | 0.17 | 0 | −0.003 | 0.9 | 0 | −0.0056 | 0.6 | 0.0873 | |||
| 0 | −0.0106 | 0.2 | 0 | −0.003 | 1.2 | 0 | −0.0056 | 0.8 | 0.0881 | |||
| 0 | −0.0105 | 0.18 | 0 | −0.003 | 1 | 0 | −0.0055 | 0.7 | 0.0874 | |||
| 0 | −0.0107 | 0.18 | 0 | −0.003 | 1 | 0 | −0.0057 | 0.7 | 0.0876 | |||
| 0 | −0.0107 | 0.18 | 0 | −0.003 | 1 | 0 | −0.0057 | 0.7 | 0.0876 | |||
| 0 | −0.0105 | 0.18 | 0 | −0.003 | 1 | 0 | −0.0055 | 0.7 | 0.0874 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alghamdi, S.; Alqarni, M.; Hammad, M.R.; AboRas, K.M. First-of-Its-Kind Frequency Enhancement Methodology Based on an Optimized Combination of FLC and TFOIDFF Controllers Evaluated on EVs, SMES, and UPFC-Integrated Smart Grid. Fractal Fract. 2023, 7, 807. https://doi.org/10.3390/fractalfract7110807
Alghamdi S, Alqarni M, Hammad MR, AboRas KM. First-of-Its-Kind Frequency Enhancement Methodology Based on an Optimized Combination of FLC and TFOIDFF Controllers Evaluated on EVs, SMES, and UPFC-Integrated Smart Grid. Fractal and Fractional. 2023; 7(11):807. https://doi.org/10.3390/fractalfract7110807
Chicago/Turabian StyleAlghamdi, Sultan, Mohammed Alqarni, Muhammad R. Hammad, and Kareem M. AboRas. 2023. "First-of-Its-Kind Frequency Enhancement Methodology Based on an Optimized Combination of FLC and TFOIDFF Controllers Evaluated on EVs, SMES, and UPFC-Integrated Smart Grid" Fractal and Fractional 7, no. 11: 807. https://doi.org/10.3390/fractalfract7110807
APA StyleAlghamdi, S., Alqarni, M., Hammad, M. R., & AboRas, K. M. (2023). First-of-Its-Kind Frequency Enhancement Methodology Based on an Optimized Combination of FLC and TFOIDFF Controllers Evaluated on EVs, SMES, and UPFC-Integrated Smart Grid. Fractal and Fractional, 7(11), 807. https://doi.org/10.3390/fractalfract7110807









