Dynamic Modeling and Response Analysis of Dielectric Elastomer Incorporating Fractional Viscoelasticity and Gent Function
Abstract
:1. Introduction
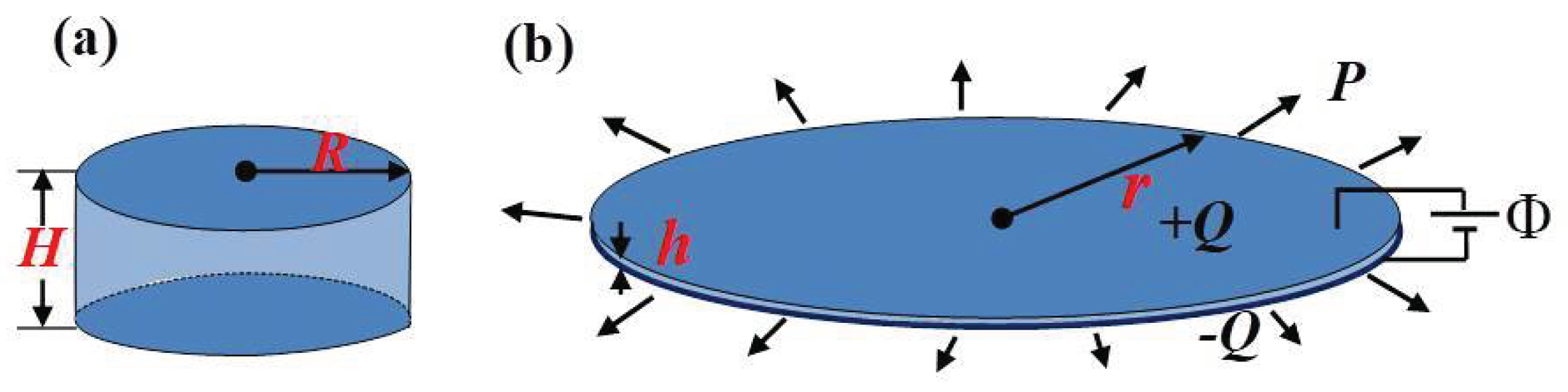
2. Governing Equation Incorporating Stiffening and Viscoelasticity
2.1. A Fractional Model of Viscoelastic Behavior of DE
2.2. The Method of Virtual Work
3. Nonlinear Dynamic Analysis of DE with Fractional Damping
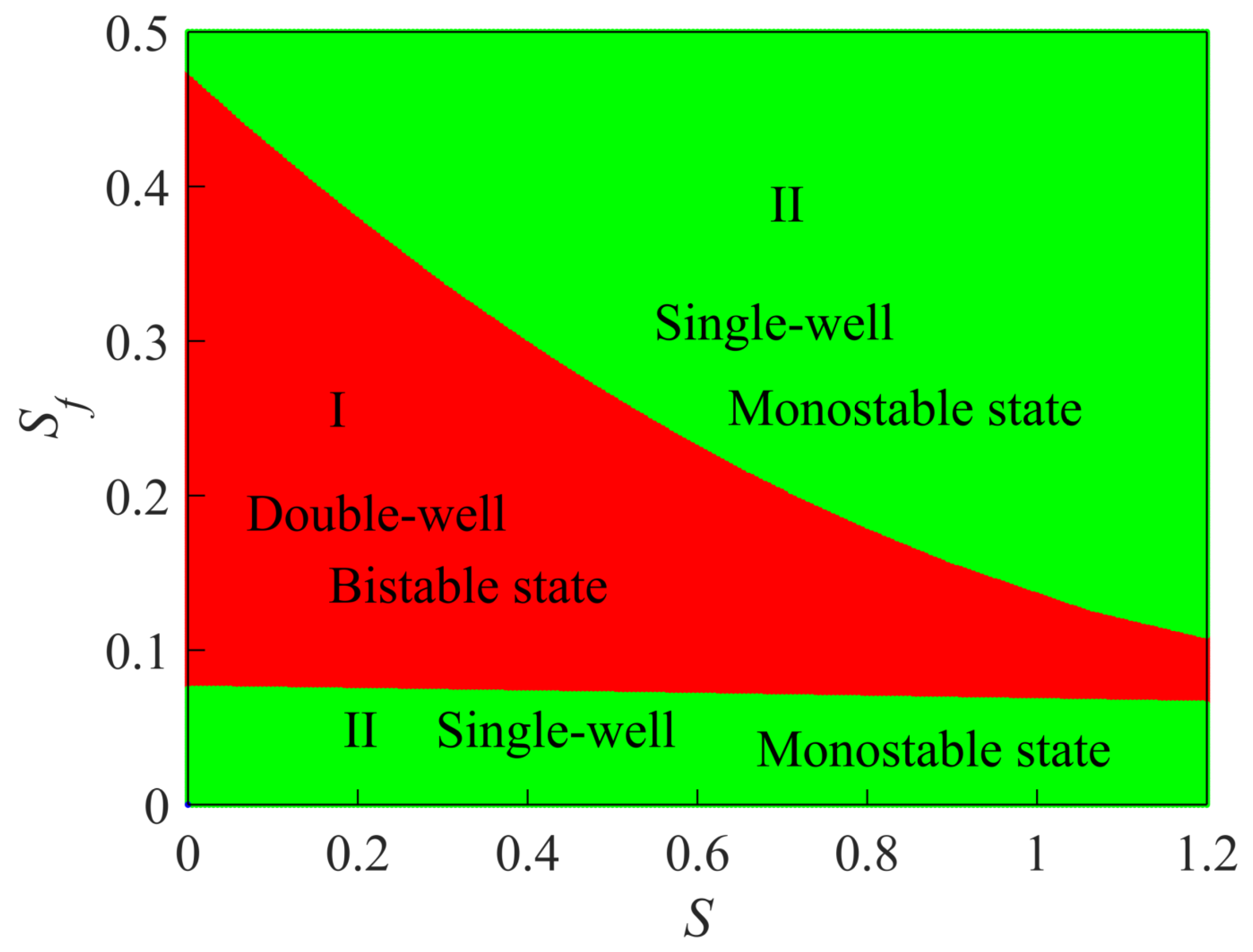
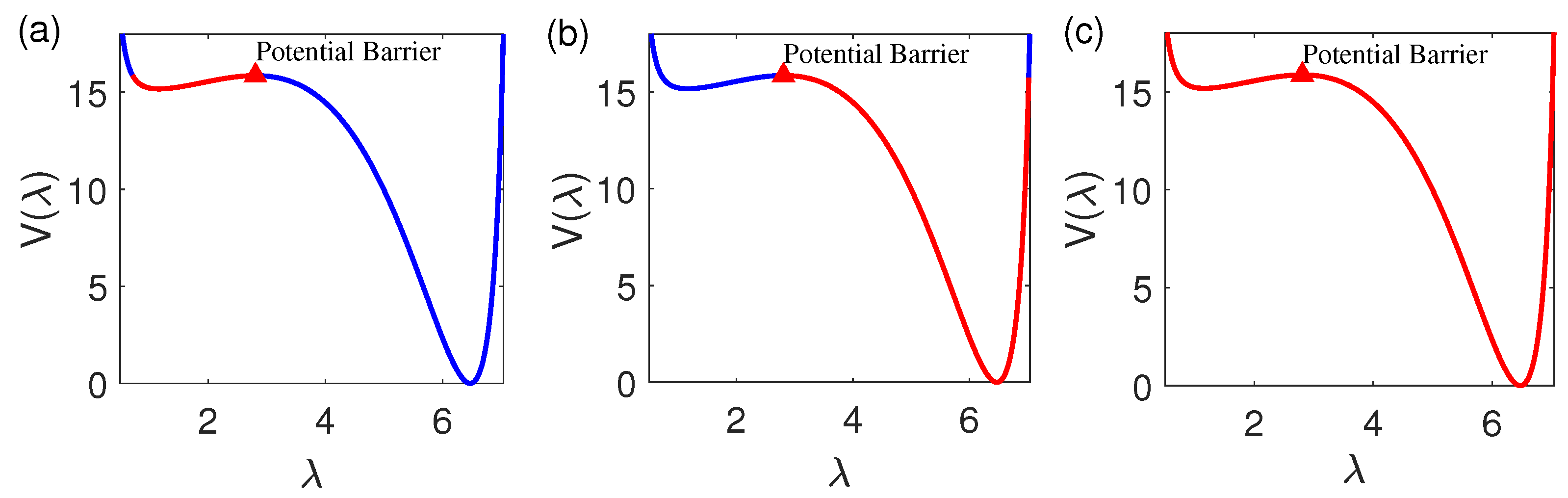
3.1. Preliminary Study of System Response Using the Potential Function
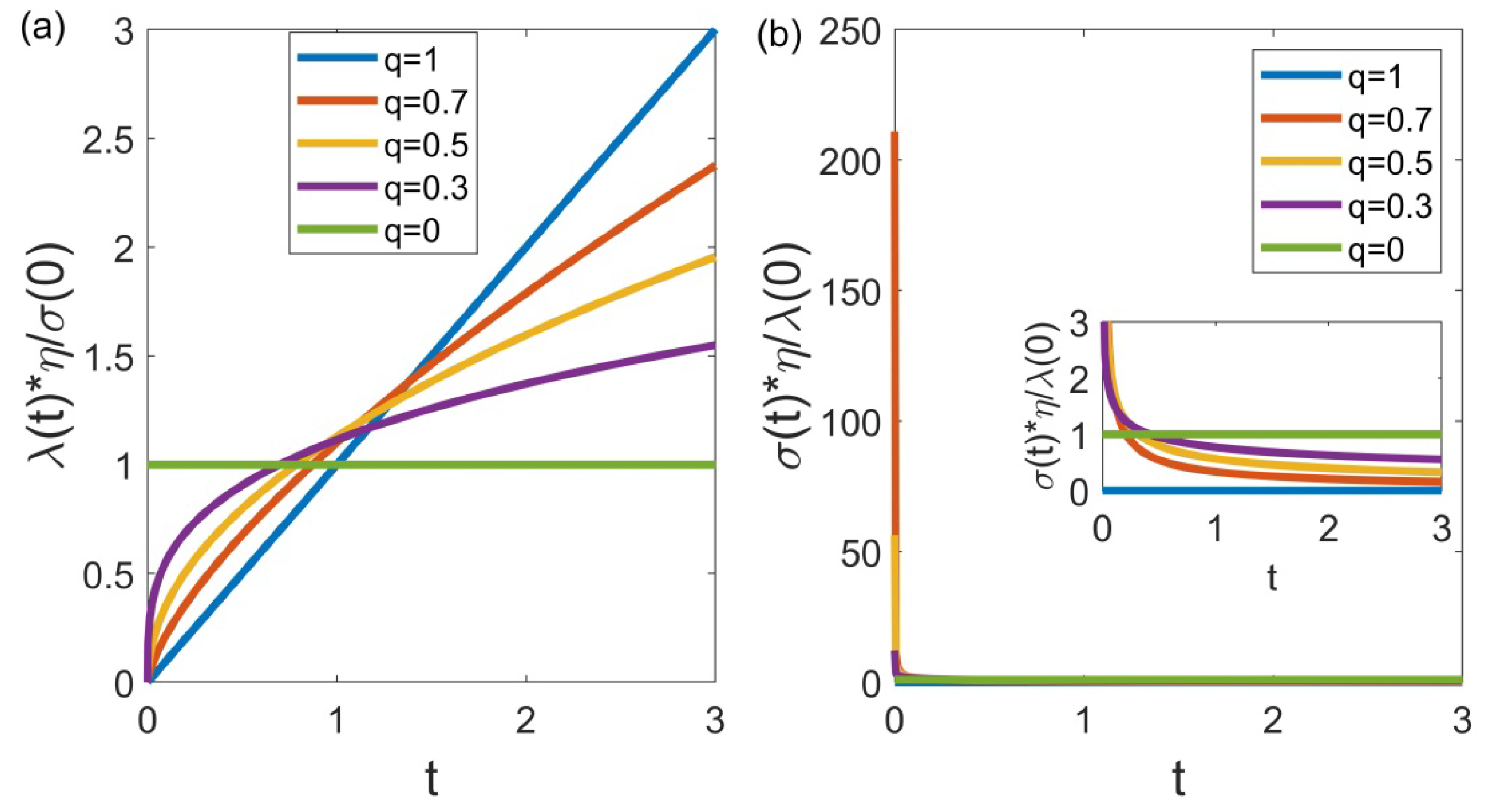
3.2. The Effect of Fractional Derivative on System
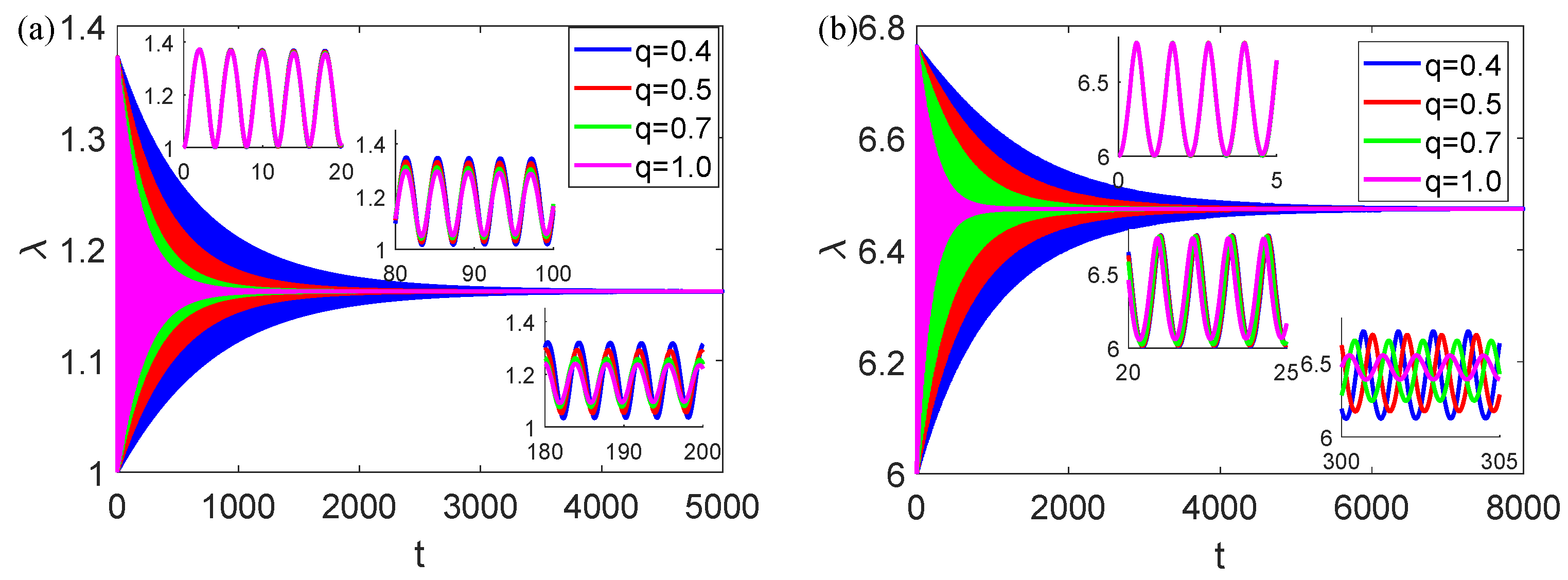
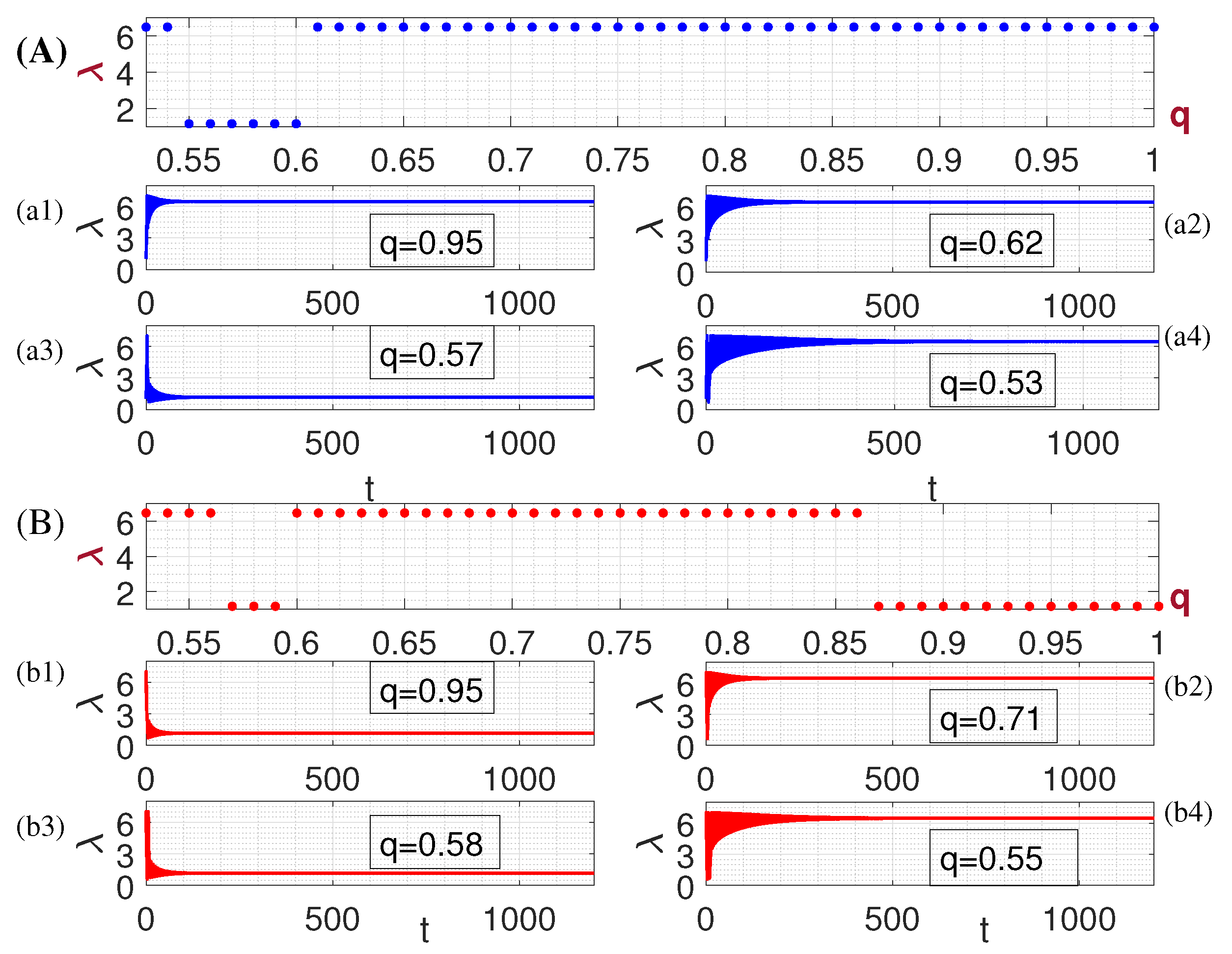
3.2.1. Oscillation in the Single Potential Well
3.2.2. Oscillation between Two Potential Wells
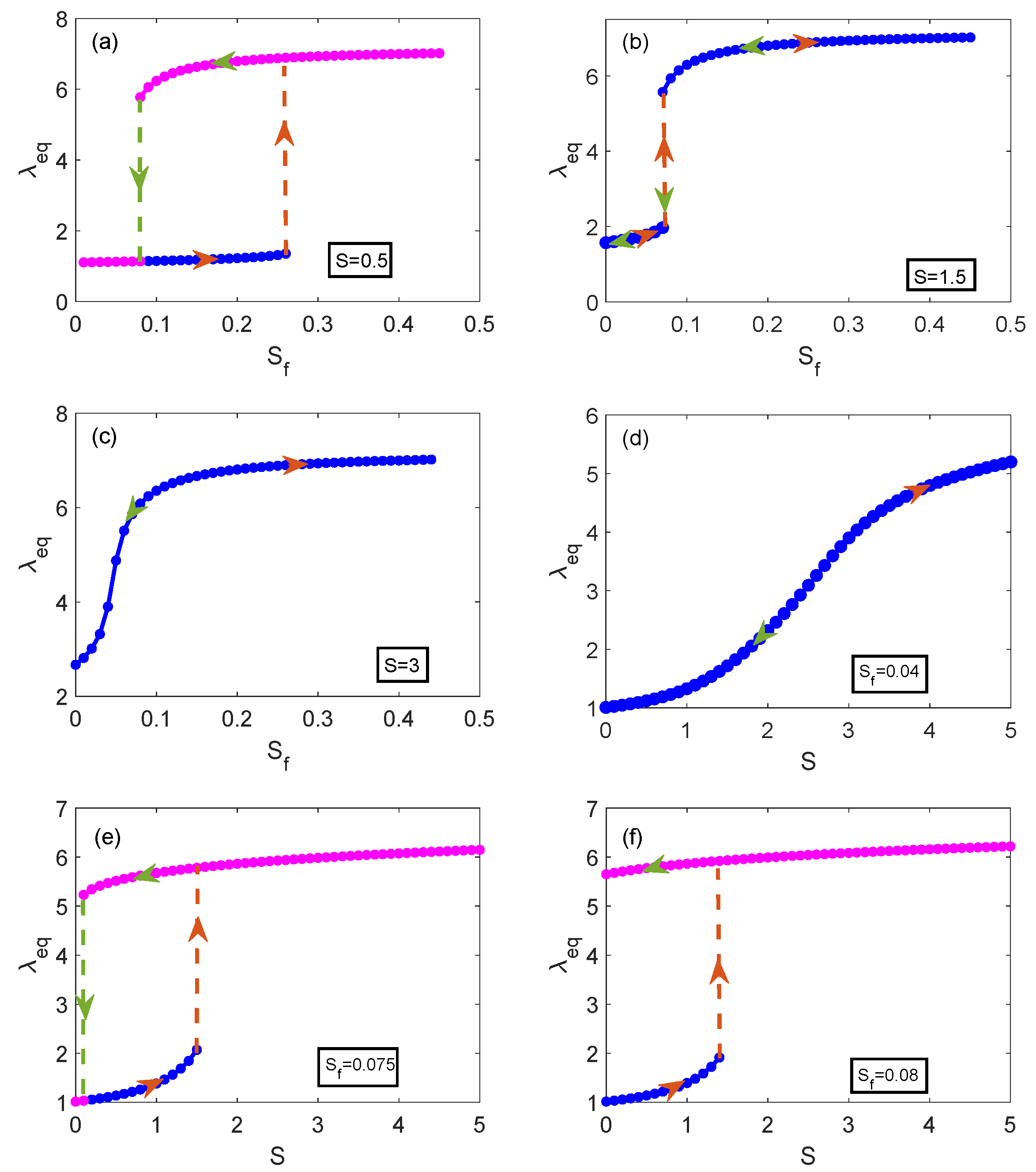
3.2.3. The Effect of Electromechanical Coupling Parameters on the Response of Equilibrium State
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Notation
| voltage (kV) | |
| P | radial force (N) |
| stretch ratio at time t | |
| radius at time t (mm) | |
| thickness at time t (mm) | |
| electric displacement at time t | |
| the charges of polarity carried on surface at time t | |
| viscoelastic stress | |
| W | the density of Helmholtz free energy |
| the work carried out by the voltage | |
| the work carried out by the radial force | |
| the work carried out by the inertial force | |
| the work carried out by the viscoelastic stress |
References
- Anderson, I.A.; Gisby, T.A.; McKay, T.G.; O’Brien, B.M.; Calius, E.P. Multi-functional dielectric elastomer artificial muscles for soft and smart machines. J. Appl. Phys. 2012, 112, 041101. [Google Scholar] [CrossRef]
- Henke, E.F.M.; Schlatter, S.; Anderson, I.A. Soft dielectric elastomer oscillators driving bioinspired robots. Soft Robot. 2017, 4, 353–366. [Google Scholar] [CrossRef] [PubMed]
- Shintake, J.; Cacucciolo, V.; Shea, H.; Floreano, D. Soft biomimetic fish robot made of dielectric elastomer actuators. Soft Robot. 2018, 5, 466–474. [Google Scholar] [CrossRef] [PubMed]
- Zhao, J.; Niu, J.; McCoul, D.; Ren, Z.; Pei, Q. Phenomena of nonlinear oscillation and special resonance of a dielectric elastomer minimum energy structure rotary joint. Appl. Phys. Lett. 2015, 106, 133504. [Google Scholar] [CrossRef]
- O’Brien, B.M.; Calius, E.P.; Inamura, T.; Xie, S.Q.; Anderson, I.A. Dielectric elastomer switches for smart artificial muscles. Appl. Phys. A 2010, 100, 385–389. [Google Scholar] [CrossRef]
- Kornbluh, R.D.; Pelrine, R.; Pei, Q.; Heydt, R.; Stanford, S.; Oh, S.; Eckerle, J. Electroelastomers: Applications of dielectric elastomer transducers for actuation, generation, and smart structures. In Proceedings of the SPIE’s 9th Annual International Symposium on Smart Structures and Materials, San Diego, CA, USA, 17–21 March 2002; Volume 4698, pp. 254–270. [Google Scholar]
- Pei, Q.; Pelrine, R.; Stanford, S.; Kornbluh, R.; Rosenthal, M. Electroelastomer rolls and their application for biomimetic walking robots. Synth. Met. 2003, 135, 129–131. [Google Scholar] [CrossRef]
- Stark, K.; Garton, C. Electric strength of irradiated polythene. Nature 1955, 176, 1225–1226. [Google Scholar] [CrossRef]
- Guo, Y.; Liu, L.; Liu, Y.; Leng, J. Review of Dielectric Elastomer Actuators and Their Applications in Soft Robots. Adv. Intell. Syst. 2021, 3, 2000282. [Google Scholar] [CrossRef]
- Cao, C.; Chen, L.; Hill, T.L.; Wang, L.; Gao, X. Exploiting Bistability for High-Performance Dielectric Elastomer Resonators. IEEE/ASME Trans. Mechatron. 2022, 27, 5994–6005. [Google Scholar] [CrossRef]
- Liu, N.; Martinez, T.; Walter, A.; Civet, Y.; Perriard, Y. Control-Oriented Modeling and Analysis of Tubular Dielectric Elastomer Actuators Dedicated to Cardiac Assist Devices. IEEE Robot. Autom. Lett. 2022, 7, 4361–4367. [Google Scholar] [CrossRef]
- Acome, E.; Mitchell, S.K.; Morrissey, T.G.; Emmett, M.B.; Benjamin, C.; King, M.; Radakovitz, M.; Keplinger, C. Hydraulically amplified self-healing electrostatic actuators with muscle-like performance. Science 2018, 359, 61–65. [Google Scholar] [CrossRef] [PubMed]
- Cacucciolo, V.; Shintake, J.; Kuwajima, Y.; Maeda, S.; Floreano, D.; Shea, H. Stretchable pumps for soft machines. Nature 2019, 572, 516–519. [Google Scholar] [CrossRef] [PubMed]
- Mockensturm, E.M.; Goulbourne, N. Dynamic response of dielectric elastomers. Int. J. Non-Linear Mech. 2006, 41, 388–395. [Google Scholar] [CrossRef]
- Fox, J.; Goulbourne, N. On the dynamic electromechanical loading of dielectric elastomer membranes. J. Mech. Phys. Solids 2008, 56, 2669–2686. [Google Scholar] [CrossRef]
- Zhu, J.; Cai, S.; Suo, Z. Nonlinear oscillation of a dielectric elastomer balloon. Polym. Int. 2010, 59, 378–383. [Google Scholar] [CrossRef]
- Son, S.; Goulbourne, N. Dynamic response of tubular dielectric elastomer transducers. Int. J. Solids Struct. 2010, 47, 2672–2679. [Google Scholar] [CrossRef]
- Yong, H.; He, X.; Zhou, Y. Dynamics of a thick-walled dielectric elastomer spherical shell. Int. J. Eng. Sci. 2011, 49, 792–800. [Google Scholar] [CrossRef]
- Yin, Y.; Zhao, D.; Liu, J.; Xu, Z. Nonlinear dynamic analysis of dielectric elastomer membrane with electrostriction. Appl. Math. Mech. 2022, 43, 793–812. [Google Scholar] [CrossRef]
- Pelrine, R.; Kornbluh, R.; Pei, Q.; Joseph, J. High-speed electrically actuated elastomers with strain greater than 100%. Science 2000, 287, 836–839. [Google Scholar] [CrossRef]
- An, L.; Wang, F.; Cheng, S.; Lu, T.; Wang, T. Experimental investigation of the electromechanical phase transition in a dielectric elastomer tube. Smart Mater. Struct. 2015, 24, 035006. [Google Scholar] [CrossRef]
- Keplinger, C.; Li, T.; Baumgartner, R.; Suo, Z.; Bauer, S. Harnessing snap-through instability in soft dielectrics to achieve giant voltage-triggered deformation. Soft Matter 2012, 8, 285–288. [Google Scholar] [CrossRef]
- Li, T.; Keplinger, C.; Baumgartner, R.; Bauer, S.; Yang, W.; Suo, Z. Giant voltage-induced deformation in dielectric elastomers near the verge of snap-through instability. J. Mech. Phys. Solids 2013, 61, 611–628. [Google Scholar] [CrossRef]
- Lu, T.Q.; Suo, Z.G. Large conversion of energy in dielectric elastomers by electromechanical phase transition. Acta Mech. Sin. 2012, 28, 1106–1114. [Google Scholar] [CrossRef]
- Zhao, X.; Hong, W.; Suo, Z. Electromechanical hysteresis and coexistent states in dielectric elastomers. Phys. Rev. B 2007, 76, 134113. [Google Scholar] [CrossRef]
- Zhou, J.; Hong, W.; Zhao, X.; Zhang, Z.; Suo, Z. Propagation of instability in dielectric elastomers. Int. J. Solids Struct. 2008, 45, 3739–3750. [Google Scholar] [CrossRef]
- Wang, F.; Lu, T.; Wang, T. Nonlinear vibration of dielectric elastomer incorporating strain stiffening. Int. J. Solids Struct. 2016, 87, 70–80. [Google Scholar] [CrossRef]
- Lv, X.; Liu, L.; Liu, Y.; Leng, J. Dynamic performance of dielectric elastomer balloon incorporating stiffening and damping effect. Smart Mater. Struct. 2018, 27, 105036. [Google Scholar] [CrossRef]
- Löwe, C.; Zhang, X.; Kovacs, G. Dielectric elastomers in actuator technology. Adv. Eng. Mater. 2005, 7, 361–367. [Google Scholar] [CrossRef]
- Kornbluh, R.D.; Pelrine, R.; Pei, Q.; Oh, S.; Joseph, J. Ultrahigh strain response of field-actuated elastomeric polymers. In Proceedings of the SPIE’s 7th Annual International Symposium on Smart Structures and Materials, Newport Beach, CA, USA, 6–9 March 2000; Volume 3987, pp. 51–64. [Google Scholar]
- Plante, J.S.; Dubowsky, S. Large-scale failure modes of dielectric elastomer actuators. Int. J. Solids Struct. 2006, 43, 7727–7751. [Google Scholar] [CrossRef]
- Yang, E.; Frecker, M.; Mockensturm, E. Viscoelastic model of dielectric elastomer membranes. In Proceedings of the SPIE Smart Structures and Materials + Nondestructive Evaluation and Health Monitoring, San Diego, CA, USA, 7–10 March 2005; Volume 5759, pp. 82–93. [Google Scholar]
- Chiang Foo, C.; Cai, S.; Jin Adrian Koh, S.; Bauer, S.; Suo, Z. Model of dissipative dielectric elastomers. J. Appl. Phys. 2012, 111, 034102. [Google Scholar] [CrossRef]
- Hong, W. Modeling viscoelastic dielectrics. J. Mech. Phys. Solids 2011, 59, 637–650. [Google Scholar] [CrossRef]
- Zhang, J.; Ru, J.; Chen, H.; Li, D.; Lu, J. Viscoelastic creep and relaxation of dielectric elastomers characterized by a Kelvin-Voigt-Maxwell model. Appl. Phys. Lett. 2017, 110, 044104. [Google Scholar] [CrossRef]
- Mashayekhi, S.; Miles, P.; Hussaini, M.Y.; Oates, W.S. Fractional viscoelasticity in fractal and non-fractal media: Theory, experimental validation, and uncertainty analysis. J. Mech. Phys. Solids 2017, 111, 134–156. [Google Scholar] [CrossRef]
- Karner, T.; Gotlih, J.; Razboršek, B.; Vuherer, T.; Berus, L.; Gotlih, K. Use of single and double fractional Kelvin–Voigt model on viscoelastic elastomer. Smart Mater. Struct. 2020, 29, 015006. [Google Scholar] [CrossRef]
- Stanisauskis, E.; Mashayekhi, S.; Pahari, B.; Mehnert, M.; Steinmann, P.; Oates, W. Fractional and fractal order effects in soft elastomers: Strain rate and temperature dependent nonlinear mechanics. Mech. Mater. 2022, 172, 104390. [Google Scholar] [CrossRef]
- Mainardi, F. Fractional Viscoelastic Models; Royal Society of Chemistry: London, UK, 2010; pp. 57–76. [Google Scholar]
- Rogosin, S.; Mainardi, F. George William Scott Blair—The pioneer of factional calculus in rheology. arXiv 2014, arXiv:1404.3295. [Google Scholar]
- Momani, S.; Al-Khaled, K. Numerical solutions for systems of fractional differential equations by the decomposition method. Appl. Math. Comput. 2005, 162, 1351–1365. [Google Scholar] [CrossRef]
- Poltem, D.; Sak-Aree-Amorn, S. Natural Homotopy Perturbation Method for System of Nonlinear Partial Differential Equations. Far East J. Math. Sci. (FJMS) 2017, 102, 631–644. [Google Scholar] [CrossRef]
- Chen, Y.Q.; Moore, K.L. Discretization schemes for fractional-order differentiators and integrators. IEEE Trans. Circuits Syst. Regul. Pap. 2002, 49, 363–367. [Google Scholar] [CrossRef]
- Chen, L.; Zhao, T.; Li, W.; Zhao, J. Bifurcation control of bounded noise excited Duffing oscillator by a weakly fractional-order feedback controller. Nonlinear Dyn. 2016, 83, 529–539. [Google Scholar] [CrossRef]
- Bagley, R.L.; Torvik, P.J. Fractional calculus—A different approach to the analysis of viscoelastically damped structures. AIAA J. 1983, 21, 741–748. [Google Scholar] [CrossRef]
- Schiessel, H.; Blumen, A. Hierarchical analogues to fractional relaxation equations. J. Phys. Math. Gen. 1993, 26, 5057. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, Q.; Sun, Z. Dynamic Modeling and Response Analysis of Dielectric Elastomer Incorporating Fractional Viscoelasticity and Gent Function. Fractal Fract. 2023, 7, 786. https://doi.org/10.3390/fractalfract7110786
Li Q, Sun Z. Dynamic Modeling and Response Analysis of Dielectric Elastomer Incorporating Fractional Viscoelasticity and Gent Function. Fractal and Fractional. 2023; 7(11):786. https://doi.org/10.3390/fractalfract7110786
Chicago/Turabian StyleLi, Qiaoyan, and Zhongkui Sun. 2023. "Dynamic Modeling and Response Analysis of Dielectric Elastomer Incorporating Fractional Viscoelasticity and Gent Function" Fractal and Fractional 7, no. 11: 786. https://doi.org/10.3390/fractalfract7110786
APA StyleLi, Q., & Sun, Z. (2023). Dynamic Modeling and Response Analysis of Dielectric Elastomer Incorporating Fractional Viscoelasticity and Gent Function. Fractal and Fractional, 7(11), 786. https://doi.org/10.3390/fractalfract7110786





