An Algorithm for the Solution of Nonlinear Volterra–Fredholm Integral Equations with a Singular Kernel
Abstract
:1. Introduction
2. The Existence and Unique Solution of Equation (1)
- (i)
- The kernel of position satisfies the discontinuity condition
- (ii)
- The kernel of time is continuous and satisfies
- (iii)
- The norm of the continuous given function is defined as
- (iv)
- The known function for the constants and satisfies:where
3. The Stability and Rate of Convergence
4. The System of Fredholm Integral Equations
5. Chebyshev Polynomials
- Case (i): For , we obtainmultiplying both sides of (30) by the term , then integrating the result from to 1, yield the following result:
- Case (iii): For , we deducewhere
- Case (iv): For , we can confirmMultiplying both sides of (34) by the term , then integrating the result from to 1, we yield the following result:
6. Convergence Analysis
6.1. The Existence and Unique Numerical Solution
6.2. Error Analysis of the Numerical Solution
- a.
- The kernel of position satisfies a condition:
- b.
- The kernel of time satisfies a condition:
- c.
- The nonlinear continuous function satisfies a condition:
7. Numerical Applications
8. General Conclusions
- In comparison to the other approaches, the one provided in this study is the most effective numerical method for solving the NVFIE with a single kernel.
- The approach described in this study can be generalized to more general kernels.
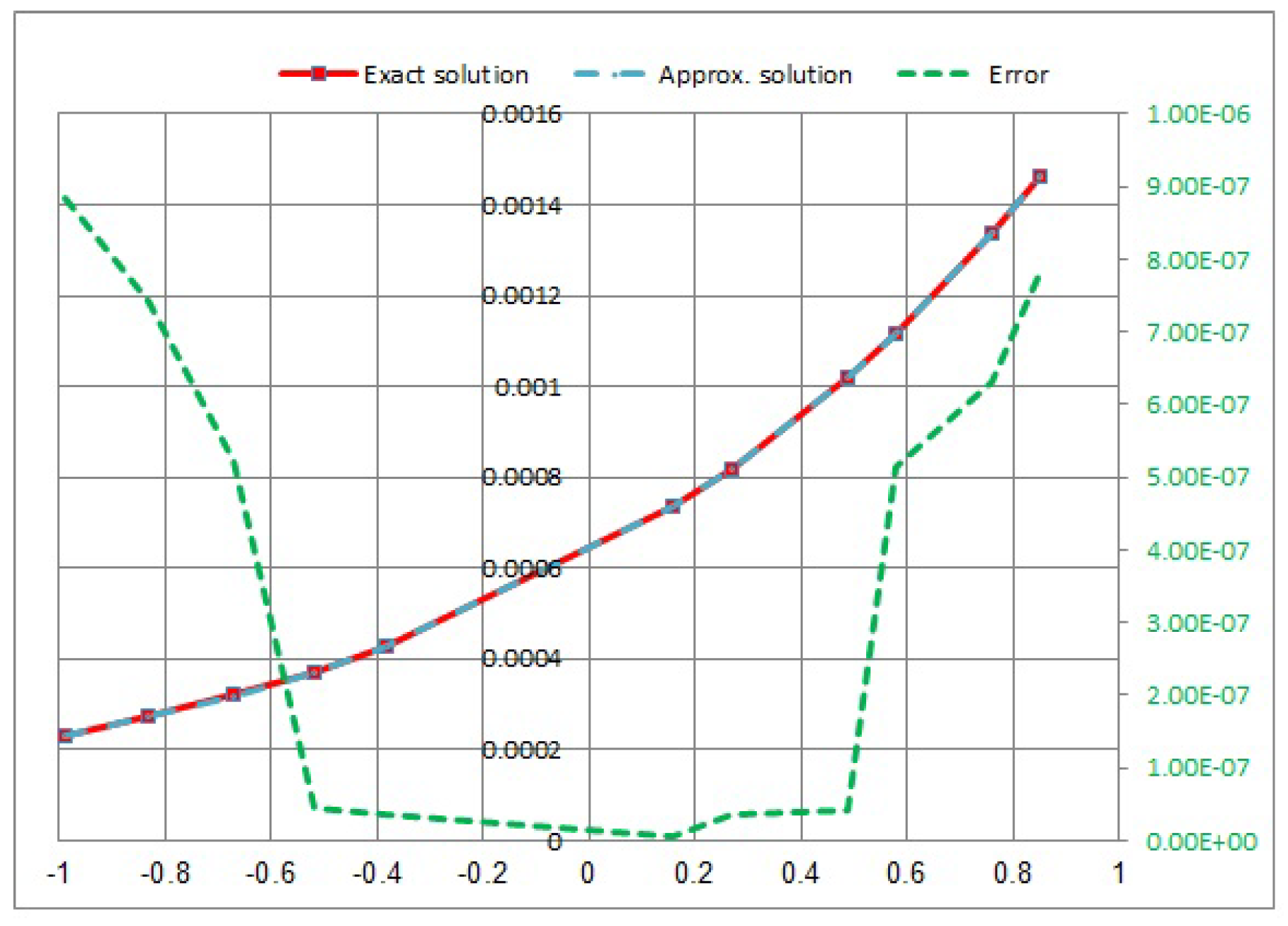
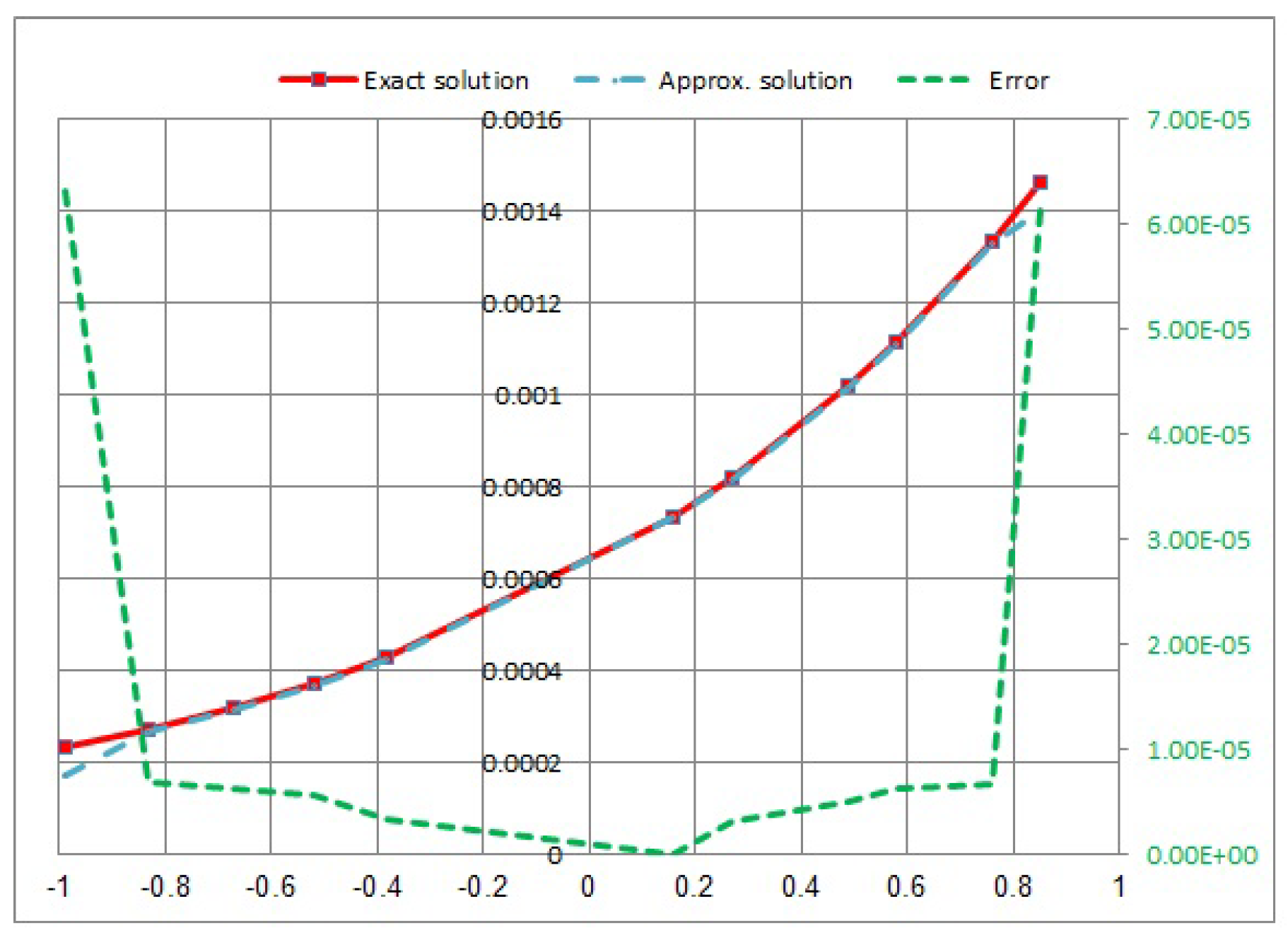
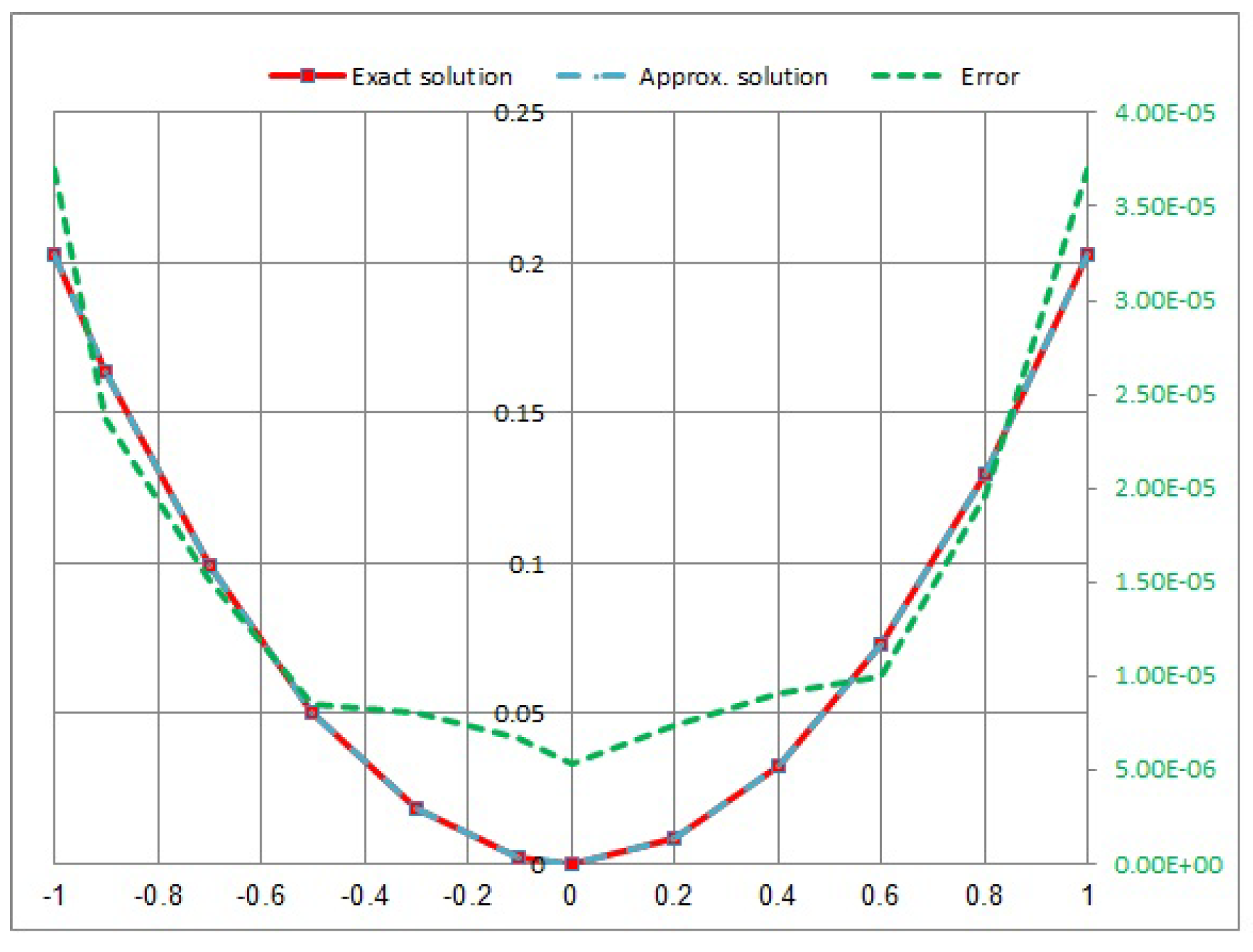
- In Example 1, the quadratic integral Equation (41) after using the quadrature method leads to a system of nonlinear integral equations. It can be observed that the error increases with an increasing M. When we take the maximum value error, Figure 4 is at . Furthermore, the minimum error value in Figure 1 is at (see Table 1).
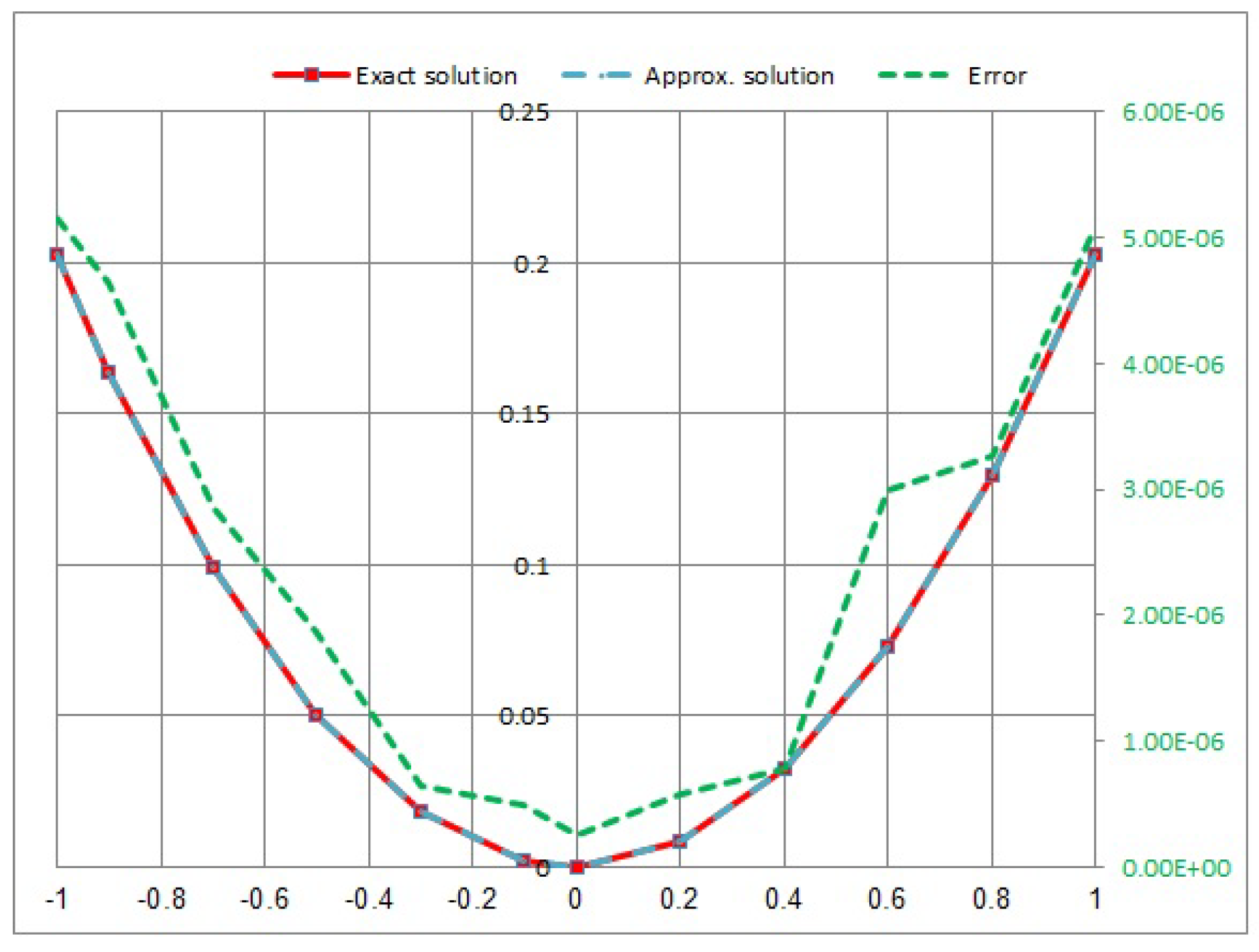
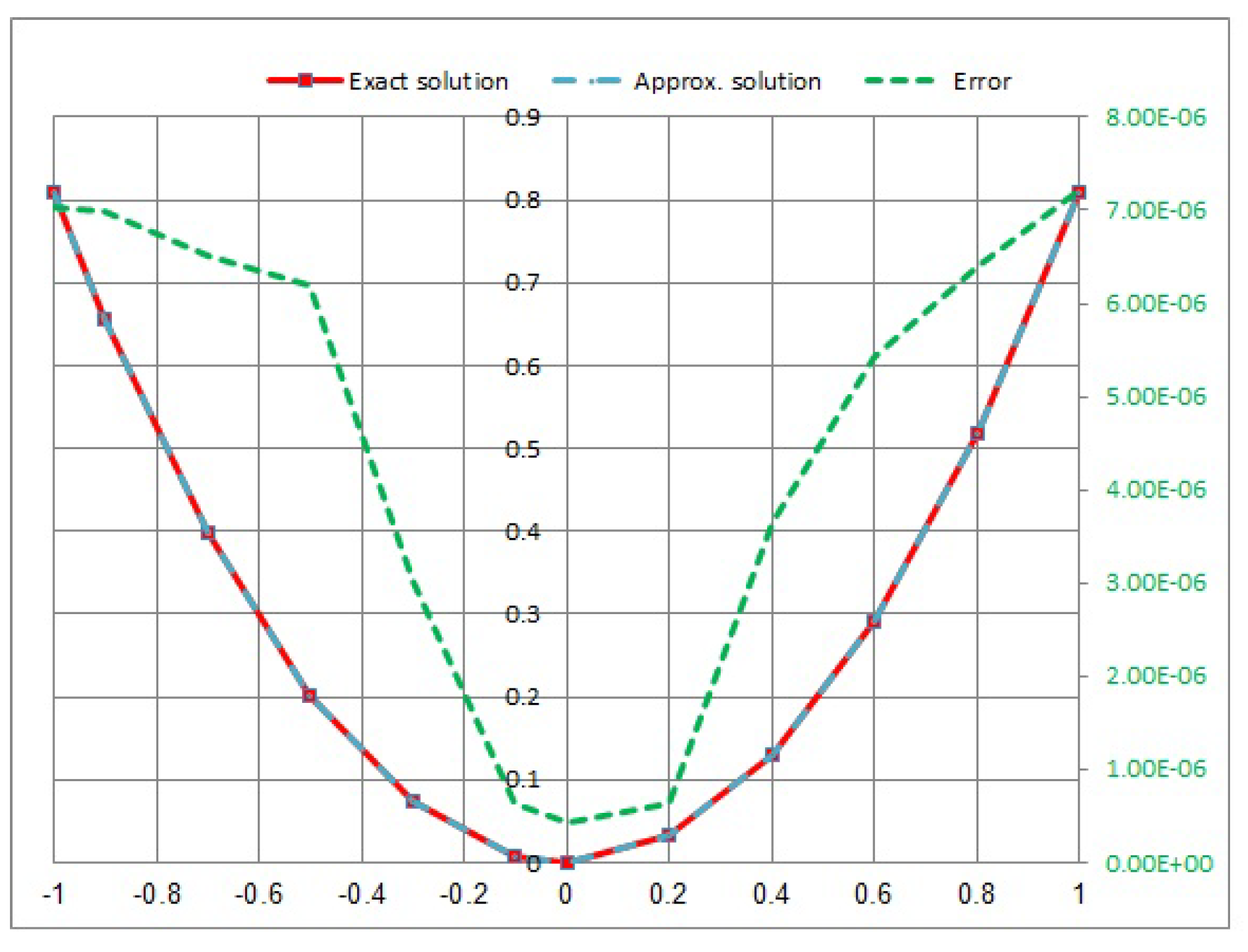
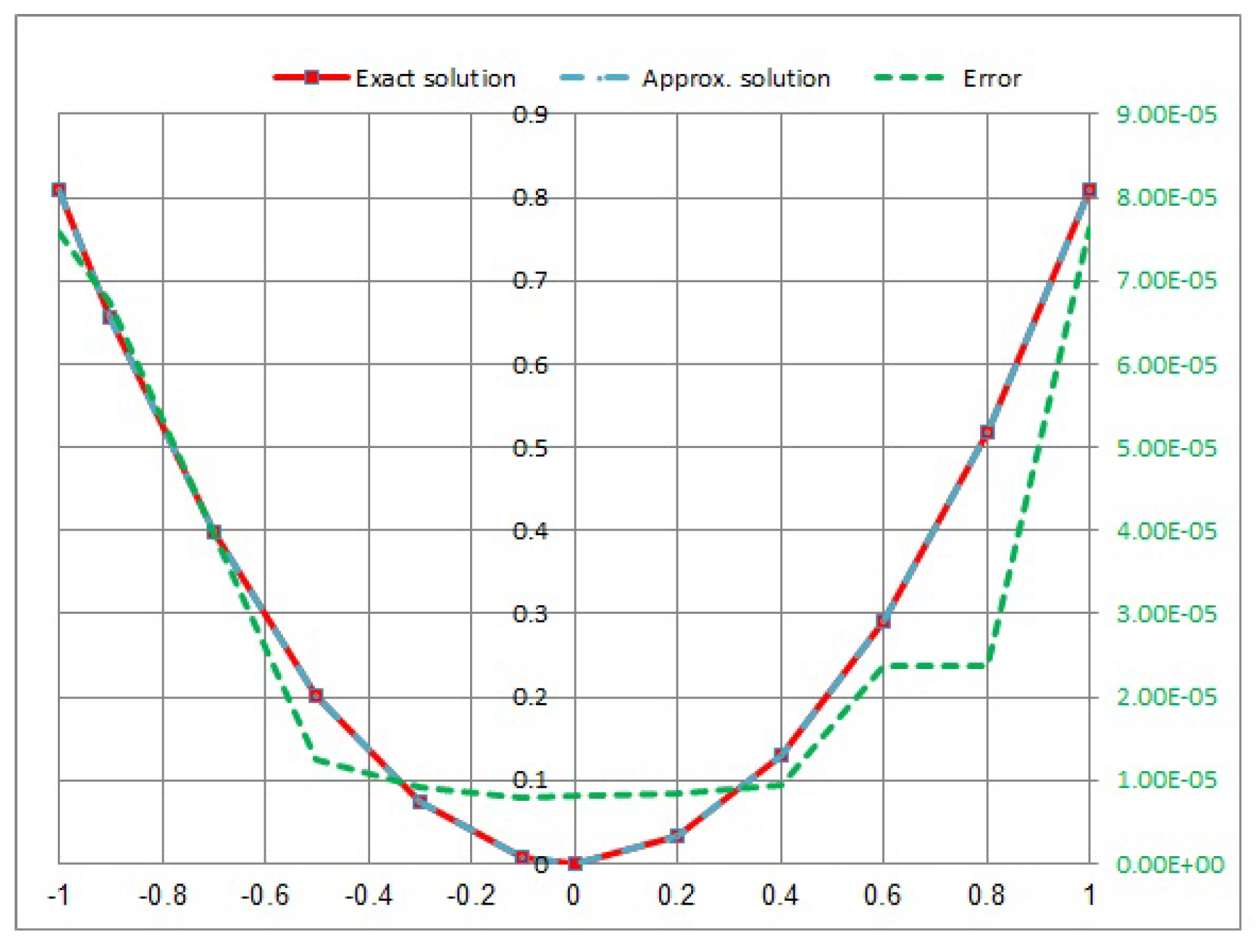
- In Example 2, the quadratic integral Equation (42) after using the Chebyshev polynomials leads to a system of nonlinear integral equations. It can be observed that the error increases with an increase in time. When we take the maximum value error, Figure 8 is at . Furthermore, the minimum error value in Figure 4 is at (see Table 2).
- In Example 3, when the kernel takes the logarithmic kernel , the results are computed at , and for (see Table 3). As increases, and the errors decrease. The error takes the maximum value at , where t is increasing, and vice versa.
- In Example 5, we consider the NVFIE with the kernel as the Carleman kernel . When we take the maximum value error, the ends of position is at . Furthermore, the minimum error value in the midpoint of the position is at (see Table 5). The error takes the maximum value at , and vice versa.
9. Future Work
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Amin, R.; Nazir, S.; García-Magariño, I. Efficient sustainable algorithm for the numeri-cal solution of nonlinear delay Fredholm-Volterra integral equations via Haar wavelet for dense sensor networks in emerging telecommuni-cations. Trans. Emerg. Telecommun. Technol. 2020, 33, 38–77. [Google Scholar]
- Warnick, K.F. Numerical Analysis for Electromagnetic Integral Equations; Artech House: Norwood, MA, USA, 2008. [Google Scholar]
- Popov, G.Y. Contact Problem for a Linearly Deformable Base; Springer: Moscow, Russian, 1982. [Google Scholar]
- Ramadan, M.A.; Osheba, H.S.; Hadhoud, A.R. A numerical method based on hybrid or-thonormal Bernstein and improved block-pulse functions for solving Volterra-Fredholm integral equations. Numer. Methods Partial Differ. Equ. 2023, 39, 268–280. [Google Scholar] [CrossRef]
- Mahdy, A.M.S.; Nagdy, A.S.; Hashem, K.H.; Mohamed, D.S. A Computational Technique for Solving Three-Dimensional Mixed Volterra–Fredholm Integral Equations. Fractal Fract. 2023, 7, 196. [Google Scholar] [CrossRef]
- Majouti, Z.E.; Jid, R.E.; Hajjaj, A. Numerical solution for three-dimensional nonlinear mixed Volterra-Fredholm integral equations via modified moving least-square method. Int. J. Comput. Math. 2022, 99, 1849–1867. [Google Scholar] [CrossRef]
- Micula, S. On Some Iterative Numerical Methods for Mixed Volterra-Fredholm Integral Equations. Symmetry 2019, 11, 1200. [Google Scholar] [CrossRef]
- Nwaigwe, C.; Micula, S. Fast and Accurate Numerical Algorithm with Performance Assess-ment for Nonlinear Functional Volterra Equations. Fractal Fract. 2023, 7, 333. [Google Scholar] [CrossRef]
- Chen, Z.; Jiang, W. An approximate solution for a mixed linear Volterra-Fredholm integral equation. Appl. Math. Lett. 2012, 25, 1131–1134. [Google Scholar] [CrossRef]
- Nasr, M.E.; Abdel-Aty, M.A. Theoretical and Numerical Discussion for the Mixed In-tegro–Differential Equations. J. Comput. Appl. 2021, 29, 880–892. [Google Scholar]
- Wazwaz, A.M. Linear and Nonlinear Integral Equations; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Erfanian, M.; Zeidabadi, H. Using of Bernstein spectral Galerkin method for solving of weakly singular Volterra-Fredholm integral equations. Math. Sci. 2018, 12, 103–109. [Google Scholar] [CrossRef]
- Das, P.; Nelakanti, G. Convergence Analysis of Legendre Spectral Galerkin Method for Volterra-Fredholm-Hammerstein Integral Equations. In Mathematical Analysis and Its Applications; Agrawal, P., Mohapatra, R., Singh, U., Srivastava, H., Eds.; Springer: New Delhi, India, 2015; Volume 143, pp. 3–15. [Google Scholar]
- Jebreen, H.B. On the Multiwavelets Galerkin Solution of the Volterra-Fredholm Integral Equa-tions by an Efficient Algorithm. J. Math. 2020, 2020, 2672683. [Google Scholar] [CrossRef]
- Wang, X. A New Wavelet Method for Solving a Class of Nonlinear Volterra-Fredholm Inte-gral Equations. Abstr. Appl. Anal. 2014, 2014, 975–985. [Google Scholar] [CrossRef]
- Abdou, M.A.; Nasr, M.E.; Abdel–Aty, M.A. A study of normality and continuity for mixed integral equations. J. Fixed Point Theory Appl. 2018, 20, 5. [Google Scholar] [CrossRef]
- Georgieva, A.; Hristova, S. Homotopy Analysis Method to Solve Two-Dimensional Nonlinear Volterra-Fredholm Fuzzy Integral Equations. Fractal Fract. 2020, 4, 9. [Google Scholar] [CrossRef]
- Dong, C.; Chen, Z.; Jiang, W. A modified homotopy perturbation method for solving the non-linear mixed Volterra-Fredholm integral equation. J. Comput. Appl. Math. 2013, 239, 359–366. [Google Scholar] [CrossRef]
- Wang, Q.; Wang, K.; Chen, S. Least squares approximation method for the solution of Volterra-Fredholm integral equations. J. Comput. Appl. Math. 2014, 272, 141–147. [Google Scholar] [CrossRef]
- Al-Bugami, A.; Al-Juaid, J. Runge–Kutta Method and Block by Block Method to Solve Non-linear Fredholm-Volterra Integral Equation with Continuous Kernel. J. Appl. Math. Phys. 2020, 82, 2043–2054. [Google Scholar] [CrossRef]
- Deniz, S. Optimal perturbation iteration technique for solving nonlinear Volterra-Fredholm in-tegral equations. Math. Methods Appl. Sci. 2020, 2020, 1–7. [Google Scholar]
- Micula, S. An iterative numerical method for Fredholm–Volterra integral equations of the second kind. Appl. Math. Comput. 2015, 270, 935–942. [Google Scholar] [CrossRef]
- Erfanian, M. The approximate solution of nonlinear mixed Volterra-Fredholm-Hammerstein integral equations with RH wavelet bases in a complex plane. Math. Methods Appl. Sci. 2018, 41, 8942–8952. [Google Scholar] [CrossRef]
- Abdeljawad, T.; Agarwal, R.P.; Karapınar, E.; Kumari, P.S. Solutions of the Nonlinear Integral Equation and Fractional Differential Equation Using the Technique of a Fixed Point with a Numerical Experiment in Extended b-Metric Space. Symmetry 2019, 11, 686. [Google Scholar] [CrossRef]
- Berenguer, M.I.; Gámez, D. Study on convergence and error of a numerical method for solving systems of nonlinear Fredholm Volterra integral equations of Hammerstein type. Appl. Anal. 2017, 96, 516–527. [Google Scholar] [CrossRef]
- Berenguer, M.I.; Gámez, D.; Linares, A.J.L. Fixed point techniques and Schauder bases to approximate the solution of the first order nonlinear mixed Fredholm–Volterra integrodifferential equation. J. Comput. Appl. Math. 2013, 252, 52–61. [Google Scholar] [CrossRef]
- Geçmen, M.Z.; GeÇelik, E. Numerical solution of Volterra-Fredholm integral equa-tions with Hosoya polynomials. Math. Methods Appl. Sci. 2021, 44, 11166–11173. [Google Scholar] [CrossRef]
- Dahaghin, M.S.; Eskandari, S. Solving two-dimensional Volterra-Fredholm integral equations of the second kind by using Bernstein polynomials. Appl. Math. J. Chin. Univ. 2017, 32, 68–78. [Google Scholar] [CrossRef]
- Esmaeili, H.; Rostami, M.; Hooshyarbakhsh, V. Numerical solution of Volterra-Fredholm inte-gral equation via hyperbolic basis functions. Int. J. Numer. Model. 2021, 34, 1–11. [Google Scholar] [CrossRef]
- Xie, J.; Huang, Q.; Zhao, F. Numerical solution of nonlinear Volterra-Fredholm-Hammerstein integral equations in twodimensional spaces based on Block Pulse functions. J. Comput. Appl. Math. 2017, 317, 565–572. [Google Scholar] [CrossRef]
- Tomasiello, S.; Macías-Díaz, J.E.; Khastan, A.; Alijani, Z. New sinusoidal basis functions and a neural network approach to solve nonlinear Volterra-Fredholm integral equations. Neural Comput. Appl. 2019, 31, 4865–4878. [Google Scholar] [CrossRef]
- Gouyandeh, Z.; Allahviranloo, T.; Armand, A. Numerical solution of nonlinear Volter-ra-Fredholm-Hammerstein integral equations via Tau-collocation method with convergence analysis. J. Comput. Appl. Math. 2016, 308, 435–446. [Google Scholar] [CrossRef]
- Ninh, K.V.; Binh, N.T. Analytical Solution of Volterra-Fredholm Integral Equations Using Hybrid of the Method of Contractive Mapping and Parameter Continuation Method. Int. J. Appl. Comput. Math. 2019, 5, 76. [Google Scholar] [CrossRef]
- Dobriţoiu, M. The Existence and Uniqueness of the Solution of a Nonlinear Fredholm–Volterra Integral Equation with Modified Argument via Geraghty Contractions. Mathematics 2021, 9, 29. [Google Scholar] [CrossRef]
- Nasr, M.E.; Abdel–Aty, M.A. Analytical discussion for the mixed integral equations. J. Fixed Point Theory Appl. 2018, 20, 115. [Google Scholar] [CrossRef]
- Atkinson, K.E. The Numerical Solution of Integral Equation of the Second Kind, Cambridge Monographs on Applied and Computational Mathematics; Cambridge University Press: Cambridge, NY, USA, 1997. [Google Scholar]
- Abdel-Aty, M.A.; Abdou, M.A.; Soliman, A.A. Solvability of quadratic integral equations with singular kernel. J. Contemp. Math. Anal. 2022, 57, 12–25. [Google Scholar] [CrossRef]
- Aleksandrovsk, V.M.; Covalence, E.V. Problems in the Mechanics of Continuous Media with Mixed Boundary Conditions; Nuka: Moscow, Russia, 1986. [Google Scholar]
- Gradstein, I.S.; Ryzhik, I.M. Tables of Integrals, 5th ed.; Series and Product; Academic Press: New York, NY, USA, 1994. [Google Scholar]
- Shoukralla, E.S. Application of Chebyshev polynomials of the second kind to the numerical solution of weakly singular Fredholm integral equations of the first kind. IAENG Int. J. Appl. Math. 2021, 51, 1–16. [Google Scholar]
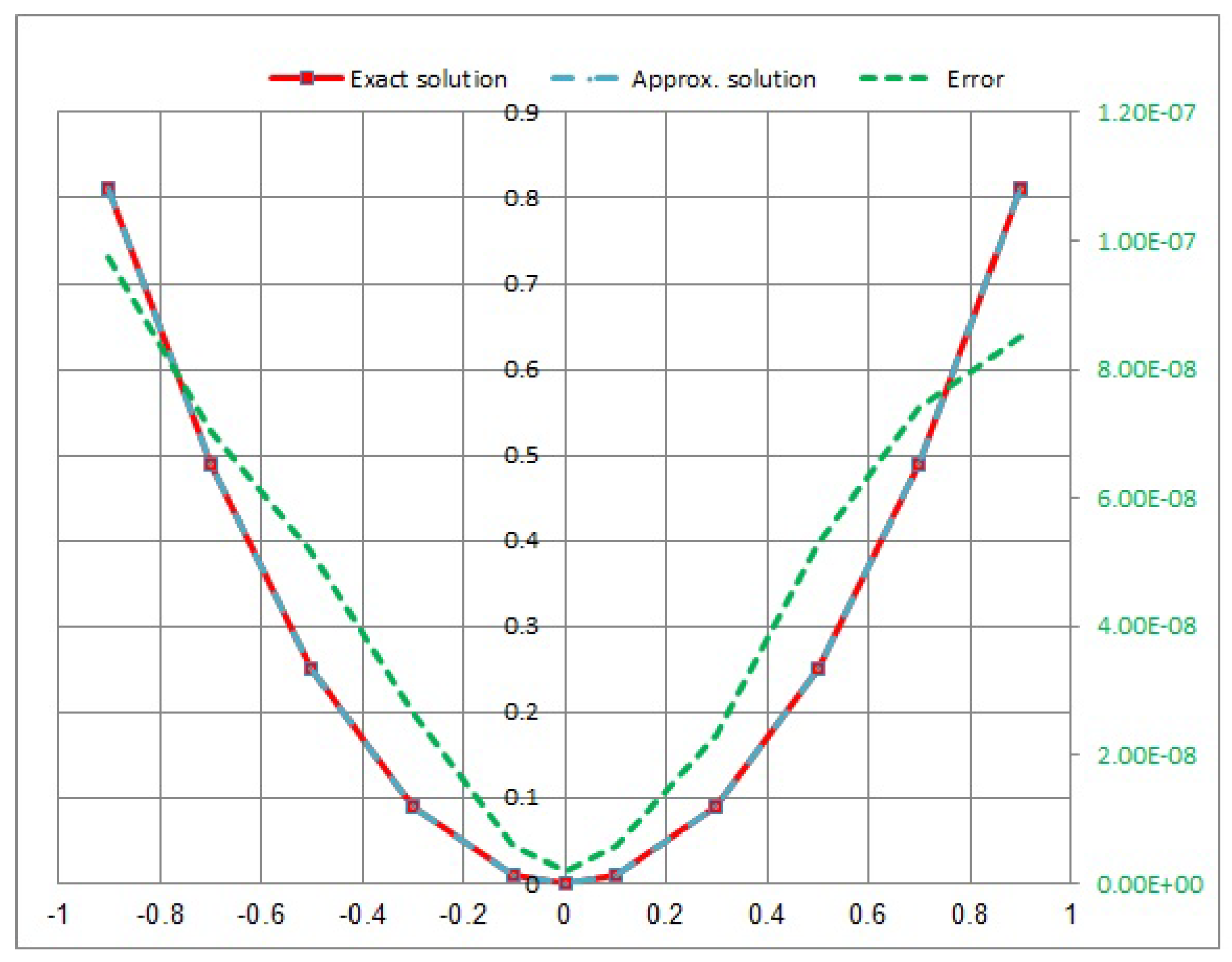
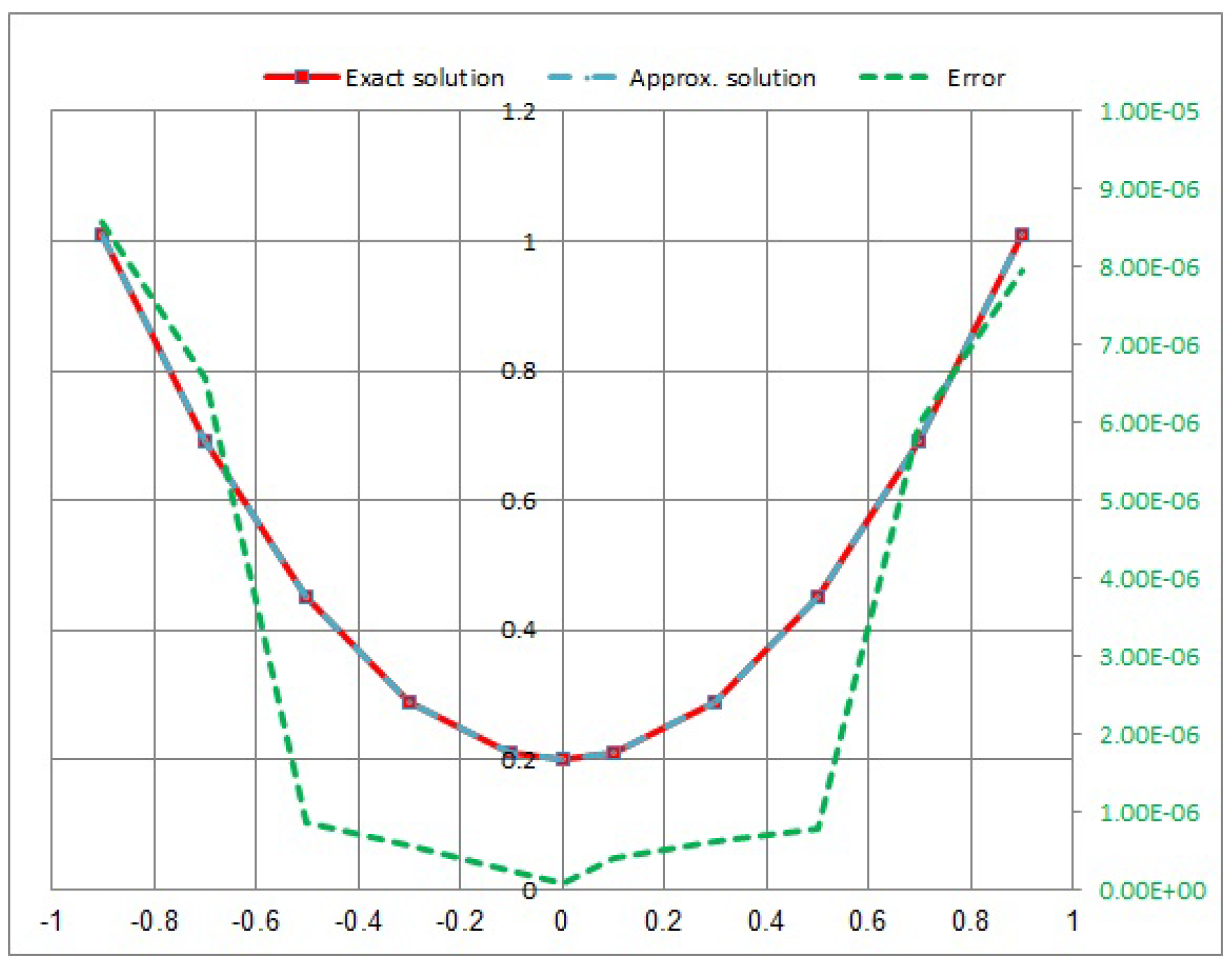
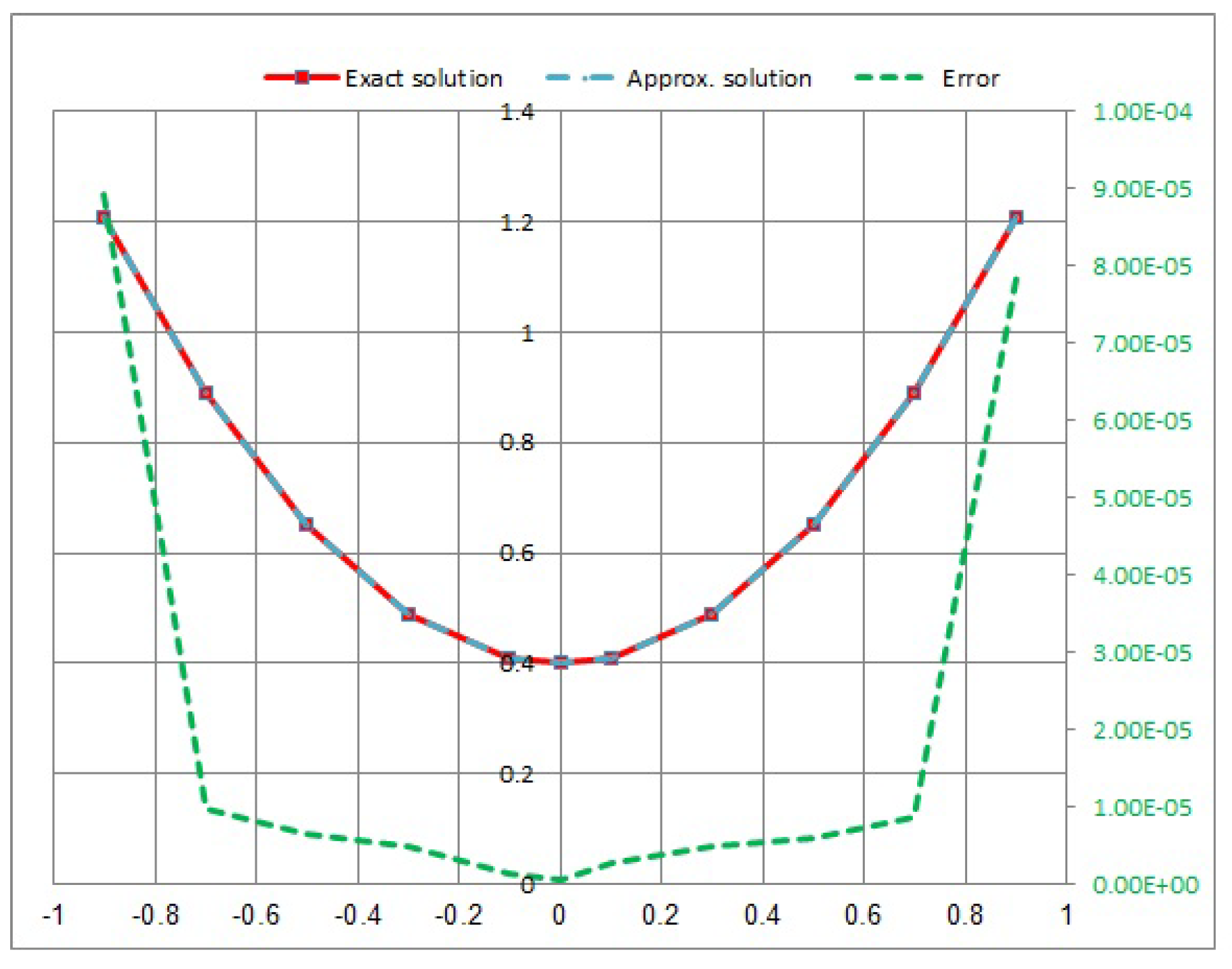
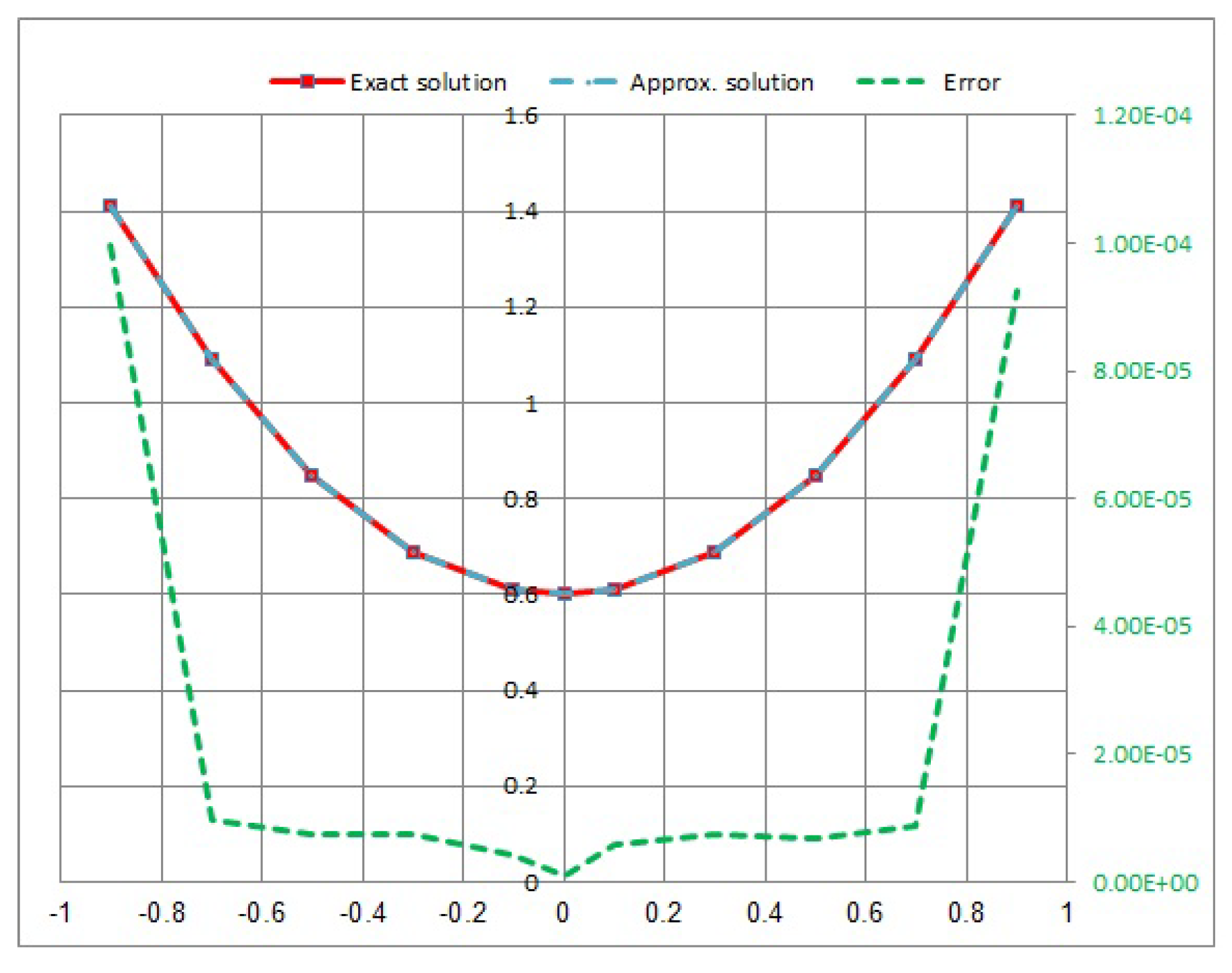

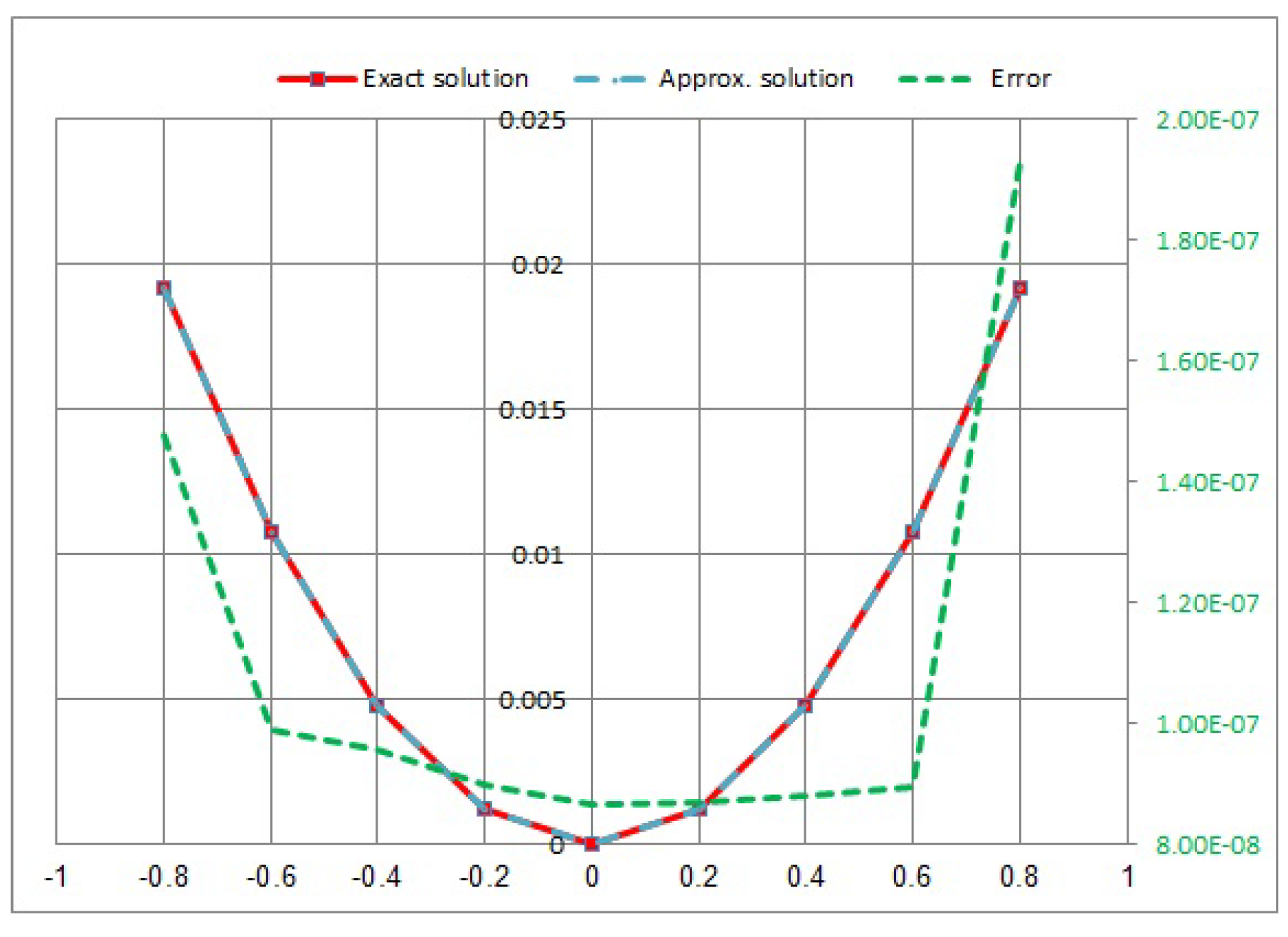
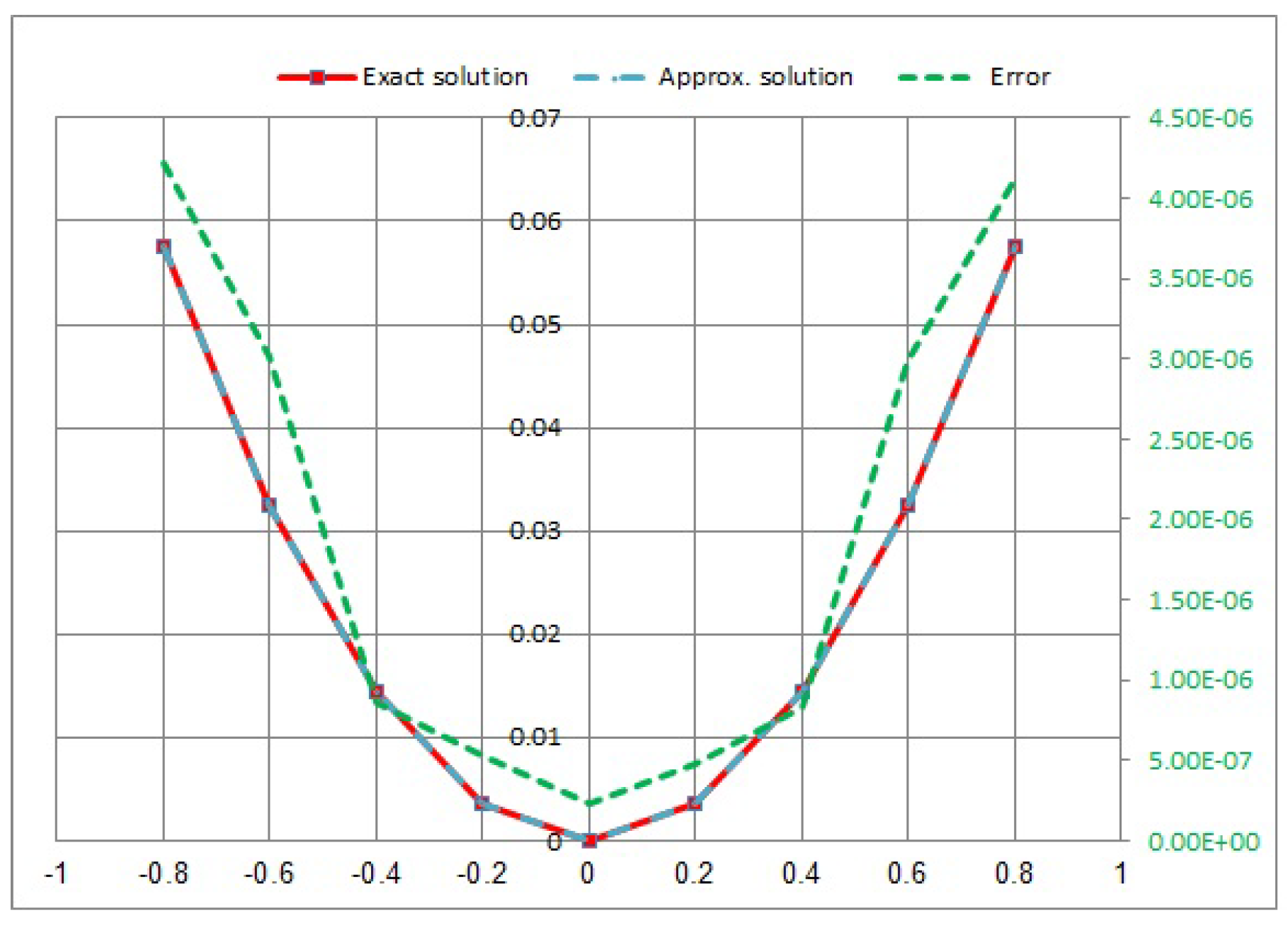

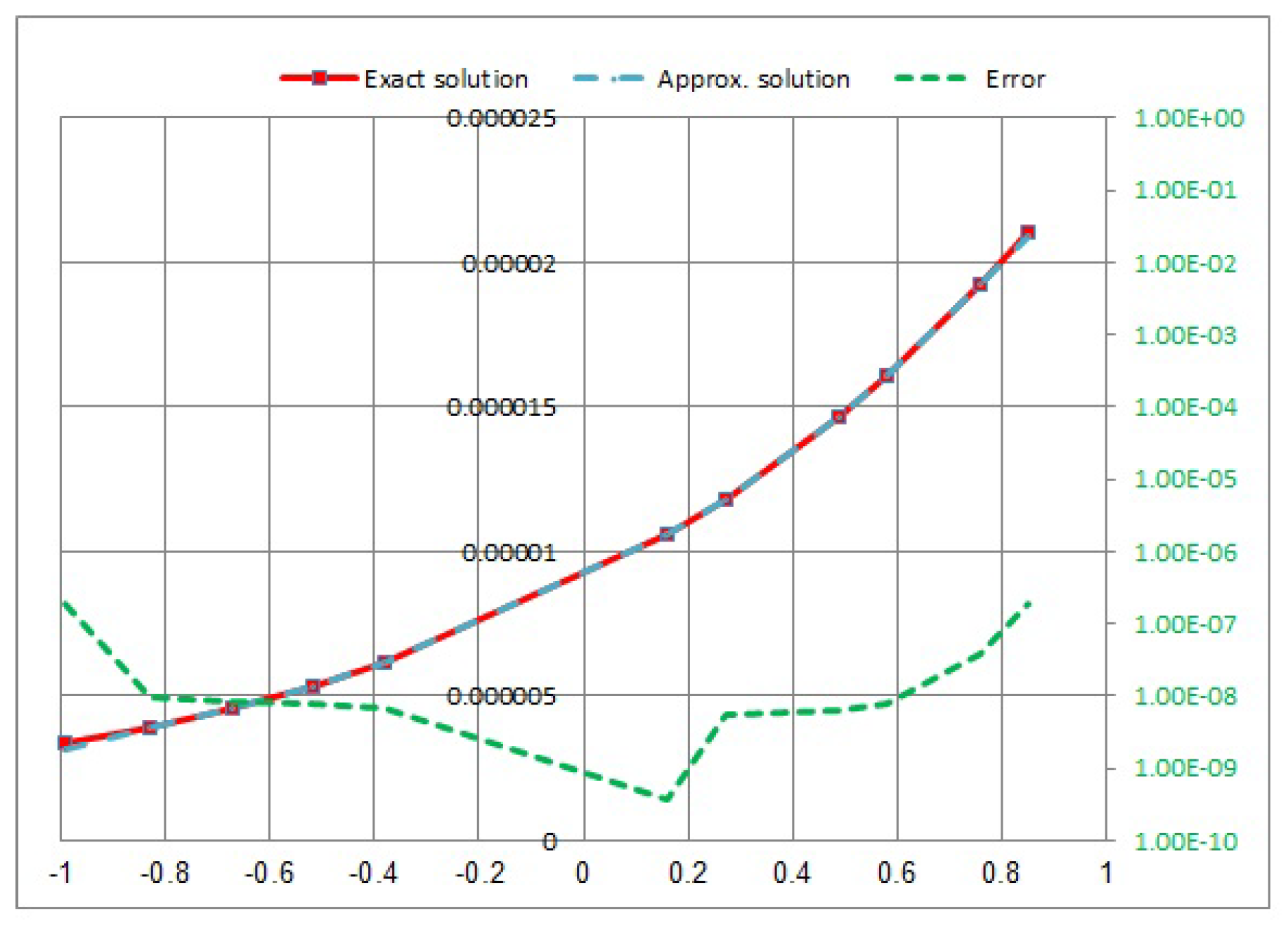
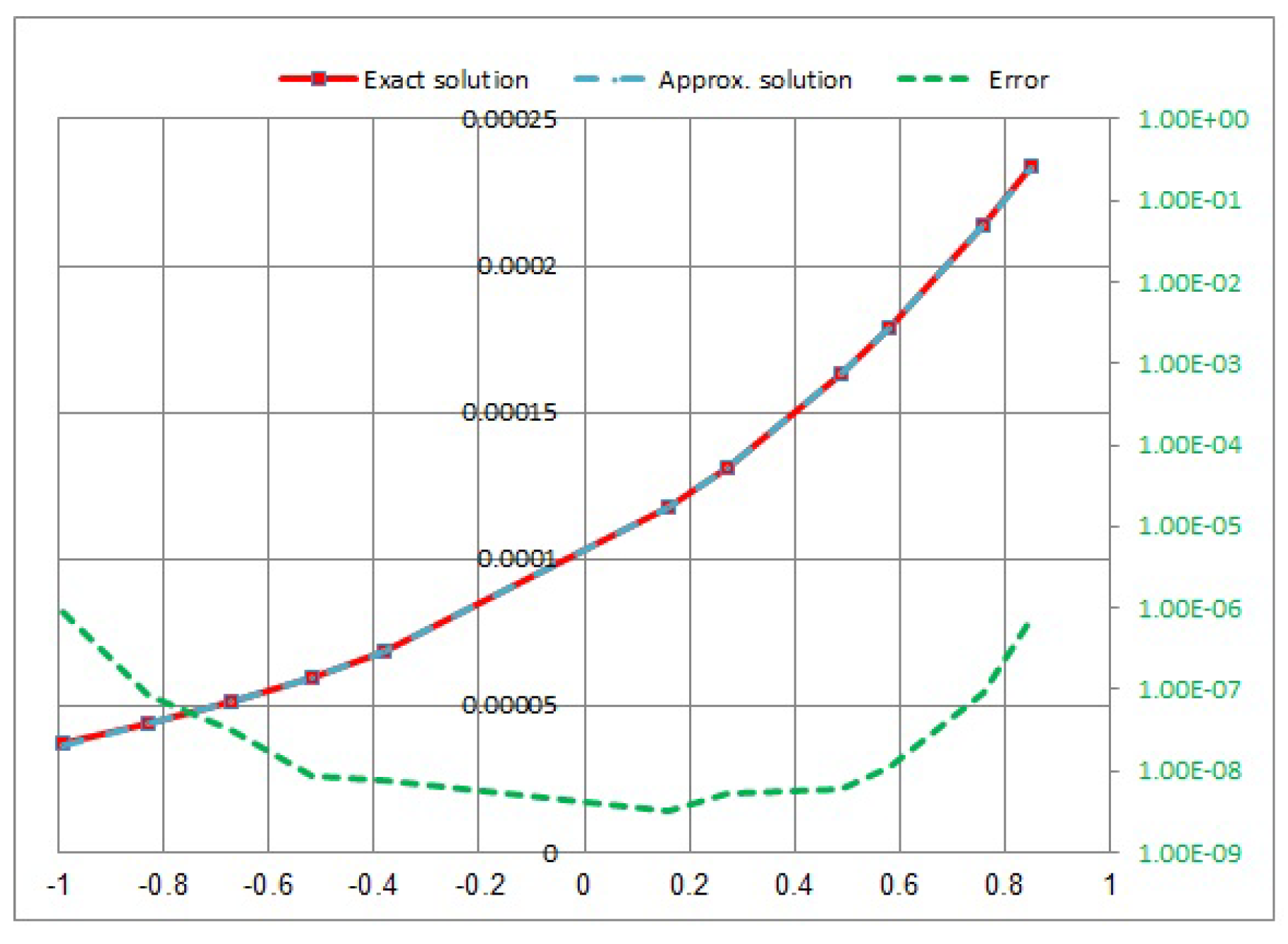
| u | |Exact-Approx.| | |Exact-Approx.| | |Exact-Approx.| | |Exact-Approx.| |
|---|---|---|---|---|
| −0.9 | 9.75485 | 8.57458 | 8.92185 | 9.96543 |
| −0.7 | 7.03693 | 6.58423 | 9.63241 | 9.86545 |
| −0.5 | 5.61475 | 8.52387 | 6.39556 | 7.51273 |
| −0.3 | 2.46587 | 5.26217 | 4.78569 | 7.42428 |
| −0.1 | 5.75813 | 2.93561 | 1.25865 | 4.23171 |
| 0 | 1.75434 | 8.56468 | 5.64758 | 8.47585 |
| 0.1 | 5.89767 | 3.89657 | 2.58769 | 5.87418 |
| 0.3 | 2.53144 | 6.03217 | 4.78557 | 7.52431 |
| 0.5 | 5.22873 | 7.84574 | 5.63264 | 6.85768 |
| 0.7 | 7.41562 | 5.98784 | 8.54282 | 8.85441 |
| 0.9 | 8.52129 | 7.96546 | 7.85668 | 9.24574 |
| u | Error of | Error of | Error of | Error of |
|---|---|---|---|---|
| −0.8 | 4.26831 | 1.47857 | 4.21364 | 8.96321 |
| −0.6 | 2.58214 | 9.89765 | 3.02146 | 7.52301 |
| −0.4 | 9.20135 | 9.56212 | 8.63254 | 6.92541 |
| −0.2 | 7.41234 | 8.96326 | 5.32874 | 7.15246 |
| 0 | 5.64123 | 8.64129 | 2.36581 | 2.63214 |
| 0.2 | 7.08745 | 8.69546 | 4.82031 | 6.30254 |
| 0.4 | 7.41233 | 8.80231 | 8.36521 | 6.12543 |
| 0.6 | 3.25647 | 8.93541 | 2.98652 | 7.45213 |
| 0.8 | 4.10258 | 1.93254 | 4.12984 | 8.12984 |
| u | Error in | Error in | Error in | Error in |
|---|---|---|---|---|
| 1.85746 | 8.76325 | 8.83697 | 6.32154 | |
| −0.83 | 9.47854 | 8.52365 | 7.41235 | 6.93254 |
| −0.67 | 8.61543 | 3.25483 | 5.23147 | 6.32154 |
| −0.52 | 7.64423 | 8.45821 | 4.36258 | 5.62147 |
| −0.38 | 6.58477 | 7.52314 | 3.56214 | 3.26984 |
| 0.16 | 3.65215 | 3.12754 | 6.12453 | 5.63021 |
| 0.27 | 5.69821 | 5.29475 | 3.56214 | 3.14752 |
| 0.49 | 6.32154 | 5.96872 | 4.21235 | 4.96235 |
| 0.58 | 7.92315 | 1.12548 | 5.14258 | 6.23154 |
| 0.76 | 3.95426 | 9.15347 | 6.32514 | 6.63215 |
| 0.85 | 1.85465 | 7.58479 | 7.81548 | 6.14789 |
| u | Approx.| | Approx.| | Approx.| | Approx.| | Approx.| | Approx.| |
|---|---|---|---|---|---|---|
| −1.00 | 0 | 0.01 | 0.02 | 0.03 | 0.0400001 | 0.0500002 |
| −0.95 | 0 | 0.0095 | 0.019 | 0.0285 | 0.038 | 0.0475001 |
| −0.71 | 0 | 0.0071 | 0.0142 | 0.0213 | 0.0284 | 0.0355001 |
| −0.54 | 0 | 0.0054 | 0.0108 | 0.0162 | 0.0216 | 0.027 |
| −0.38 | 0 | 0.0038 | 0.0076 | 0.0114 | 0.0152 | 0.019 |
| 0.00 | 0 | 0 | 0 | 0 | 0 | 0 |
| 0.26 | 0 | 0.0026 | 0.0052 | 0.0078 | 0.0104 | 0.013 |
| 0.43 | 0 | 0.0043 | 0.0086 | 0.0129 | 0.0172 | 0.0215 |
| 0.65 | 0 | 0.0065 | 0.013 | 0.0195 | 0.026 | 0.0325001 |
| 0.86 | 0 | 0.0086 | 0.0172 | 0.0258 | 0.0344 | 0.0430001 |
| 0.99 | 0 | 0.0099 | 0.0198 | 0.0297 | 0.0396001 | 0.0495002 |
| Error in | Error in | Error in | Error in | |
| −1.0 | 5.15478 | 7.02587 | 3.69851 | 7.58742 |
| −0.9 | 4.63897 | 6.98521 | 2.36823 | 6.74185 |
| −0.7 | 2.85217 | 6.52147 | 1.50698 | 3.96587 |
| −0.5 | 1.87456 | 5.19854 | 0.85236 | 1.25874 |
| −0.3 | 0.64423 | 3.02587 | 8.08574 | 0.91114 |
| −0.1 | 4.85214 | 6.32541 | 6.63242 | 7.98524 |
| 0.0 | 2.58746 | 4.36985 | 5.36985 | 8.21475 |
| 0.2 | 5.68712 | 6.39478 | 7.36985 | 8.52147 |
| 0.4 | 0.77632 | 3.65287 | 9.02584 | 9.51236 |
| 0.6 | 2.98571 | 5.41721 | 9.98526 | 2.36985 |
| 0.8 | 3.25874 | 6.39852 | 1.96358 | 5.36575 |
| 1.0 | 5.08745 | 7.21456 | 3.69852 | 7.63988 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Abusalim, S.M.; Abdou, M.A.; Nasr, M.E.; Abdel-Aty, M.A. An Algorithm for the Solution of Nonlinear Volterra–Fredholm Integral Equations with a Singular Kernel. Fractal Fract. 2023, 7, 730. https://doi.org/10.3390/fractalfract7100730
Abusalim SM, Abdou MA, Nasr ME, Abdel-Aty MA. An Algorithm for the Solution of Nonlinear Volterra–Fredholm Integral Equations with a Singular Kernel. Fractal and Fractional. 2023; 7(10):730. https://doi.org/10.3390/fractalfract7100730
Chicago/Turabian StyleAbusalim, Sahar M., Mohamed A. Abdou, Mohamed E. Nasr, and Mohamed A. Abdel-Aty. 2023. "An Algorithm for the Solution of Nonlinear Volterra–Fredholm Integral Equations with a Singular Kernel" Fractal and Fractional 7, no. 10: 730. https://doi.org/10.3390/fractalfract7100730
APA StyleAbusalim, S. M., Abdou, M. A., Nasr, M. E., & Abdel-Aty, M. A. (2023). An Algorithm for the Solution of Nonlinear Volterra–Fredholm Integral Equations with a Singular Kernel. Fractal and Fractional, 7(10), 730. https://doi.org/10.3390/fractalfract7100730









