Petviashvili Method for the Fractional Schrödinger Equation
Abstract
1. Introduction
2. Materials and Methods
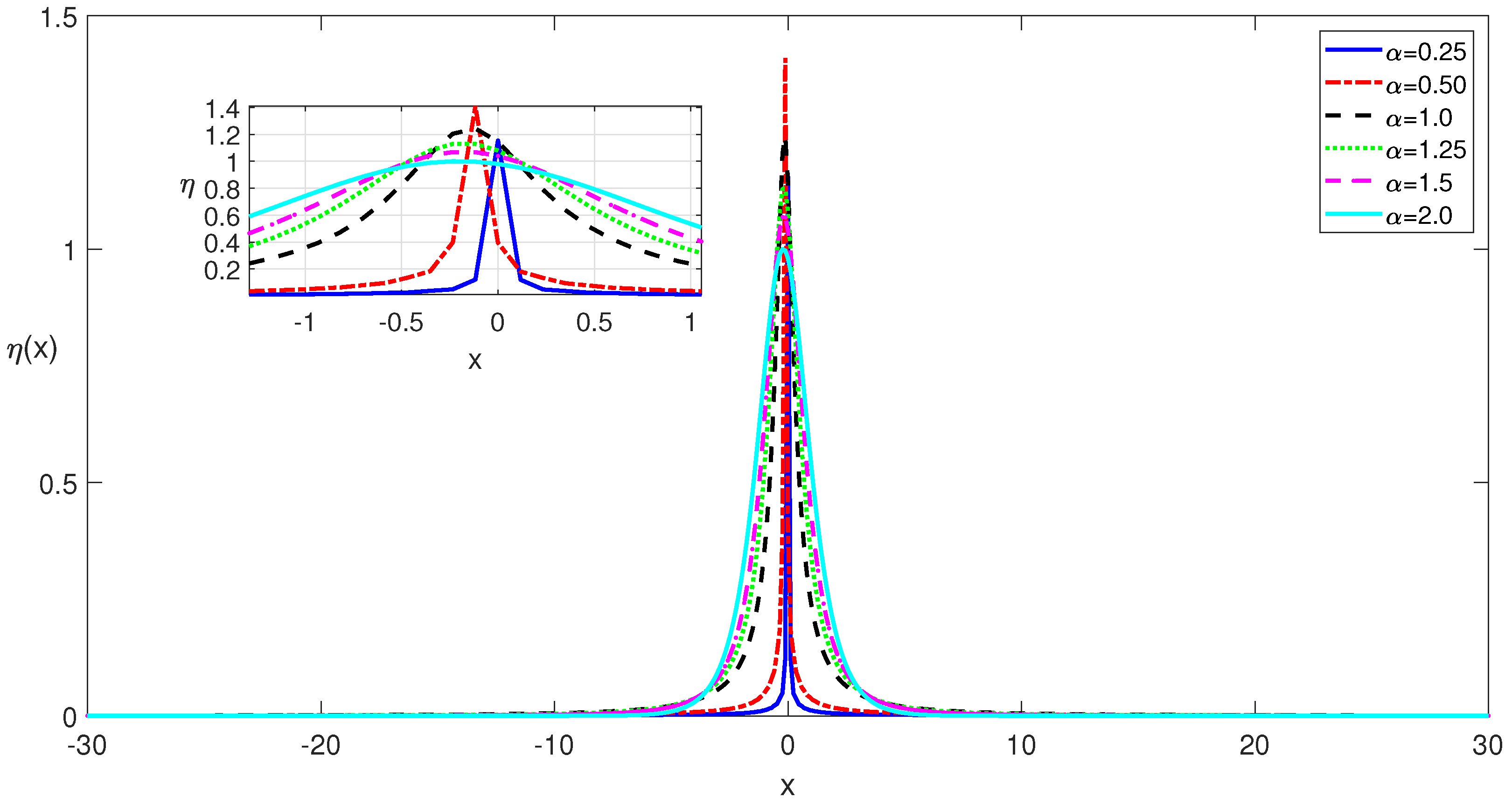
Mathematical Formulation of the Petviashvili’s Method for the Solution of the Fractional Nonlinear Schrödinger Equation
3. Results and Discussion
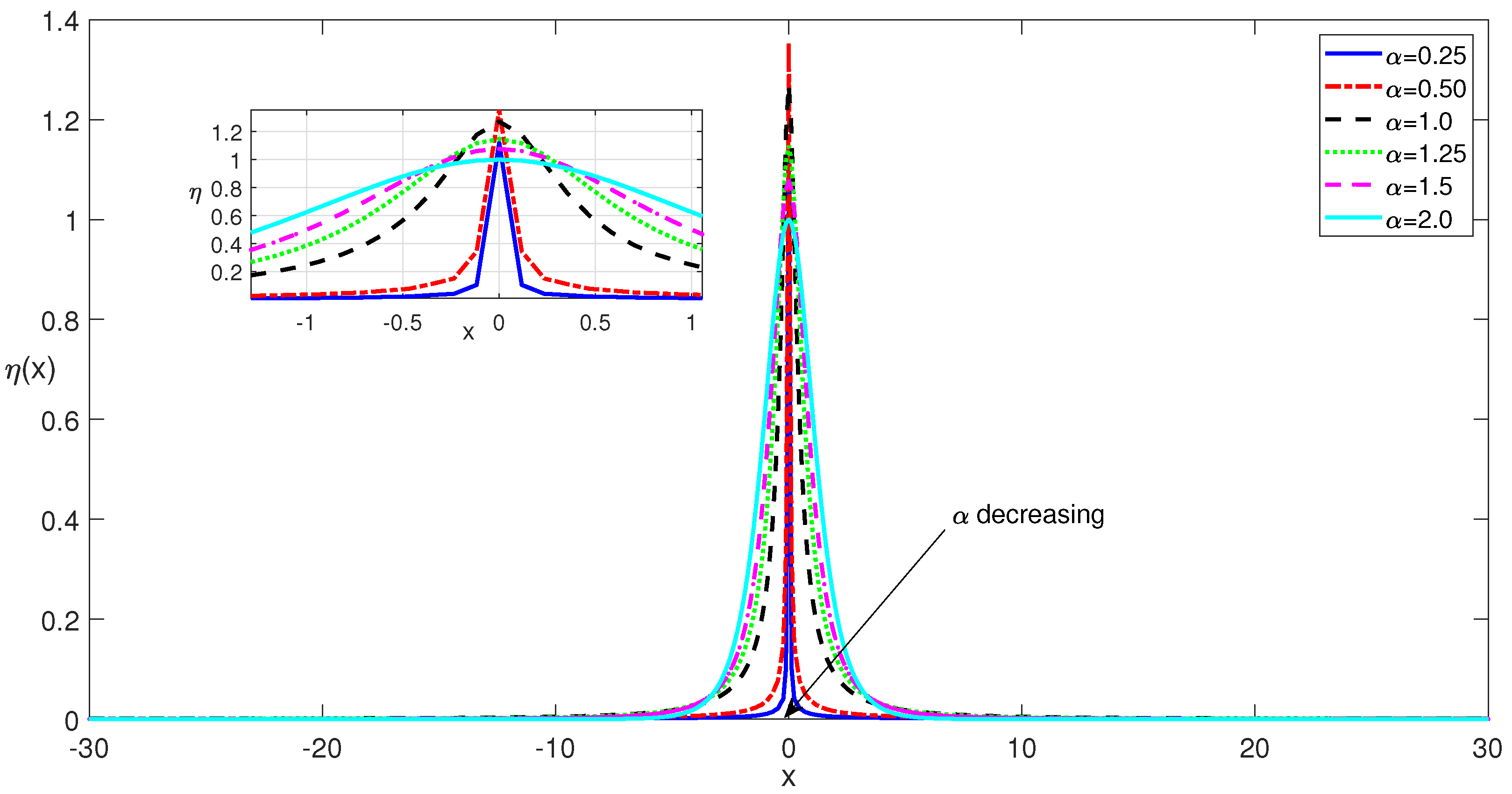
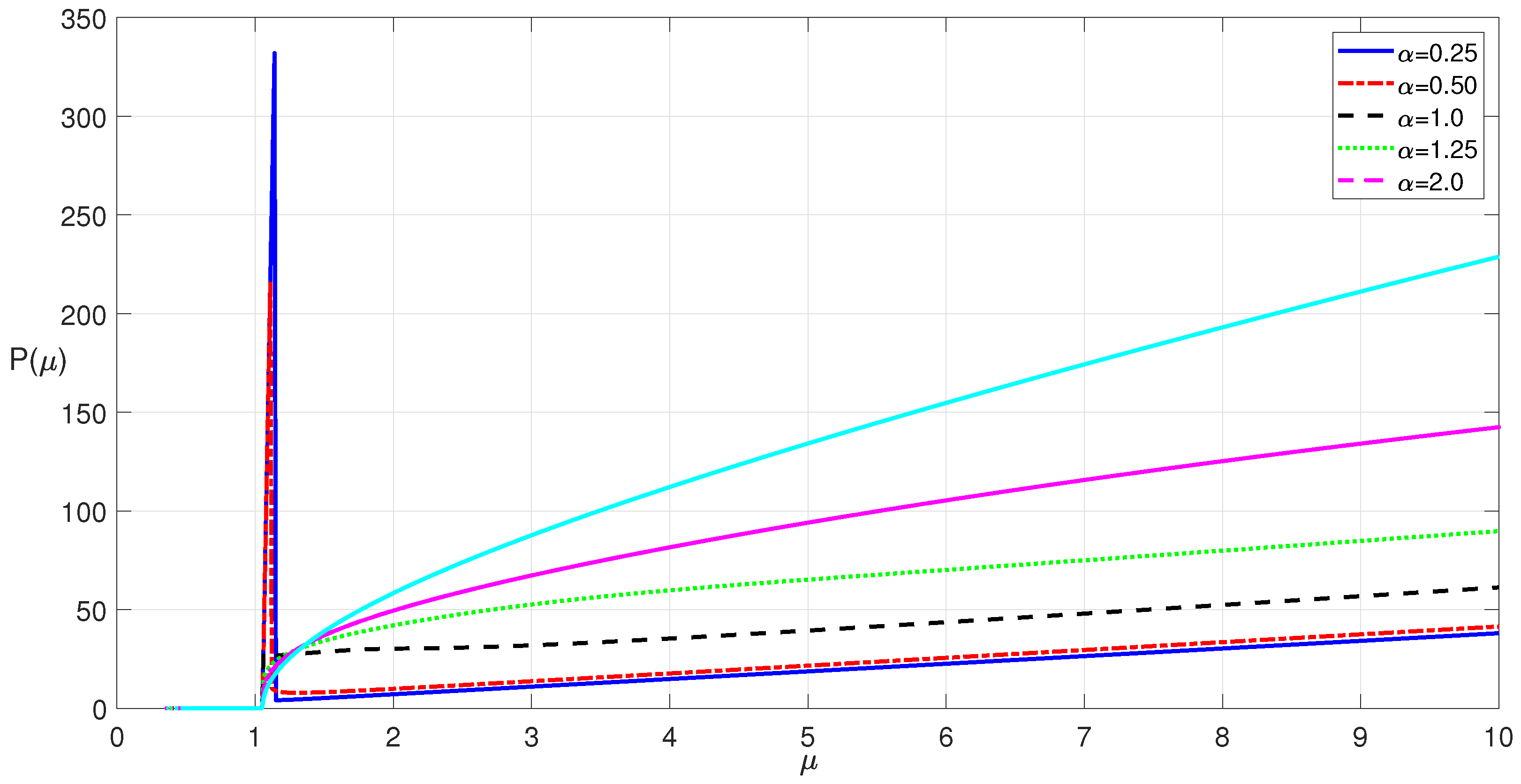
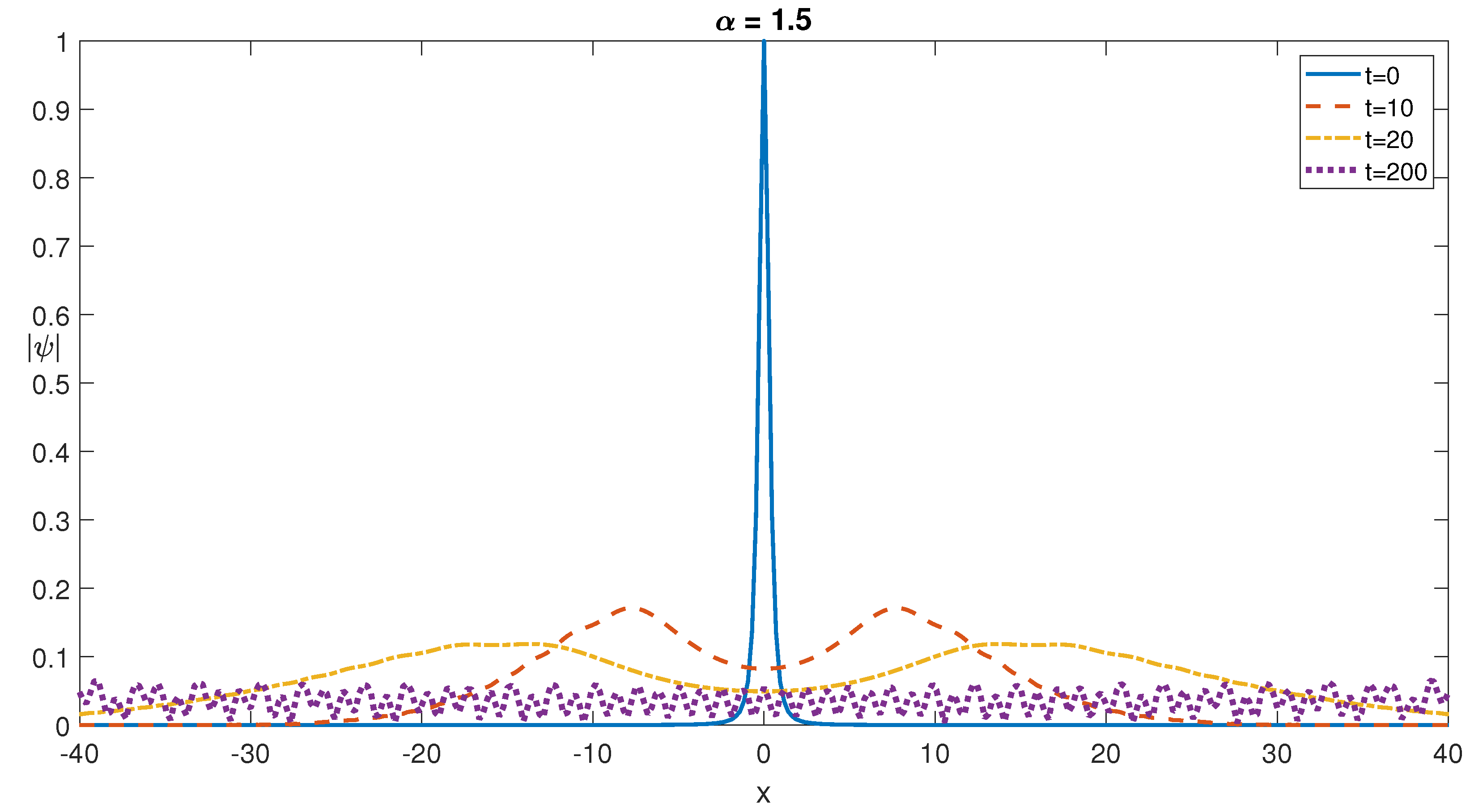
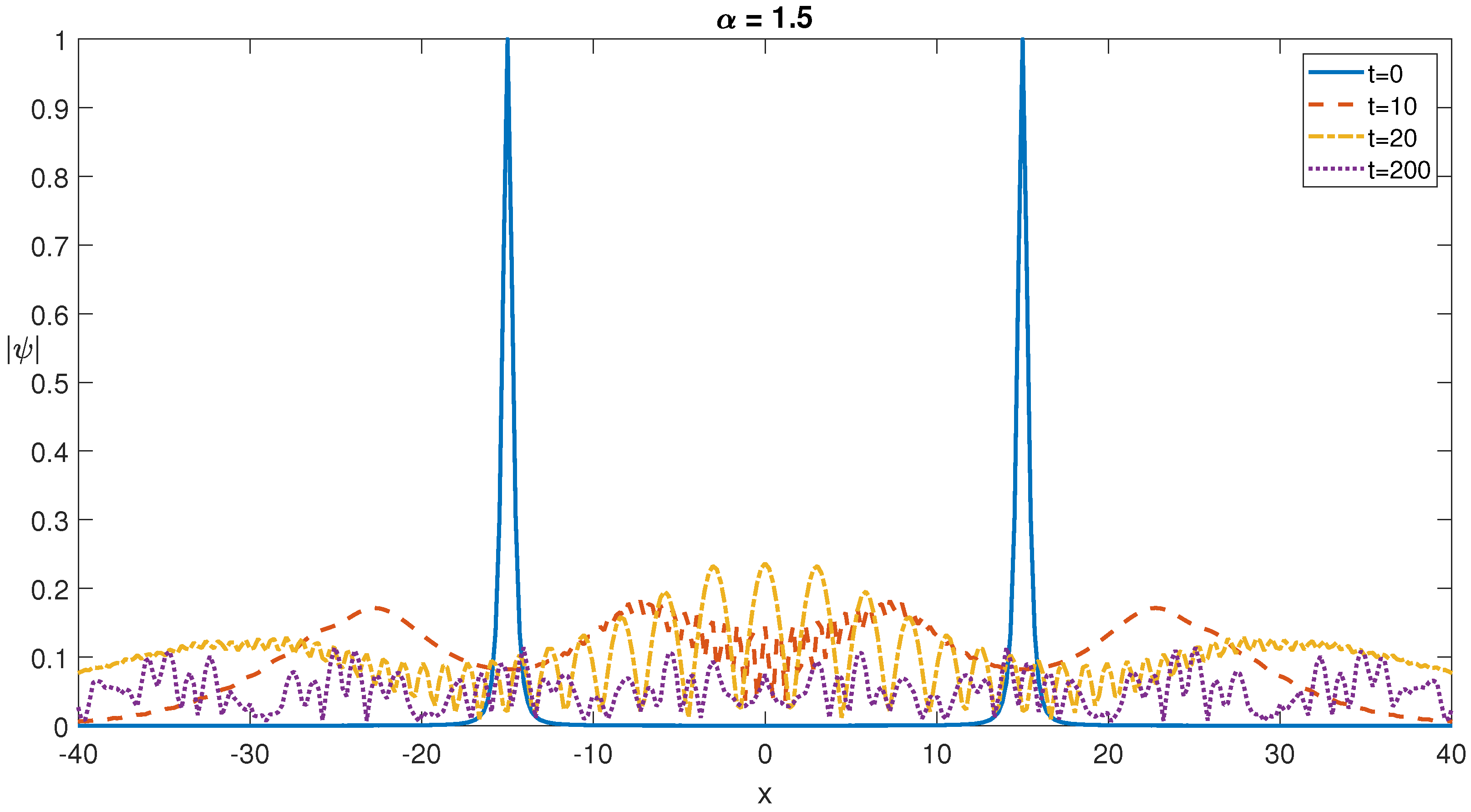
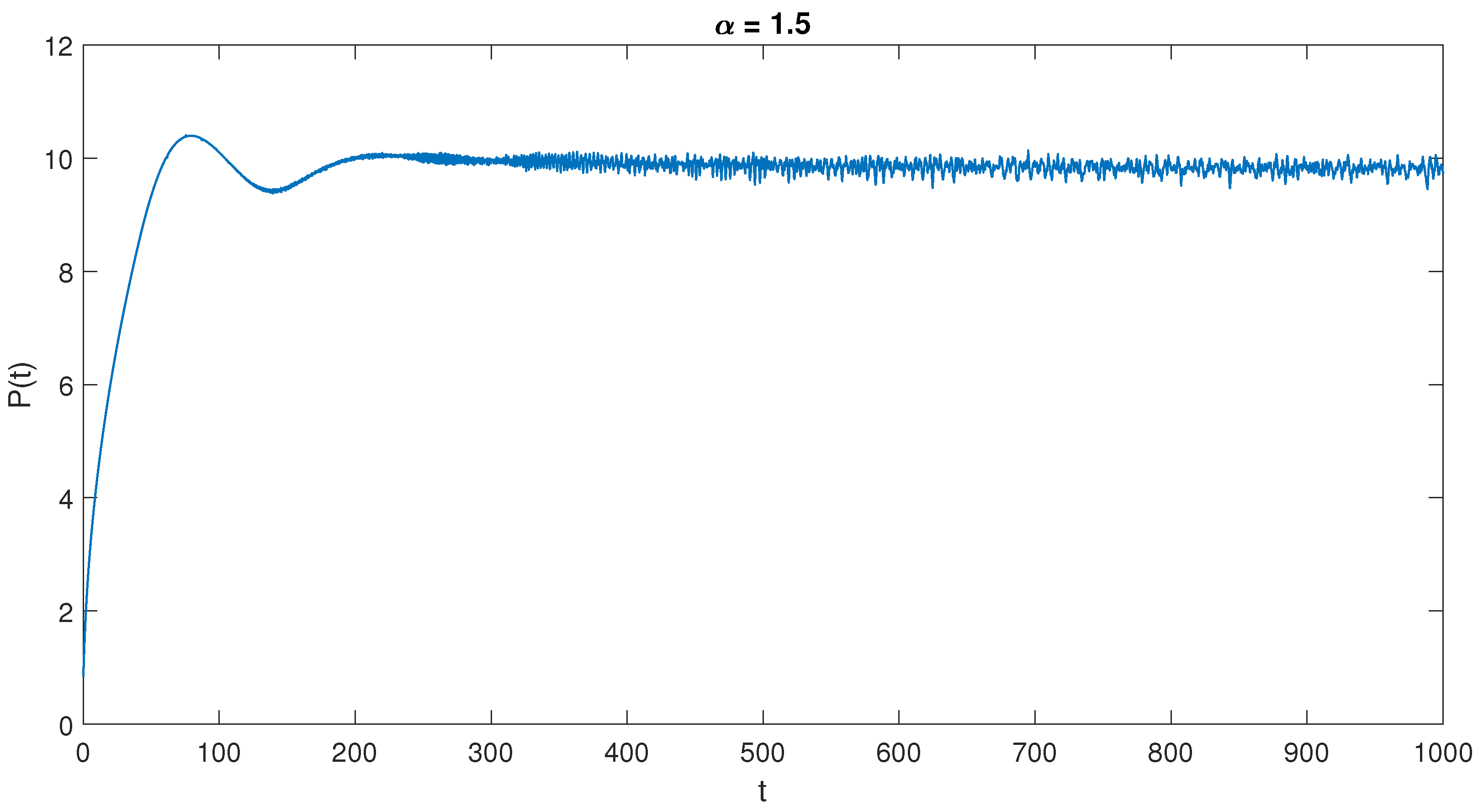
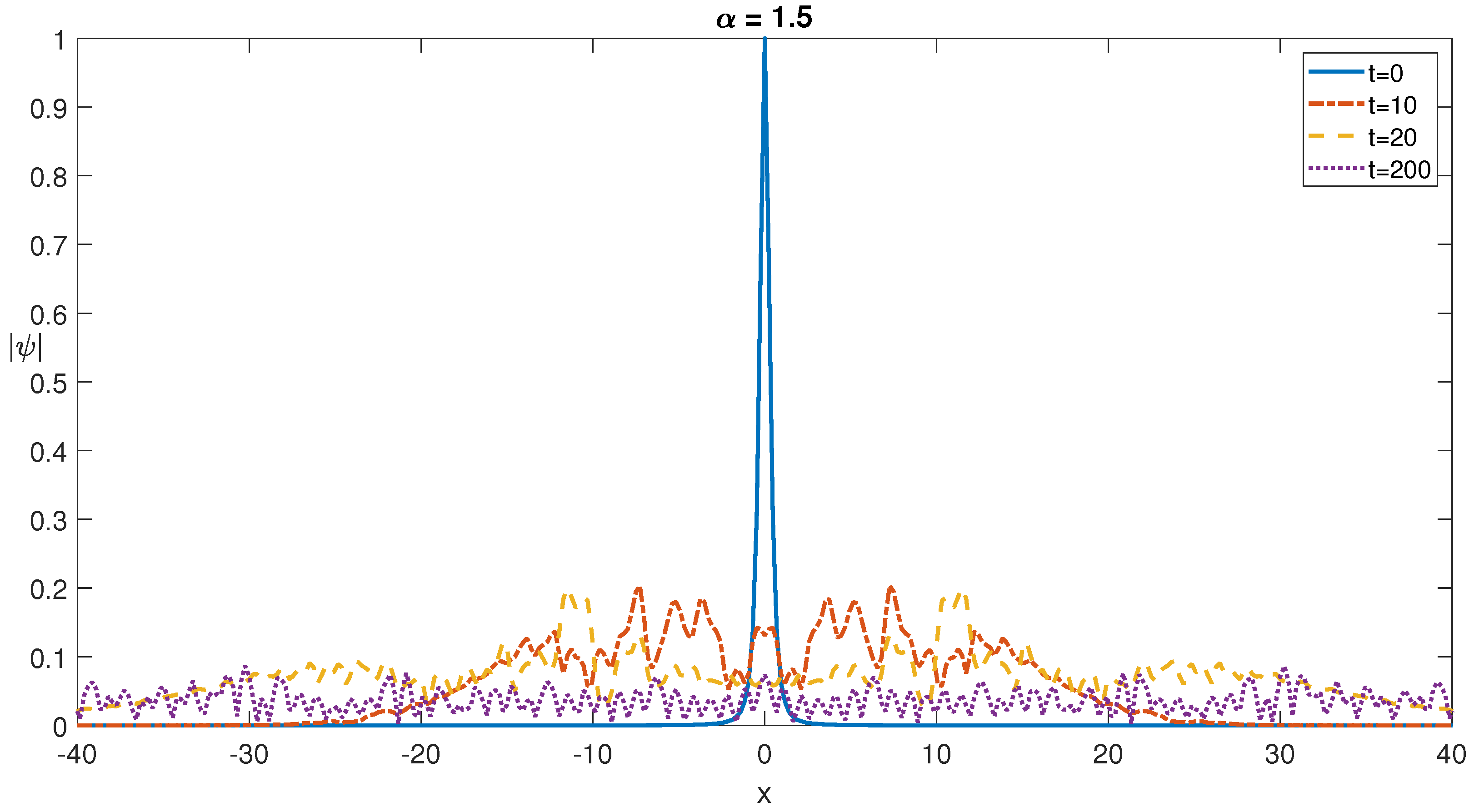
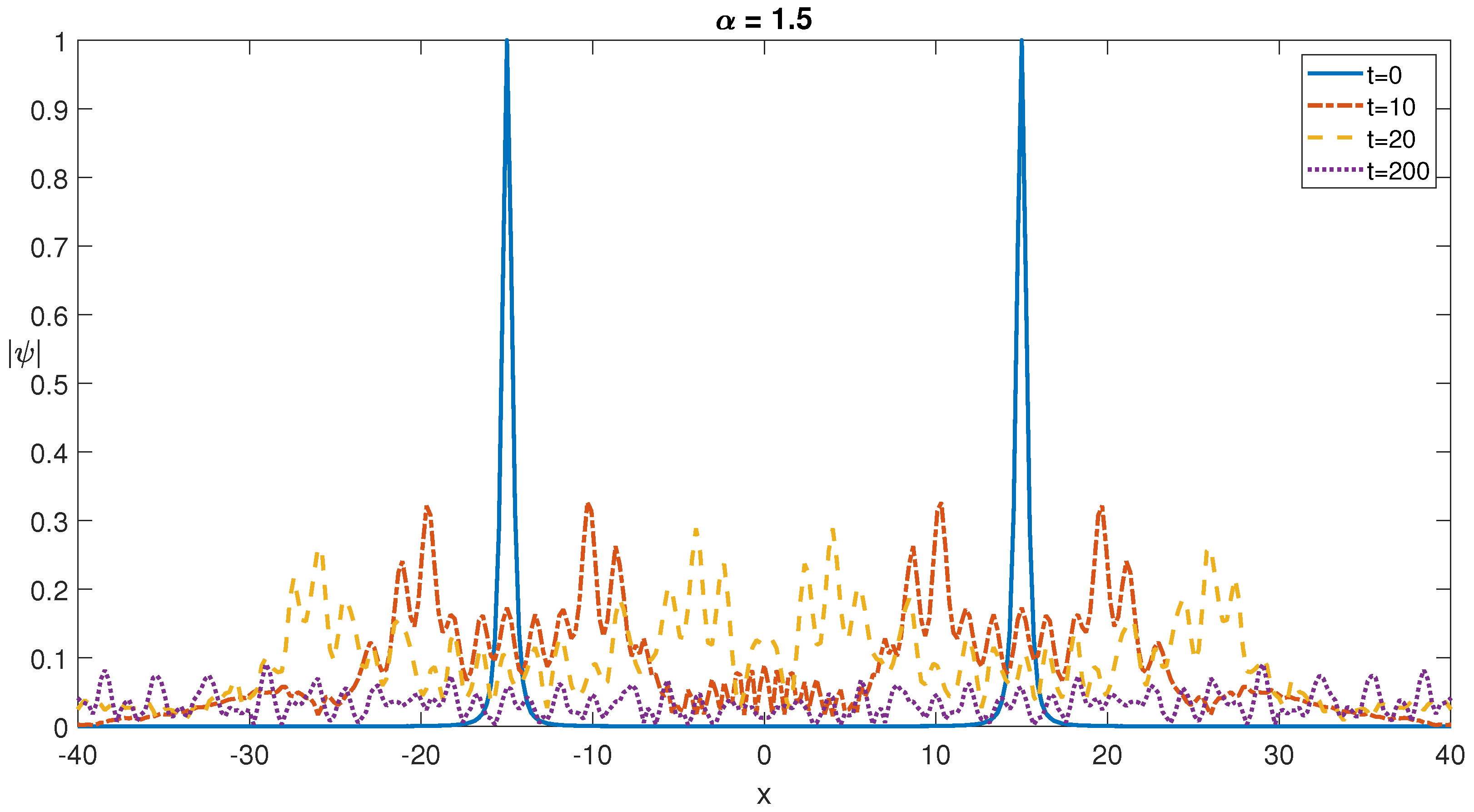
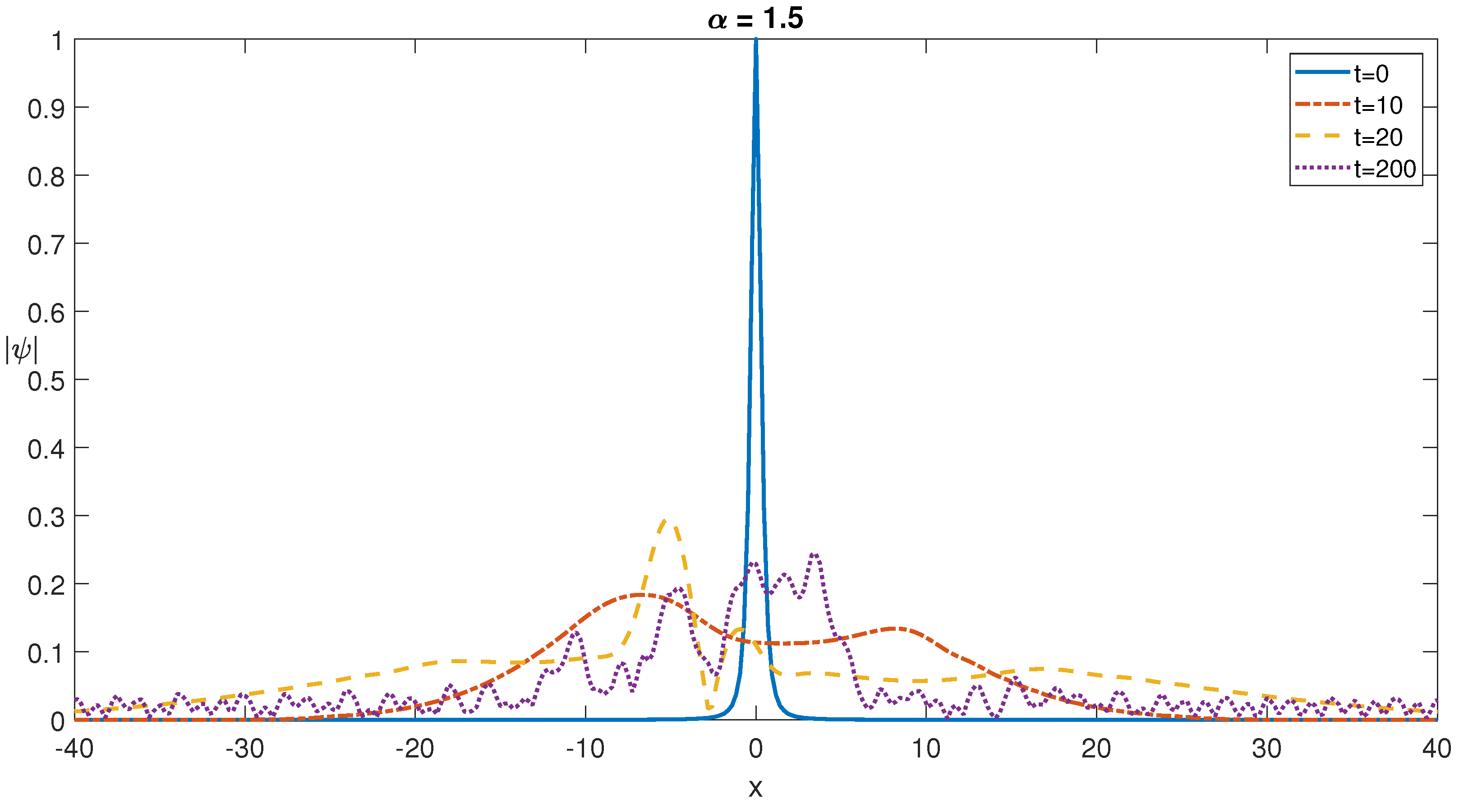
3.1. Results for Zero Potential,
3.2. Results for Photorefractive Potential,
3.3. Results for q-Deformed Rosen-Morse Potential,
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| PM | Petviashvili method |
| fNLSE | fractional nonlinear Schrödinger equation |
| KP | Kadomtsev-Petviashvili |
| SRM | spectral renormalization method |
| CSRM | compressive spectral renormalization method |
References
- Laskin, N. Fractional Schrödinger equation. Phys. Rev. E 2002, 66, 056108. [Google Scholar] [CrossRef] [PubMed]
- Fei, M.; Zhang, G.; Wang, N.; Huang, C. A linearized conservative Galerkin–Legendre spectral method for the strongly coupled nonlinear fractional Schrödinger equations. Adv. Differ. Equ. 2020, 2020, 661. [Google Scholar] [CrossRef]
- Shao, J.; Guo, B. The Cauchy problem for Schrödinger-damped Boussinesq system. J. Math. Anal. Appl. 2021, 494, 124639. [Google Scholar] [CrossRef]
- Chen, P.; Liu, X. Ground states for asymptotically linear fractional Schrödinger–Poisson systems. J. -Pseudo-Differ. Oper. Appl. 2021, 12, 8. [Google Scholar] [CrossRef]
- Gu, G.; Tang, X.; Shen, J. Multiple solutions for fractional Schrödinger–Poisson system with critical or supercritical nonlinearity. Appl. Math. Lett. 2021, 111, 106605. [Google Scholar] [CrossRef]
- An, X.; Lipeng, D.; Peng, Y. Semi-classical analysis for fractional Schrödinger equations with fast decaying potentials. Appl. Anal. 2021, 101, 5138–5155. [Google Scholar] [CrossRef]
- Jamshir, N.; Lari, B.; Hassanabadi, H. The time independent fractional Schrödinger equation with position-dependent mass. Phys. A Stat. Mech. Appl. 2021, 565, 125616. [Google Scholar] [CrossRef]
- Alouini, B. Finite dimensional global attractor for a fractional Schrödinger type equation with mixed anisotropic dispersion. J. Dyn. Differ. Equ. 2022, 34, 1237–1268. [Google Scholar] [CrossRef]
- Liemert, A.; Kienle, A. Fractional Schrödinger equation in the presence of the linear potential. Mathematics 2016, 4, 31. [Google Scholar] [CrossRef]
- Petviashvili, V.I. Equation of an extraordinary soliton. Fiz. Plazmy 1976, 2, 469–472. [Google Scholar]
- Ablowitz, M.J.; Musslimani, Z.H. Spectral renormalization method for computing self-localized solutions to nonlinear systems. Opt. Lett. 2005, 30, 2140–2142. [Google Scholar] [CrossRef] [PubMed]
- Bonatsos, D.; Lenis, D.; Raychev, P.; Terziev, P. Deformed harmonic oscillators for metal clusters: Analytic properties and supershells. Phys. Rev. A 2002, 65, 033203. [Google Scholar] [CrossRef]
- Altintas, A.A.; Arik, M.; Arikan, A.S.; Dil, E. Inhomogeneous quantum invariance group of multi-dimensional multi-parameter deformed boson algebra. Chin. Phys. Lett. 2012, 29, 010203. [Google Scholar] [CrossRef]
- Molaee, Z.; Ghominejad, M.; Hassanabadi, H.; Zarrinkamar, S. S-wave solutions of spin-one DKP equation for a deformed Hulthén potential in (1+ 3) dimensions. Eur. Phys. J. Plus 2012, 127, 116. [Google Scholar] [CrossRef]
- Altintas, A.A.; Ozaydin, F.; Yesilyurt, C.; Bugu, S.; Arik, M. Constructing quantum logic gates using q-deformed harmonic oscillator algebras. Quantum Inf. Process. 2014, 13, 1035–1044. [Google Scholar] [CrossRef]
- Altintas, A.A.; Ozaydin, F.; Bayındır, C. q-Deformed three-level quantum logic. Quantum Inf. Process. 2020, 19, 247. [Google Scholar] [CrossRef]
- Hasegawa, H. Quantum Fisher Information and q-Deformed Relative Entropies: Additivity vs Nonadditivity. Prog. Theor. Phys. 2006, 162, 183–189. [Google Scholar] [CrossRef][Green Version]
- Güvenilir, E.; Ozaydin, F.; Müstecaplıoğlu, Ö.E.; Hakioğlu, T. Work harvesting by q-deformed statistical mutations in an Otto engine. arXiv 2022, arXiv:2208.08565. [Google Scholar]
- Kwaśnicki, M. Ten Equivalent Definitions of the Fractional Laplace Operator. Fract. Calc. Appl. Anal. 2017, 20, 7–51. [Google Scholar] [CrossRef]
- Bayındır, C. Rogue quantum harmonic oscillations. Phys. A Stat. Mech. Appl. 2020, 547, 124462. [Google Scholar] [CrossRef]
- Kivshar, Y.S.; Alexander, T.J.; Turitsyn, S.K. Nonlinear modes of a macroscopic quantum oscillator. Phys. Lett. A 2001, 278, 225–230. [Google Scholar] [CrossRef]
- Bayındır, C.; Ozaydin, F. Freezing optical rogue waves by Zeno dynamics. Opt. Commun. 2018, 413, 141–146. [Google Scholar] [CrossRef]
- Bayındır, C.; Ozaydin, F. Self-localized soliton solutions of the nonlinear quantum harmonic oscillator. TWMS J. Appl. Eng. Math. 2022, 12, 412. [Google Scholar]
- Wang, Y.; Hao, Z.; Du, R. A Linear Finite Difference Scheme for the Two-Dimensional Nonlinear Schrödinger Equation with Fractional Laplacian. J. Sci. Comput. 2021, 90, 24. [Google Scholar] [CrossRef]
- Li, M.; Gu, X.M.; Huang, C.; Fei, M.; Zhang, G. A fast linearized conservative finite element method for the strongly coupled nonlinear fractional Schrödinger equations. J. Comput. Phys. 2018, 358, 256–282. [Google Scholar] [CrossRef]
- Duo, S.; Zhang, Y. Mass-conservative Fourier spectral methods for solving the fractional nonlinear Schrödinger equation. Comput. Math. Appl. 2016, 71, 2257–2271. [Google Scholar] [CrossRef]
- Bayındır, C.; Altintas, A.A.; Ozaydin, F. Self-localized solitons of a q-deformed quantum system. Commun. Nonlinear Sci. Numer. Simul. 2021, 92, 105474. [Google Scholar] [CrossRef]
- Bayındır, C. Self-localized solitons of the nonlinear wave blocking problem. Dyn. Atmos. Ocean. 2021, 93, 101189. [Google Scholar] [CrossRef]
- Fibich, G. The Nonlinear Schrödinger Equation; Springer: Berlin/Heidelberg, Germany, 2015; Volume 192. [Google Scholar] [CrossRef]
- Fokas, A.S.; Cao, Y.; He, J. Multi-Solitons, Multi-Breathers and Multi-Rational Solutions of Integrable Extensions of the Kadomtsev–Petviashvili Equation in Three Dimensions. Fractal Fract. 2022, 6, 425. [Google Scholar] [CrossRef]
- Bayındır, C. Self-localized solutions of the Kundu-Eckhaus equation in nonlinear waveguides. Results Phys. 2019, 14, 102362. [Google Scholar] [CrossRef]
- Trefethen, L.N. Spectral Methods in MATLAB; SIAM: Philadelphia, PA, USA, 2000. [Google Scholar]
- Canuto, C.; Hussaini, M.Y.; Quarteroni, A.; Zang, T.A. Spectral Methods: Fundamentals in Single Domains; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Vakhitov, N.G.; Kolokolov, A.A. Stationary solutions of the wave equation in the medium with nonlinearity saturation. Radiophys. Quantum Electron. 1973, 16, 783–789. [Google Scholar] [CrossRef]
- Weinstein, M.I. Modulational stability of ground states of nonlinear Schrödinger equations. SIAM J. Math. Anal. 1985, 16, 472–491. [Google Scholar] [CrossRef]
- Sivan, Y.; Fibich, G.; Ilan, B.; Weinstein, M. Qualitative and quantitative analysis of stability and instability dynamics of positive lattice solitons. Phys. Rev. E 2008, 78, 046602. [Google Scholar] [CrossRef] [PubMed]
- Bayındır, C. Compressive spectral method for the simulation of the nonlinear gravity waves. Sci. Rep. 2016, 6, 22100. [Google Scholar] [CrossRef] [PubMed]
- Bayındır, C. Compressive spectral renormalization method. TWMS J. Appl. Eng. Math. 2018, 8, 425–437. [Google Scholar]
- Macfarlane, A.J. On q-analogues of the quantum harmonic oscillator and the quantum group SU(2)q. J. Phys. Math. Gen. 1989, 22, 4581–4588. [Google Scholar] [CrossRef]
- Biedenharn, L.C. The quantum group SUq(2) and a q-analogue of the boson operators. J. Phys. Math. Gen. 1989, 22, L873–L878. [Google Scholar] [CrossRef]
- Biedenharn, L.C.; Lohe, M.A. Quantum Group Symmetry and q-Tensor Algebras; World Scientific: Singapore, 1995. [Google Scholar]
- Kharif, C.; Pelinovsky, E. Physical mechanisms of the rogue wave phenomenon. Eur. J. Mech.—B/Fluids 2003, 22, 603–634. [Google Scholar] [CrossRef]
- Khaykovich, L.; Schreck, F.; Ferrari, G.; Bourdel, T.; Cubizolles, J.; Carr, L.D.; Castin, Y.; Salomon, C. Formation of a matter-wave bright soliton. Science 2002, 296, 1290–1293. [Google Scholar] [CrossRef]
- Strecker, K.E.; Partridge, G.B.; Truscott, A.G.; Hulet, R.G. Formation and propagation of matter-wave soliton trains. Nature 2002, 417, 150–153. [Google Scholar] [CrossRef]
- Nguyen, J.H.; Luo, D.; Hulet, R.G. Formation of matter-wave soliton trains by modulational instability. Science 2017, 356, 422–426. [Google Scholar] [CrossRef] [PubMed]
- Falaye, B.; Oyewumi, K.; Abbas, M. Exact solution of Schrödinger equation with q-deformed quantum potentials using Nikiforov—Uvarov method. Chin. Phys. B 2013, 22, 110301. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bayındır, C.; Farazande, S.; Altintas, A.A.; Ozaydin, F. Petviashvili Method for the Fractional Schrödinger Equation. Fractal Fract. 2023, 7, 9. https://doi.org/10.3390/fractalfract7010009
Bayındır C, Farazande S, Altintas AA, Ozaydin F. Petviashvili Method for the Fractional Schrödinger Equation. Fractal and Fractional. 2023; 7(1):9. https://doi.org/10.3390/fractalfract7010009
Chicago/Turabian StyleBayındır, Cihan, Sofi Farazande, Azmi Ali Altintas, and Fatih Ozaydin. 2023. "Petviashvili Method for the Fractional Schrödinger Equation" Fractal and Fractional 7, no. 1: 9. https://doi.org/10.3390/fractalfract7010009
APA StyleBayındır, C., Farazande, S., Altintas, A. A., & Ozaydin, F. (2023). Petviashvili Method for the Fractional Schrödinger Equation. Fractal and Fractional, 7(1), 9. https://doi.org/10.3390/fractalfract7010009







