New Fractional Integral Inequalities Pertaining to Center-Radius (cr)-Ordered Convex Functions
(This article belongs to the Section Engineering)
Abstract
1. Introduction
2. Preliminaries
3. Interval-Valued -Convex Function
4. Integral Inequalities Pertaining to R-L Fractional Integrals
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Alomari, M.; Darus, M.; Kirmaci, U.S. Refinements of Hadamard-type inequalities for quasi-convex functions with applications to trapezoidal formula and to special means. Comput. Math. Appl. 2010, 59, 225–232. [Google Scholar] [CrossRef]
- Mumcu, İ; Set, E.; Akdemir, A.O.; Jarad, F. New extensions of Hermite-Hadamard inequalities via generalized proportional fractional integral. Numer. Methods Partial Differ. Equ. 2021. [Google Scholar] [CrossRef]
- Liu, K.; Wang, J.; O’Regan, D. On the Hermite-Hadamard type inequality for Ψ-Riemann-Liouville fractional integrals via convex functions. J. Inequal. Appl. 2019, 2019, 27. [Google Scholar] [CrossRef]
- Xi, B.Y.; Qi, F. Some integral inequalities of Hermite-Hadamard type for convex functions with applications to means. J. Funct. Spaces Appl. 2012, 2012, 980438. [Google Scholar] [CrossRef]
- Kirmaci, U.S.; Özdemir, M.E. On some inequalities for differentiable mappings and applications to special means of real numbers and to midpoint formula. Appl. Math. Comput. 2004, 153, 361–368. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Kashuri, A.; Mohammed, P.O.; Nonlaopon, K. Certain Inequalities pertaining to some new generalized fractional integral operators. Fractal Fract. 2021, 5, 160. [Google Scholar] [CrossRef]
- Dragomir, S.S.; Agarwal, R.P. Two inequalities for diferentiable mappings and applications to special means fo real numbers and to trapezoidal formula. Appl. Math. Lett. 1998, 11, 91–95. [Google Scholar] [CrossRef]
- Özdemir, M.E.; Avci, M.; Set, E. On some inequalities of Hermite-Hadamard type via m-convexity. Appl. Math. Lett. 2010, 23, 1065–1070. [Google Scholar] [CrossRef]
- Íşcan, Í. Hermite-Hadamard type inequalities for harmonically convex functions. Hacet. J. Math. Statist. 2013, 43, 935–942. [Google Scholar]
- Ahmad, H.; Tariq, M.; Sahoo, S.K.; Baili, J.; Cesarano, C. New estimations of Hermite–Hadamard type integral inequalities for special functions. Fractal Fract. 2021, 5, 144. [Google Scholar] [CrossRef]
- Dragomir, S.S.; Pearce, C.E.M. Selected Topics on Hermite-Hadamard Type Inequalities and Applications. RGMIA Monographs. 2000. Available online: http://rgmia.vu.edu.au/monographs/hermitehadamard.html (accessed on 1 July 2021).
- Moore, R.E. Interval Analysis; Prentice Hall: Englewood Cliffs, NJ, USA, 1966. [Google Scholar]
- Costa, T.M.; Román-Flores, H.; Chalco-Cano, Y. Opial-type inequalities for interval-valued functions. Fuzzy Set. Syst. 2019, 358, 48–63. [Google Scholar] [CrossRef]
- Flores-Franulič, A.; Chalco-Cano, Y.; Román-Flores, H. An Ostrowski type inequality for interval-valued functions. In Proceedings of the 2013 Joint IFSA World Congress and NAFIPS Annual Meeting (IFSA/NAFIPS), Edmonton, AB, Canada, 24–28 June 2013; Volume 35, pp. 1459–1462. [Google Scholar]
- Chalco-Cano, Y.; Flores-Franulič, A.; Román-Flores, H. Ostrowski type inequalities for interval-valued functions using generalized Hukuhara derivative. Comput. Appl. Math. 2012, 1, 457–472. [Google Scholar]
- Zhao, D.; An, T.; Ye, G.; Liu, W. New Jensen and Hermite-Hadamard type inequalities for h-convex interval-valued functions. J. Inequal. Appl. 2018, 2018, 302. [Google Scholar] [CrossRef]
- Khan, M.B.; Noor, M.A.; Mohammed, P.O.; Guirao, J.L.G.; Noor, K.I. Some integral inequalities for generalized convex fuzzy-interval-valued functions via fuzzy Riemann integrals. Int. J. Comput. Intell. Syst. 2021, 14, 158. [Google Scholar] [CrossRef]
- Bhunia, A.; Samanta, S. A study of interval metric and its application in multi-objective optimization with interval objectives. Comput. Ind. Eng. 2014, 74, 169–178. [Google Scholar] [CrossRef]
- Srivastava, H.M.; Sahoo, S.K.; Mohammed, P.O.; Baleanu, D.; Kodamasingh, B. Hermite-Hadamard type inequalities for interval-valued preinvex functions via fractional integral operators. Internat. J. Comput. Intel. Syst. 2022, 15, 8. [Google Scholar] [CrossRef]
- Sahoo, S.K.; Latif, M.A.; Alsalami, O.M.; Treanţă, S.; Sudsutad, W.; Kongson, J. Hermite-Hadamard, Fejér and Pachpatte-Type Integral Inequalities for Center-Radius Order Interval-Valued Preinvex Functions. Fractal Fract. 2022, 6, 506. [Google Scholar] [CrossRef]
- Costa, T.M. Jensen’s inequality type integral for fuzzy-interval-valued functions. Fuzzy Sets Syst. 2017, 327, 31–47. [Google Scholar] [CrossRef]
- Chalco-Cano, Y.; Lodwick, W.A. Condori-Equice. Ostrowski type inequalities and applications in numerical integration for interval-valued functions. Soft Comput. 2015, 19, 3293–3300. [Google Scholar] [CrossRef]
- Román-Flores, H.; Chalco-Cano, Y.; Lodwick, W.A. Some integral inequalities for interval-valued functions. Comput. Appl. Math. 2018, 37, 1306–1318. [Google Scholar] [CrossRef]
- Sahoo, S.K.; Al-Sarairah, E.; Mohammed, P.O.; Tariq, M.; Nonlaopon, K. Modified Inequalities on Center-Radius Order Interval-Valued Functions Pertaining to Riemann-Liouville Fractional Integrals. Axioms 2022, 11, 732. [Google Scholar] [CrossRef]
- Adjabi, Y.; Jarad, F.; Baleanu, D.; Abdeljawad, T. On Cauchy problems with Caputo-Hadamard fractional derivatives. Math. Meth. Appl. Sci. 2016, 40, 661–681. [Google Scholar]
- Zhou, X.S.; Huang, C.X.; Hu, H.J.; Liu, L. Inequality estimates for the boundedness of multilinear singular and fractional integral operators. J. Inequal. Appl. 2013, 2013, 303. [Google Scholar] [CrossRef]
- Liu, F.W.; Feng, L.B.; Anh, V.; Li, J. Unstructured-mesh Galerkin finite element method for the two-dimensional multi-term time-space fractional Bloch–Torrey equations on irregular convex domains. Comput. Math. Appl. 2019, 78, 1637–1650. [Google Scholar] [CrossRef]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations; North-Holland Mathematics Studies; Elsevier Science B.V.: Amsterdam, The Netherlands, 2006; Volume 204. [Google Scholar]
- Srivastava, H.M. An introductory overview of fractional-calculus operators based upon the Fox-Wright and related higher transcendental functions. J. Adv. Engrg. Comput. 2021, 5, 135–166. [Google Scholar] [CrossRef]
- Cloud, M.J.; Drachman, B.C.; Lebedev, L. Inequalities, 2nd ed.; Springer: Cham, Switzerland, 2014. [Google Scholar]
- Miller, K.S.; Ross, B. An Introduction to the Fractional Calculus and Fractional Differential Equations; Wiley: New York, NY, USA, 1993. [Google Scholar]
- Samko, S.G.; Kilbas, A.A.; Marichev, O.I. Fractional Integrals and Derivatives: Theory and Applications; Gordon & Breach Science Publishers: Yverdon, Switzerland, 1993. [Google Scholar]
- Sarikaya, M.Z.; Set, E.; Yaldiz, H.; Başak, N. Hermite–Hadamard inequalities for fractional integrals and related fractional inequalities. Math. Comput. Model. 2013, 57, 2403–2407. [Google Scholar] [CrossRef]
- Fernandez, A.; Mohammed, P.O. Hermite-Hadamard inequalities in fractional calculus defined using Mittag-Leffler kernels. Math. Meth. Appl. Sci. 2021, 44, 8414–8431. [Google Scholar] [CrossRef]
- Sarikaya, M.Z.; Yildirim, H. On Hermite-Hadamard type inequalities for Riemann-Liouville fractional integrals. Miskolc Math. Notes 2016, 17, 1049–1059. [Google Scholar] [CrossRef]
- Khan, T.U.; Khan, M.A. Hermite-Hadamard inequality for new generalized conformable fractional operators. AIMS Math. 2020, 6, 23–38. [Google Scholar] [CrossRef]
- Set, E. New inequalities of Ostrowski type for mapping whose derivatives are s-convex in the second-sense via fractional integrals. Comput. Math. Appl. 2012, 63, 1147–1154. [Google Scholar] [CrossRef]
- Ogulmus, H.; Sarikaya, M.Z. Hermite-Hadamard-Mercer type inequalities for fractional integrals. Filomat 2021, 35, 2425–2436. [Google Scholar] [CrossRef]
- Andrić, M.; Pečarič, J.; Perić, I. A multiple Opial type inequality for the Riemann-Liouville fractional derivatives. J. Math. Inequal. 2013, 2013 7, 139–150. [Google Scholar] [CrossRef]
- Sahoo, S.K.; Tariq, M.; Ahmad, H.; Kodamasingh, B.; Shaikh, A.A.; Botmart, T.; El-Shorbagy, M.A. Some Novel Fractional Integral Inequalities over a New Class of Generalized Convex Function. Fractal Fract. 2022, 6, 42. [Google Scholar] [CrossRef]
- Sahoo, S.K.; Mohammed, P.O.; Kodamasingh, B.; Tariq, M.; Hamed, Y.S. New Fractional Integral Inequalities for Convex Functions Pertaining to Caputo-Fabrizio Operator. Fractal Fract. 2022, 6, 171. [Google Scholar] [CrossRef]
- Niculescu, C.P.; Persson, L.E. Convex Functions and Their Applications; Springer: New York, NY, USA, 2006. [Google Scholar]
- Hadamard, J. Étude sur les propriétés des fonctions entières en particulier d’une fonction considérée par Riemann. J. Math. Pures. Appl. 1893, 58, 171–215. [Google Scholar]
- Shi, F.; Ye, G.; Liu, W.; Zhao, D. cr-h-convexity and some inequalities for cr-h-convex function. Filomat, 2022; submitted. [Google Scholar]
- Budak, H.; Tunç, T.; Sarikaya, M.Z. Fractional Hermite-Hadamard-type inequalities for interval-valued functions. Proc. Am. Math. Soc. 2020, 148, 705–718. [Google Scholar] [CrossRef]

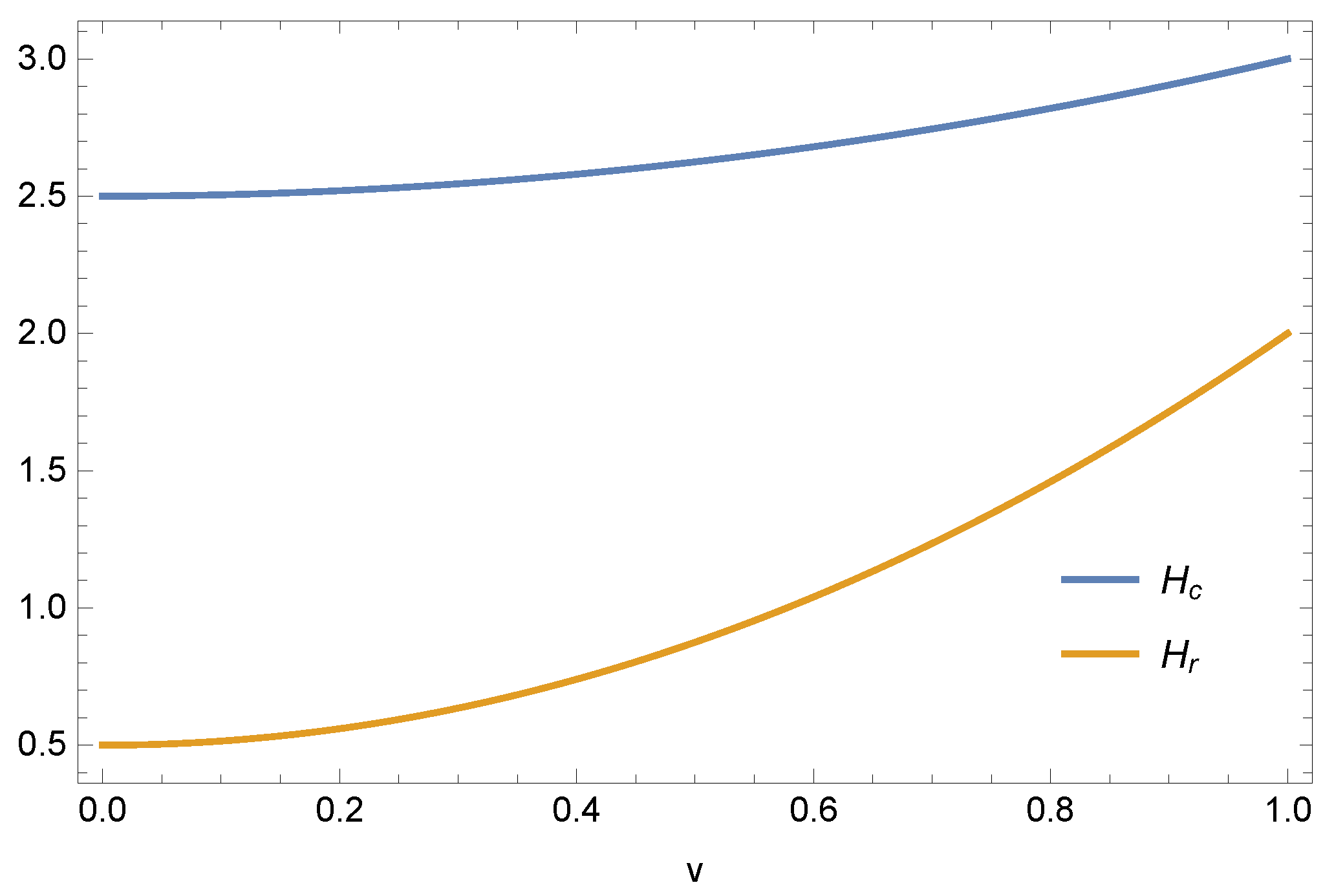
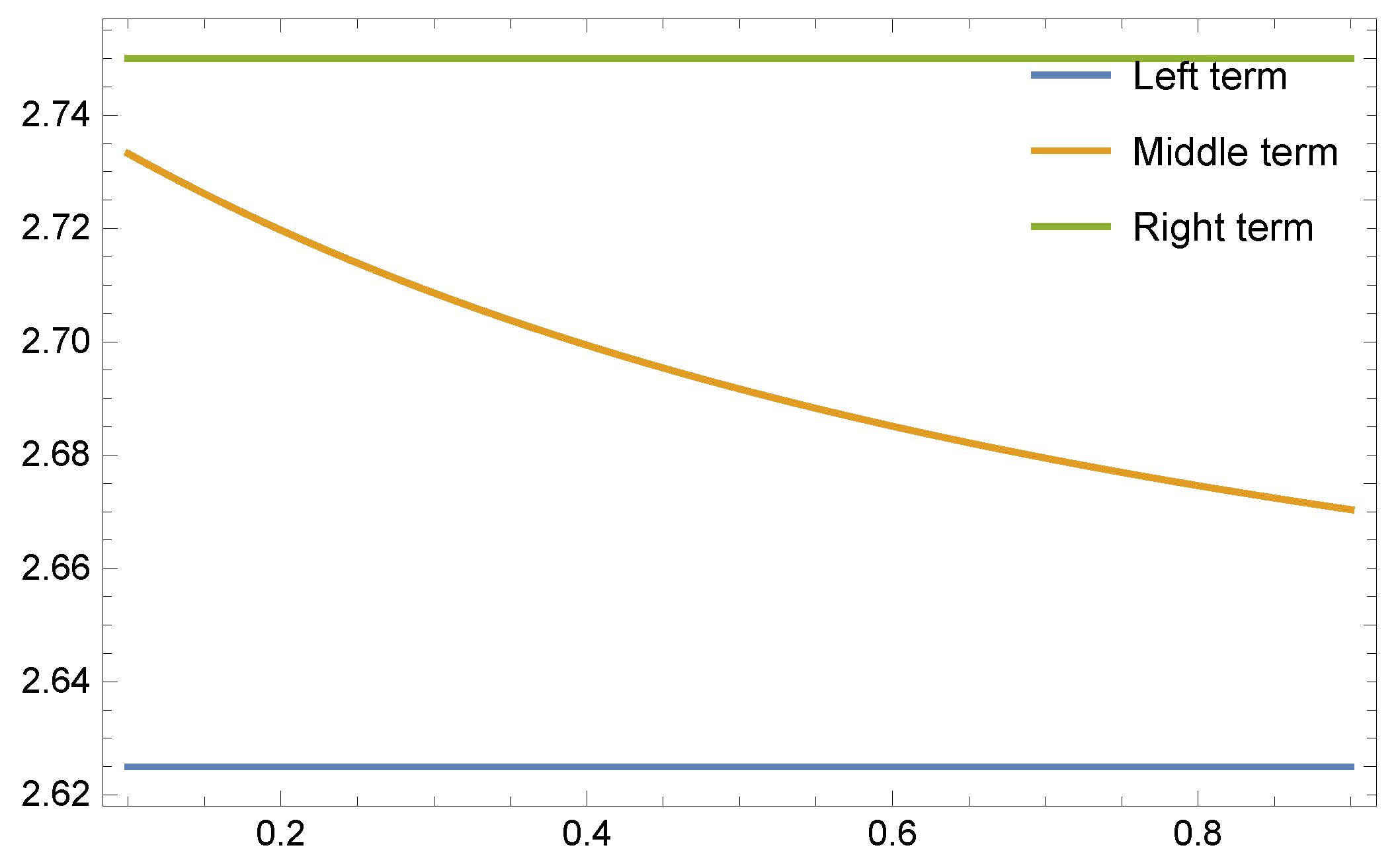
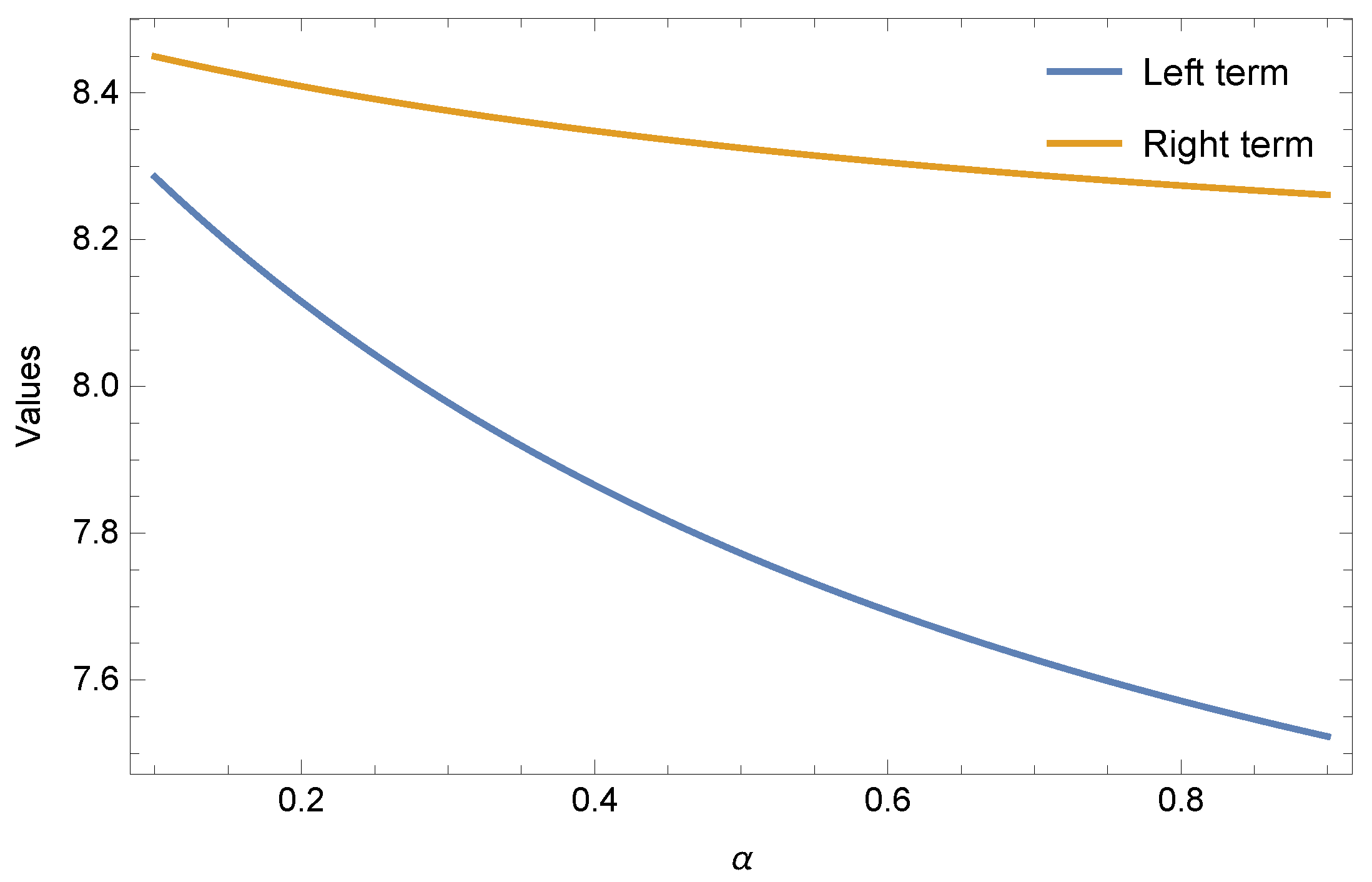
| Value of | Center Value of | Center Value of |
|---|---|---|
| 2.905 | 2.95 | |
| 2.820 | 2.90 | |
| 2.745 | 2.85 | |
| 2.680 | 2.80 | |
| 2.625 | 2.75 | |
| 2.580 | 2.70 | |
| 2.545 | 2.65 | |
| 2.520 | 2.60 | |
| 2.505 | 2.55 |
| Values of | Center Values of | ||
|---|---|---|---|
| the Left Term | the Middle Term | the Right Term | |
| 2.625 | 2.73323 | 2.75 | |
| 2.625 | 2.71970 | 2.75 | |
| 2.625 | 2.70861 | 2.75 | |
| 2.625 | 2.69940 | 2.75 | |
| 2.625 | 2.69167 | 2.75 | |
| 2.625 | 2.68510 | 2.75 | |
| 2.625 | 2.67947 | 2.75 | |
| 2.625 | 2.67460 | 2.75 | |
| 2.625 | 2.67037 | 2.75 | |
| Values of | Center Values of the Left Term | Center Values of the Right Term |
|---|---|---|
| 8.28571 | 8.44968 | |
| 8.11554 | 8.40909 | |
| 7.97817 | 8.37584 | |
| 7.86567 | 8.34821 | |
| 7.77240 | 8.32500 | |
| 7.69423 | 8.30529 | |
| 7.62808 | 8.28840 | |
| 7.57161 | 8.27381 | |
| 7.52303 | 8.26112 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sahoo, S.K.; Alrweili, H.; Treanţă, S.; Khan, Z.A. New Fractional Integral Inequalities Pertaining to Center-Radius (cr)-Ordered Convex Functions. Fractal Fract. 2023, 7, 81. https://doi.org/10.3390/fractalfract7010081
Sahoo SK, Alrweili H, Treanţă S, Khan ZA. New Fractional Integral Inequalities Pertaining to Center-Radius (cr)-Ordered Convex Functions. Fractal and Fractional. 2023; 7(1):81. https://doi.org/10.3390/fractalfract7010081
Chicago/Turabian StyleSahoo, Soubhagya Kumar, Hleil Alrweili, Savin Treanţă, and Zareen A. Khan. 2023. "New Fractional Integral Inequalities Pertaining to Center-Radius (cr)-Ordered Convex Functions" Fractal and Fractional 7, no. 1: 81. https://doi.org/10.3390/fractalfract7010081
APA StyleSahoo, S. K., Alrweili, H., Treanţă, S., & Khan, Z. A. (2023). New Fractional Integral Inequalities Pertaining to Center-Radius (cr)-Ordered Convex Functions. Fractal and Fractional, 7(1), 81. https://doi.org/10.3390/fractalfract7010081







