Structural and Fractal Analysis of Soil Cracks Due to the Roots of Setaria Viridis
Abstract
1. Introduction
2. Materials and Methods
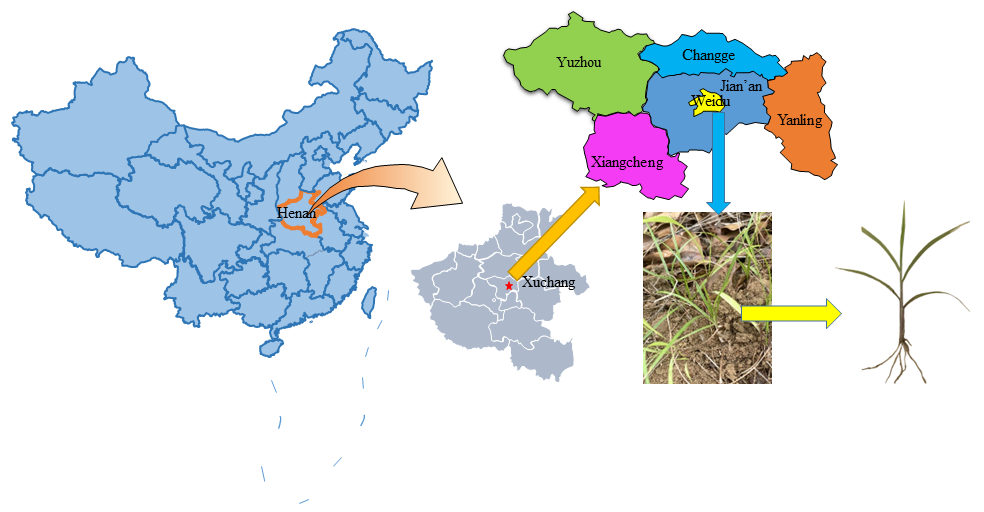
2.1. Research Background
2.2. Materials
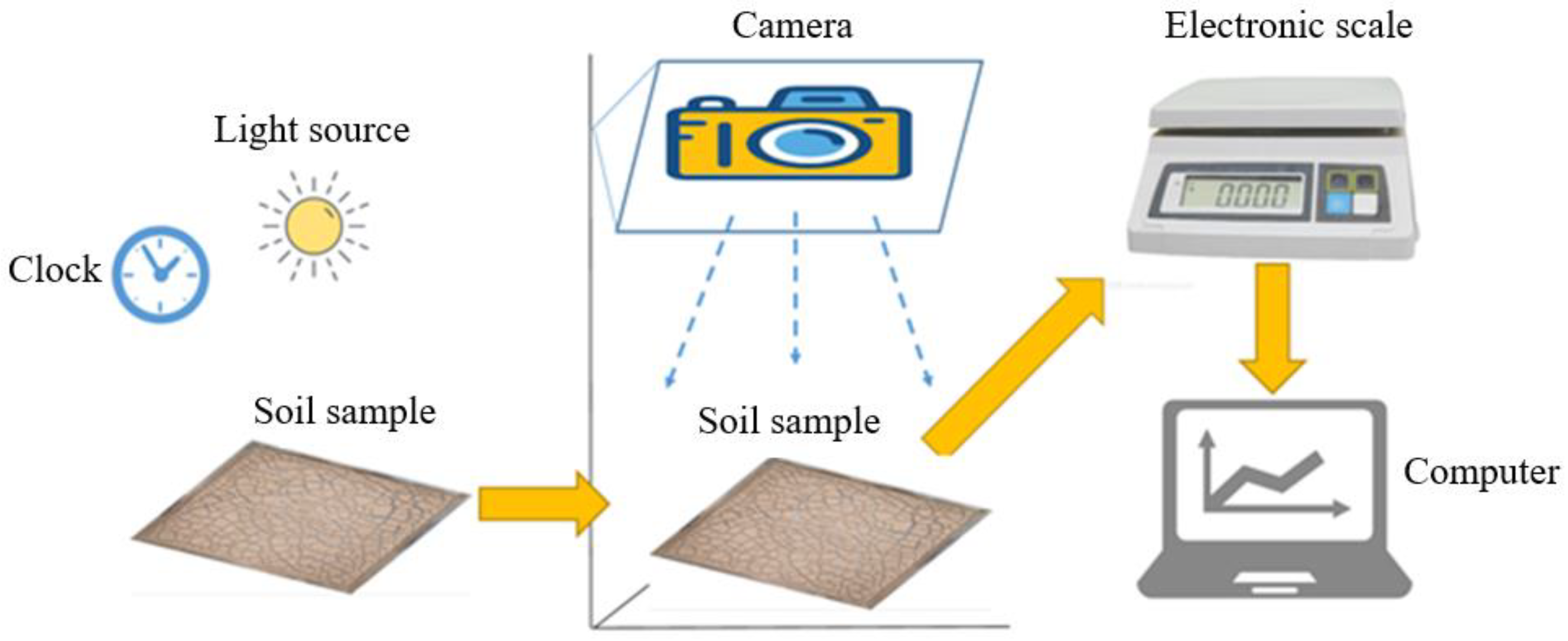
2.3. Experimental Process
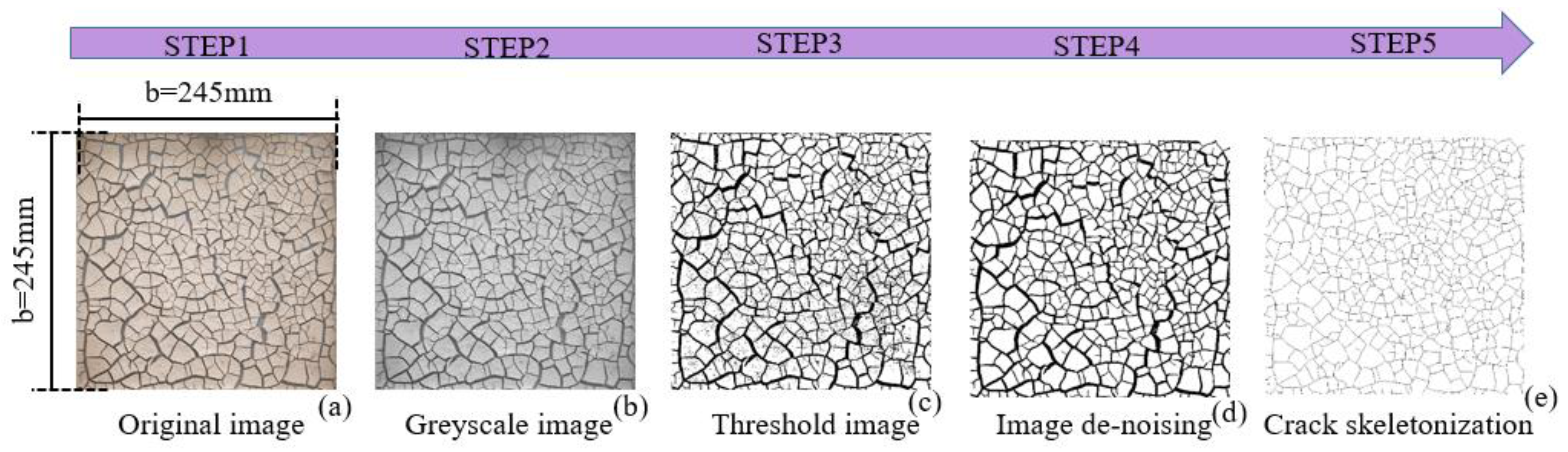
2.4. Image Processing
3. Analysis of Test Results
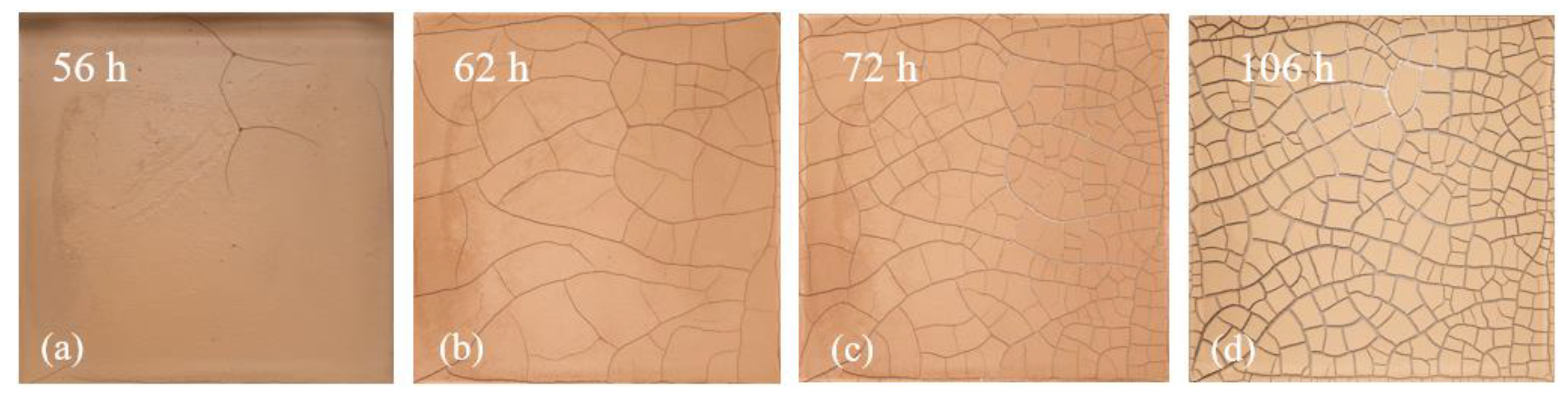
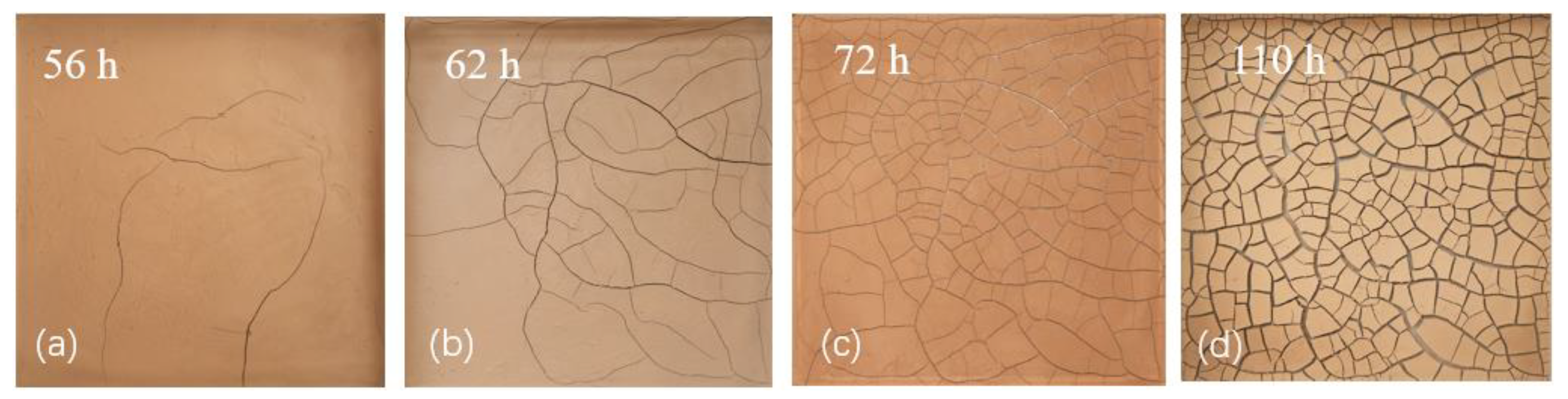
3.1. Effect of Root Distribution on Fracture Distribution
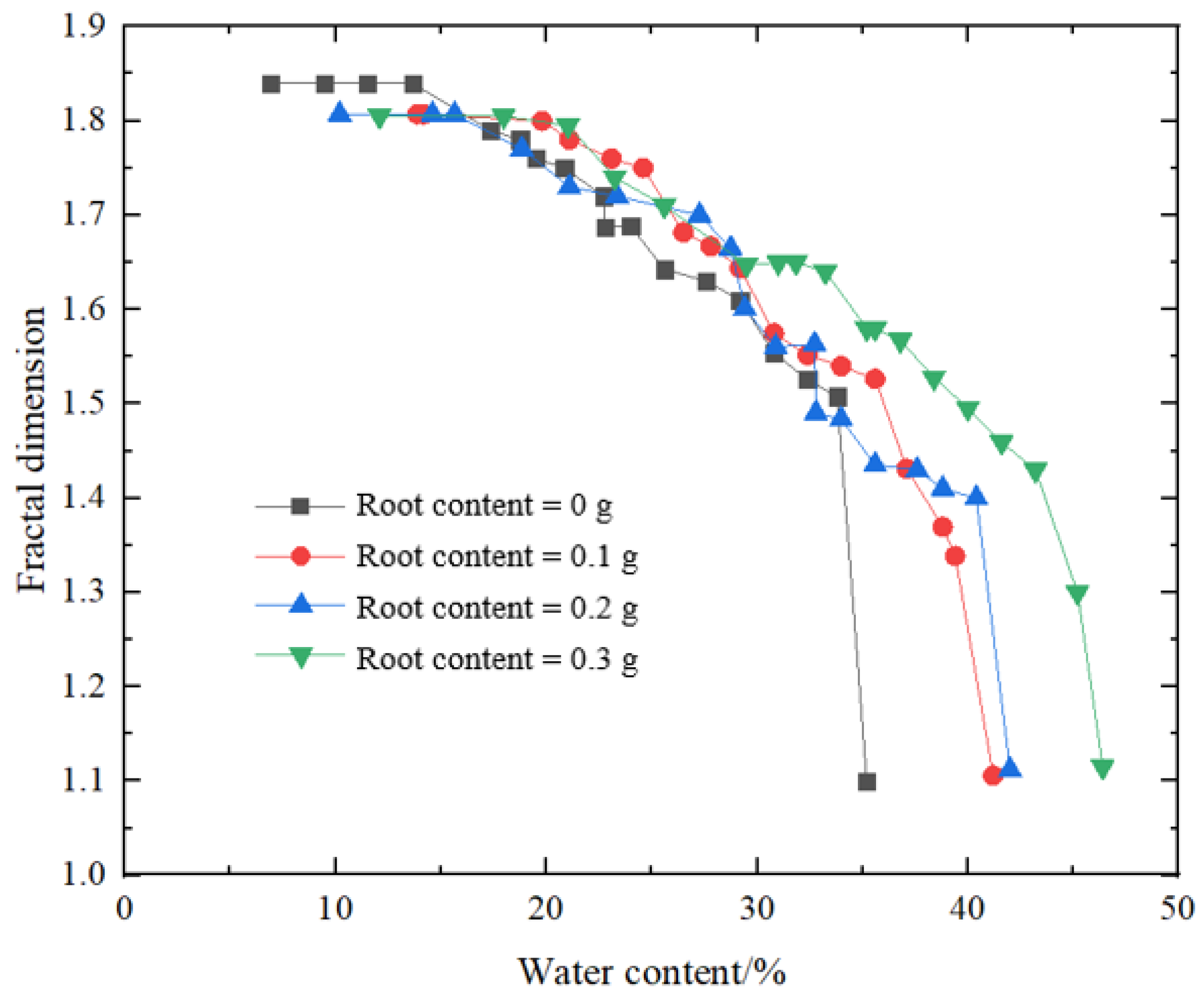
3.2. Effect of Root Content on Fractal Dimension of Soil Fissure Network
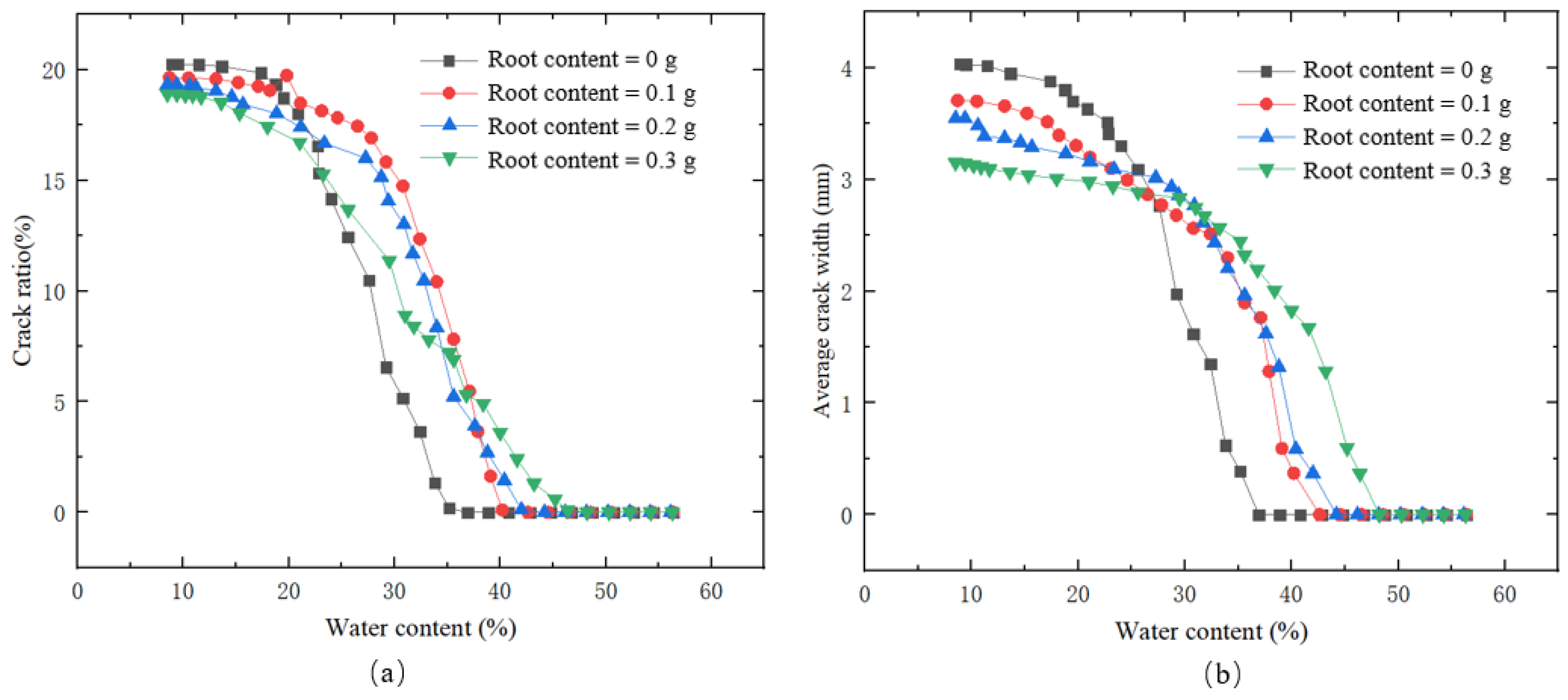
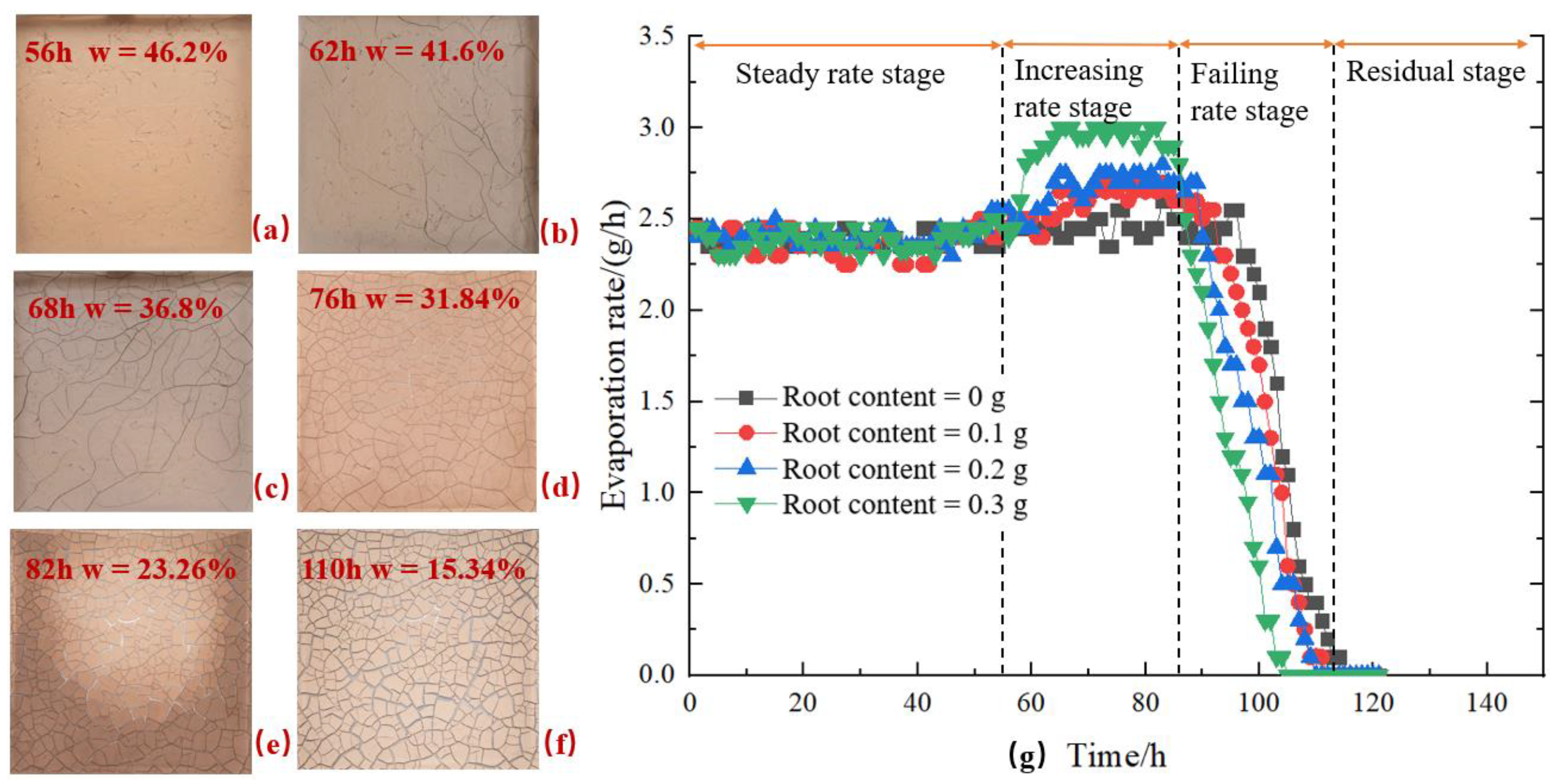
3.3. Effect of Setaria Viridis Root Content on Crack Rate and Crack Width
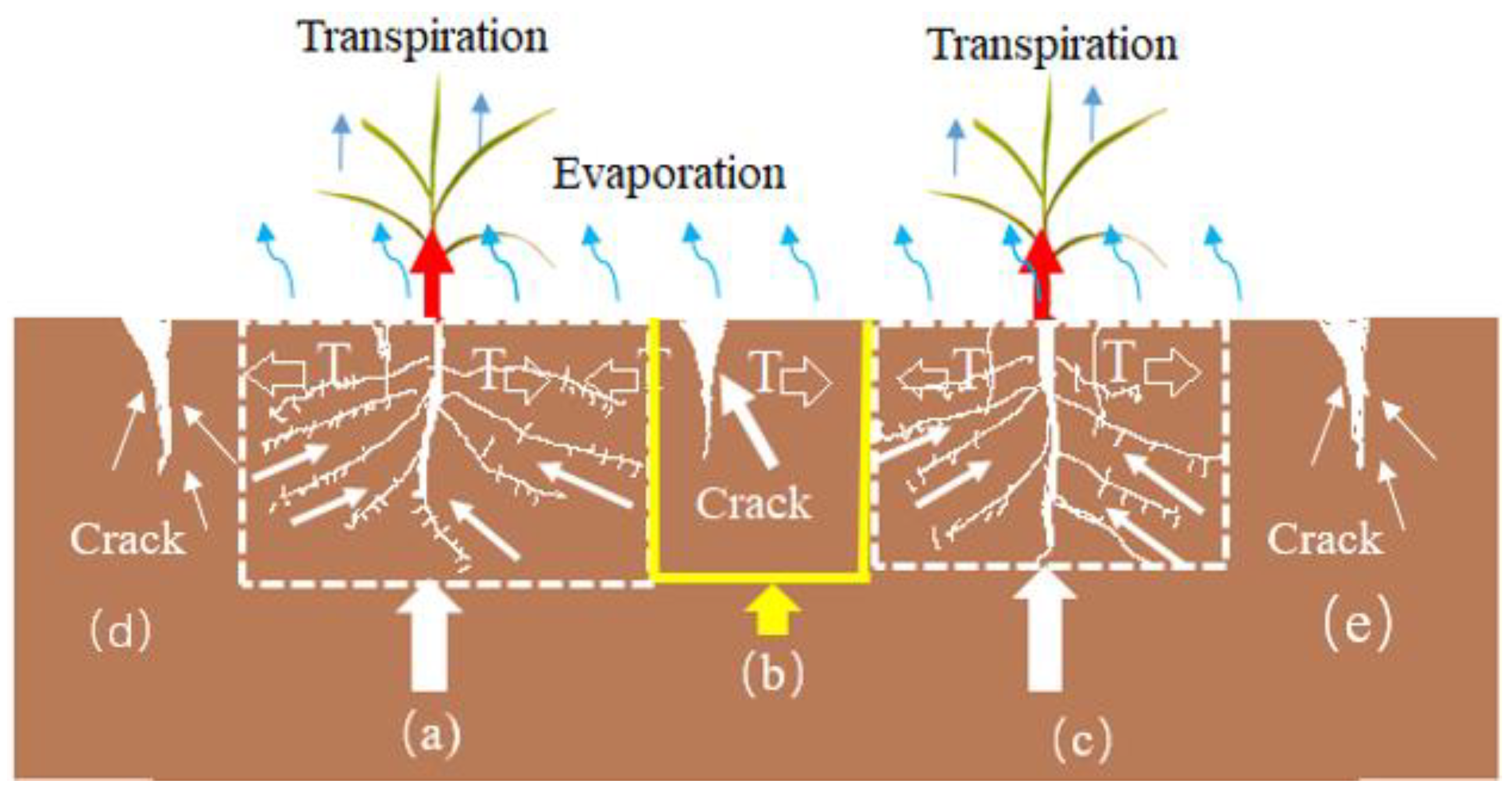
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rieu, M.; Sposito, G. Fractal Fragmentation, Soil Porosity, and Soil Water Properties: I. Theory. Soil Sci. Soc. Am. J. 1991, 55, 1231–1238. [Google Scholar] [CrossRef]
- Li, J.H.; Zhang, L.M. Geometric parameters and REV of a crack network in soil. Comput. Geotech. 2010, 37, 466–475. [Google Scholar] [CrossRef]
- Bordoloi, S.; Ni, J.; Ng, C. Soil desiccation cracking and its characterization in vegetated soil: A perspective review. Sci. Total Environ. 2020, 729, 138760. [Google Scholar] [CrossRef]
- Yuan, B.; Chen, W.; Zhao, J.; Li, L.; Liu, F.; Guo, Y.; Zhang, B. Addition of alkaline solutions and fibers for the reinforcement of kaolinite-containing granite residual soil. Appl. Clay Sci. 2022, 228, 106644. [Google Scholar] [CrossRef]
- Zhang, Z.; Tao, M.; Morvant, M. Cohesive slope surface failure and evaluation. J. Geotech. Geoenviron. Eng. 2005, 131, 898–906. [Google Scholar] [CrossRef]
- Wang, L.; Li, G.; Li, X.; Guo, F.; Tang, S.; Lu, X.; Hanif, A. Influence of reactivity and dosage of MgO expansive agent on shrinkage and crack resistance of face slab concrete. Cem. Conc. Compos. 2022, 126, 104333. [Google Scholar] [CrossRef]
- Wang, L.; Zeng, X.; Li, Y.; Yang, H.; Tang, S. Influences of MgO and PVA Fiber on the Abrasion and Cracking Resistance, Pore Structure and Fractal Features of Hydraulic Concrete. Fractal Fract. 2022, 6, 674. [Google Scholar] [CrossRef]
- Painuli, D.K.; Mohanty, M.; Sinha, N.K.; Misra, A.K. Crack formation in a swell–shrink soil under various managements. Agric. Res. 2017, 6, 66–72. [Google Scholar] [CrossRef]
- Fatahi, B.; Khabbaz, H.; Indraratna, B. Bioengineering ground improvement considering root water uptake model. Ecol. Eng. 2010, 36, 222–229. [Google Scholar] [CrossRef]
- Yuan, B.; Li, Z.; Chen, W.; Zhao, J.; Lv, J.; Song, J.; Cao, X. Influence of Groundwater Depth on Pile–Soil Mechanical Properties and Fractal Characteristics under Cyclic Loading. Fractal Fract. 2022, 6, 198. [Google Scholar] [CrossRef]
- Wu, T.H.; McKinnell, W.P., III; Swanston, D.N. Strength of tree roots and landslides on Prince of Wales Island, Alaska. Can. Geotech. J. 1979, 16, 19–33. [Google Scholar] [CrossRef]
- Wang, L.; Yu, Z.; Liu, B.; Zhao, F.; Tang, S.; Jin, M. Effects of Fly Ash Dosage on Shrinkage, Crack Resistance and Fractal Characteristics of Face Slab Concrete. Fractal Fract. 2022, 6, 335. [Google Scholar] [CrossRef]
- Abdullah, M.N.; Ali, F.H.; Osman, N. Soil-root Shear Strength Properties of Some Slope Plants. Sains Malays. 2011, 40, 1065–1073. [Google Scholar]
- Zhang, G.; Wang, R.; Qian, J.; Zhang, J.M.; Qian, J. Effect study of cracks on behavior of soil slope under rainfall conditions. Soils Found. 2012, 52, 634–643. [Google Scholar] [CrossRef]
- Wang, L.; Huang, Y.; Zhao, F.; Huo, T.; Chen, E.; Tang, S. Comparison between the influence of finely ground phosphorous slag and fly ash on frost resistance, pore structures and fractal features of hydraulic concrete. Fractal Fract. 2022, 6, 598. [Google Scholar] [CrossRef]
- Zhan, T.L.; Ng, C.W.W.; Fredlund, D.G. Field study of rainfall infiltration into a grassed unsaturated expansive soil slope. Can. Geotech. J. 2007, 44, 392–408. [Google Scholar] [CrossRef]
- Yuan, B.; Chen, M.; Chen, W.; Luo, Q.; Li, H. Effect of Pile-Soil Relative Stiffness on Deformation Characteristics of the Laterally Loaded Pile. Adv. Mater. Sci. Eng. 2022, 2022, 4913887. [Google Scholar] [CrossRef]
- Coppin, N.J.; Richards, I.G. Use of Vegetation in Civil Engineering; CIRIA Butterworths: London, UK, 1990. [Google Scholar]
- Freer, R. Bio-engineering: The use of vegetation in civil engineering. Constr. Build. Mater. 1991, 5, 23–26. [Google Scholar] [CrossRef]
- Morel, J.L.; Habib, L.; Plantureux, S.; Guckert, A. Influence of maize root mucilage on soil aggregate stability. Plant Soil 1991, 136, 111–119. [Google Scholar] [CrossRef]
- Xuan, W.; Band, L.R.; Kumpf, R.P.; Van Damme, D.; Parizot, B.; De Rop, G.; Opdenacker, D.; Möller, B.K.; Skorzinski, N.; Njo, M.F.; et al. Cyclic programmed cell death stimulates hormone signaling and root development in Arabidopsis. Science 2016, 351, 384. [Google Scholar] [CrossRef]
- Yan, Z.X.; Yan, C.M.; Wang, H.Y. Mechanical interaction between roots and soil mass in slope vegetation. Sci. China Tech. Sci. 2010, 53, 3039–3044. [Google Scholar] [CrossRef]
- Bordoloi, S.; Hussain, R.; Gadi, V.K.; Bora, H.; Sahoo, L.; Karangat, R.; Garg, A.; Sreedeep, S. Monitoring soil cracking and plant parameters for a mixed grass species. Géotech. Lett. 2018, 8, 49–55. [Google Scholar] [CrossRef]
- Li, J.H.; Li, L.; Chen, R.; Li, D.Q. Cracking and vertical preferential flow through landfill clay liners. Eng. Geol. 2016, 206, 33–41. [Google Scholar] [CrossRef]
- Yoshida, S.; Adachi, K. Effects of cropping and puddling practices on the cracking patterns in paddy fields. Soil Sci. Plant Nutri. 2001, 47, 519–532. [Google Scholar] [CrossRef]
- Zhang, H.; Liu, Z.; Chen, H.; Tang, M. Symbiosis of arbuscular mycorrhizal fungi and Robinia pseudoacacia L. improves root tensile strength and soil aggregate stability. PLoS ONE 2016, 11, e0153378. [Google Scholar] [CrossRef] [PubMed]
- Song, L.; Li, J.H.; Zhou, T.; Fredlund, D.G. Experimental study on unsaturated hydraulic properties of vegetated soil. Ecol. Eng. 2017, 103, 207–216. [Google Scholar] [CrossRef]
- Genet, M.; Stokes, A.; Salin, F.; Mickovski, S.B.; Fourcaud, T.; Dumail, J.F.; Van Beek, R. The influence of cellulose content on tensile strength in tree roots. Plant Soil 2005, 278, 1–9. [Google Scholar] [CrossRef]
- Zhu, H.; Indupriya, M.; Gadi, V.K.; Sreedeep, S.; Mei, G.X.; Garg, A. Assessment of the coupled effects of vegetation leaf and root characteristics on soil suction: An integrated numerical modeling and probabilistic approach. Acta Geotech. 2020, 15, 1331–1339. [Google Scholar] [CrossRef]
- Boldrin, D.; Leung, A.K.; Bengough, A.G. Correlating hydrologic reinforcement of vegetated soil with plant traits during establishment of woody perennials. Plant Soil. 2017, 416, 437–451. [Google Scholar] [CrossRef]
- Gadi, V.K.; Bordoloi, S.; Garg, A.; Sahoo, L.; Berretta, C.; Sekharan, S. Effect of shoot parameters on cracking in vegetated soil. J. Environ. Geotech. 2017, 5, 1–31. [Google Scholar] [CrossRef]
- Wang, L.; Zhou, S.; Shi, Y.; Huang, Y.; Zhao, F.; Huo, T.; Tang, S. The influence of fly ash dosages on the permeability, pore structure and fractal features of face slab concrete. Fractal Fract. 2022, 6, 476. [Google Scholar] [CrossRef]
- Cucci, G.; Lacolla, G.; Garanfa, G. Spatial distribution of roots and cracks in soils cultivated with sunflower. Archiv. Agron. Soil Sci. 2018, 64, 13–24. [Google Scholar] [CrossRef]
- Li, J.H.; Zhang, L.M.; Li, X. Soil-water characteristic curve and permeability function for unsaturated cracked soil. Can. Geotech. J. 2011, 48, 1010–1031. [Google Scholar] [CrossRef]
- Yuan, B.; Chen, W.; Zhao, J.; Yang, F.; Luo, Q.; Chen, T. The Effect of Organic and Inorganic Modifiers on the Physical Properties of Granite Residual Soil. Adv. Mater. Sci. Eng. 2022, 2022, 9542258. [Google Scholar] [CrossRef]
- Li, J.H.; Lu, Z.; Guo, L.B.; Zhang, L.M. Experimental study on soil-water characteristic curve for silty clay with desiccation cracks. Eng. Geol. 2017, 218, 70–76. [Google Scholar] [CrossRef]
- Ledesma, A.; Lakshmikantha, M.R.; Prat, P.C. Boundary effects in the desiccation of soil layers with controlled environmental conditions. Geotech. Test. J. 2018, 41, 675–697. [Google Scholar]
- Mandelbrot, B.B. The Fractal Geometry of Nature. Am. J. Phys. 1983. [Google Scholar] [CrossRef]
- Dang, W.; Wu, W.; Konietzky, H.; Qian, J. Effect of shear-induced aperture evolution on fluid flow in rock fractures. Comput. Geotech. 2019, 114, 103152. [Google Scholar] [CrossRef]
- Sui, L.; Yu, J.; Cang, D.; Miao, W.; Wang, H.; Zhang, J.; Yin, S.; Chang, K. The fractal description model of rock fracture networks characterization. Chaos Solitons Fractals 2019, 129, 71–76. [Google Scholar] [CrossRef]
- Yasrebi, A.B.; Wetherelt, A.; Foster, P.; Coggan, J.; Afzal, P.; Agterberg, F.; Ahangaran, D.K. Application of a density–volume fractal model for rock characterisation of the Kahang porphyry deposit. Int. J. Rock Mech. Min. Sci. 2014, 66, 188–193. [Google Scholar] [CrossRef]
- Tang, C.S.; Zhu, C.; Cheng, Q.; Zeng, H.; Xu, J.J.; Tian, B.G.; Shi, B. Desiccation cracking of soils: A review of investigation approaches, underlying mechanisms, and influencing factors. Earth-Sci. Rev. 2021, 216, 103586. [Google Scholar] [CrossRef]
- Tang, C.; Cheng, Q.; Lin, L. Study on the dynamic mechanism of soil desiccation cracking by surface strain/displacement analysis. Comput. Geotech. 2022, 152, 104998. [Google Scholar] [CrossRef]
- Baer, J.U.; Kent, T.F.; Anderson, S.H. Image analysis and fractal geometry to characterize soil desiccation cracks. Geoderma 2009, 154, 153–163. [Google Scholar] [CrossRef]
- Vallejo, L.E. Fractal analysis of temperature-induced cracking in clays and rocks. Géotechnique 2009, 59, 283–286. [Google Scholar] [CrossRef]
- Leung, A.K.; Garg, A.; Ng, C. Effects of plant roots on soil-water retention and induced suction in vegetated soil. Eng. Geol. 2015, 193, 183–197. [Google Scholar] [CrossRef]
- Kodikara, J.; Costa, S. Desiccation Cracking in Clayey Soils: Mechanisms and Modelling. In Multiphysical Testing of Soils and Shales; Laloui, L., Ferrari, A., Eds.; Springer Series in Geomechanics and Geoengineering; Springer: Berlin/Heidelberg, Germany, 2013. [Google Scholar]
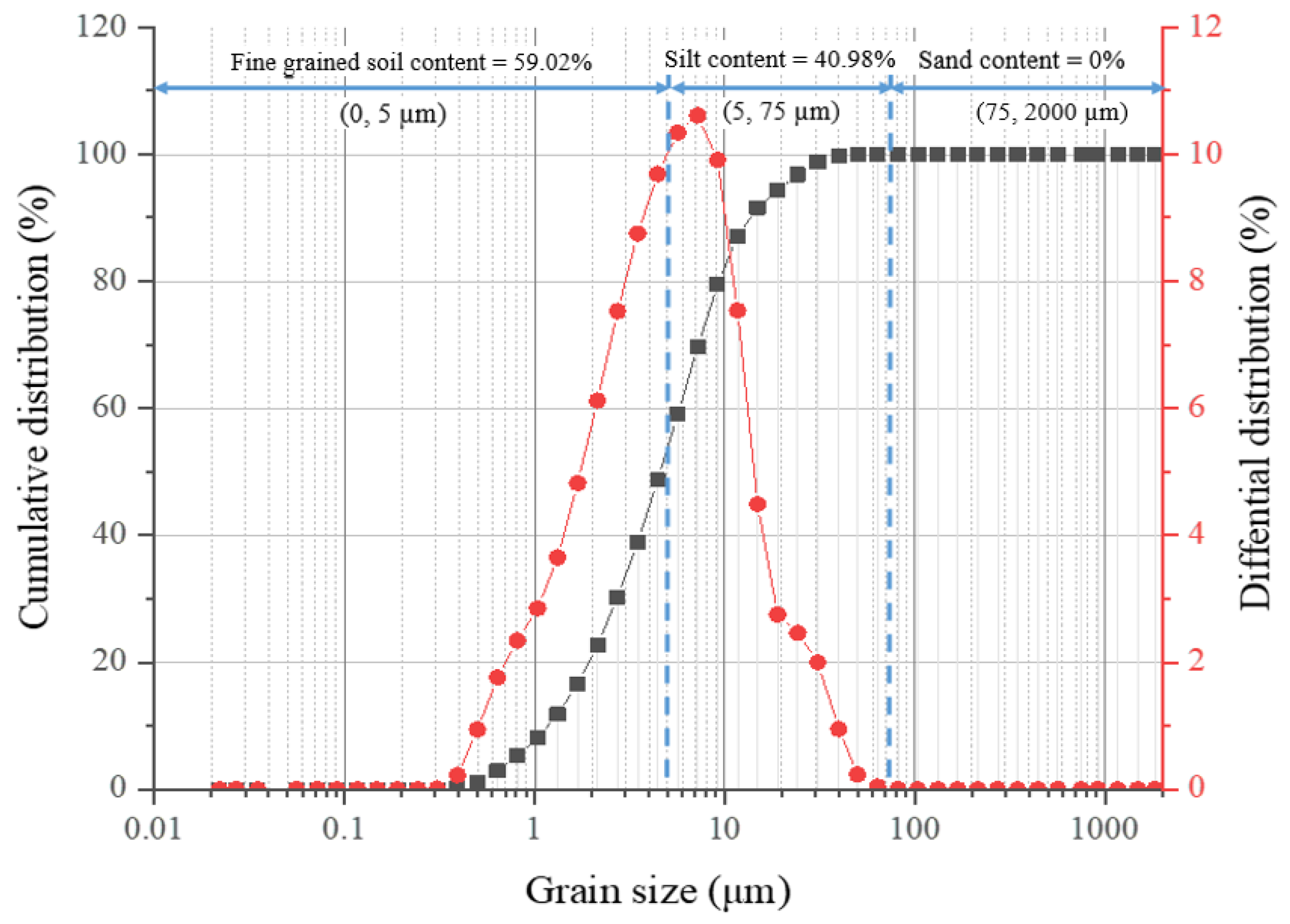

| Specific Gravity | Natural Water Content /% | Density /(g/cm3) | Plastic Limit % | Liquid Limit % | Plasticity Index |
|---|---|---|---|---|---|
| 2.72 | 9.3 | 1.76 | 20.4 | 35.4 | 15 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tang, Y.; Yang, B.; Zhao, X.; Yang, C. Structural and Fractal Analysis of Soil Cracks Due to the Roots of Setaria Viridis. Fractal Fract. 2023, 7, 19. https://doi.org/10.3390/fractalfract7010019
Tang Y, Yang B, Zhao X, Yang C. Structural and Fractal Analysis of Soil Cracks Due to the Roots of Setaria Viridis. Fractal and Fractional. 2023; 7(1):19. https://doi.org/10.3390/fractalfract7010019
Chicago/Turabian StyleTang, Yuchen, Binbin Yang, Xiaoming Zhao, and Changde Yang. 2023. "Structural and Fractal Analysis of Soil Cracks Due to the Roots of Setaria Viridis" Fractal and Fractional 7, no. 1: 19. https://doi.org/10.3390/fractalfract7010019
APA StyleTang, Y., Yang, B., Zhao, X., & Yang, C. (2023). Structural and Fractal Analysis of Soil Cracks Due to the Roots of Setaria Viridis. Fractal and Fractional, 7(1), 19. https://doi.org/10.3390/fractalfract7010019






