New Numerical Approach of Solving Highly Nonlinear Fractional Partial Differential Equations via Fractional Novel Analytical Method
Abstract
:1. Introduction
2. Preliminaries of Fractional Calculus
3. Highly Nonlinear Partial Differential Equations of Fractional Order via Fractional Novel Analytical Method
4. Novel Analytical Method Convergence Analysis
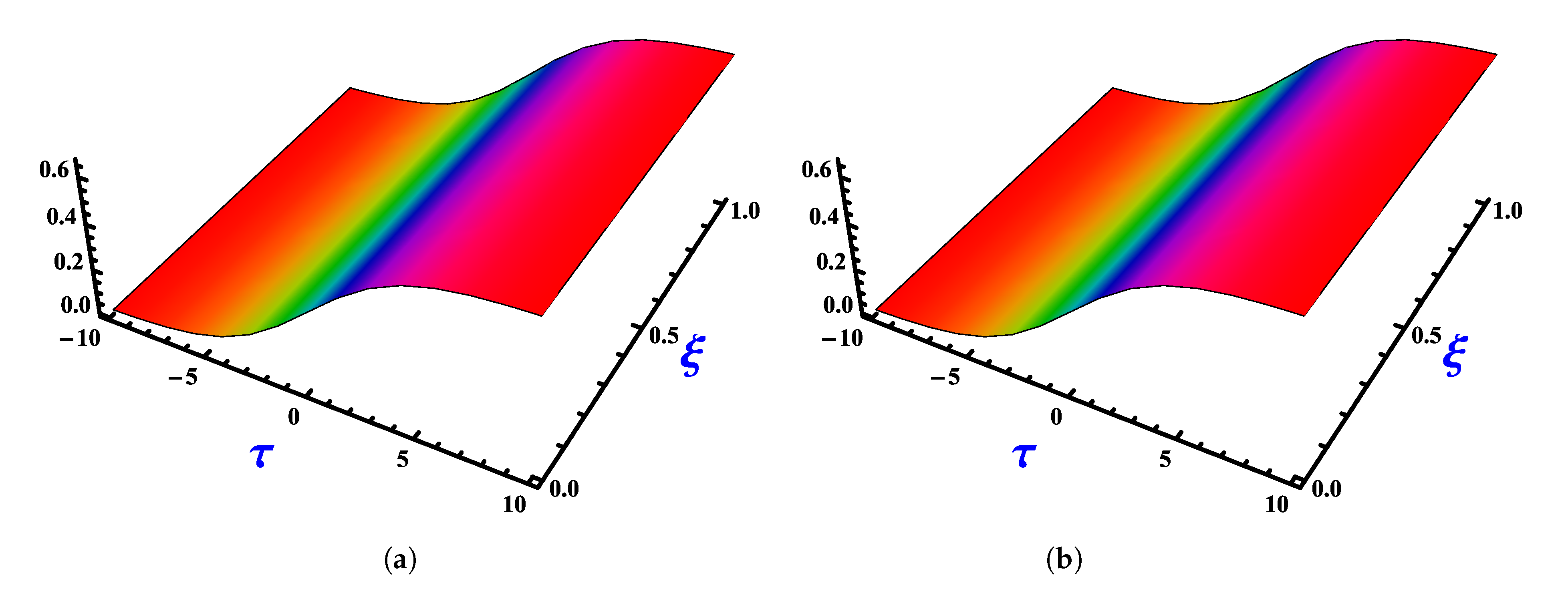
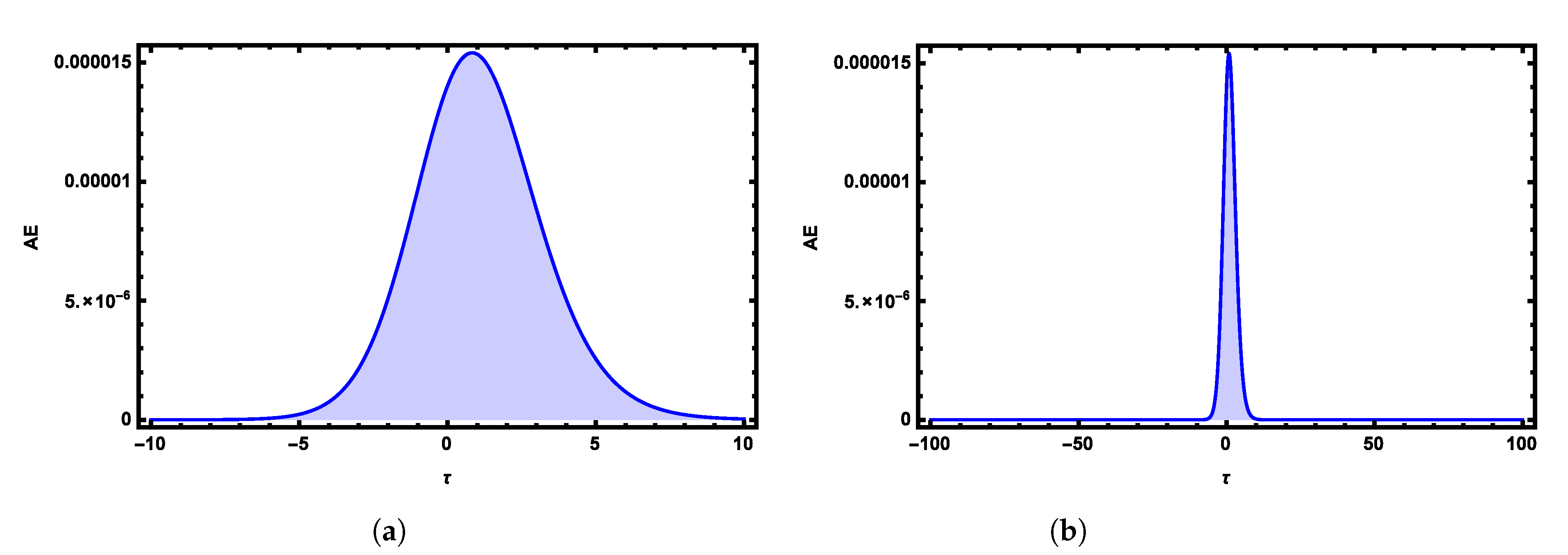
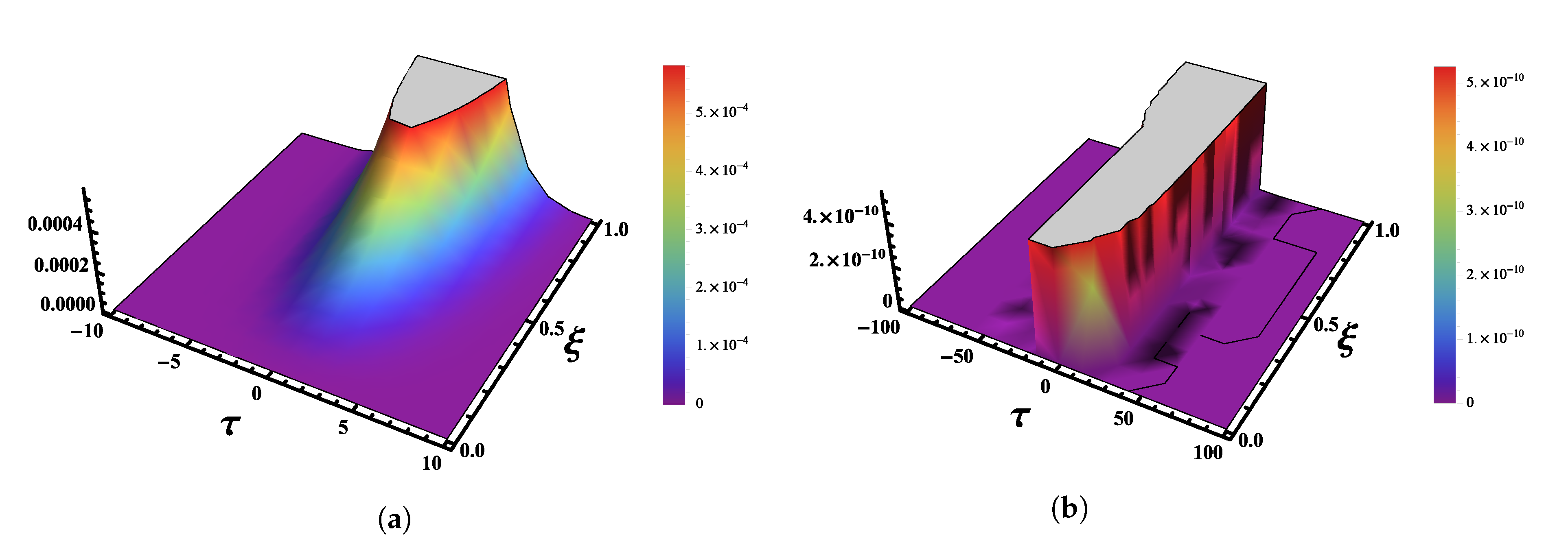
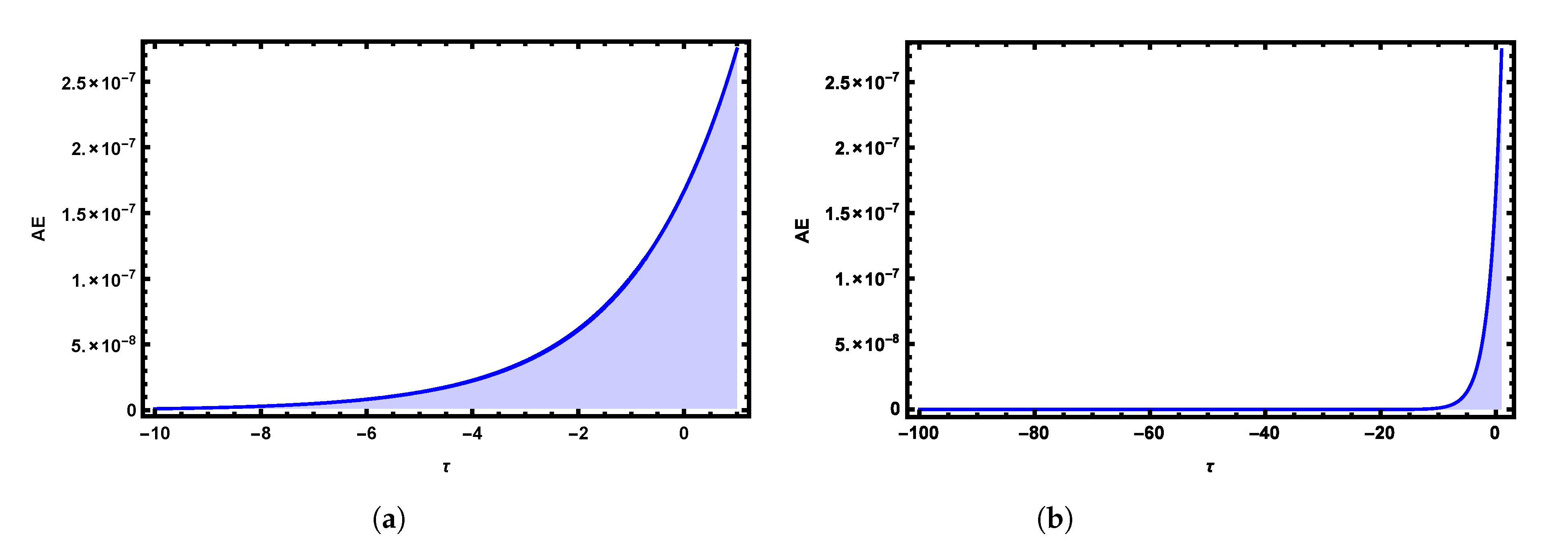
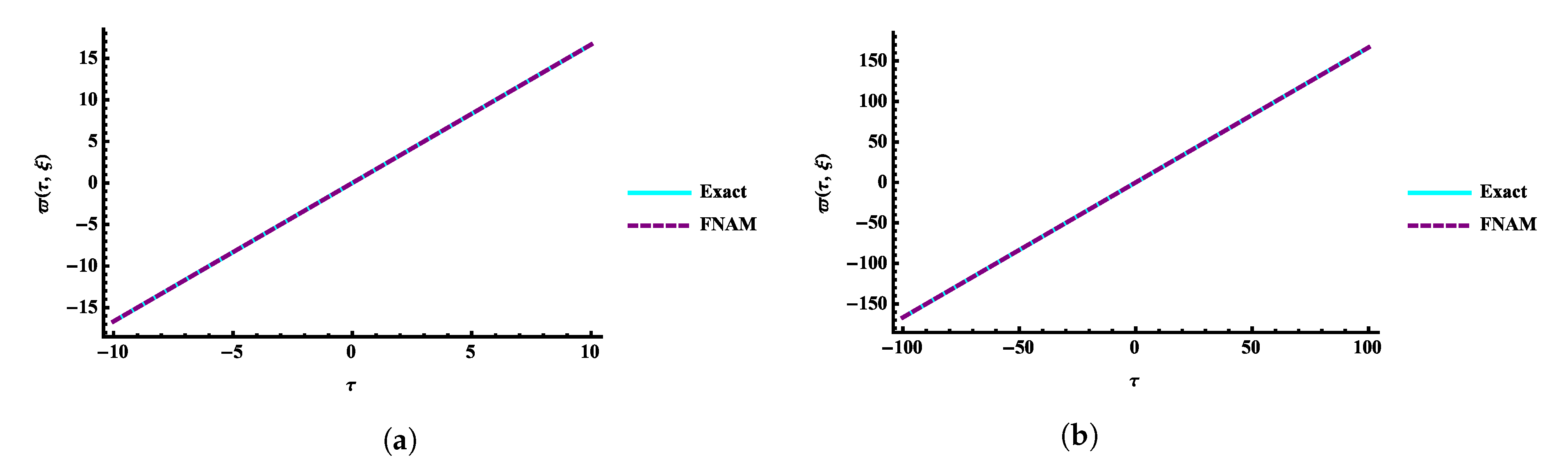
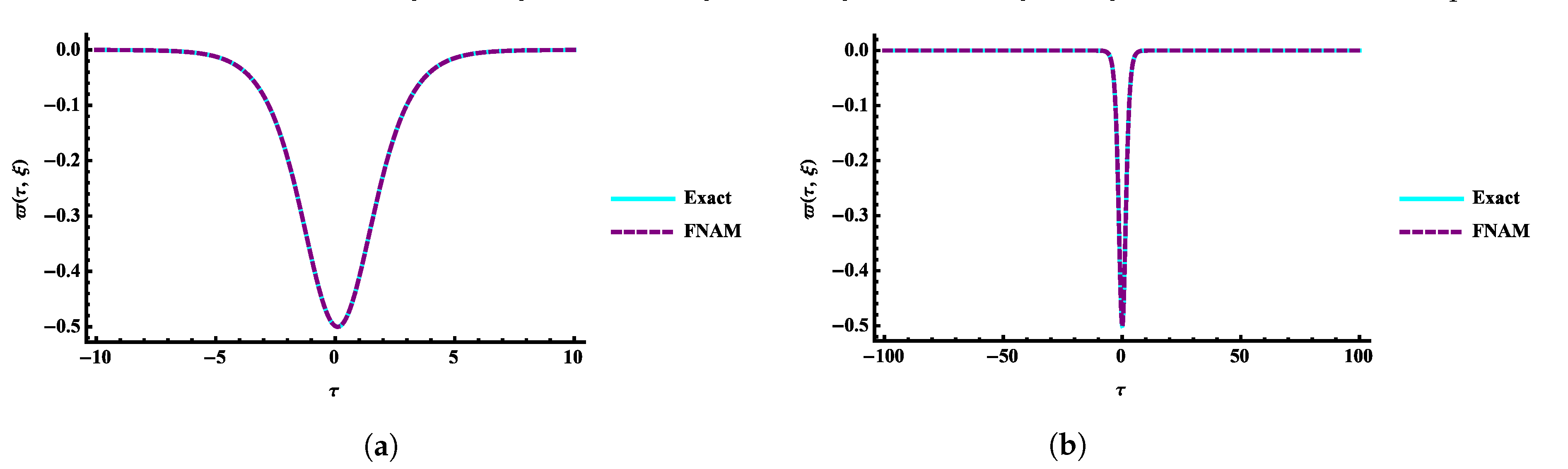
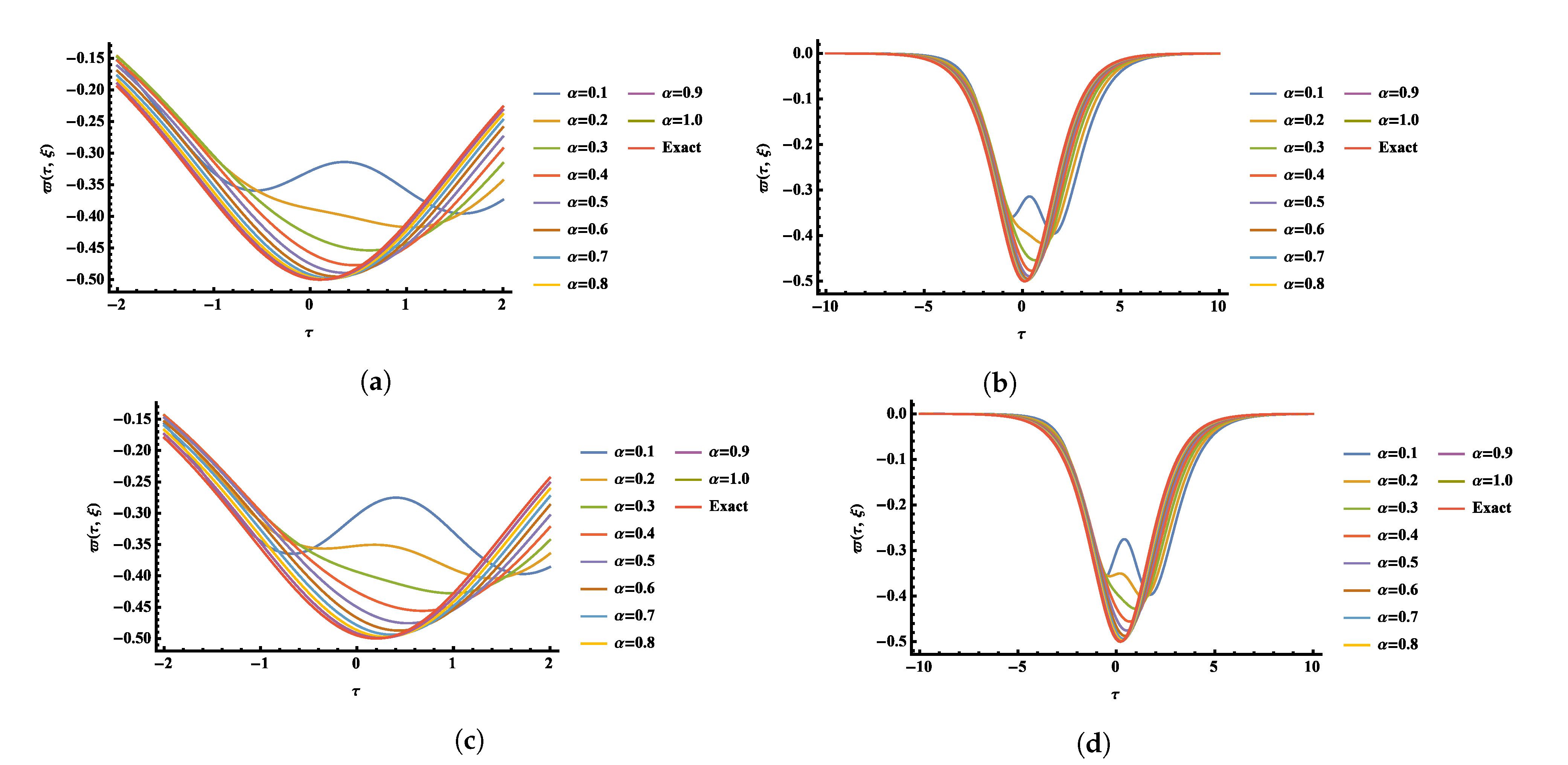
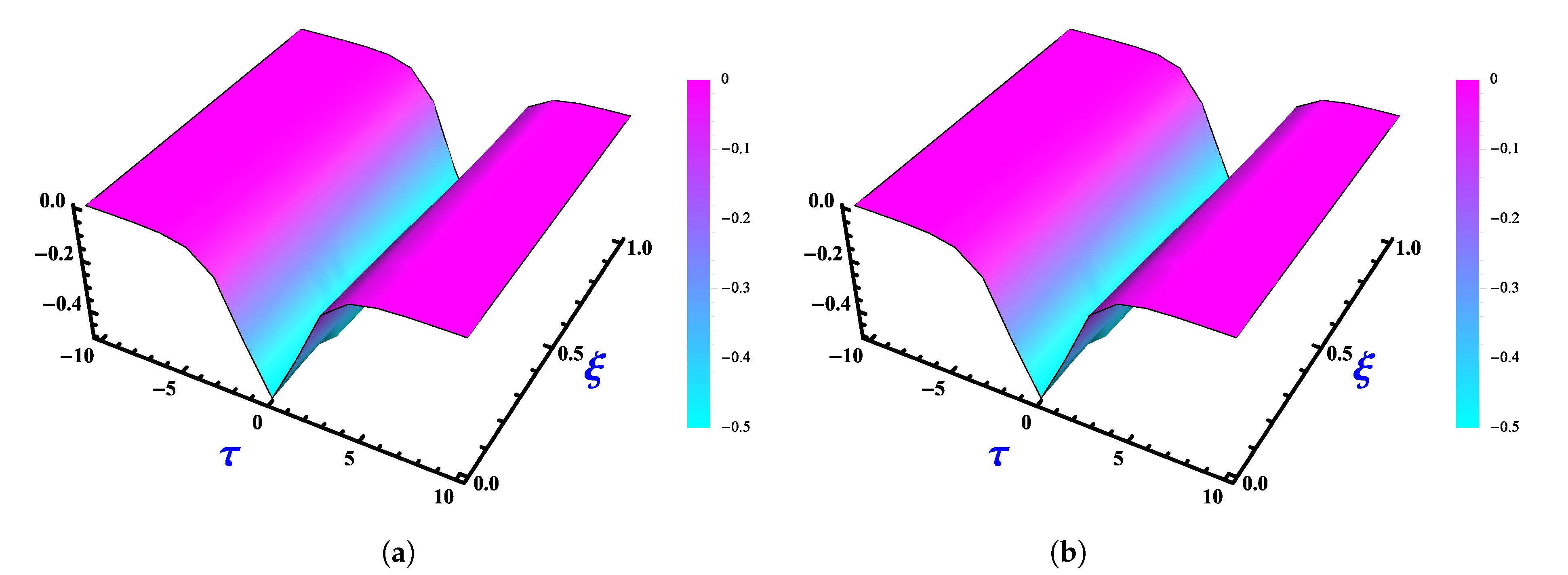
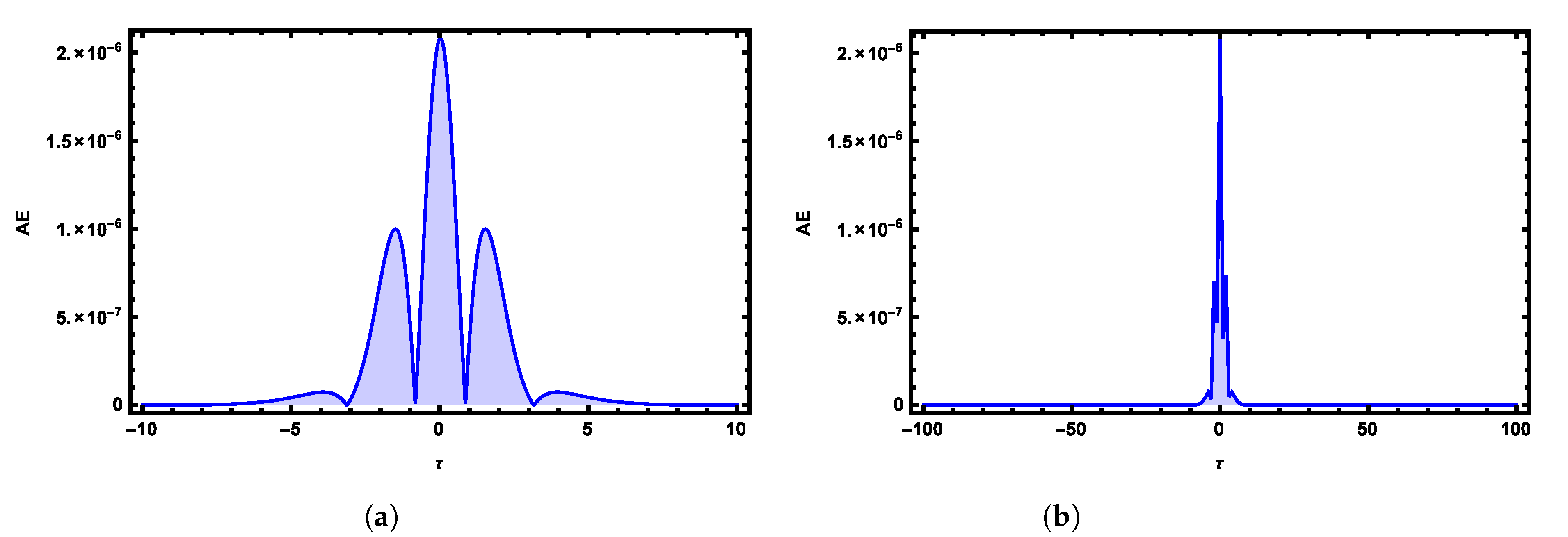
5. Numerical Application for Fractional Novel Analytical Method for Highly Nonlinear Partial Differential Equations
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Brezis, H.M.; Browder, F. Partial differential equations in the 20th century. Adv. Math. 1998, 135, 76–144. [Google Scholar] [CrossRef]
- Noor, M.A.; Noor, K.I.; Waheed, A.; Al-Said, E.A. Some new solitonary solutions of the modified Benjamin–Bona–Mahony equation. Comput. Math. Appl. 2011, 62, 2126–2131. [Google Scholar] [CrossRef]
- Eleuch, H.; Rostovtsev, Y.V. Analytical solution for 3D stationary Schrödinger equation: Implementation of Huygens’ principle for matter waves. J. Mod. Opt. 2010, 57, 1877–1881. [Google Scholar] [CrossRef]
- Cajori, F. The early history of partial differential equations and of partial differentiation and integration. Am. Math. Mon. 1928, 35, 459–467. [Google Scholar] [CrossRef]
- Boutabba, N.; Eleuch, H.; Bouchriha, H. Thermal bath effect on soliton propagation in three-level atomic system. Synth. Met. 2009, 159, 1239–1243. [Google Scholar] [CrossRef]
- Gómez S., C.A.; Salas, A.H.; Frias, B.A. New periodic and soliton solutions for the generalized BBM and BBM–Burgers equations. Appl. Math. Comput. 2010, 217, 1430–1434. [Google Scholar] [CrossRef]
- Solin, P.; Segeth, K.; Dolezel, I. Higher-order Finite Element Methods, 1st ed.; Chapman & Hall/CRC Press: New York, NY, USA, 2003. [Google Scholar] [CrossRef]
- Zhou, J.K. Differential Transformation and Its Applications for Electrical Circuits; Huazhong University Press: Wuhan, China, 1986. [Google Scholar]
- Carey, G.F.; Shen, Y. Least-squares finite element approximation of Fishers reaction-diffusion equation. Numer. Methods Partial Diff. Equ. 1995, 11, 175–186. [Google Scholar] [CrossRef]
- Hariharan, G.; Kannan, K.; Sharma, K.R. Haar wavelet method for solving Fisher’s equation. Appl. Math. Comput. 2009, 211, 284–292. [Google Scholar] [CrossRef]
- Boyd, J.P. Chebyshev and Fourier Spectral Methods; Second Revised Edition; Dover Publication, Inc.: Mineola, NY, USA, 2001. [Google Scholar]
- Biazar, J.; Eslami, M. Homotopy perturbation method for systems of partial differential equations. Int. J. Nonlinear Sci. Numer. Simul. 2007, 8, 411–416. [Google Scholar] [CrossRef]
- Nassar, C.J.; Revelli, J.F.; Bowman, R.J. Application of the homotopy analysis method to the Poisson–Boltzmann equation for semiconductor devices. Commun. Nonlinear Sci. Numer. Simul. 2011, 16, 2501–2512. [Google Scholar] [CrossRef]
- El-Sayed, A.; Gaber, M. The Adomian decomposition method for solving partial differential equations of fractal order in finite domains. Phys. Lett. A 2006, 359, 175–182. [Google Scholar] [CrossRef]
- Abdel-Aty, A.H.; Khater, M.M.A.; Attia, R.A.M.; Abdel-Aty, M.; Eleuch, H. On the new explicit solutions of the fractional nonlinear space-time nuclear model. Fractals 2020, 28, 2040035. [Google Scholar] [CrossRef]
- Khalid, M.; Sultana, M.; Zaidi, F.; Arshad, U. Solving Linear and Nonlinear Klein-Gordon Equations by New Perturbation Iteration Transform Method. TWMS J. Appl. Eng. Math. 2016, 6, 115–125. [Google Scholar]
- Dehghan, M.; Abbaszadeh, M.; Mohebbi, A. The use of interpolating element-free Galerkin technique for solving 2D generalized Benjamin–Bona–Mahony–Burgers and regularized long-wave equations on non-rectangular domains with error estimate. J. Comput. Appl. Math. 2015, 286, 211–231. [Google Scholar] [CrossRef]
- Dehghan, M.; Abbaszadeh, M.; Mohebbi, A. The numerical solution of nonlinear high dimensional generalized Benjamin–Bona–Mahony–Burgers equation via the meshless method of radial basis functions. Comput. Math. Appl. 2014, 68, 212–237. [Google Scholar] [CrossRef]
- Dehghan, M.; Heris, J.M. Study of the wave-breakings qualitative behavior of the Fornberg–Whitham equation via quasi-numeric approaches. Int. J. Numer. Methods Heat Fluid Flow 2012, 22, 537–553. [Google Scholar] [CrossRef]
- Tabatabaei, A.H.A.E.; Shakour, E.; Dehghan, M. Some implicit methods for the numerical solution of Burgers’ equation. Appl. Math. Comput. 2007, 191, 560–570. [Google Scholar] [CrossRef]
- Dehghan, M.; Heris, J.M.; Saadatmandi, A. Application of semi-analytic methods for the Fitzhugh–Nagumo equation, which models the transmission of nerve impulses. Math. Methods Appl. Sci. 2010, 33, 1384–1398. [Google Scholar] [CrossRef]
- Wazwaz, A.M. Partial Differential Equations and Solitary Waves Theory. Nonlinear Physical Science; Springer: Berlin/Heidelberg, Germany, 2009. [Google Scholar] [CrossRef]
- Wazwaz, A.M. Partial Differential Equations Methods and Applications; A.A. Balkema Publishers: Tokyo, Japan, 2002. [Google Scholar]
- Aljaberi, A.; Hameed, E.M.; Abdul-Wahab, M.S. A novel analytic method for solving linear and nonlinear Telegraph Equation. Periódico Tchê Química 2020, 17. [Google Scholar] [CrossRef]
- Sultana, M.; Arshad, U.; Alam, M.N.; Bazighifan, O.; Askar, S.; Awrejcewicz, J. New Results of the Time-Space Fractional Derivatives of Kortewege-De Vries Equations via Novel Analytic Method. Symmetry 2021, 13, 2296. [Google Scholar] [CrossRef]
- Wiwatwanich, A. A Novel Technique for Solving Nonlinear Differential Equations. Ph.D. Thesis, Faculty of Science Burapha University, Saen Suk, Thailand, 2016. [Google Scholar]
- Sarikaya, M.Z.; Ogunmez, H. On New Inequalities via Riemann-Liouville Fractional Integration. Abstract Appl. Anal. 2012, 2012, 428983. [Google Scholar] [CrossRef]
- Farid, G. Some Riemann–Liouville fractional integral inequalities for convex functions. J. Anal. 2019, 27, 1095–1102. [Google Scholar] [CrossRef]
- Awan, M.U.; Talib, S.; Chu, Y.M.; Noor, M.A.; Noor, K.I. Some New Refinements of Hermite–Hadamard-Type Inequalities Involving ψk-Riemann–Liouville Fractional Integrals and Applications. Hindawi Math. Prob. Eng. 2020, 2020, 3051920. [Google Scholar] [CrossRef]
- Sontakke, B.R.; Shaikh, A. Properties of Caputo Operator and Its Applications to Linear Fractional Differential Equations. Int. J. Eng. Res. Appl. 2015, 5, 22–27. [Google Scholar]
- Kilbas, A.A.; Srivastava, H.M.; Trujillo, J.J. Theory and Applications of Fractional Differential Equations, 1st ed.; Elsevier Science: Amsterdam, The Netherlands, 2006. [Google Scholar]
- Alaoui, M.K.; Nonlaopon, K.; Zidan, A.M.; Khan, A.; Shah, R. Analytical Investigation of Fractional-Order Cahn–Hilliard and Gardner Equations Using Two Novel Techniques. Mathematics 2022, 10, 1643. [Google Scholar] [CrossRef]
- Korpinar, Z.; Inc, M.; Baleanu, D.; Bayram, M. Theory and application for the time fractional Gardner equation with Mittag-Leffler kernel. J. Taibah Univ. Sci. 2019, 13, 813–819. [Google Scholar] [CrossRef]
- Sartanpara, P.P.; Meher, R.; Meher, S.K. The generalized time-fractional Fornberg–Whitham equation: An analytic approach. Partial Differ. Equ. Appl. Math. 2022, 5, 100350. [Google Scholar] [CrossRef]
- Alderremy, A.A.; Khan, H.; Shah, R.; Aly, S.; Baleanu, D. The Analytical Analysis of Time-Fractional Fornberg–Whitham Equations. Mathematics 2020, 8, 987. [Google Scholar] [CrossRef]
- Gupta, P.K.; Singh, M. Homotopy perturbation method for fractional Fornberg–Whitham equation. Comput. Math. Appl. 2011, 61, 250–254. [Google Scholar] [CrossRef]
- El-Ajou, A.; Arqub, O.A.; Momani, S. Approximate analytical solution of the nonlinear fractional KdV–Burgers equation: A new iterative algorithm. J. Comput. Phys. 2015, 293, 81–95. [Google Scholar] [CrossRef]
- Saad, K.M.; Al-Sharif, E.H.F. Analytical study for time and time-space fractional Burgers’ equation. Adv. Differ. Equ. 2017, 2017, 300. [Google Scholar] [CrossRef] [Green Version]
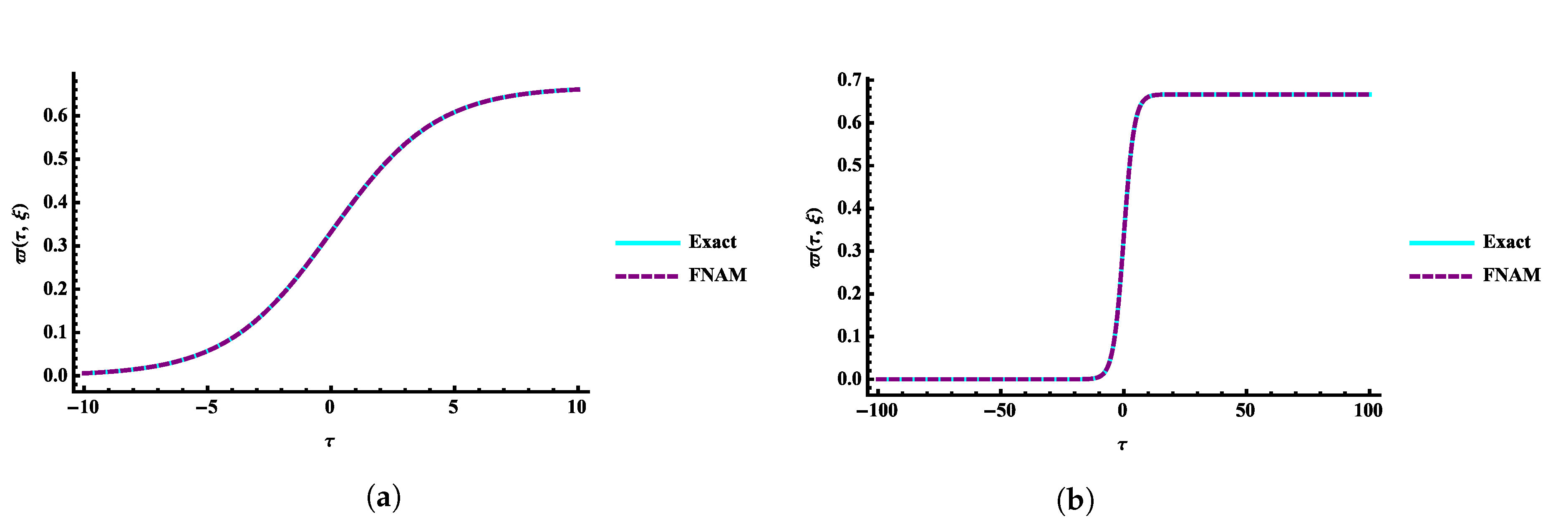
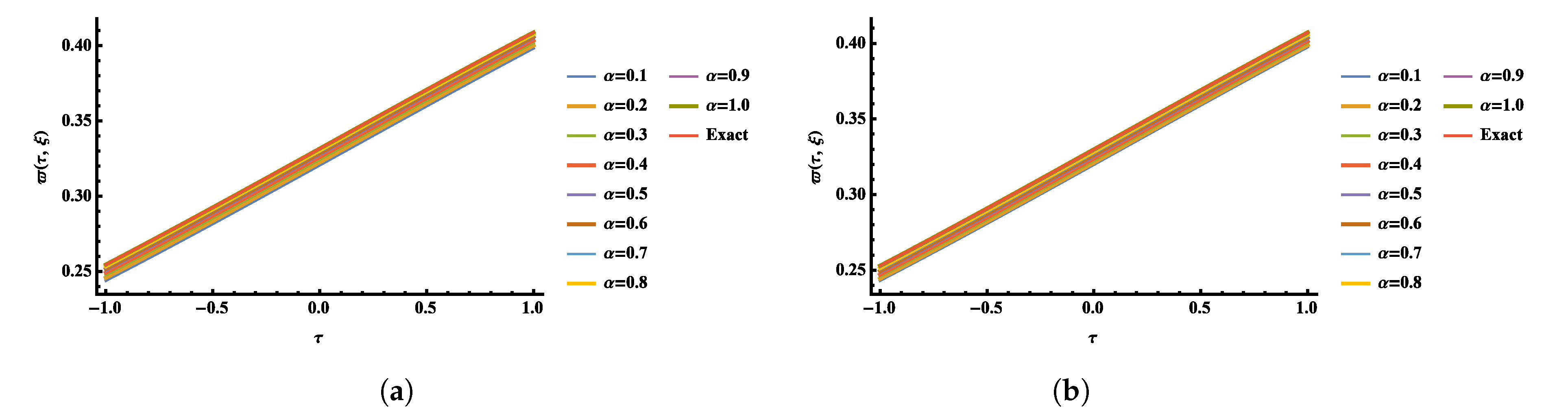

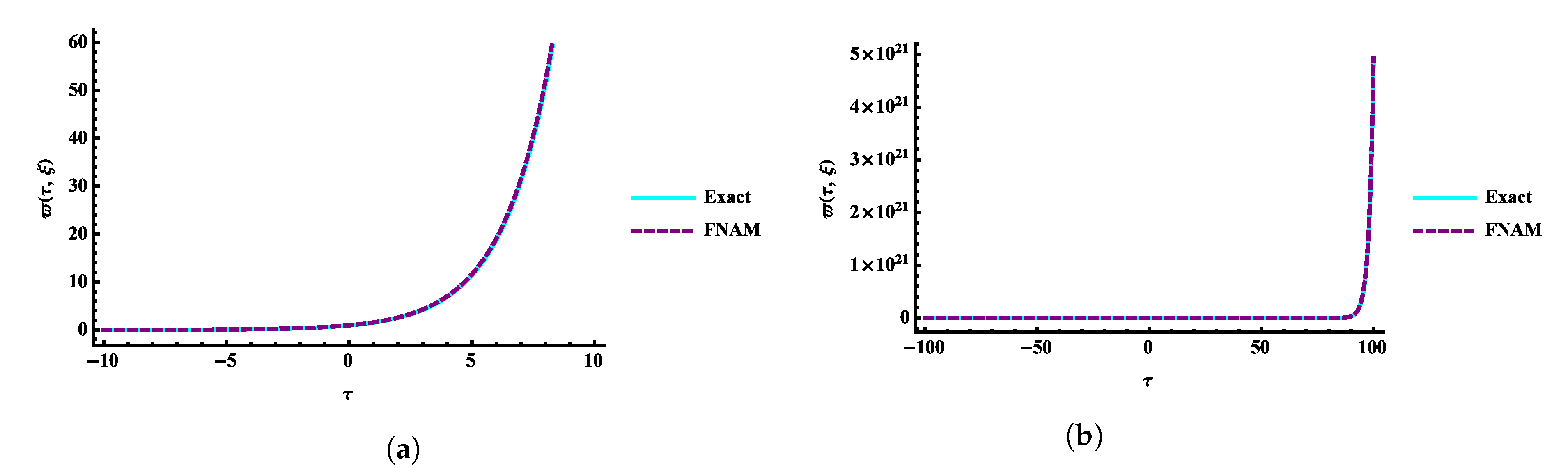
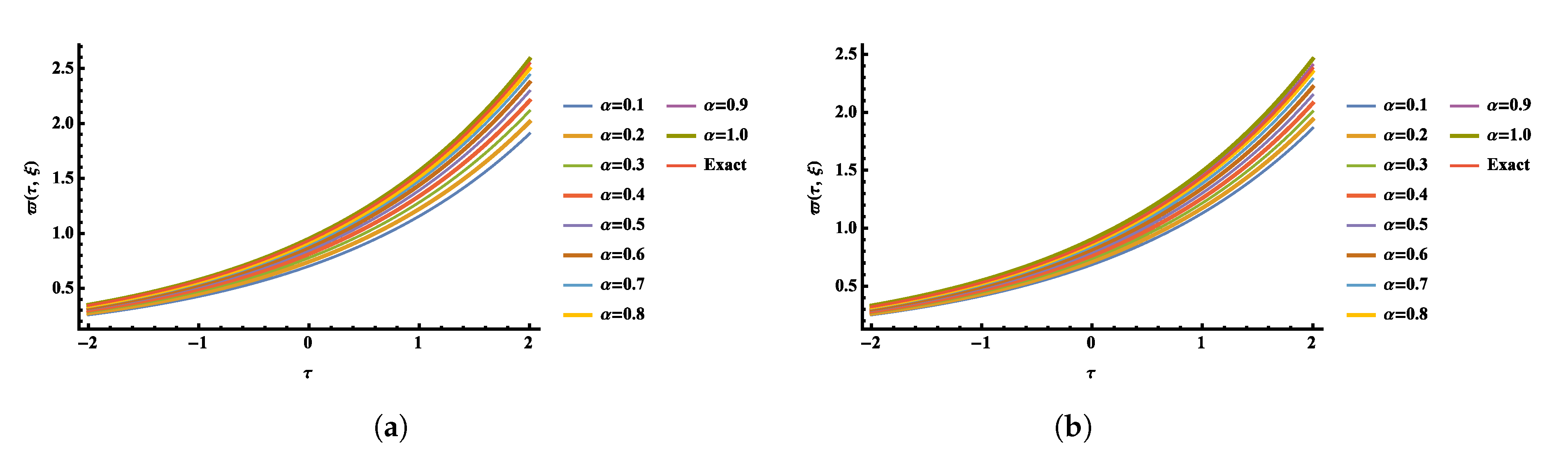


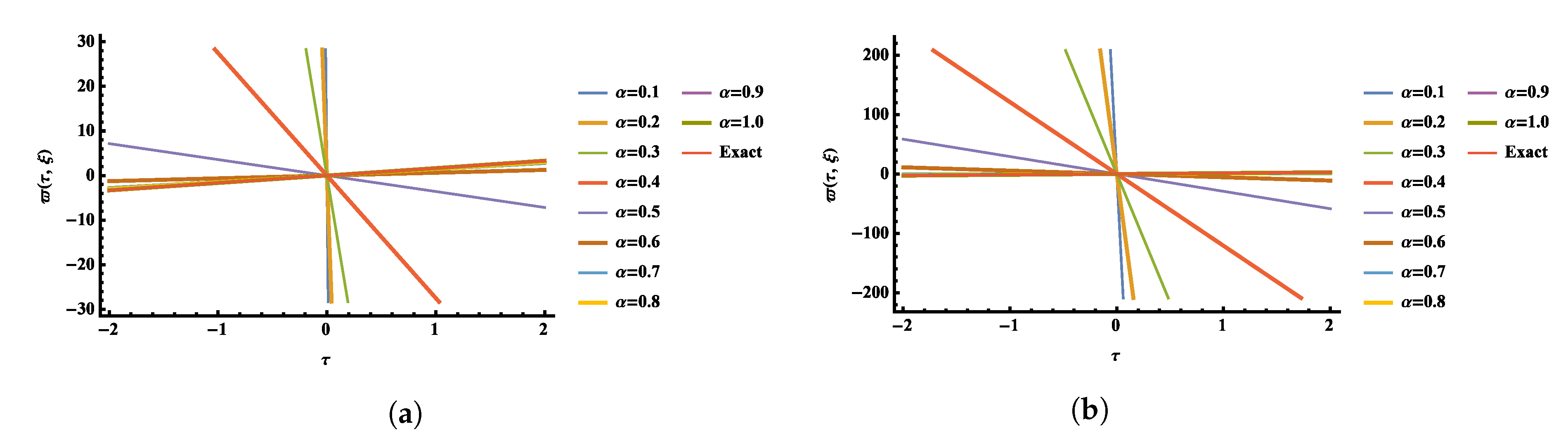



Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sultana, M.; Arshad, U.; Abdel-Aty, A.-H.; Akgül, A.; Mahmoud, M.; Eleuch, H. New Numerical Approach of Solving Highly Nonlinear Fractional Partial Differential Equations via Fractional Novel Analytical Method. Fractal Fract. 2022, 6, 512. https://doi.org/10.3390/fractalfract6090512
Sultana M, Arshad U, Abdel-Aty A-H, Akgül A, Mahmoud M, Eleuch H. New Numerical Approach of Solving Highly Nonlinear Fractional Partial Differential Equations via Fractional Novel Analytical Method. Fractal and Fractional. 2022; 6(9):512. https://doi.org/10.3390/fractalfract6090512
Chicago/Turabian StyleSultana, Mariam, Uroosa Arshad, Abdel-Haleem Abdel-Aty, Ali Akgül, Mona Mahmoud, and Hichem Eleuch. 2022. "New Numerical Approach of Solving Highly Nonlinear Fractional Partial Differential Equations via Fractional Novel Analytical Method" Fractal and Fractional 6, no. 9: 512. https://doi.org/10.3390/fractalfract6090512
APA StyleSultana, M., Arshad, U., Abdel-Aty, A.-H., Akgül, A., Mahmoud, M., & Eleuch, H. (2022). New Numerical Approach of Solving Highly Nonlinear Fractional Partial Differential Equations via Fractional Novel Analytical Method. Fractal and Fractional, 6(9), 512. https://doi.org/10.3390/fractalfract6090512








