Simultaneous Identification of Volatility and Mean-Reverting Parameter for European Option under Fractional CKLS Model
Abstract
:1. Introduction
2. Problem Formulation
3. Problem Regularization
3.1. Existence and Stability of the Solutions of the Minimization Problem
3.2. ADMM Algorithm for the Regularized Problem
4. Computational Experiments
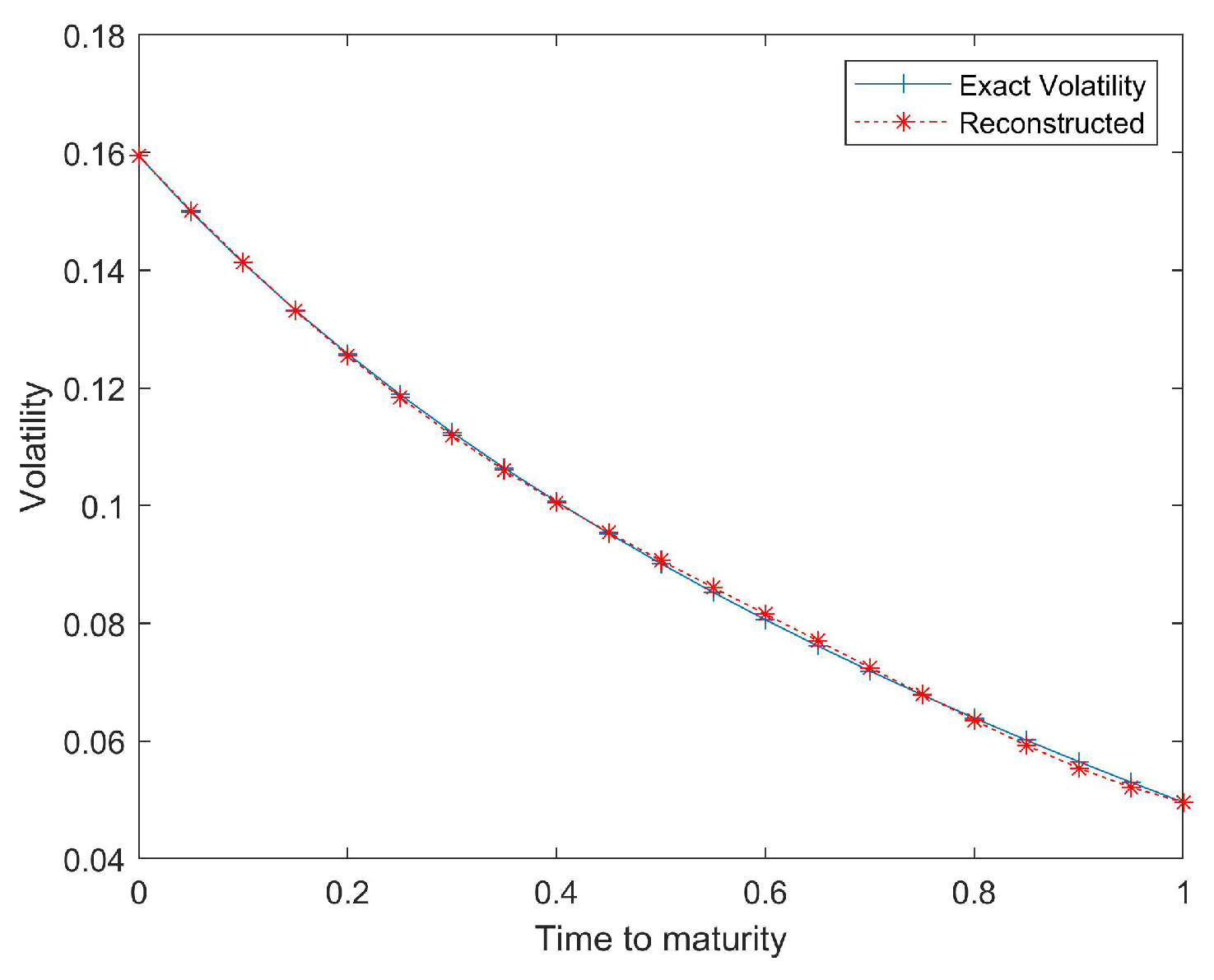
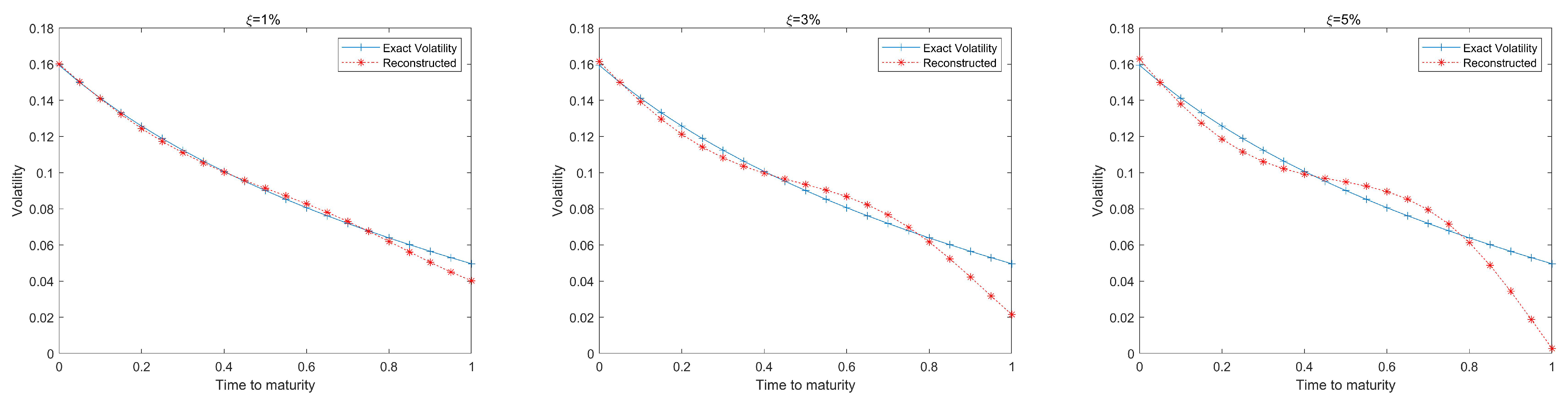
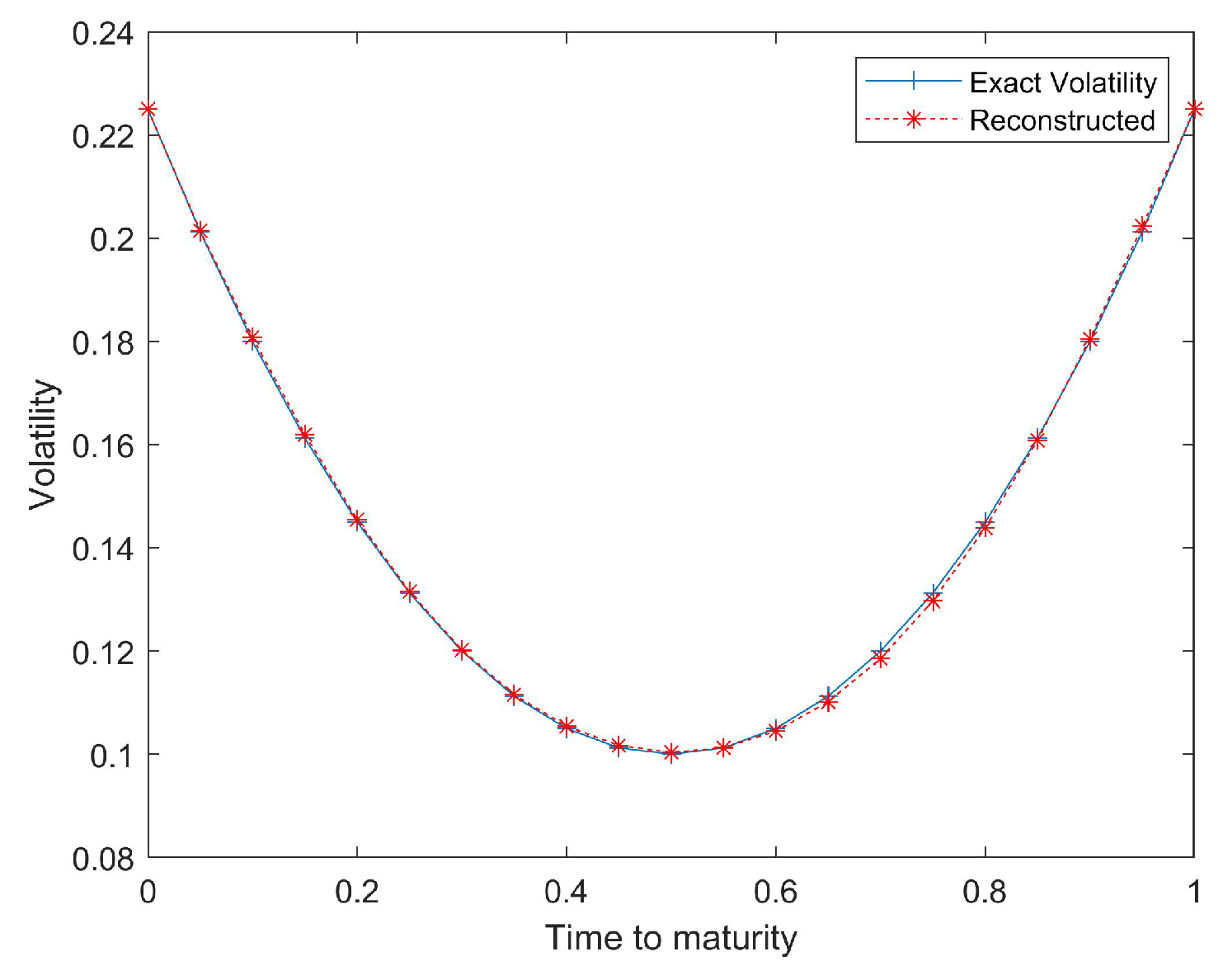
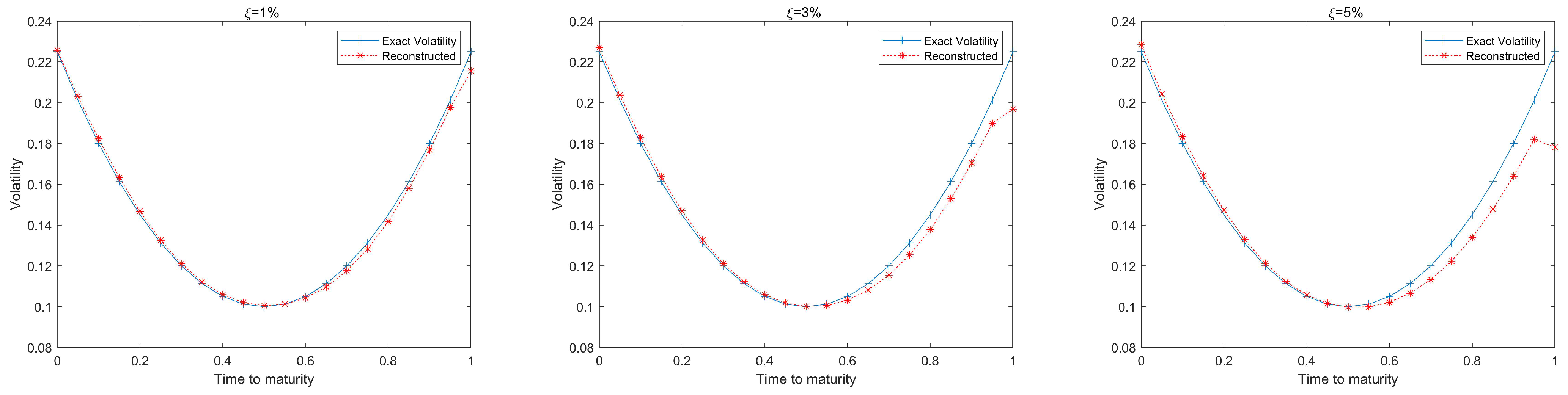
4.1. Numerical Simulation
4.2. Empirical Analysis
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Black, F.; Scholes, M. The pricing of option and corporate liabilities. J. Polit. Econ. 1973, 81, 637–654. [Google Scholar] [CrossRef] [Green Version]
- Mandelbrot, B.B.; Ness, J. Fractional brownian motions, fractional noises and applications. SIAM Rev. 1968, 10, 422–437. [Google Scholar] [CrossRef]
- Hu, Y.Z.; Øksendal, B. Fractional white noise calculus and applications to finance. Infin. Dimens. Anal. Quantum Probab. Relat. Top. 2003, 6, 1–32. [Google Scholar] [CrossRef]
- Necula, C. Option pricing in a fractional Brownian motion environment. Adv. Econ. Financ.-Res.—Dofin Work. Pap. Ser. 2008, 2, 259–273. [Google Scholar] [CrossRef]
- Huang, W.L.; Tao, X.X.; Li, S.H. Pricing Formulae for European Options under the Fractional Vasicek Interest Rate Model. Acta Math. Sin. 2012, 55, 219–230. [Google Scholar]
- Vasicek, O.A. An Equilibrium Characterization of the Term Structure. J. Financ. Econ. 1977, 5, 177–188. [Google Scholar] [CrossRef]
- Cox, J.C.; Ingersoll, J.E.; Ross, S.A. A theory of the term structure of interest rates. Econometrica 1985, 53, 385–407. [Google Scholar] [CrossRef]
- Cox, J.C. The constant elasticity of variance option pricing model. J. Portf. Manag. 1996, 23, 15–17. [Google Scholar] [CrossRef]
- Chan, K.C.; Karolyi, G.A.; Longstaff, F.A.; Sanders, A.B. An empirical comparison of alternative models of the short term interest rate. J. Financ. 1992, 47, 1209–1227. [Google Scholar] [CrossRef]
- Brennan, M.J.; Schwartz, E.S. Analyzing convertible bonds. J. Financ. Quant. Anal. 1980, 15, 907–929. [Google Scholar] [CrossRef]
- Glasserman, P. Monte Carlo Methods in Financial Engineering; Springer: Berlin, Germany, 2013. [Google Scholar]
- Choi, Y.; Wirjanto, T.S. An analytic approximation formula for pricing zero-coupon bonds. Financ. Res. Lett. 2007, 4, 116–126. [Google Scholar] [CrossRef]
- Stehlíková, B. A simple analytic approximation formula for the bond price in the Chan-Karolyi-Longstaff-Sanders model. Int. J. Numer. Anal. Model. Ser. B 2013, 4, 224–234. [Google Scholar]
- Stehlíková, B.; Ševcovič, D. Approximate formulae for pricing zero-coupon bonds and their asymptotic analysis. Int. J. Numer. Anal. Model. 2009, 6, 274–283. [Google Scholar]
- Nowman, K.B.; Sorwar, G. Pricing UK and US securities within the CKLS model Further results. Int. Rev. Financ. Anal. 1999, 8, 235–245. [Google Scholar] [CrossRef]
- Nowman, K.B.; Sorwar, G. Derivative prices from interest rate models: Results for Canada, Hong Kong, and United States. Int. Rev. Financ. Anal. 2005, 14, 428–438. [Google Scholar] [CrossRef]
- Mao, X.R.; Truman, A.; Yuan, C. Euler–Maruyama approximations in mean-reverting stochastic volatility model under regime-switching. J. Appl. Math. Stoch. Anal. 2006, 7, 1–20. [Google Scholar] [CrossRef]
- Wu, F.K.; Mao, X.R.; Chen, K. A highly sensitive mean-reverting process in finance and the Euler–Maruyama approximations. J. Math. Anal. Appl. 2008, 348, 540–554. [Google Scholar] [CrossRef] [Green Version]
- Marie, N. A generalized mean-reverting equation and applications. ESAIM Probab. Stat. 2014, 18, 799–828. [Google Scholar] [CrossRef] [Green Version]
- Kubilius, K.; Medžiūnas, A. Positive solutions of the fractional SDEs with fon-lipschitz diffusion coefficient. Mathematics 2021, 9, 18. [Google Scholar] [CrossRef]
- Jiang, L.S.; Tao, Y.S. Identifying the volatility of underlying assets from option prices. Inverse Probl. 2001, 17, 137–155. [Google Scholar]
- Xu, Z.L.; Jia, X.Y. The calibration of volatility for option pricing models with jump diffusion processes. Appl. Anal. 2019, 98, 810–827. [Google Scholar] [CrossRef]
- Zhao, J.J.; Xu, Z.L. Calibration of time-dependent volatility for European options under the fractional Vasicek model. AIMS Math. 2022, 7, 11053–11069. [Google Scholar] [CrossRef]
- Cristofol, M.; Roques, L. Simultaneous determination of the drift and diffusion coefficients in stochastic differential equations. Inverse Probl. 2017, 33, 095006. [Google Scholar] [CrossRef] [Green Version]
- Naumova, V.; Pereverzyev, S.V. Multi-penalty regularization with a component-wise penalization. Inverse Probl. 2013, 29, 075002. [Google Scholar] [CrossRef]
- Hofmann, C.; Hofmann, B.; Pichler, A. Simultaneous identification of volatility and interest rate functions-a two-parameter regularization approach. Electron. Trans. Numer. Anal. 2019, 51, 99–117. [Google Scholar] [CrossRef]
- Georgiev, S.G.; Vulkov, L.G. Simultaneous identification of time-dependent volatility and interest rate for European options. Electron. Trans. Numer. Anal. 2020, 2333, 1–8. [Google Scholar] [CrossRef]
- Haentjens, T.; Hout, K. ADI finite difference schemes for the Heston-Hull-White PDE. J. Comput. Financ. 2012, 16, 83–110. [Google Scholar] [CrossRef]
- Boyd, S.; Parikh, N.; Chu, E.; Peleato, B.; Eckstein, J. Distributed Optimization and Statistical Learning via the Alternating Direction Method of Multipliers. Now Found. Trends 2010, 3, 1–122. [Google Scholar]
- Lagnado, R.; Osher, S. A technique for calibrating derivative security pricing models: Numerical solution of an inverse problem. J. Comput. Financ. 1997, 1, 13–25. [Google Scholar] [CrossRef]
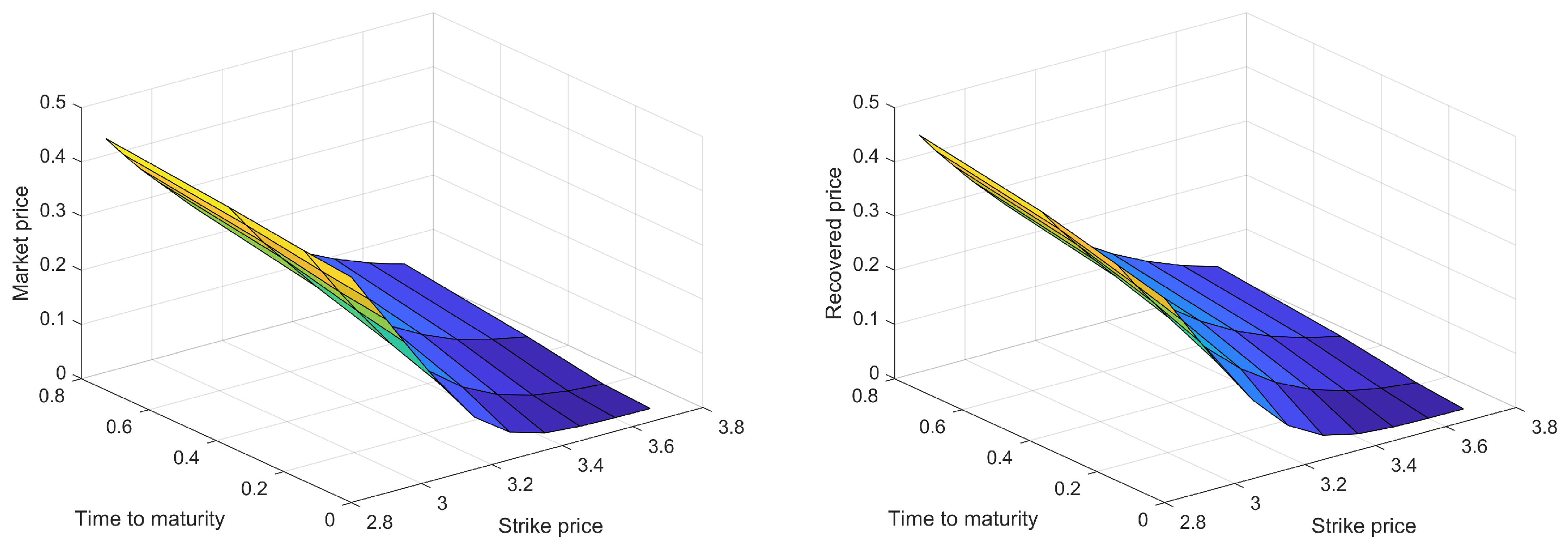
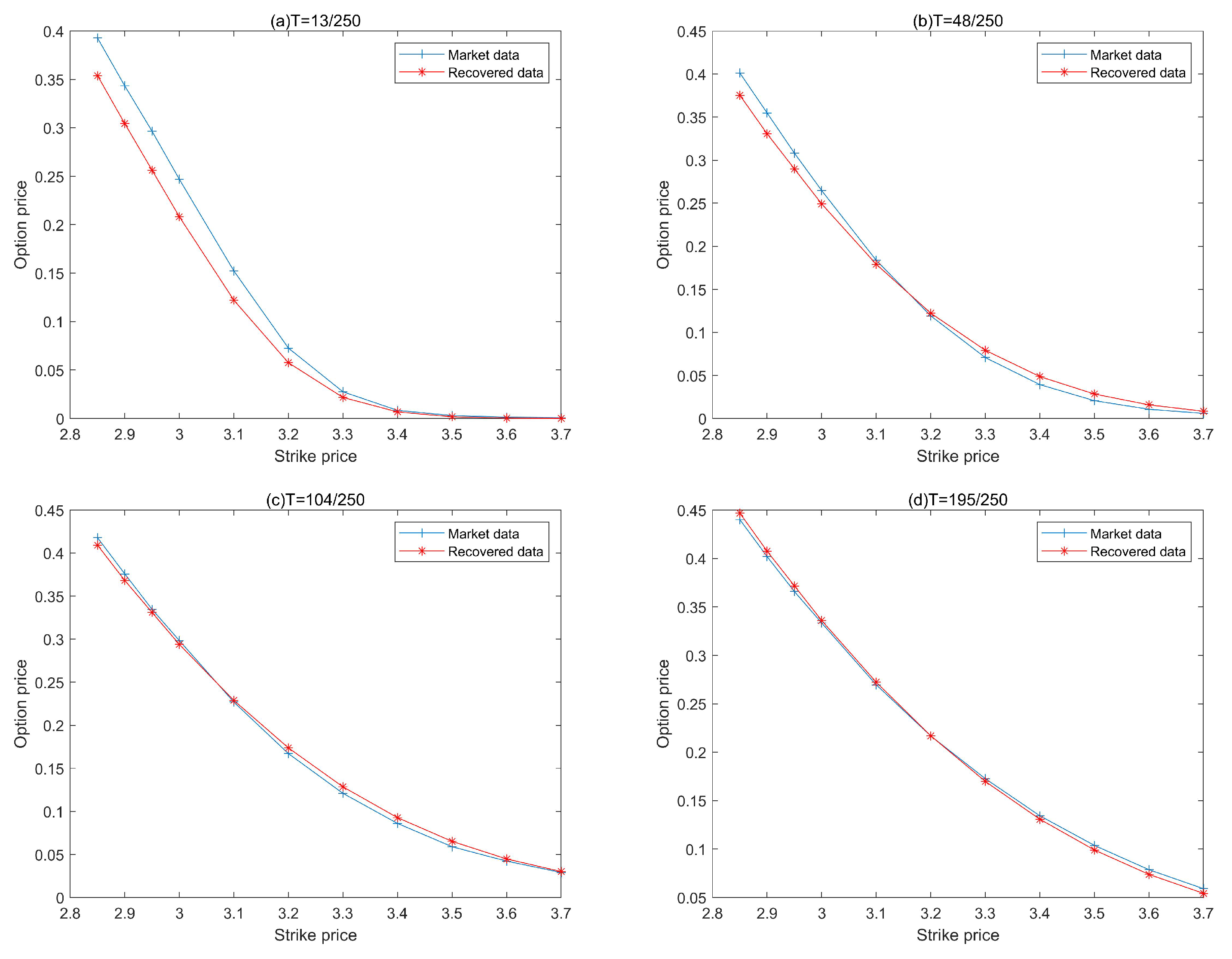
| K | 64 | 68 | 72 | 76 | 80 |
|---|---|---|---|---|---|
| 1.4032 | 0.4094 | 0.0917 | 0.0165 | 0.0025 | |
| 2.5149 | 1.1465 | 0.4555 | 0.1600 | 0.0506 |
| RMSE | AE | ||||
|---|---|---|---|---|---|
| 1.9305 × 10 | 5.7762 × 10 | 1.0623 × 10 | 1.327 | 0.0056 | |
| 3.8220 × 10 | 3.0564 × 10 | 9.3685 × 10 | 1.171 | 0.1504 | |
| 7.7861 × 10 | 8.1093 × 10 | 2.8106 × 10 | 1.028 | 0.2934 | |
| 1.2144 × 10 | 1.1219 × 10 | 4.6803 × 10 | 0.9512 | 0.3702 |
| RMSE | AE | ||||
|---|---|---|---|---|---|
| 7.7267 × 10 | 7.7984 × 10 | 1.6680 × 10 | 0.802 | 0.002 | |
| 7.6843 × 10 | 9.3685 × 10 | 2.1668 × 10 | 0.811 | 0.011 | |
| 7.5211 × 10 | 5.2394 × 10 | 2.8106 × 10 | 0.754 | 0.046 | |
| 7.4837 × 10 | 8.4450 × 10 | 4.6843 × 10 | 0.712 | 0.088 |
| Strike Price K | ||||
|---|---|---|---|---|
| 2.85 | 0.3928 | 0.4013 | 0.4179 | 0.4401 |
| 2.90 | 0.3434 | 0.3548 | 0.3758 | 0.4019 |
| 2.95 | 0.2966 | 0.3082 | 0.3347 | 0.3659 |
| 3.00 | 0.2470 | 0.2647 | 0.2984 | 0.3334 |
| 3.10 | 0.1523 | 0.1837 | 0.2269 | 0.2695 |
| 3.20 | 0.0726 | 0.1192 | 0.1671 | 0.2167 |
| 3.30 | 0.0275 | 0.0707 | 0.1210 | 0.1727 |
| 3.40 | 0.0084 | 0.0393 | 0.0860 | 0.1343 |
| 3.50 | 0.0030 | 0.0207 | 0.0590 | 0.1038 |
| 3.60 | 0.0014 | 0.0107 | 0.0422 | 0.0787 |
| 3.70 | 0.0008 | 0.0059 | 0.0290 | 0.0591 |
| RMSE | ||||
|---|---|---|---|---|
| Empirical results | 4.99 × 10 | 0.0151 | 0.0402 | 0.8451 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhao, J.; Xu, Z. Simultaneous Identification of Volatility and Mean-Reverting Parameter for European Option under Fractional CKLS Model. Fractal Fract. 2022, 6, 344. https://doi.org/10.3390/fractalfract6070344
Zhao J, Xu Z. Simultaneous Identification of Volatility and Mean-Reverting Parameter for European Option under Fractional CKLS Model. Fractal and Fractional. 2022; 6(7):344. https://doi.org/10.3390/fractalfract6070344
Chicago/Turabian StyleZhao, Jiajia, and Zuoliang Xu. 2022. "Simultaneous Identification of Volatility and Mean-Reverting Parameter for European Option under Fractional CKLS Model" Fractal and Fractional 6, no. 7: 344. https://doi.org/10.3390/fractalfract6070344
APA StyleZhao, J., & Xu, Z. (2022). Simultaneous Identification of Volatility and Mean-Reverting Parameter for European Option under Fractional CKLS Model. Fractal and Fractional, 6(7), 344. https://doi.org/10.3390/fractalfract6070344





