The Oscillatory Flow of Oldroyd-B Fluid with Magnetic Disturbance
Abstract
:1. Introduction
2. Mathematical Model
3. Numerical Discretization
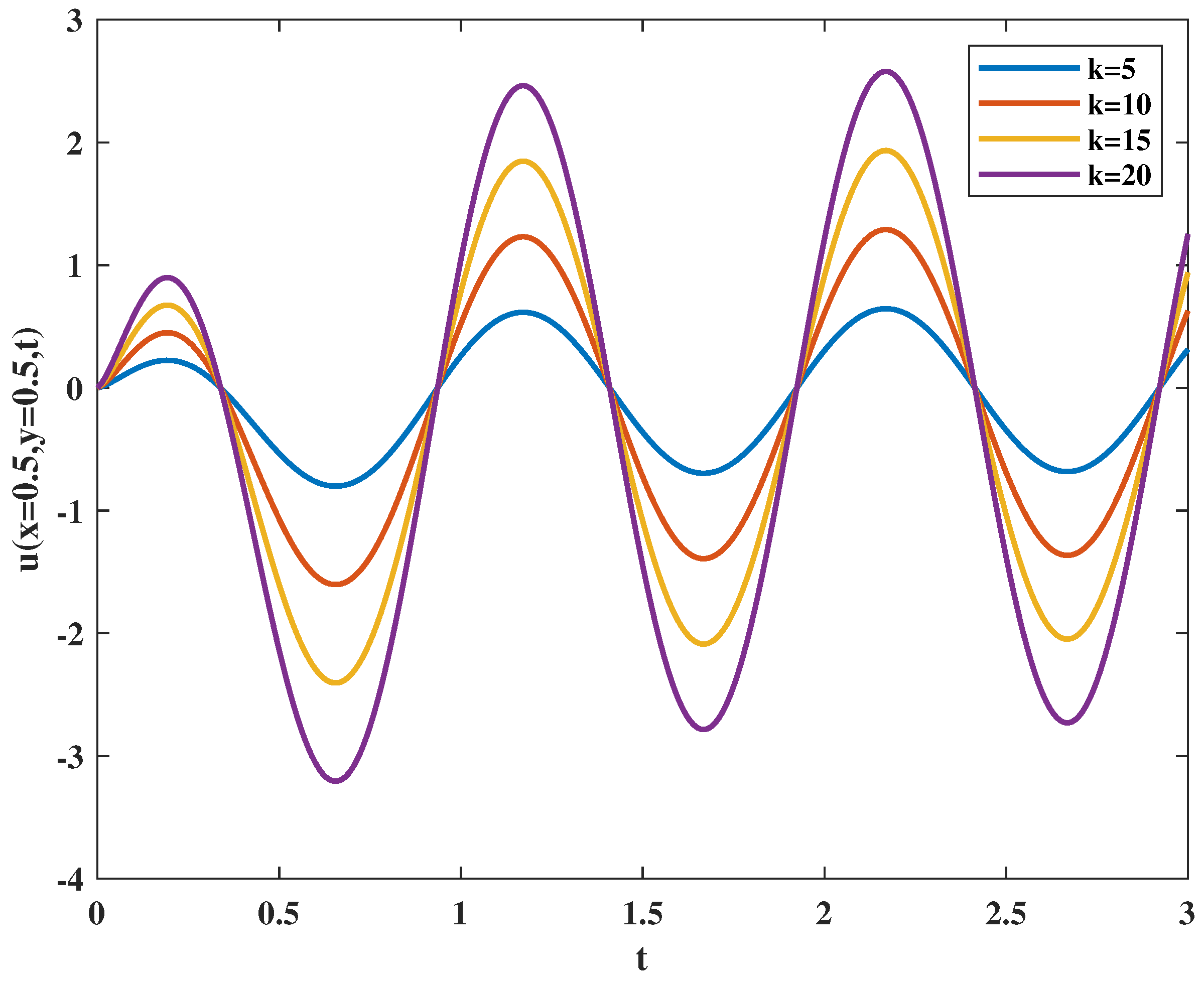
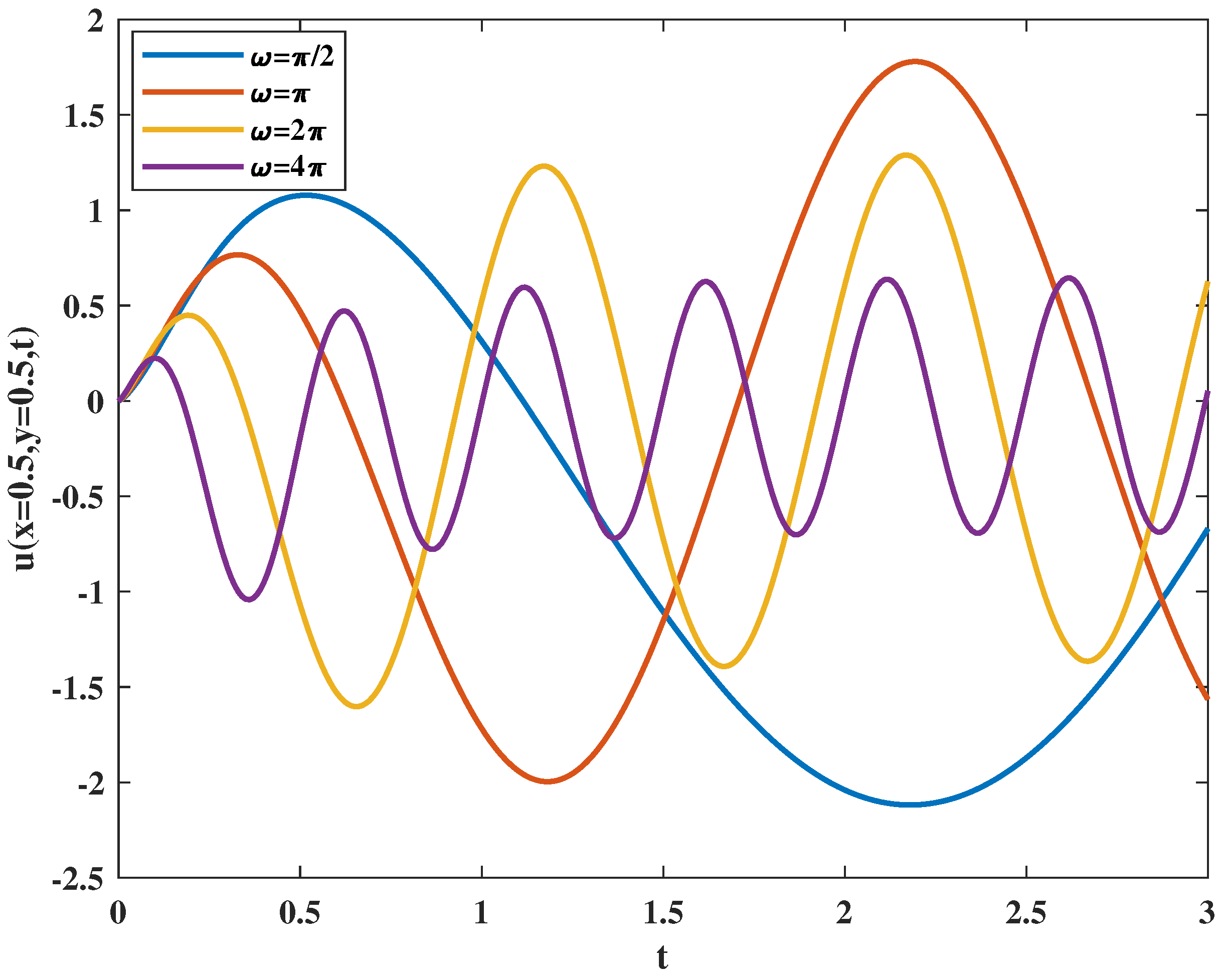
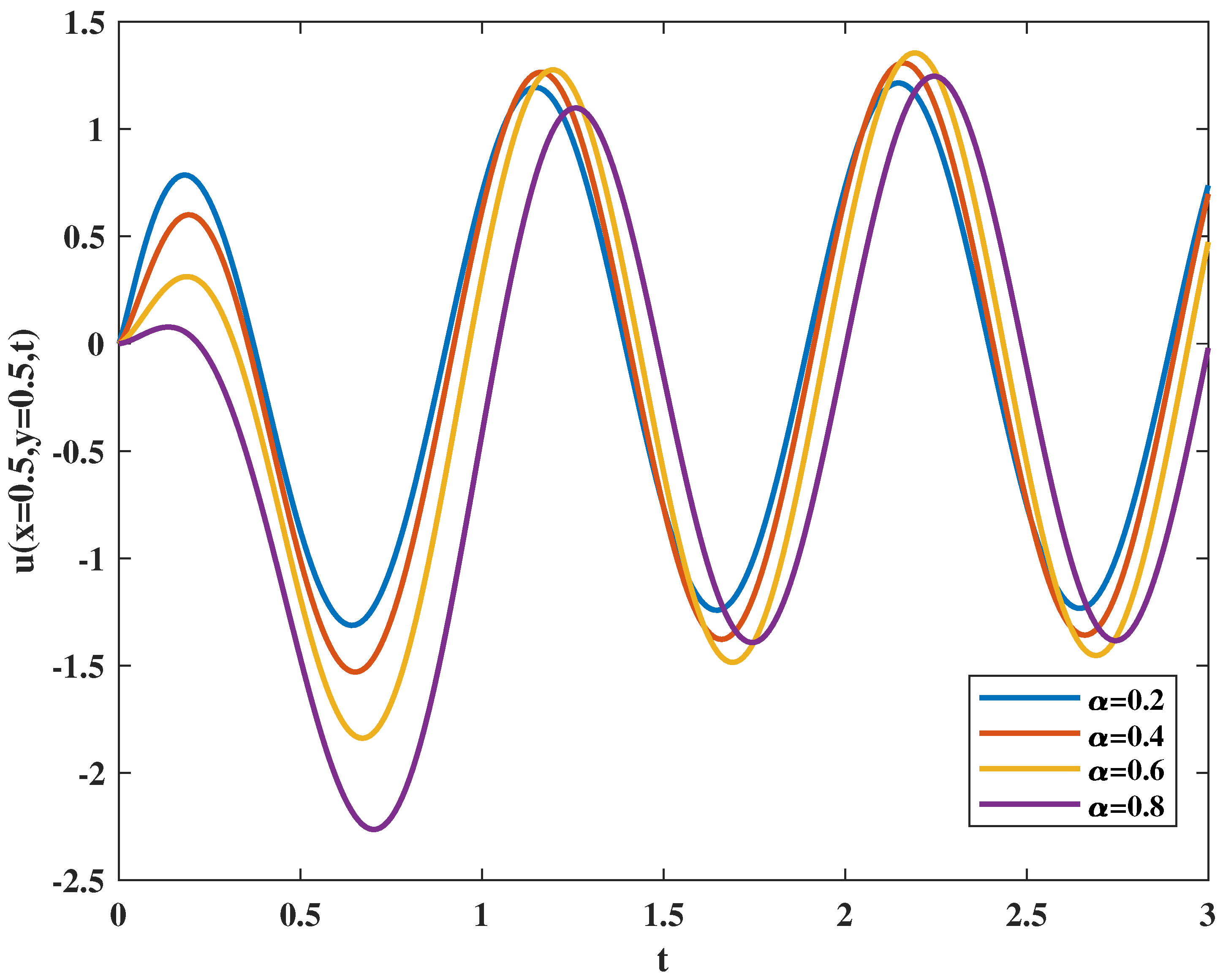
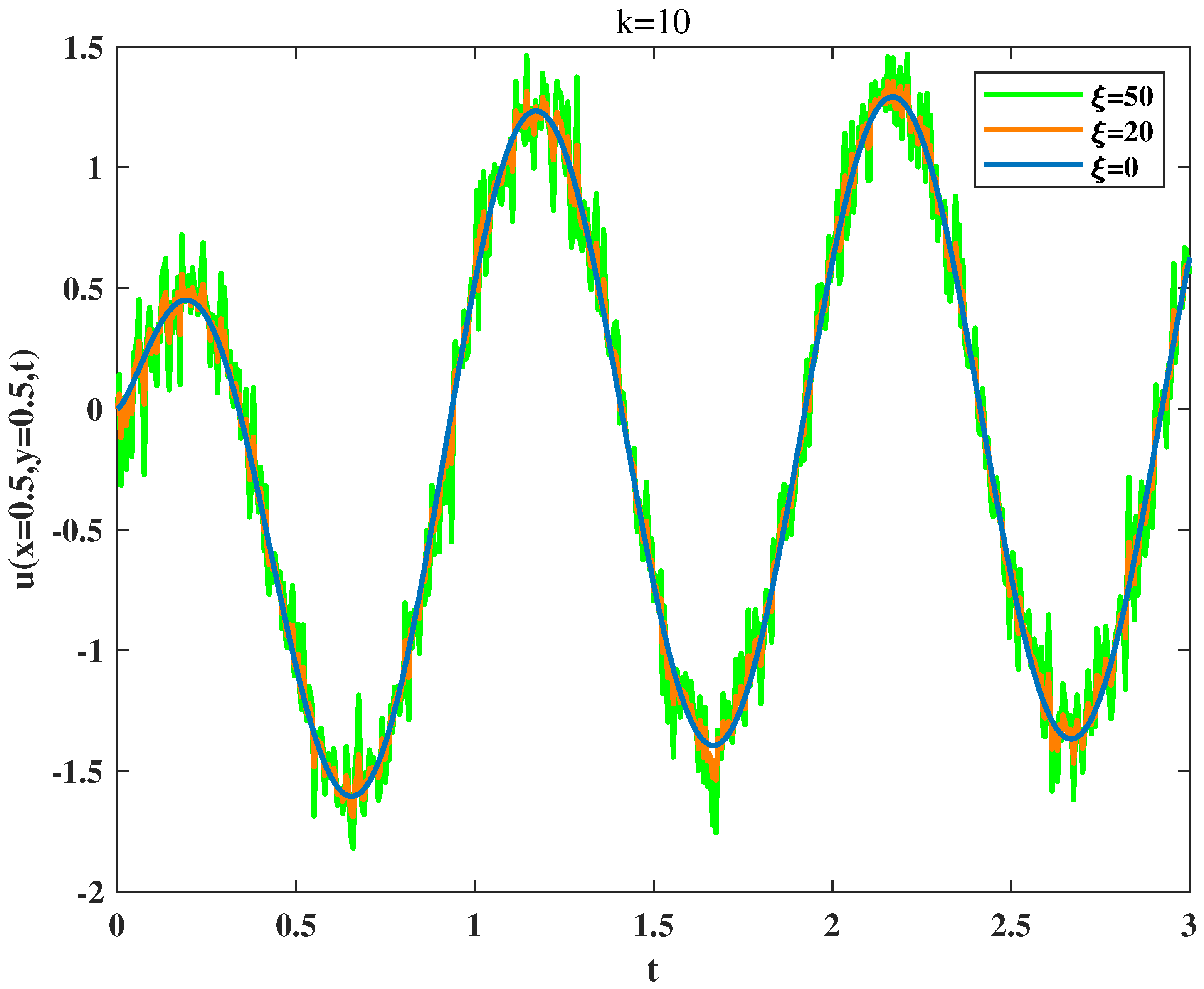
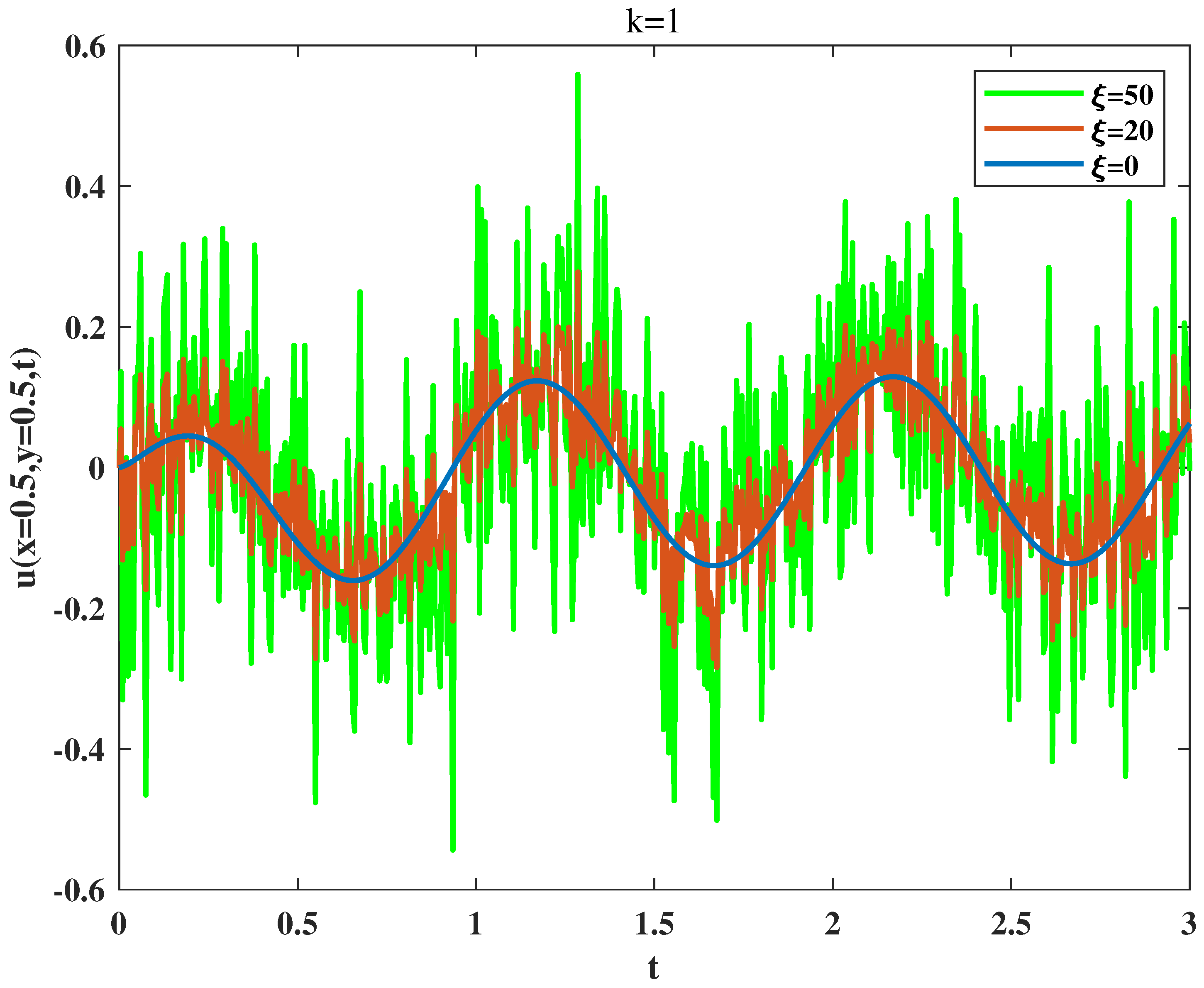
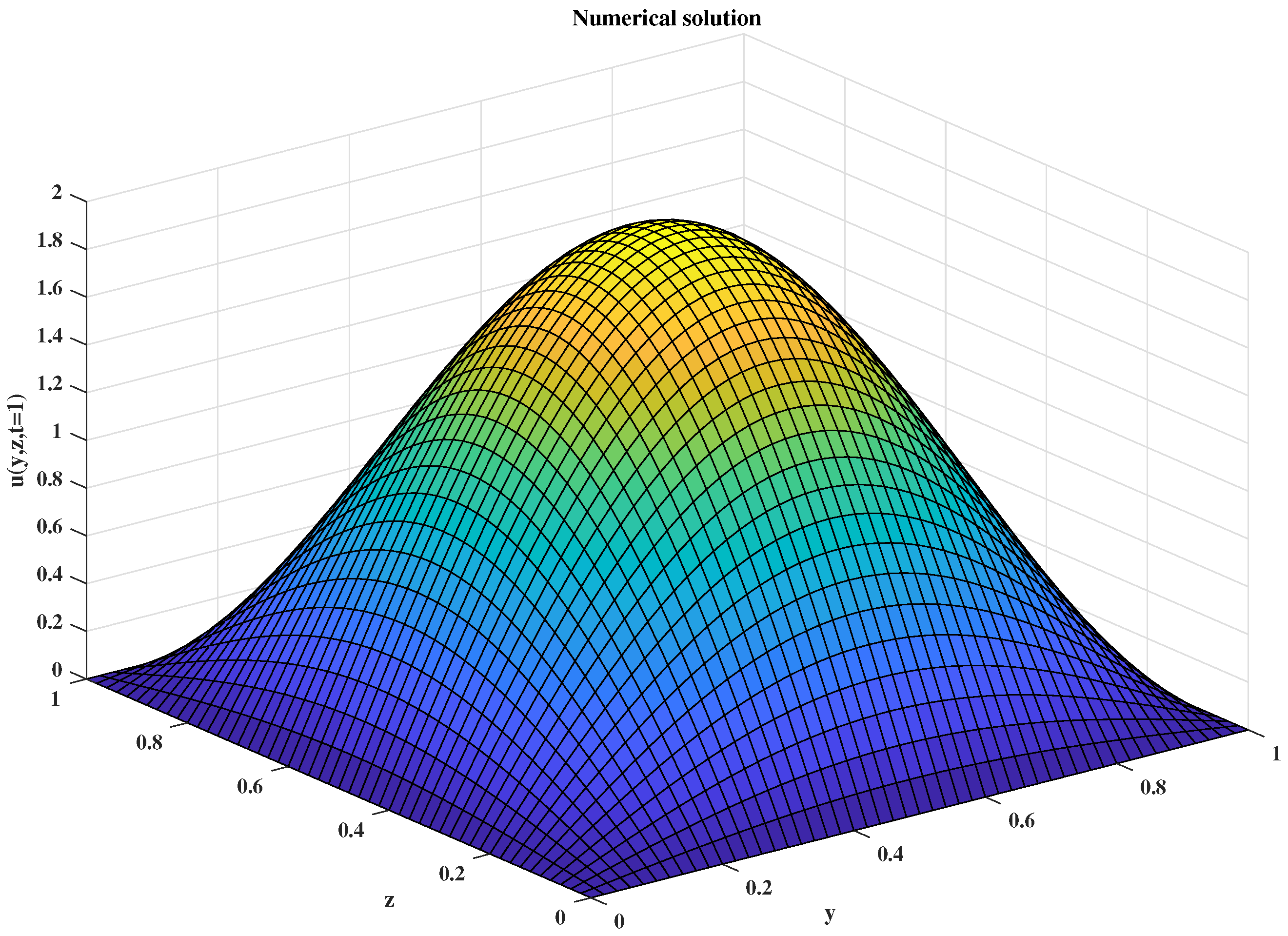
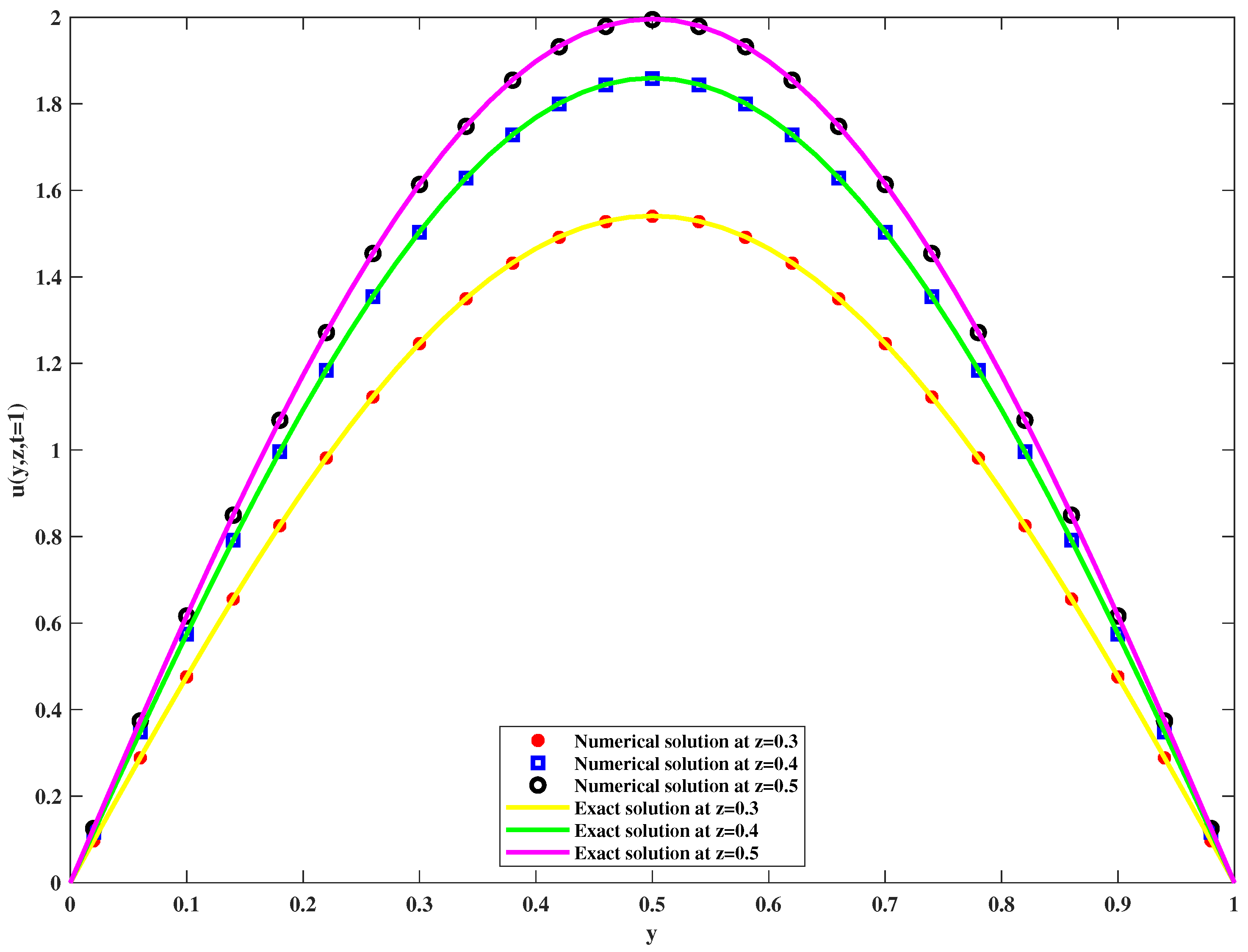
4. Results and Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Symbols
| ∇ | gradient operator | V | viscoelastic fluid velocity |
| constant density of the fluid | pressure gradient | ||
| S | the extra-stress tensor | body force | |
| retardation time | dynamic viscosity of the fluid | ||
| order of the fractional derivative | first Rivlin–Ericksen tensor | ||
| dynamic viscosity coefficient of fluid | magnetic field intensity | ||
| perturbation factor | electrical conductivity of the fluid | ||
| Laplace operator | k | amplitude of the pressure gradient | |
| periodic of the pressure gradient | M | magnetic parameter | |
| intensity of noise | W | Wiener process |
References
- Sun, H.G.; Yong, Z.; Baleanu, D.; Wen, C.; Chen, Y.Q. A new collection of real world applications of fractional calculus in science and engineering. Commun. Nonlinear Sci. Numer. Simul. 2018, 64, 213–231. [Google Scholar] [CrossRef]
- Rasheed, A.; Anwar, M.S. Simulations of variable concentration aspects in a fractional nonlinear viscoelastic fluid flow. Commun. Nonlinear Sci. Numer. Simul. 2018, 65, 216–230. [Google Scholar] [CrossRef]
- Tanner, R.I. Notes on the Rayleigh parallel problem for a viscoelastic fluid. Z. Angew. Math. Phys. 1962, 13, 573–580. [Google Scholar] [CrossRef]
- Guillop’E, C.; Saut, J.C. Existence results for the flow of viscoelastic fluids with a differential constitutive law. Nonlinear Anal. Theory Methods Appl. 1990, 15, 849–869. [Google Scholar] [CrossRef] [Green Version]
- Baranovskii, E.S.; Artemov, M.A. Global Existence Results for Oldroyd Fluids with Wall Slip. Acta Appl. Math. Int. J. Appl. Math. Math. Appl. 2017, 147, 197–210. [Google Scholar] [CrossRef]
- Baranovskii, E.S. Steady Flows of an Oldroyd Fluid with Threshold Slip. Commun. Pure Appl. Anal. 2019, 18, 735–750. [Google Scholar] [CrossRef] [Green Version]
- Shen, B.; Zheng, L.; Chen, S. Fractional boundary layer flow and radiation heat transfer of MHD viscoelastic fluid over an unsteady stretching surface. AIP Adv. 2015, 5, 107–133. [Google Scholar] [CrossRef] [Green Version]
- Zhang, Y.; Zhao, H.; Liu, F.; Bai, Y. Analytical and numerical solutions of the unsteady 2D flow of MHD fractional Maxwell fluid induced by variable pressure gradient. Comput. Math. Appl. 2017, 75, 965–980. [Google Scholar] [CrossRef]
- Feng, L.; Liu, F.; Turner, I. Finite difference/finite element method for a novel 2D multi-term time-fractional mixed sub-diffusion and diffusion-wave equation on convex domains. Commun. Nonlinear Sci. Numer. Simul. 2019, 70, 354–371. [Google Scholar] [CrossRef]
- Kumar, P.; Mohan, H.; Singh, G.J. Rayleigh-Taylor Instability of Rotating Oldroydian Viscoelastic Fluids in Porous Medium in Presence of a Variable Magnetic Field. Transp. Porous Media 2004, 56, 199–208. [Google Scholar] [CrossRef]
- Bhatti, M.M.; Ellahi, R.; Zeeshan, A. Study of variable magnetic field on the peristaltic flow of Jeffrey fluid in a non-uniform rectangular duct having compliant walls. J. Mol. Liq. 2016, 222, 101–108. [Google Scholar] [CrossRef]
- Sugama, H.; Okamoto, M.; Wakatani, M. Vlasov Equation in the Stochastic Magnetic Field. J. Phys. Soc. Jpn. 1992, 62, 514–523. [Google Scholar] [CrossRef]
- Park, J.K.; Boozer, A.H.; Menard, J.E.; Garofalo, A.M.; Schaffer, M.J.; Hawryluk, R.J.; Kaye, S.M.; Gerhardt, S.P.; Sabbagh, S.A.; Team, N. Importance of plasma response to nonaxisymmetric perturbations in tokamaksa). Phys. Plasmas 2009, 16, 056115. [Google Scholar] [CrossRef]
- Wang, Q.; Zha, X.; Lu, H.; Wang, X.; Wu, B.; Zhu, S. Numerical Modeling on Heat Transport Across Stochastic Magnetic Field. Contrib. Plasma Phys. 2016, 56, 830–836. [Google Scholar] [CrossRef]
- Xu, L.; Shen, T.; Yang, X.; Liang, J. Analysis of time fractional and space nonlocal stochastic incompressible Navier-Stokes equation driven by white noise. Comput. Math. Appl. 2019, 78, 1669–1680. [Google Scholar] [CrossRef]
- Razafimandimby, P.A. On Stochastic Models Describing the Motions of Randomly Forced Linear Viscoelastic Fluids. J. Inequalities Appl. 2010, 2010, 932053. [Google Scholar] [CrossRef] [Green Version]
- Mohan, M.T. Well posedness, large deviations and ergodicity of the stochastic 2D Oldroyd model of order one. Stoch. Process. Their Appl. 2020, 130, 4513–4562. [Google Scholar] [CrossRef]
- Manna, U.; Mukherjee, D. Strong Solutions of Stochastic Models for Viscoelastic Flows of Oldroyd Type. Nonlinear Anal. 2017, 165, 198–242. [Google Scholar] [CrossRef] [Green Version]
- Razafimandimby, P.A.; Sango, M. Strong solution for a stochastic model of two-dimensional second grade fluids: Existence, uniqueness and asymptotic behavior. Nonlinear Anal. 2012, 75, 4251–4270. [Google Scholar] [CrossRef] [Green Version]
- Cipriano, F.; Didier, P.; Guerra, S. Well-posedness of stochastic third grade fluid equation. J. Differ. Equations 2021, 285, 496–535. [Google Scholar] [CrossRef]
- Chen, J.; Chen, Z. Stochastic non-Newtonian fluid motion equations of a nonlinear bipolar viscous fluid. J. Math. Anal. Appl. 2010, 369, 486–509. [Google Scholar] [CrossRef] [Green Version]
- Doan, T.S.; Huong, P.T.; Kloeden, P.E.; Vu, A.M. Euler-Maruyama scheme for Caputo stochastic fractional differential equations. J. Comput. Appl. Math. 2020, 380, 112989. [Google Scholar] [CrossRef]
- Yang, Z.; Zheng, X.; Zang, H.; Wang, H. Strong convergence of Euler-Maruyama scheme to a variable-order fractional stochastic differential equation driven by a multiplicative white noise. Chaos Solitons Fractals 2020, 142, 110392. [Google Scholar] [CrossRef]
- Zhou, Y.; Wang, Q.; Zhang, Z. Physical Properties Preserving Numerical Simulation of Stochastic Fractional Nonlinear Wave Equation. Commun. Nonlinear Sci. Numer. Simul. 2021, 99, 105832. [Google Scholar] [CrossRef]
- Liu, X.; Yang, X. Mixed finite element method for the nonlinear time-fractional stochastic fourth-order reaction-diffusion equation. Comput. Math. Appl. 2021, 84, 39–55. [Google Scholar] [CrossRef]
- Li, J.; Liu, Q.; Yue, J. Numerical analysis of fully discrete finite element methods for the stochastic Navier-Stokes equations with multiplicative noise. Appl. Numer. Math. 2021, 170, 398–417. [Google Scholar] [CrossRef]
- Friedrich, C. Relaxation and retardation functions of the Maxwell model with fractional derivatives. Rheol. Acta 1991, 30, 151–158. [Google Scholar] [CrossRef]
- Song, D.Y.; Jiang, T.Q. Study on the constitutive equation with fractional derivative for the viscoelastic fluids—Modified Jeffreys model and its application. Rheol. Acta 1998, 37, 512–517. [Google Scholar] [CrossRef]
- Tong, D.; Zhang, X.; Zhang, X. Unsteady helical flows of a generalized Oldroyd-B fluid. J. Non-Newton. Fluid Mech. 2009, 156, 75–83. [Google Scholar] [CrossRef]
- Liu, Y.; Zhang, H.; Jiang, X. Fast evaluation for magnetohydrodynamic flow and heat transfer of fractional Oldroyd-B fluids between parallel plates. ZAMM J. Appl. Math. Mech. Z. Angew. Math. Mech. 2021, 101, e202100042. [Google Scholar] [CrossRef]
- Podlubny, I. Fractional Differential Equations. Math. Sci. Eng. 1999, 198, 41–117. [Google Scholar]
- Lord, G.J.; Powell, C.E.; Shardlow, T. An Introduction to Computational Stochastic PDEs; Cambridge University Press: Cambridge, UK, 2014. [Google Scholar]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yue, P.; Ming, C. The Oscillatory Flow of Oldroyd-B Fluid with Magnetic Disturbance. Fractal Fract. 2022, 6, 322. https://doi.org/10.3390/fractalfract6060322
Yue P, Ming C. The Oscillatory Flow of Oldroyd-B Fluid with Magnetic Disturbance. Fractal and Fractional. 2022; 6(6):322. https://doi.org/10.3390/fractalfract6060322
Chicago/Turabian StyleYue, Pujie, and Chunying Ming. 2022. "The Oscillatory Flow of Oldroyd-B Fluid with Magnetic Disturbance" Fractal and Fractional 6, no. 6: 322. https://doi.org/10.3390/fractalfract6060322
APA StyleYue, P., & Ming, C. (2022). The Oscillatory Flow of Oldroyd-B Fluid with Magnetic Disturbance. Fractal and Fractional, 6(6), 322. https://doi.org/10.3390/fractalfract6060322




