Dynamics of Different Nonlinearities to the Perturbed Nonlinear Schrödinger Equation via Solitary Wave Solutions with Numerical Simulation
Abstract
:1. Introduction
2. Model and Method Description
2.1. The Modified Extended tanh Expansion Method
2.2. Mathematical Analysis of the Model
3. Application of the Method with Different Nonlinear Medias
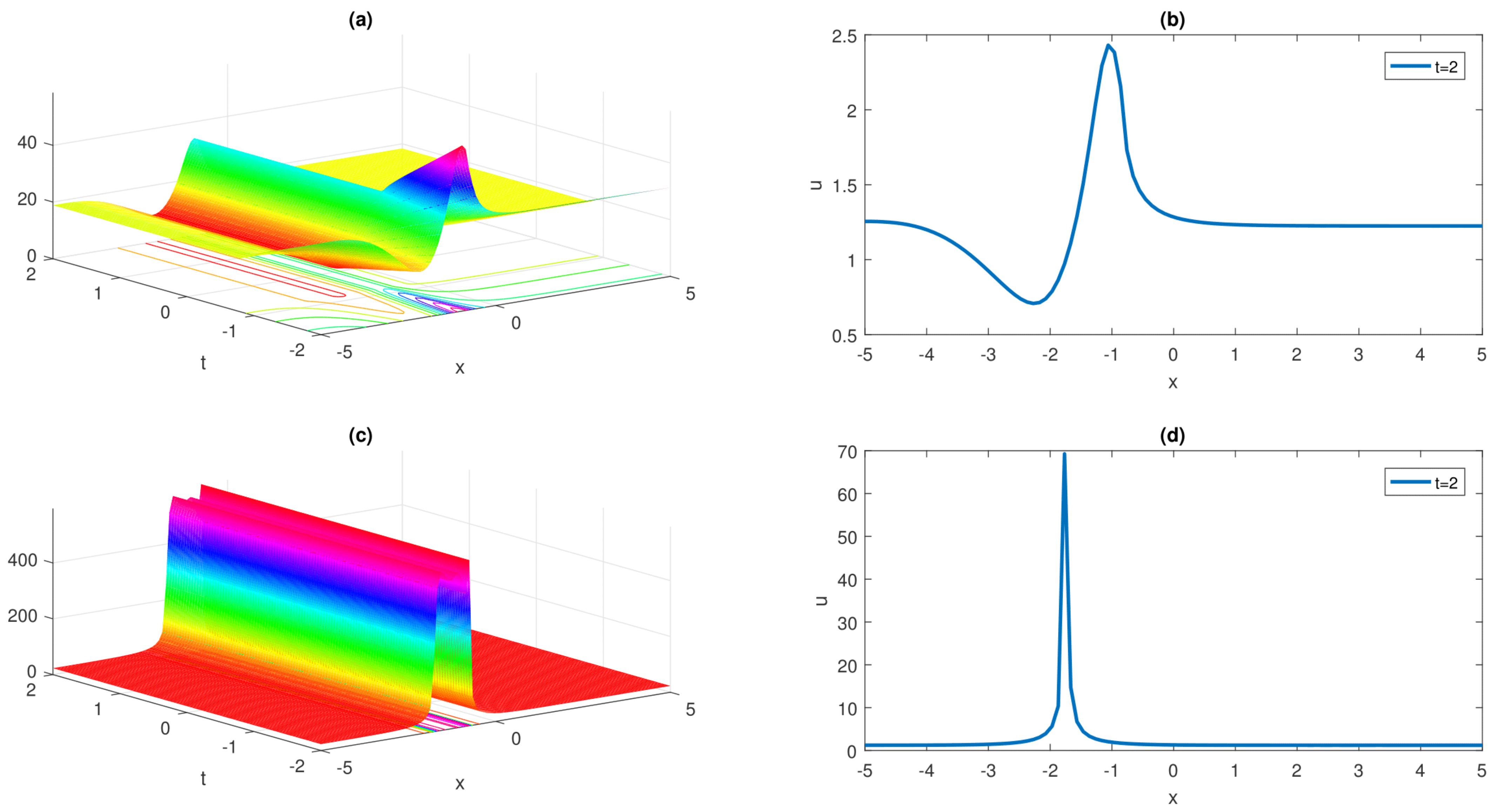
3.1. Kerr Law
3.2. Power Law
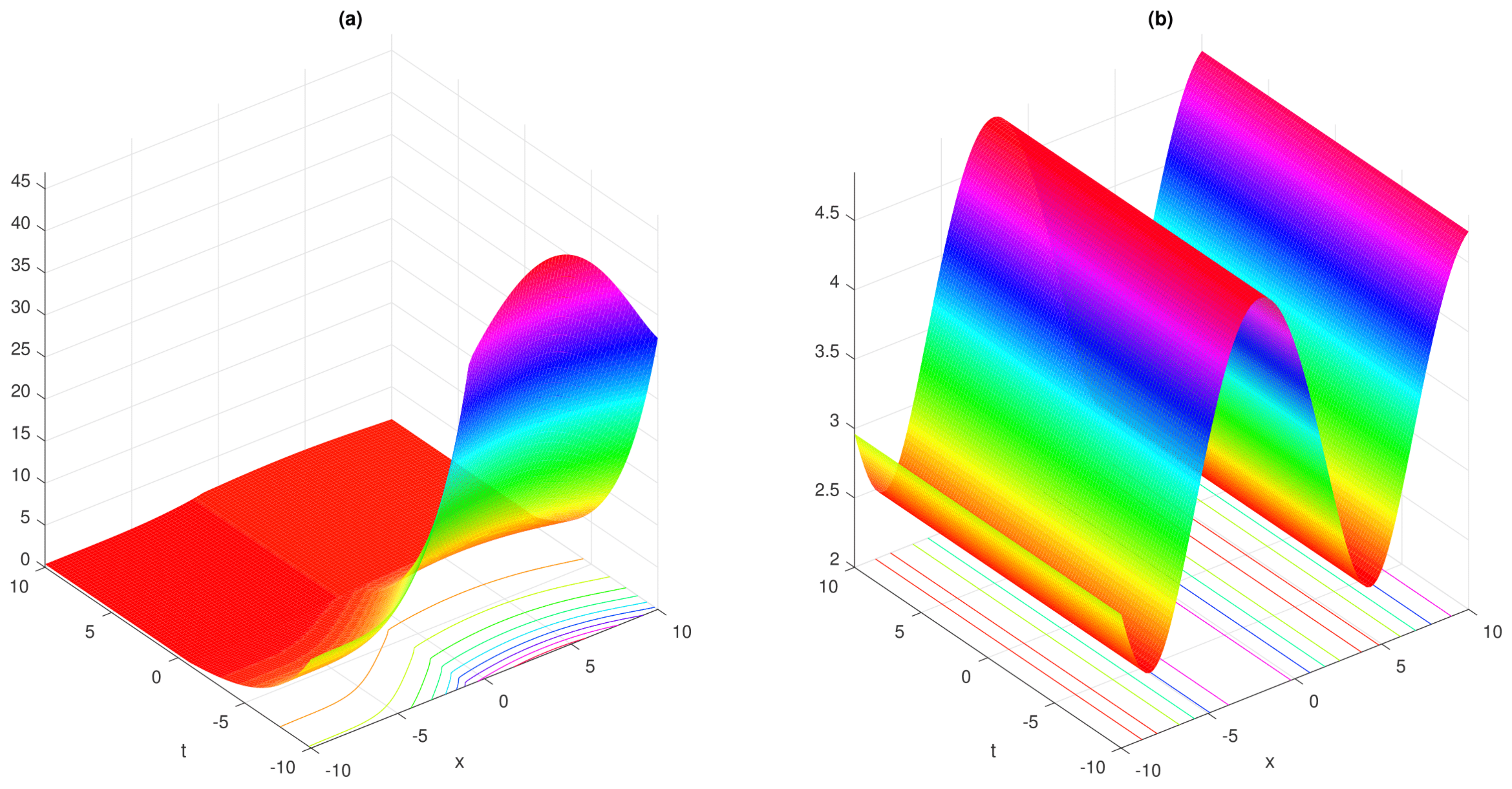
3.3. Quadratic-Cubic Law
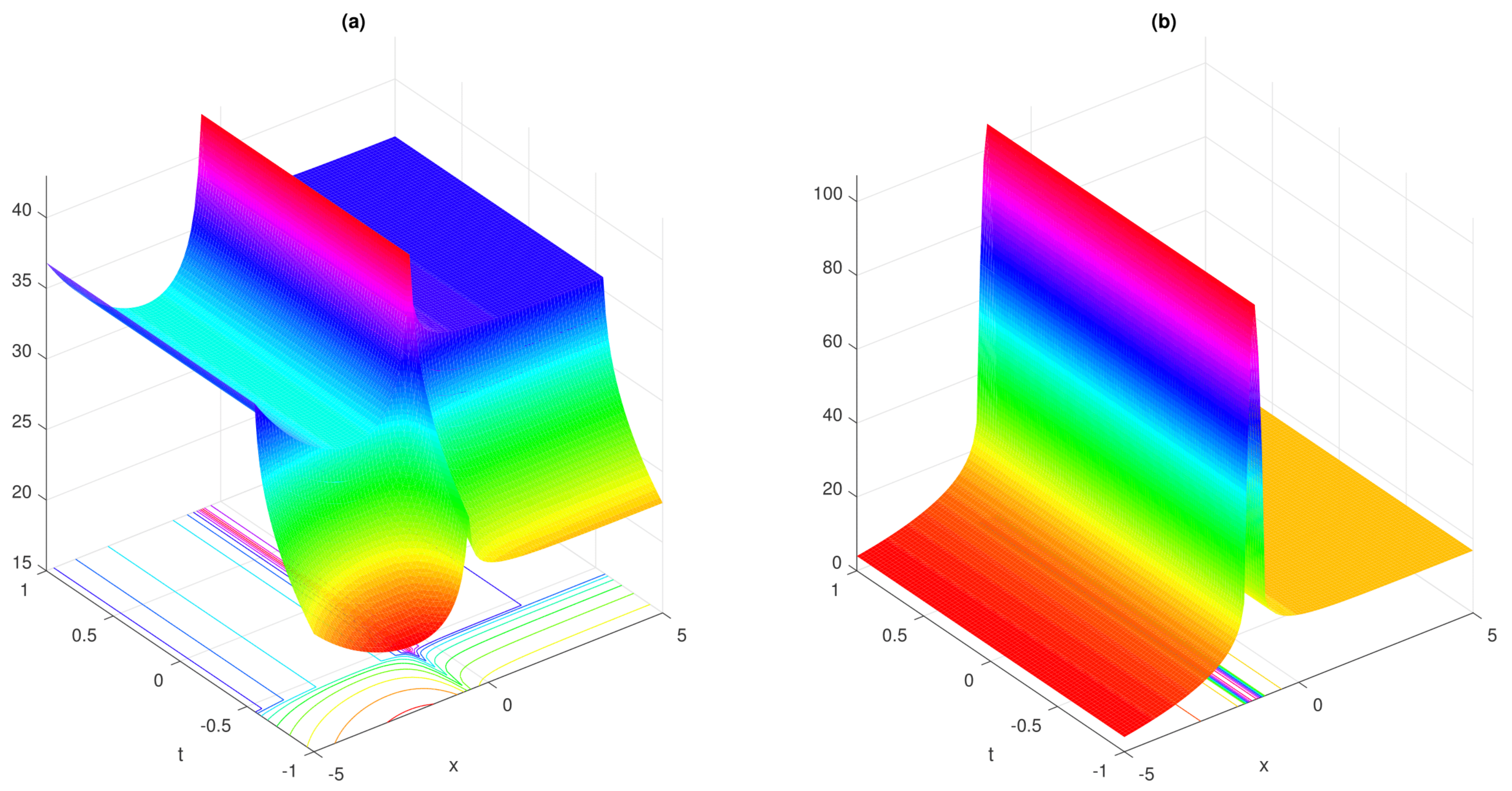
3.4. Anti-Cubic Law
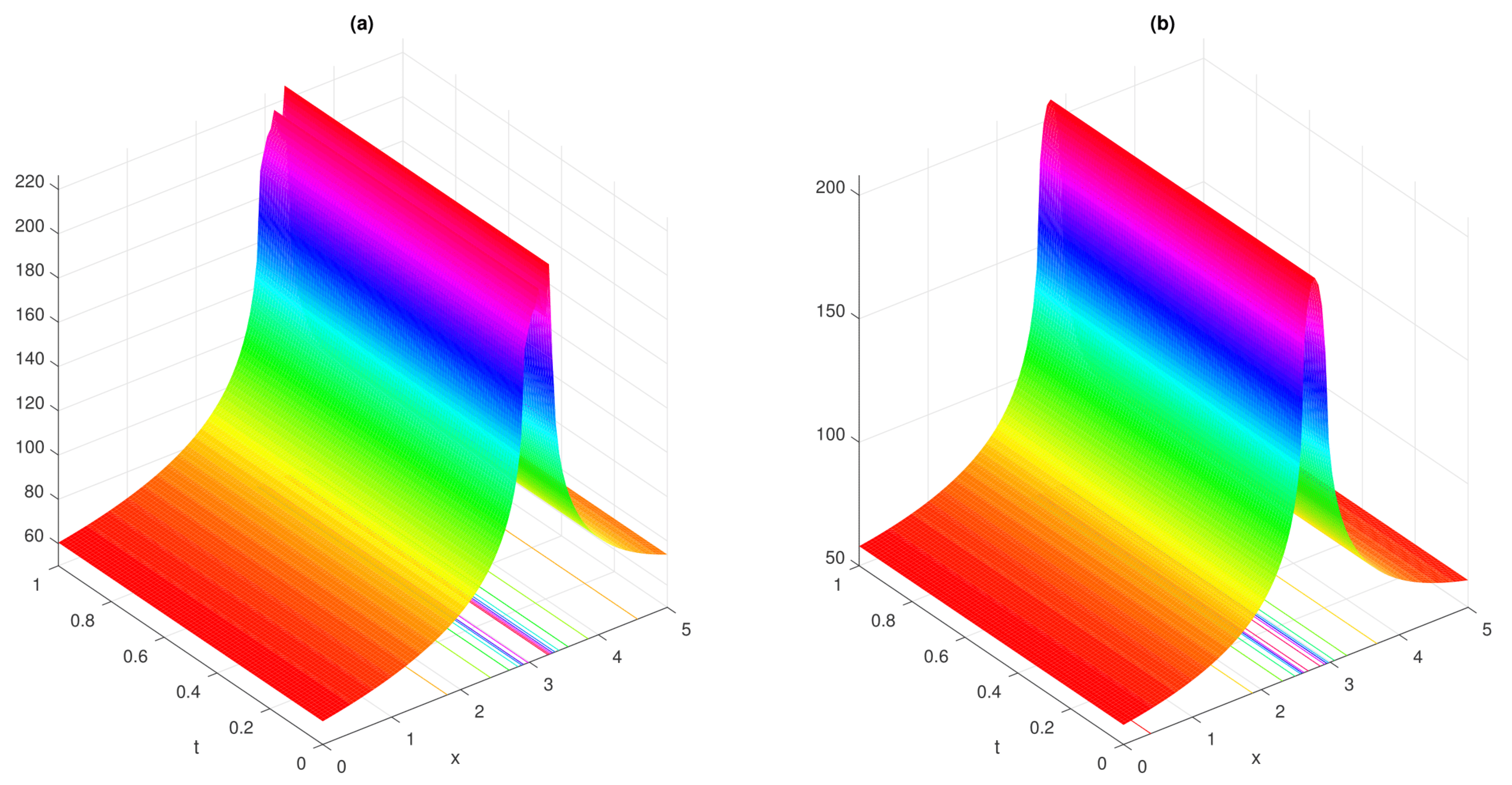
3.5. Dual Power Law
3.6. Triple Power Law
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Osman, M.S.; Machado, J.A.T. New nonautonomous combined multi-wave solutions for (2 + 1)-dimensional variable coefficients KdV equation. Nonlinear Dyn. 2018, 93, 733–740. [Google Scholar] [CrossRef]
- Osman, M.S.; Abdel-Gawad, H.I.; El Mahdy, M.A. Two-layer-atmospheric blocking in a medium with high nonlinearity and lateral dispersion. Results Phys. 2018, 8, 1054–1060. [Google Scholar] [CrossRef]
- Biswas, A.; Konar, S. Introduction to Non-Kerr Law Optical Solitons; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
- Wazwaz, A.M. A study on linear and nonlinear Schrodinger equations by the variational iteration method. Chaos Solitons Fractals 2008, 37, 1136–1142. [Google Scholar] [CrossRef]
- Ma, X.W.; Chen, M. Direct search for exact solutions to the nonlinear Schrödinger equation. Appl. Math. Comput. 2009, 215, 2835–2842. [Google Scholar] [CrossRef] [Green Version]
- Bi, Y.; Zhang, Z.; Liu, Q.; Liu, T. Research on nonlinear waves of blood flow in arterial vessels. Commun. Nonlinear Sci. Numer. Simul. 2021, 102, 105918. [Google Scholar] [CrossRef]
- Manafian, J. Optical soliton solutions for Schrodinger type nonlinear evolution equations by the tan(ϕ/2)-expansion method. Optik 2016, 127, 4222–4245. [Google Scholar] [CrossRef]
- Zhou, Q.; Biswas, A. Optical soliton in parity-time-symmetric mixed linear and nonlinear lattice with non-Kerr law nonlinearity. Superlatices Microstruct. 2017, 109, 588–598. [Google Scholar] [CrossRef]
- Boutabba, N. Kerr-effect analysis in a three-level negative index material under magneto cross-coupling. J. Opt. 2018, 20, 025102. [Google Scholar] [CrossRef]
- Eleuch, H.; Elser, D.; Bennaceur, R. Soliton propagation in an absorbing three-level atomic system. Laser Phys. Lett. 2004, 1, 391. [Google Scholar] [CrossRef]
- Boutabba, N.; Eleuch, H.; Bouchriha, H. Thermal bath effect on soliton propagation in three-level atomic system. Synth. Met. 2009, 159, 1239–1243. [Google Scholar] [CrossRef]
- Biswas, A.; Alqahtani, R.T. Chirp-free bright optical solitons for perturbed Gerdjikov-Ivanov equation by semi-inverse variational principle. Optik 2017, 147, 72–76. [Google Scholar] [CrossRef]
- Rezazadeh, H.; Osman, M.S.; Eslami, M.; Mirzazadeh, M.; Zhou, Q.; Badri, S.A.; Korkmaz, A. Hyperbolic rational solutions to a variety of conformable fractional Boussinesq-Like equations. Nonlinear Eng. 2019, 8, 224–230. [Google Scholar] [CrossRef]
- Biswas, A.; Yildirim, Y.; Yasar, E.; Triki, H.; Zhou, Q.; Moshokoa, S.P.; Ullah, M.Z.; Belic, M. Optical soliton with full nonlinearity in polarization-preserving fibers using trial equation method. J. Optoelectron. Adv. Mater. 2018, 20, 385–402. [Google Scholar]
- Ekici, M.; Sonmezoglu, A.; Zhou, Q.; Moshokoa, S.P.; Ullah, M.Z.; Biswas, A.; Belic, M. Solitons in magneto-optic waveguides by extended trial function scheme. Superlattices Microstruct. 2017, 107, 197–218. [Google Scholar] [CrossRef] [Green Version]
- Ekici, M.; Sonmezoglu, A.; Zhou, Q.; Biswas, A.; Ullah, M.Z.; Asma, M.; Moshokoa, S.P.; Belic, M. Optical solitons in DWDM system by extended trial equation method. Optik 2017, 141, 157–167. [Google Scholar] [CrossRef]
- Ekici, M.; Mirzazadeh, M.; Sonmezoglu, A.; Ullah, M.Z.; Zhou, Q.; Triki, H.; Moshokoa, S.P.; Biswas, A. Optical solitons with anti-cubic nonlinearity by extended trial equation method. Optik 2017, 136, 368–373. [Google Scholar] [CrossRef]
- Osman, M.S. On multi-soliton solutions for the (2 + 1)-dimensional breaking soliton equation with variable coefficients in a graded-index waveguide. Comput. Math. Appl. 2018, 75, 1–6. [Google Scholar] [CrossRef]
- Osman, M.S.; Liu, J.G.; Hosseini, K.; Yusuf, A. Different wave structures and stability analysis for the generalized (2 + 1)-dimensional Camassa-Holm-Kadomtsev-Petviashvili equation. Phys. Scr. 2020, 95, 035229. [Google Scholar] [CrossRef]
- Ma, W.X.; Huang, T.; Zhang, Y. A multiple exp-function method for nonlinear differential equations and its application. Phys. Scr. 2010, 82, 065003. [Google Scholar] [CrossRef]
- Ma, W.X.; Zhu, Z. Solving the (3 + 1)-dimensional generalized KP and BKP equations by the multiple exp-function algorithm. Appl. Math. Comput. 2012, 218, 11871–11879. [Google Scholar] [CrossRef]
- Ghanbari, B.; Gómez-Aguilar, J.F. The generalized exponential rational function method for Radhakrishnan-Kundu-Lakshmanan equation with β-conformable time derivative. Rev. Mex. Física 2019, 65, 503–518. [Google Scholar] [CrossRef] [Green Version]
- Hosseini, K.; Mirzazadeh, M.; Ilie, M.; Gómez-Aguilar, J.F. Biswas-Arshed equation with the beta time derivative: Optical solitons and other solutions. Optik 2020, 217, 164801. [Google Scholar] [CrossRef]
- Hosseini, K.; Mirzazadeh, M.; Ilie, M.; Radmehr, S. Dynamics of optical solitons in the perturbed Gerdjikov-Ivanov equation. Optik 2020, 206, 164350. [Google Scholar] [CrossRef]
- Zafar, A.; Bekir, A.; Khalid, B.; Rezazadeh, H. Abundant solitary wave solutions for the fractional coupled Jaulent-Miodek equations arising in applied physics. Int. J. Mod. Phys. B 2020, 34, 2050279. [Google Scholar] [CrossRef]
- Abdel-Gawad, H.I.; Osman, M.S. On the variational approach for analyzing the stability of solutions of evolution equations. Kyungpook Math. J. 2013, 53, 661–680. [Google Scholar] [CrossRef] [Green Version]
- Zhang, W.H.; Yang, H.W.; Gao, D.Z. Time fractional order mZK model and its exact solution in dust plasma. Math. Model. Appl. 2020, 9, 66–73. [Google Scholar]
- Wazwaz, A.M. The extended tanh method for the Zakharov-Kuznestsov(ZK) equation, the modified ZK equation, and its generalized forms. Commun. Nonlinear Sci. 2008, 13, 1039–1047. [Google Scholar] [CrossRef]
- Ma, W.X.; Lee, J.H. A transformed rational function method and exact solutions to the (3 + 1) dimensional Jimbo-Miwa equation. Chaos Solitons Fractals 2009, 42, 1356–1363. [Google Scholar] [CrossRef] [Green Version]
- Zafar, A.; Raheel, M.; Bekir, A. Expolring the dark and singular soliton solutions of Biswas-Arshed model with full nonlinear form. Optik 2020, 204, 164133. [Google Scholar] [CrossRef]
- Latha, M.M.; Christal, V.C. An integrable model of (2 + 1)-dimensional Heisenberg ferromagnetic spin chain and soliton excitations. Phys. Scr. 2014, 89, 065204. [Google Scholar] [CrossRef]
- Boutabba, N.; Eleuch, H. Soliton-pair propagation under thermal bath effect. Math. Model. Nat. Phenom. 2012, 7, 32–37. [Google Scholar] [CrossRef]
- Boutabba, N.; Eleuch, H. Slowing light control for a soliton-pair. Appl. Math. Inf. Sci. 2013, 7, 1505. [Google Scholar] [CrossRef]
- Kohl, R.; Biswas, A.; Milovic, D.; Zerrad, E. Optical soliton perturbation in a non-Kerr law media. Opt. Laser Technol. 2008, 40, 647–662. [Google Scholar] [CrossRef]
- Kohl, R.; Milovic, D.; Zerrad, E.; Biswas, A. Optical solitons by He’s variational principle in a non-Kerr law media. J. Infrared Millim. Terahertz Waves 2009, 30, 526–537. [Google Scholar] [CrossRef]
- Biswas, A.; Milovic, D. Optical solitons in (1 + 2)- dimensions with time-dependent dispersion, nonlinearity and attenuation in a Kerr-Law media. J. Electromagn. Waves Appl. 2008, 22, 1801–1808. [Google Scholar] [CrossRef]
- Atangana, A.; Alqahtani, R.T. Modelling the spread of river blindness disease via the Caputo fractional derivative and the beta-derivative. Entropy 2016, 18, 40. [Google Scholar] [CrossRef]
- Yusuf, A.; Inc, M.; Aliyu, A.I.; Baleanu, D. Optical solitons possessing beta derivative of the Chen-Lee-Liu equation in optical fibers. Front. Phys. 2019, 7, 34. [Google Scholar] [CrossRef] [Green Version]
- Uddin, M.F.; Hafez, M.G.; Hammouch, Z.; Baleanu, D. Periodic and rogue waves for Heisenberg models of ferromagnetic spin chains with fractional beta derivative evolution and obliqueness. Waves Random Complex Media 2020. [Google Scholar] [CrossRef]
- Arshed, S. New soliton solutions to the perturbed nonlinear Schrödinger equation by exp(−ϕ(ξ))-expansion method. Optik 2020, 220, 165123. [Google Scholar] [CrossRef]
- Raslan, K.R.; Khalid, K.A.; Shallal, M.A. The modified extended tanh method with the Riccati equation for solving the space-time fractional EW and MEW equations. Chaos Solitons Fractals 2017, 103, 404–409. [Google Scholar] [CrossRef]





Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zafar, A.; Raheel, M.; Zafar, M.Q.; Nisar, K.S.; Osman, M.S.; Mohamed, R.N.; Elfasakhany, A. Dynamics of Different Nonlinearities to the Perturbed Nonlinear Schrödinger Equation via Solitary Wave Solutions with Numerical Simulation. Fractal Fract. 2021, 5, 213. https://doi.org/10.3390/fractalfract5040213
Zafar A, Raheel M, Zafar MQ, Nisar KS, Osman MS, Mohamed RN, Elfasakhany A. Dynamics of Different Nonlinearities to the Perturbed Nonlinear Schrödinger Equation via Solitary Wave Solutions with Numerical Simulation. Fractal and Fractional. 2021; 5(4):213. https://doi.org/10.3390/fractalfract5040213
Chicago/Turabian StyleZafar, Asim, Muhammad Raheel, Muhammad Qasim Zafar, Kottakkaran Sooppy Nisar, Mohamed S. Osman, Roshan Noor Mohamed, and Ashraf Elfasakhany. 2021. "Dynamics of Different Nonlinearities to the Perturbed Nonlinear Schrödinger Equation via Solitary Wave Solutions with Numerical Simulation" Fractal and Fractional 5, no. 4: 213. https://doi.org/10.3390/fractalfract5040213
APA StyleZafar, A., Raheel, M., Zafar, M. Q., Nisar, K. S., Osman, M. S., Mohamed, R. N., & Elfasakhany, A. (2021). Dynamics of Different Nonlinearities to the Perturbed Nonlinear Schrödinger Equation via Solitary Wave Solutions with Numerical Simulation. Fractal and Fractional, 5(4), 213. https://doi.org/10.3390/fractalfract5040213







