Transient 3D CFD Simulation of a Pelton Turbine—A State-of-the-Art Approach for Pelton Development and Optimisation †
Abstract
1. Introduction
2. Numerical Simulation
2.1. Prototype Turbine Model
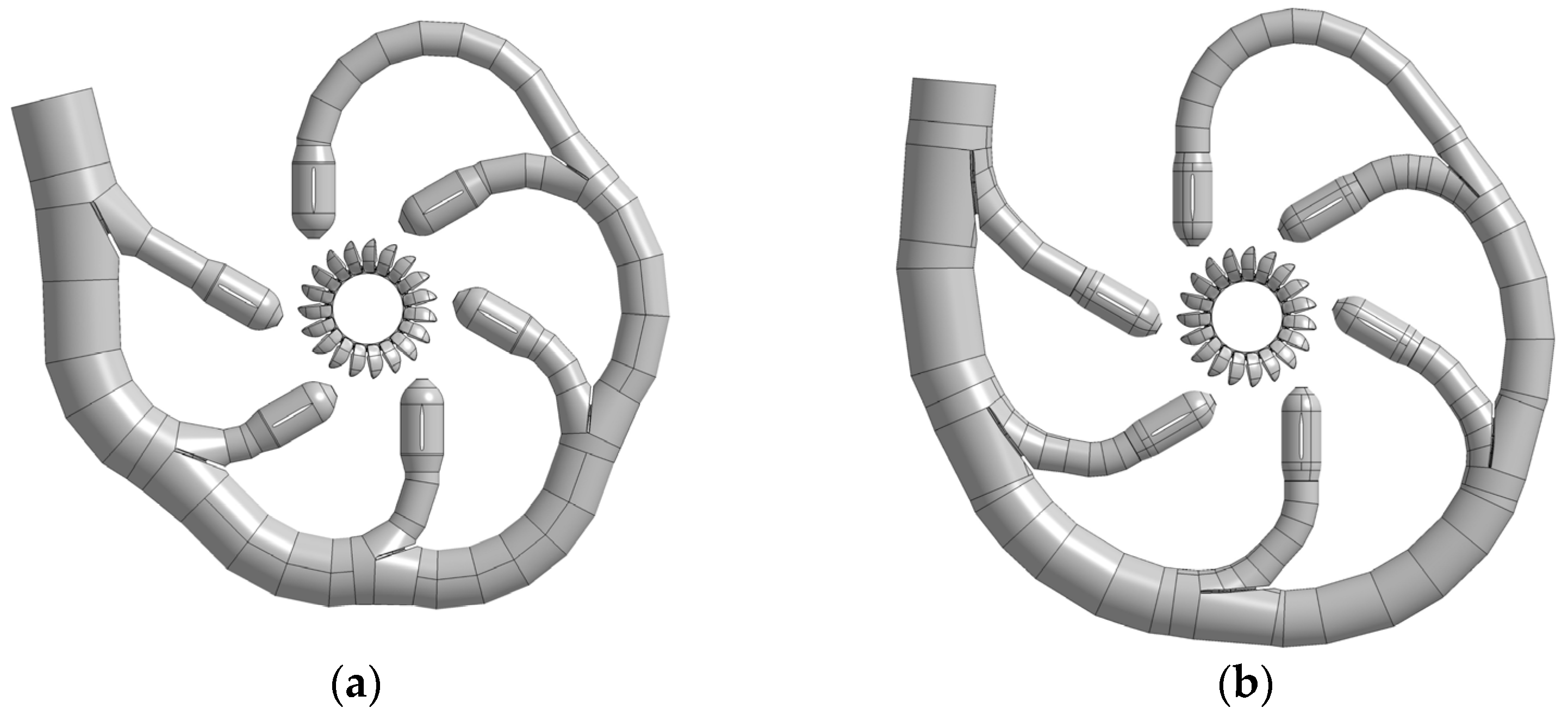
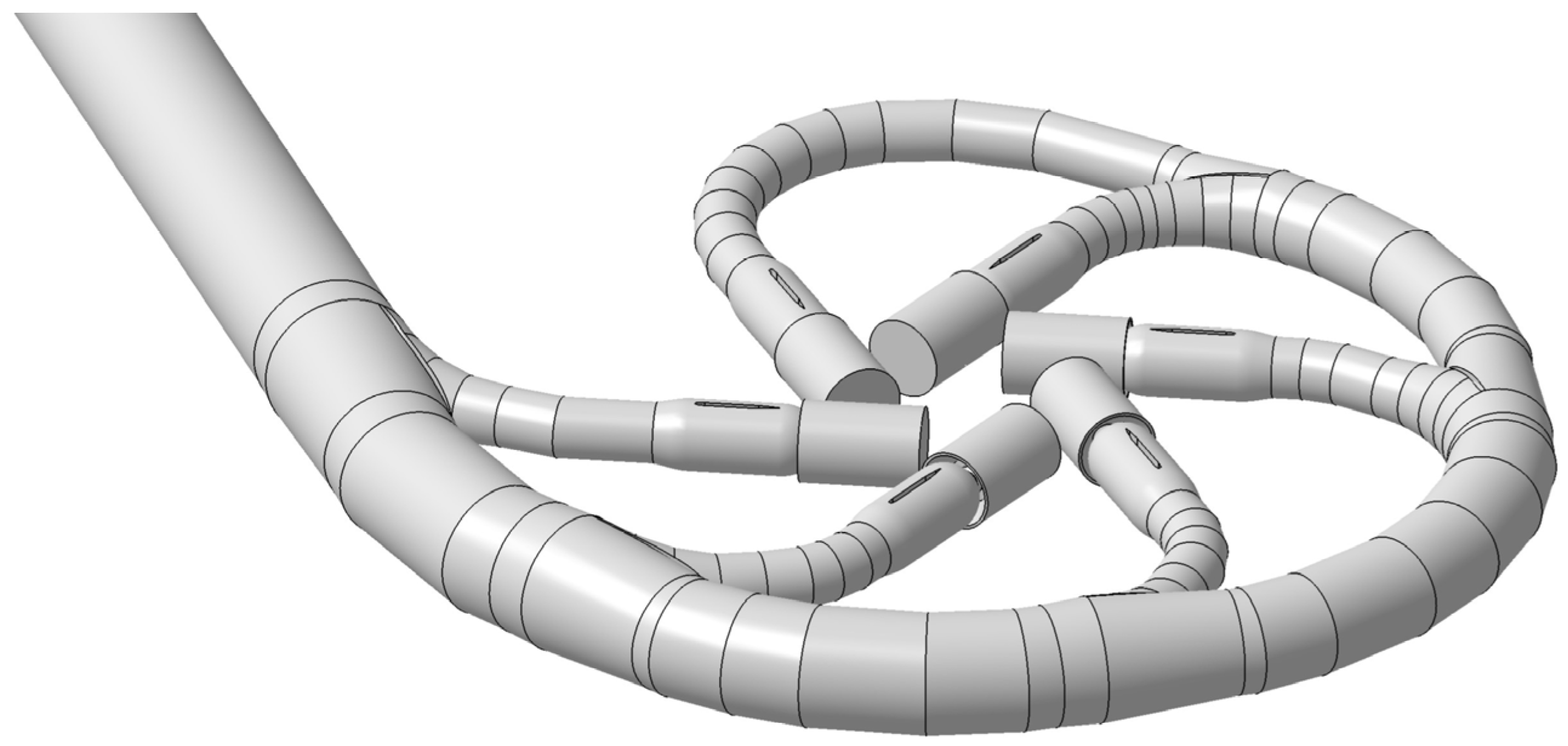
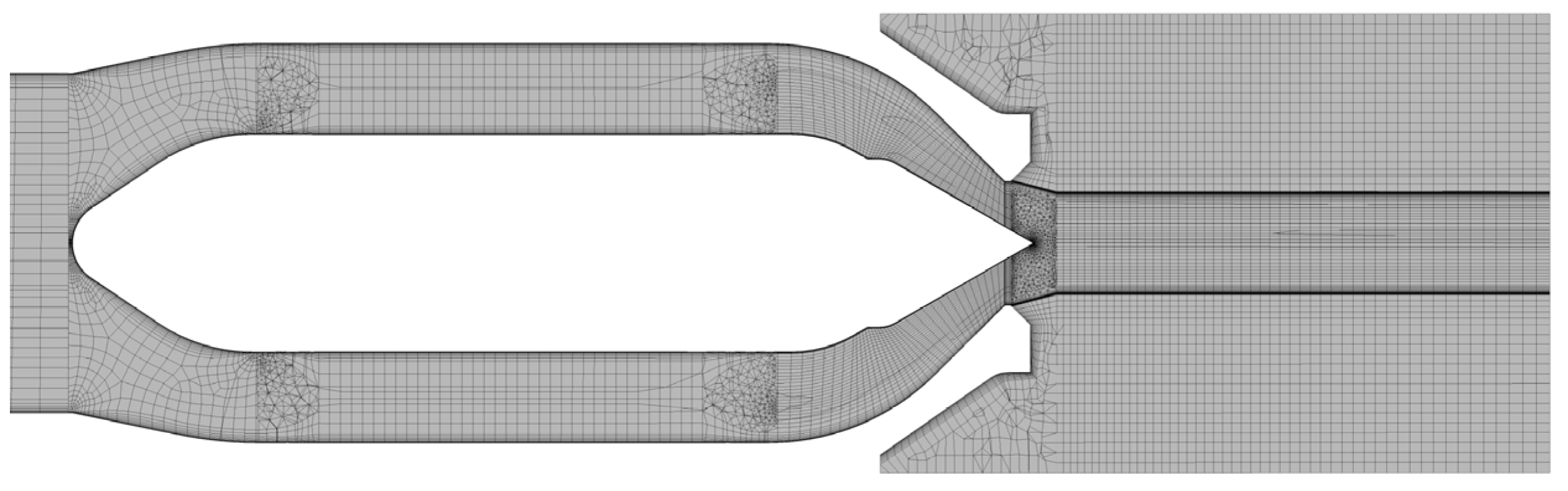
2.2. Numerical Modelling of the Distributor
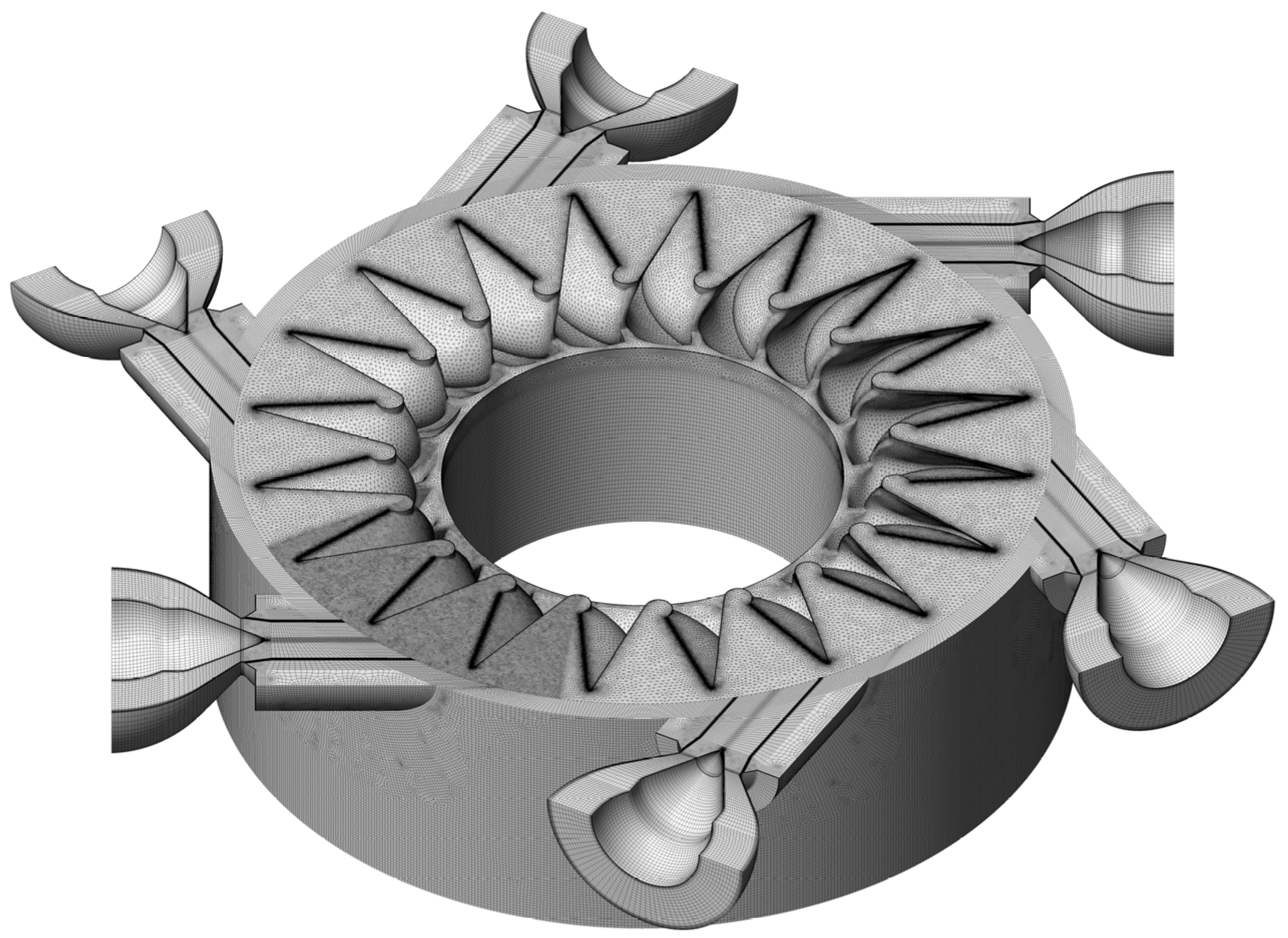
2.3. Numerical Modelling of the Runner
3. Results
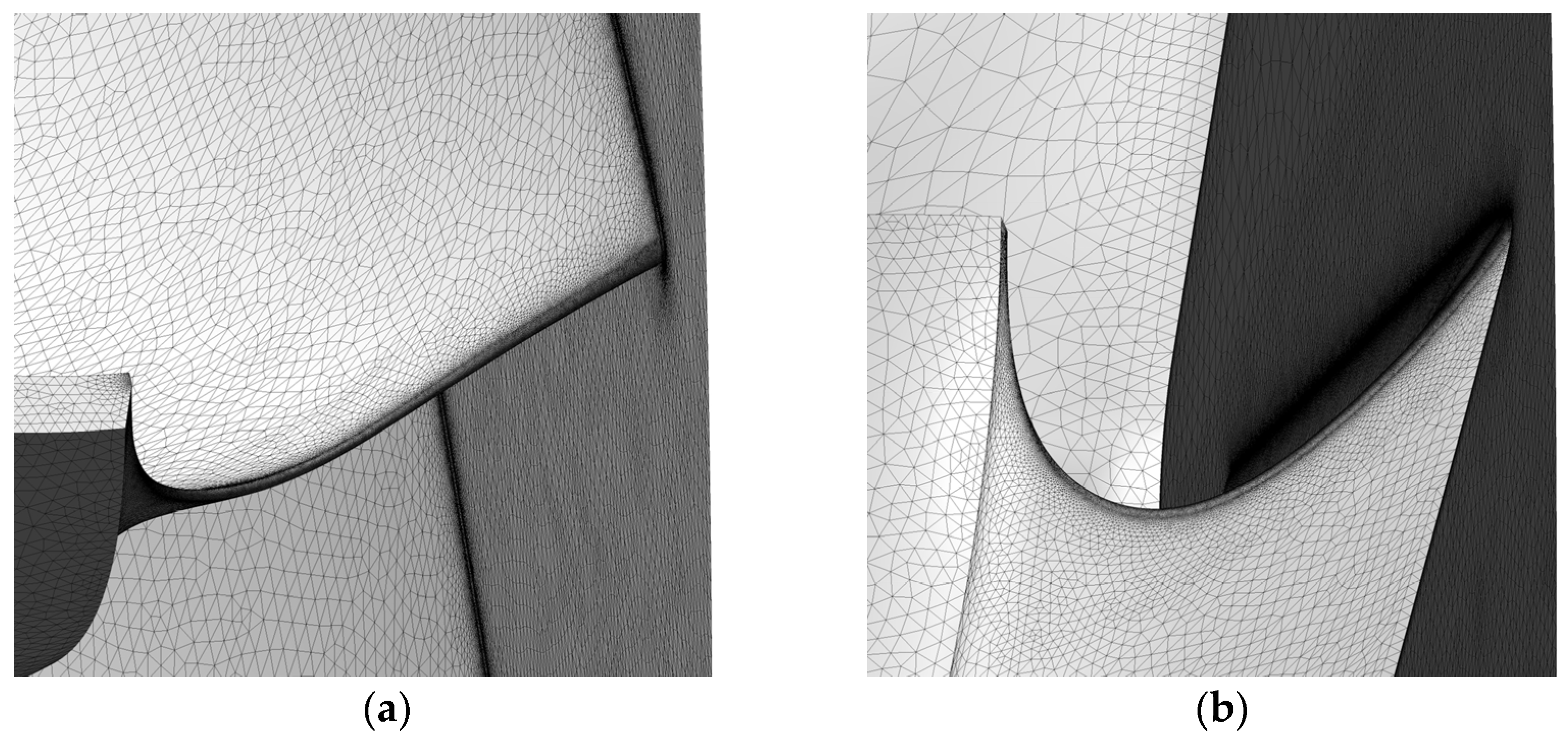
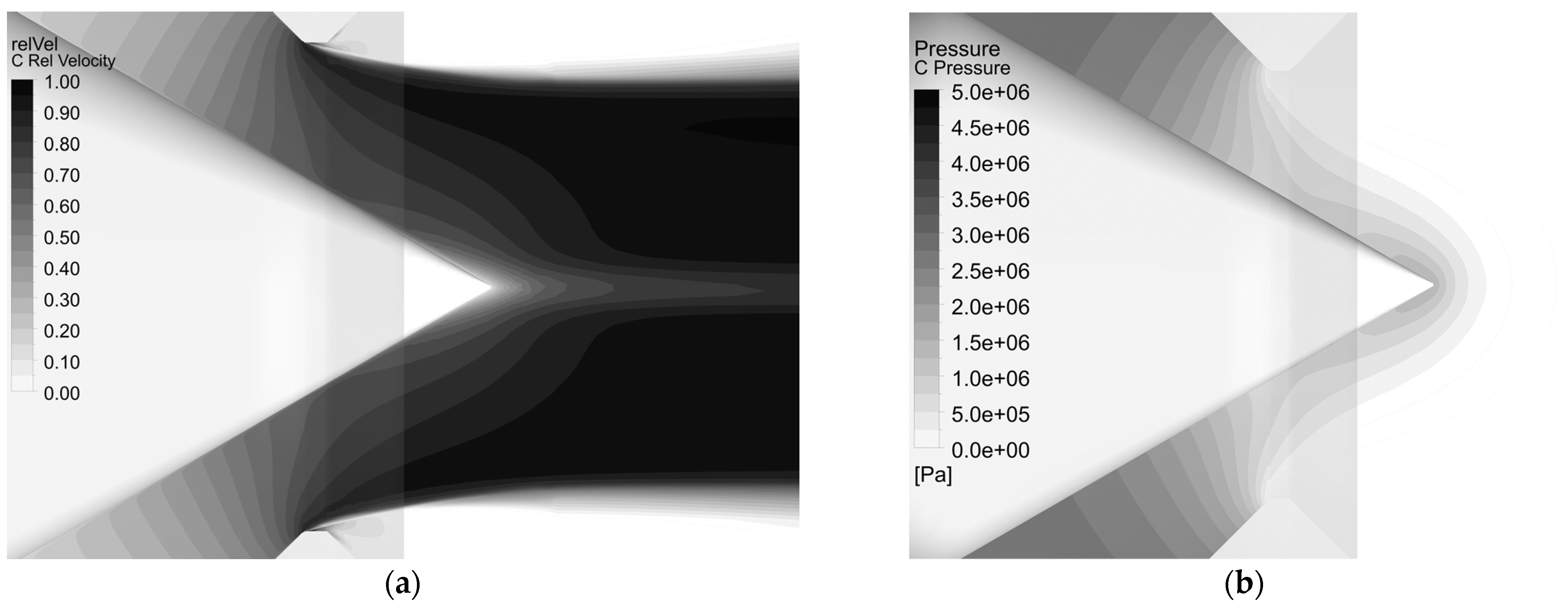
3.1. Results of the Distributor Analysis
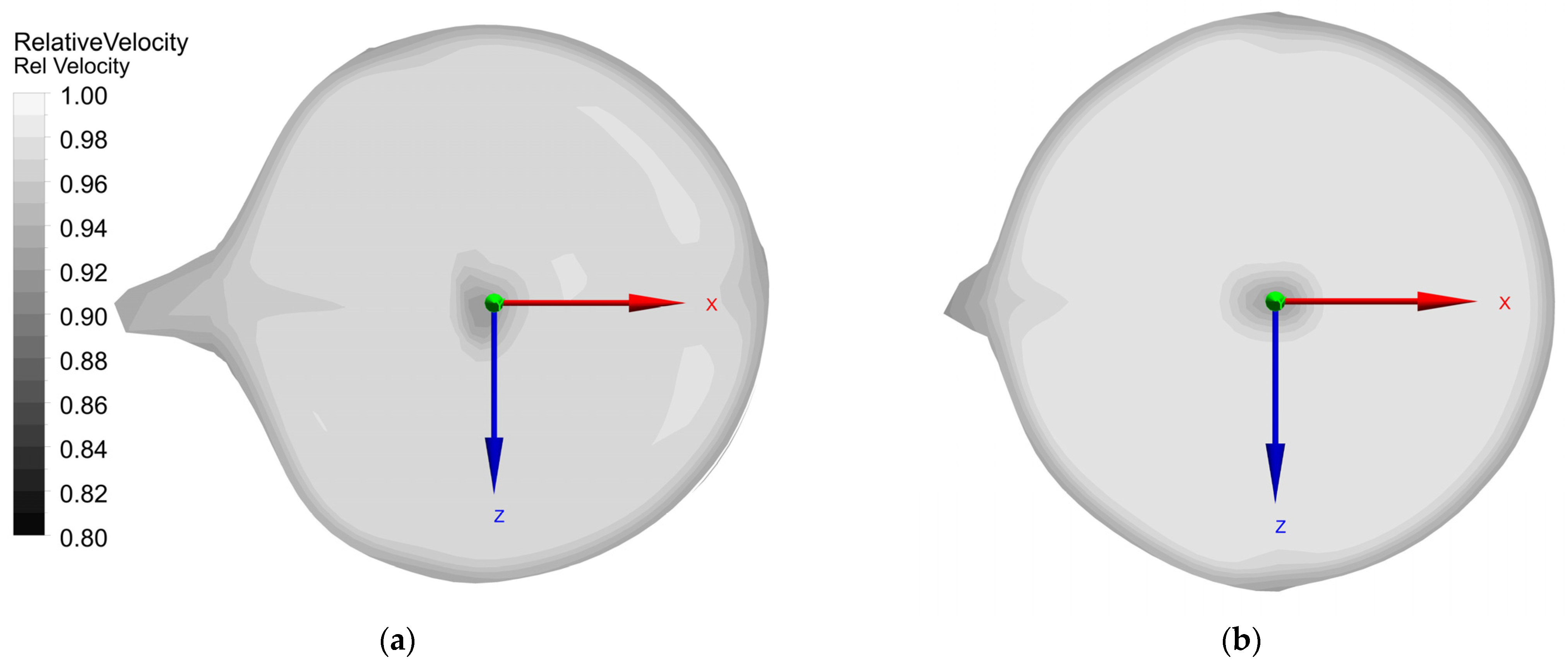
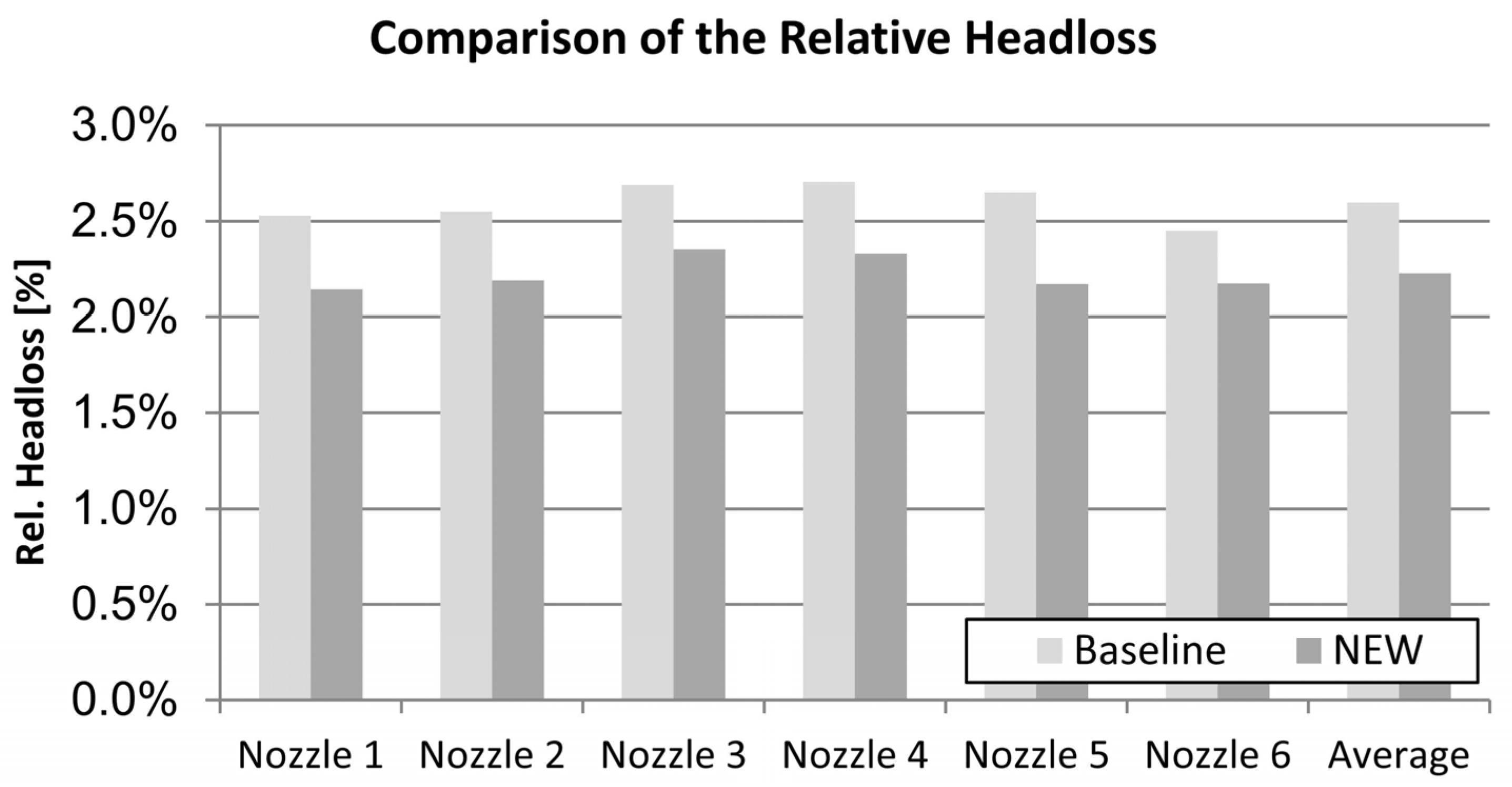
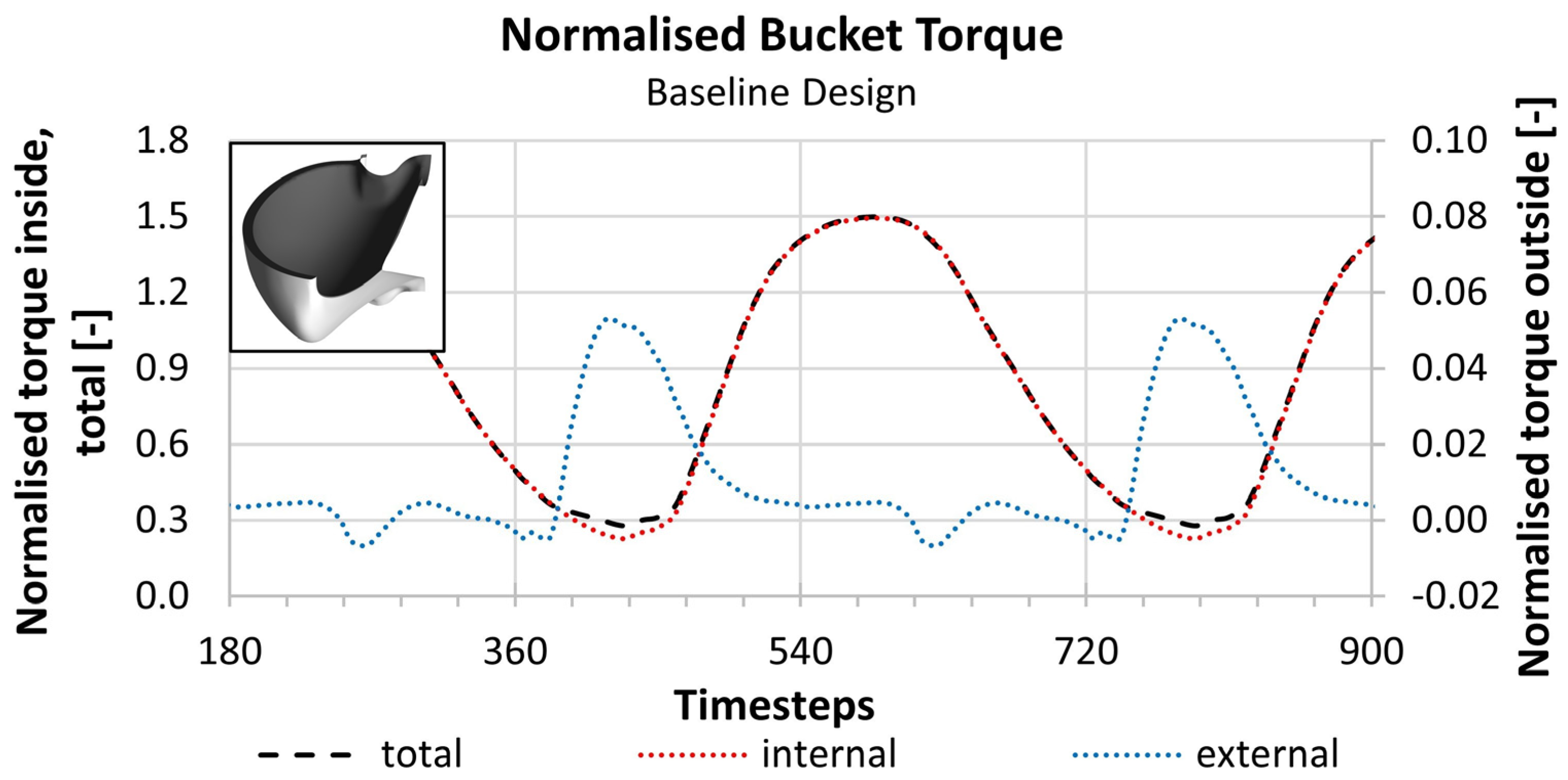
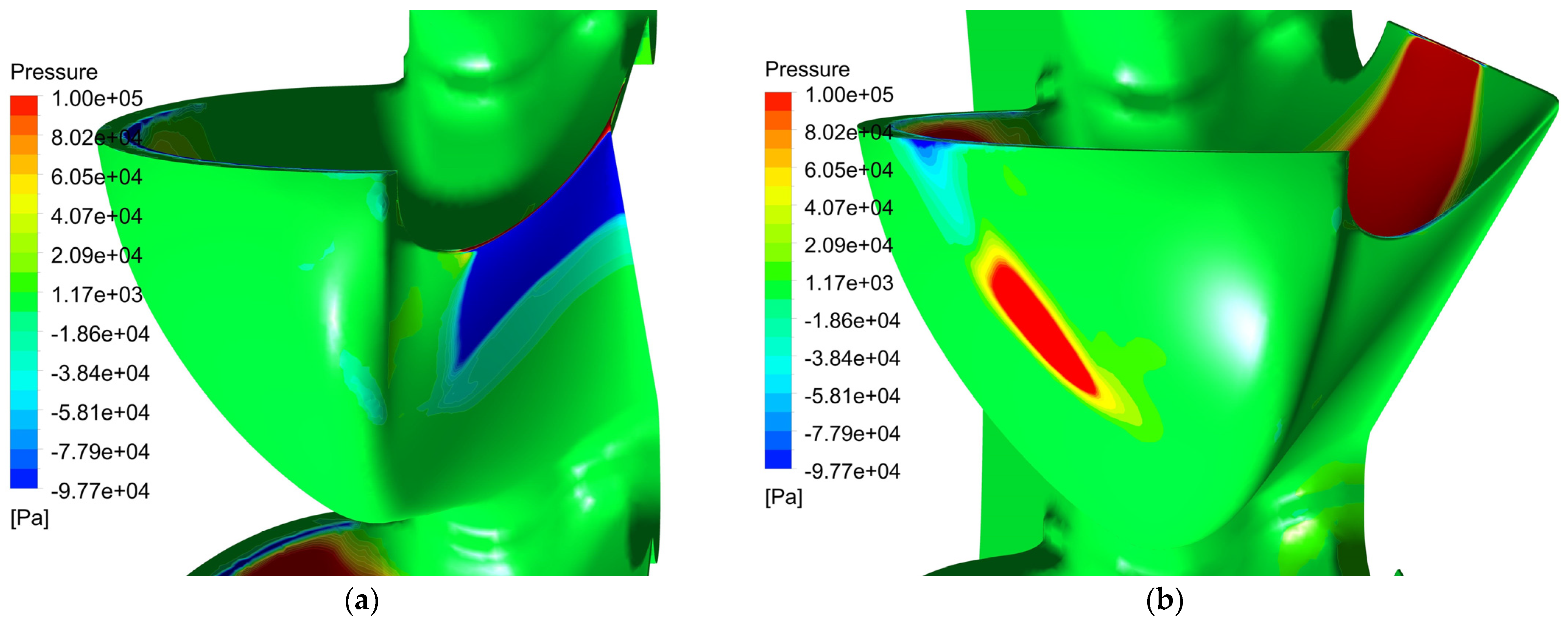
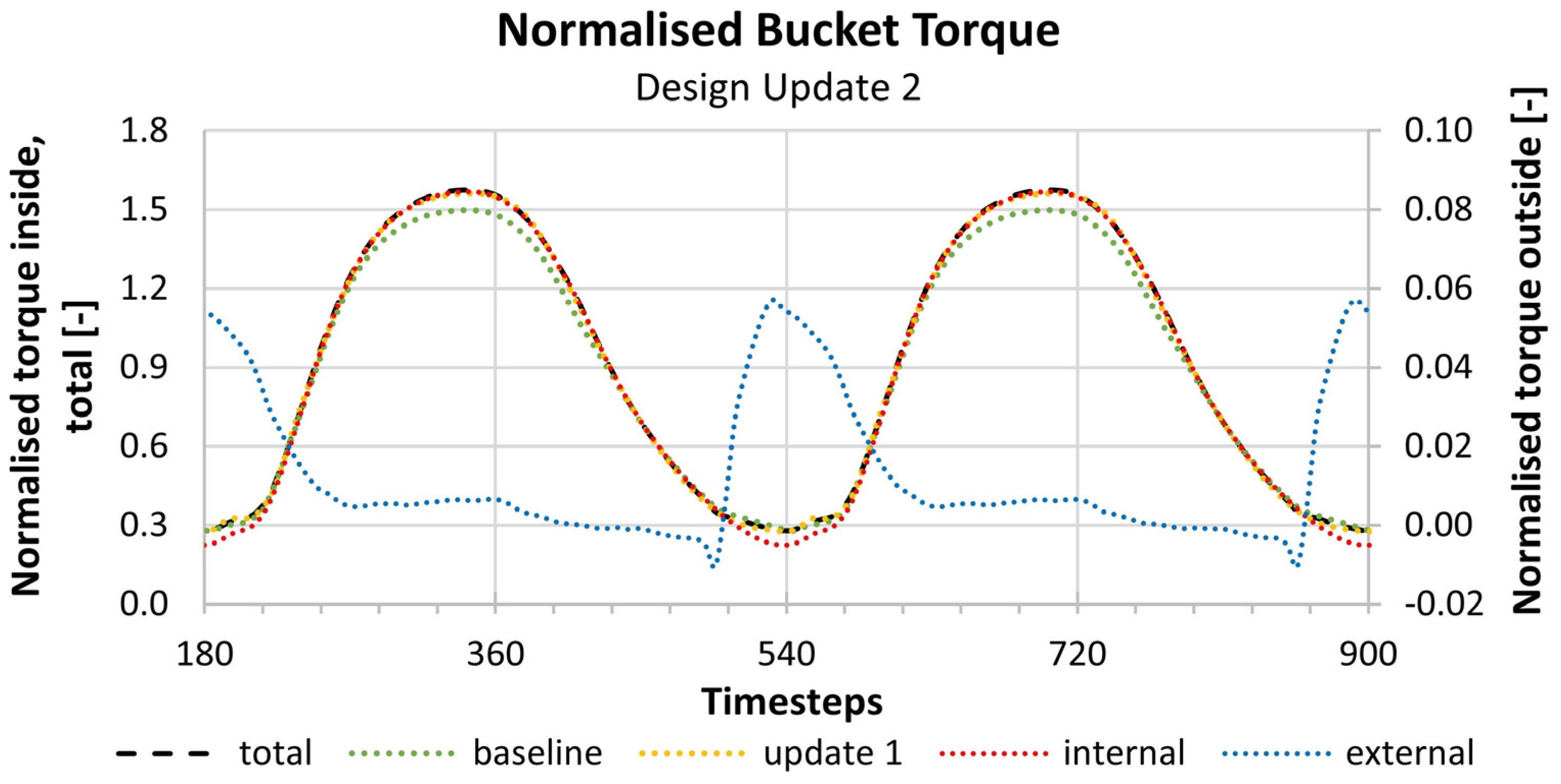
3.2. Results of the Runner Simulation
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Keck, H.; Sick, M. Thirty years of numerical flow simulation in hydraulic turbomachines. Acta Mech. 2008, 201, 211–229. [Google Scholar] [CrossRef]
- Sick, M.; Schindler, M.; Drtina, P.; Schärer, C.; Keck, H. Numerical and experimental analysis of Pelton turbine flow. Part 1: Distributor an Injector. In Proceedings of the IAHR Symposium, Charlotte, NC, USA, 6–9 August 2000. [Google Scholar]
- Muggli, F.A.; Zhang, J.; Schärer, C.; Geppert, L. Numerical and experimental analysis of Pelton turbine flow. Part 2: The Free Surface Jet Flow. In Proceedings of the IAHR Symposium, Charlotte, NC, USA, 6–9 August 2000. [Google Scholar]
- Zoppé, B.; Pellone, C.; Maitre, T.; Leroy, P. Flow analysis inside a Pelton turbine bucket. J. Turbomach. 2006, 128, 500–511. [Google Scholar] [CrossRef]
- Zeng, C.; Xiao, Y.; Luo, Y.; Zhang, J.; Wang, Z.; Fan, H.; Ahn, S.-H. Hydraulic performance prediction of a prototype four-nozzle Pelton turbine by entire flow path simulation. Renew. Energy 2018, 125, 270–282. [Google Scholar] [CrossRef]
- Židonis, A. Optimisation and Efficiency Improvement of Pelton Hydro Turbine Using Computational Fluid Dynamics and Experimental Testing. Ph.D. Thesis, Lancaster University, Lancaster, UK, 2015. [Google Scholar]
- Rossetti, A.; Pavesi, G.; Cavazzini, G.; Santolin, A.; Ardizzon, G. Influence of the bucket geometry on the Pelton performance. Inst. Mech. Eng. Part A J. Power Energy 2014, 228, 33–45. [Google Scholar] [CrossRef]
- Perrig, A.; Avellan, F.; Kueny, J.-L.; Farhat, M.; Parkinson, E. Flow in a Pelton turbine bucket: Numerical and experimental investigations. J. Fluids Eng. 2006, 128, 350–358. [Google Scholar] [CrossRef]
- Rentschler, M.; Marongiu, J.C.; Neuhauser, M.; Parkinson, E. Overview of SPH-ALE applications for hydraulic turbines in ANDRITZ Hydro. J. Hydrodyn. 2018, 30, 114–121. [Google Scholar] [CrossRef]
- Bhattarai, S.; Vichare, P.; Dahal, K.; Al Makky, A.; Olabi, A.G. Novel trends in modelling techniques of Pelton Turbine bucket for increased renewable energy production. Renew. Sustain. Energy Rev. 2019, 112, 87–101. [Google Scholar] [CrossRef]
- Jošt, D.; Mežnar, P.; Lipej, A. Numerical prediction of Pelton turbine efficiency. IOP Conf. Ser. Earth Environ. Sci. 2010, 12, 12080. [Google Scholar] [CrossRef]
- Xiao, Y.; Wang, Z.; Zhang, J.; Zeng, C.; Yan, Z. Numerical and experimental analysis of the hydraulic performance of a prototype Pelton turbine. Inst. Mech. Eng. Part A J. Power Energy 2014, 228, 46–55. [Google Scholar] [CrossRef]
- Zeng, C.; Xiao, Y.; Wang, Z.; Zhang, J.; Luo, Y. Numerical analysis of a Pelton bucket free surface sheet flow and dynamic performance affected by operating head. Inst. Mech. Eng. Part A J. Power Energy 2017, 231, 182–196. [Google Scholar] [CrossRef]
- Santolin, A.; Cavazzini, G.; Ardizzon, G.; Pavesi, G. Numerical investigation of the interaction between jet and bucket in a Pelton turbine. Inst. Mech. Eng. Part A J. Power Energy 2009, 223, 721–728. [Google Scholar] [CrossRef]
- Perrig, A. Hydrodynamics of the Free Suface Flow in Pelton Turbine Buckets. Ph.D. Thesis, EPFL, Lausanne, Switzerland, 2007. [Google Scholar]
- Židonis, A.; Aggidis, G.A. State of the art in numerical modelling of Pelton turbines. Renew. Sustain. Energy Rev. 2015, 45, 135–144. [Google Scholar] [CrossRef]
- Chitrakar, S.; Solemslie, B.W.; Neopane, H.P.; Dahlhaug, O.G. Review on numerical techniques applied in impulse hydro turbines. Renew. Energy 2020, 159, 843–859. [Google Scholar] [CrossRef]
- Drazin, P.G.; Reid, W.H. (Eds.) Hydrodynamic Stability; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]


| Characteristic Dimensions | Values |
|---|---|
| 1310.00 mm | |
| 375.25 mm | |
| 115.00 mm | |
| 3.50 | |
| 19 |
| Mesh Metric | Minimum | Maximum | Average |
|---|---|---|---|
| Min. Face Angle | 11.3° | 90.0° | 57.8° |
| Max. Face Angle | 60.0° | 157.3° | 97.2° |
| Aspect Ratio | 1 | 351 | 24.1 |
| Y Plus | - | - | 41.1 |
| Mesh Region | Nodes | Elements |
|---|---|---|
| Nozzle, Casing | 2.604 | 2.352 |
| Runner fine | 4.530 | 17.101 |
| Runner coarse | 5.597 | 16.511 |
| Total | 12.732 | 35.965 |
| Mesh Metric | Minimum | Maximum | Average |
|---|---|---|---|
| Min. Face Angle | 10.4° | 90.0° | 47.9° |
| Max. Face Angle | 60.0° | 153.2° | 92.5° |
| Aspect Ratio | 1 | 226 | 6.3 |
| Y Plus | - | - | 53.1 |
| Design | Nozzle 1 | Nozzle 2 | Nozzle 3 | Nozzle 4 | Nozzle 5 | Nozzle 6 |
|---|---|---|---|---|---|---|
| Baseline Design | 0.027 | 0.039 | 0.041 | 0.032 | 0.036 | 0.023 |
| New Design | 0.057 | 0.115 | 0.069 | 0.121 | 0.087 | 0.065 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Sandmaier, L.; Meusburger, P.; Benigni, H. Transient 3D CFD Simulation of a Pelton Turbine—A State-of-the-Art Approach for Pelton Development and Optimisation. Int. J. Turbomach. Propuls. Power 2023, 8, 10. https://doi.org/10.3390/ijtpp8010010
Sandmaier L, Meusburger P, Benigni H. Transient 3D CFD Simulation of a Pelton Turbine—A State-of-the-Art Approach for Pelton Development and Optimisation. International Journal of Turbomachinery, Propulsion and Power. 2023; 8(1):10. https://doi.org/10.3390/ijtpp8010010
Chicago/Turabian StyleSandmaier, Lukas, Peter Meusburger, and Helmut Benigni. 2023. "Transient 3D CFD Simulation of a Pelton Turbine—A State-of-the-Art Approach for Pelton Development and Optimisation" International Journal of Turbomachinery, Propulsion and Power 8, no. 1: 10. https://doi.org/10.3390/ijtpp8010010
APA StyleSandmaier, L., Meusburger, P., & Benigni, H. (2023). Transient 3D CFD Simulation of a Pelton Turbine—A State-of-the-Art Approach for Pelton Development and Optimisation. International Journal of Turbomachinery, Propulsion and Power, 8(1), 10. https://doi.org/10.3390/ijtpp8010010







