A Comparative Performance Analysis of the Novel TurboAux Engine with a Turbojet Engine, and a Low-Bypass Ratio Turbofan Engine with an Afterburner
Abstract
1. Introduction
2. Optimization Analysis
2.1. Low-Bypass Turbofan as a Baseline for the TurboAux Engine
2.2. Low-Bypass Turbofan Engine Optimization–Engineering the TurboAux Engine
3. High-Fidelity Formulation of the TurboAux, Turbojet, and Turbofan Engines
- All component efficiencies and specific heat capacities are constant.
- Combustion chambers are adiabatic but account for frictional losses.
- The streams will mix fully in the constant-area mixing duct.
- The is no dissociation occurring in the products of combustion.
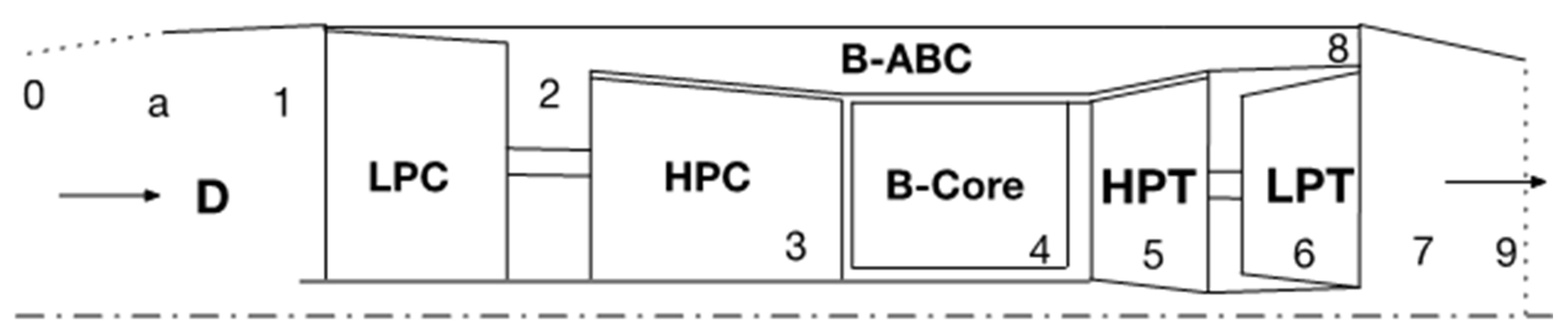
3.1. TurboAux
3.2. Turbojet Engine with Afterburner
3.3. Turbofan Engine with Mixing and an Afterburner
4. Results and Discussion
5. Conclusions and Future Work
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| B | bypass ratio |
| C | local speed of sound |
| Cp0 | specific heat capacity at constant pressure |
| factual | actual fuel to air ratio of core stream |
| faux | actual fuel to air ratio of auxiliary stream |
| fideal | ideal fuel to air ratio |
| fo | overall actual fuel to air ratio of core and auxiliary streams |
| Fs | specific thrust |
| HrpCO2 | enthalpy of reaction of CO2 |
| Hrpf | enthalpy of reaction of fuel |
| HV | heating value of fuel |
| Ma | flight speed |
| Mair | molar mass of air |
| ṁaux | mass flow of auxiliary stream |
| ṁcore | mass flow of core stream |
| ṁf1 | mass flow of fuel into core stream |
| ṁf2 | mass flow of fuel into auxiliary stream |
| Mfuel | molar mass of fuel |
| P0 | stagnation pressure |
| Pa | ambient static pressure |
| R | specific gas constant |
| T | thrust |
| T0 | stagnation temperature |
| Ta | ambient static temperature |
| Tp | static temperature of the products of combustion |
| Tr | static temperature of the reactants of combustion |
| TSFC | thrust specific fuel consumption |
| Va | velocity of air at inlet |
| wCHP | specific work required to drive high-pressure compressor |
| wCLP | specific work required to drive low-pressure compressor |
| ΔP0b | stagnation pressure loss due to aerodynamic resistance and momentum changes from combustion |
| Ycc | moles of air required for stoichiometric combustion |
| γ | ratio of specific heat at constant pressure to specific heat at constant volume |
| ηb | burner efficiency |
| ηc | compressor efficiency |
| ηd | diffuser efficiency |
| ηf | fan chute efficiency |
| ηm | mechanical efficiency |
| ηn | nozzle efficiency |
| ηo | overall efficiency |
| ηp | propulsive efficiency |
| ηt | turbine efficiency |
| ηth | thermal efficiency |
| πc | overall pressure ratio |
| πHP | high-pressure compressor pressure ratio |
| πLP | low-pressure compressor pressure ratio |
Appendix A
References
- Saravanamuttoo, H.I.H.; Rogers, G.F.C.; Cohen, H. Gas Turbine Theory; Pearson Education: New York, NY, USA, 2001. [Google Scholar]
- Asundi, S.A.; Ali, S.F. Parametric Study of a Turbofan Engine with an Auxiliary High-Pressure Bypass. Int. J. Turbomach. 2019, 4, 2. [Google Scholar] [CrossRef]
- Raymer, D.P. Aircraft Design: A Conceptual Approach, 5th ed.; AIAA Education Series; American Institute of Aeronautics & Astronautics: Reston, VA, USA, 2012. [Google Scholar]
- MacIsaac, B.; Langston, R. Gas Turbine Propulsion Systems, 1st ed.; Wiley: Hoboken, NJ, USA, 2011. [Google Scholar]
- Vaferi, K.; Vajdi, M.; Nekahi, S.; Nekahi, S.; Moghanlou, F.S.; Asl, M.S.; Mohammadi, M. Thermo-Mechanical Simulation of Ultrahigh Temperature Ceramic Composites as Alternative Materials for Gas Turbine Stator Blades. Ceram. Int. 2021, 47, 567–580. [Google Scholar] [CrossRef]
- Fetahi, K.; Asundi, S.A.; Taylor, A.C.; Ali, S.F.; Ibrahim, A.H. Parametric Study of a Turbojet Engine with Auxiliary Bypass Combustion—The TurboAux Engine. In Proceedings of the AIAA Propulsion and Energy Forum, New Orleans, LA, USA, 24 August 2020; pp. 1–11. [Google Scholar]
- Lee, A.S.; Singh, R.; Probert, S.D. Two-Combustor Engines’ Performances Under Design and Off-Design Conditions. In Proceedings of the 45th AIAA/ASME/SAE/ASEE Joint Propulsion Conference & Exhibit, Denver, CO, USA, 2–5 August 2009. [Google Scholar] [CrossRef]
- Campbell, A.S. Thermodynamic Analysis of Combustion Engines, 1st ed.; Wiley: Hoboken, NJ, USA, 1979. [Google Scholar]
- Hill, P.; Peterson, C. Mechanics and Thermodynamics of Propulsion, 2nd ed.; Prentice Hall: Upper Saddle River, NJ, USA.


| Flight Conditions | Ma = 0.84 | Pa = 54.05 kPa | Ta = 255.7 K |
| Air Properties | Cp0air = 1004.5 J/kg·K | γair = 1.4 | Rair = 287 J/kg·K |
| Gas Properties | Cp0gas = 1148 J/kg·K | γgas = 1.3333 | Rgas = 287 J/kg·K |
| Other Parameters | TINT = T04 = 1922 K | Taux = T08 = 2516 K | πc = 50 |
| Isentropic Efficiencies | ηd = 0.93 | ηc = 0.87 | ηb = 0.98 |
| ηm = 0.99 | ηt = 0.90 | ηn = 0.95 | |
| Fuel Properties | Hrpf = −8,561,991.6 kJ/kmol | Mfuel = 197.7 kmol/kg | HV = 43308000 J/kg |
| Moles of Carbon (MC) = 14.4 | Moles of Hydrogen (MH) = 24.9 | Moles of Oxygen (MO) = 0 | |
| Other Properties | HrpCO2 = 282,800 kJ/kmol | Mair = 28.97 kmol/kg | ΔP0b = 0.04 |
| Engine | Fs (N·s/kg) | TSFC (kg/N·hr) | Propulsive Efficiency | Thermal Efficiency | Overall Efficiency |
|---|---|---|---|---|---|
| Conventional Turbofan | 771.869 | 0.121204 | 60.54% | 30.50% | 18.47% |
| Military-style Turbofan | 800.846 | 0.116818 | 62.47% | 30.67% | 19.16% |
| Engine | Fs (N·s/kg) | TSFC (kg/N·hr) | Propulsive Efficiency | Thermal Efficiency | Overall Efficiency |
|---|---|---|---|---|---|
| Conventional Turbofan | 616.897 | 0.109334 | 67.38% | 30.38% | 20.47% |
| Military-style Turbofan | 658.395 | 0.102443 | 71.16% | 30.70% | 21.85% |
| Engine | Fs (N·s/kg) | TSFC (kg/N·hr) | Propulsive Efficiency | Thermal Efficiency | Overall Efficiency |
|---|---|---|---|---|---|
| Conventional Turbofan | 447.215 | 0.089937 | 87.97% | 28.29% | 24.89% |
| Military-style Turbofan | 507.307 | 0.079283 | 83.25% | 33.91% | 28.23% |
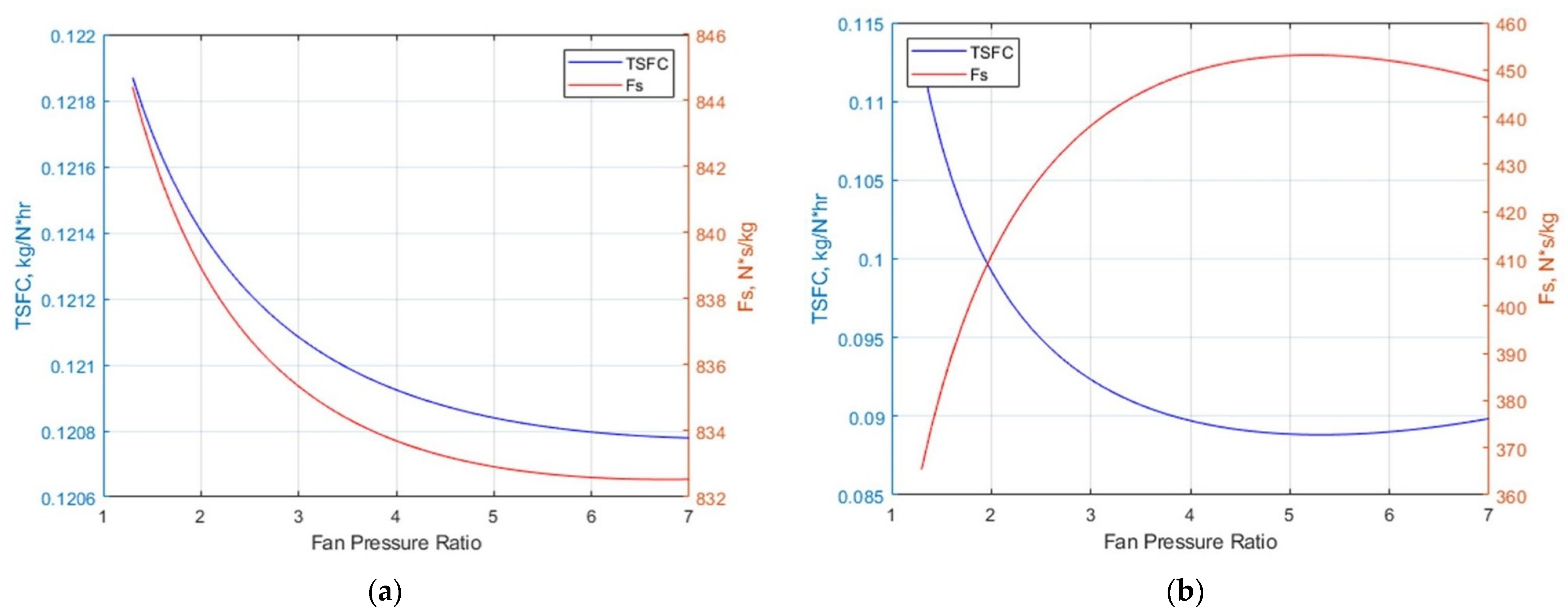
| Bypass Ratio | Optimum FPR (for min TSFC) | TSFC (kg/N·hr) | Optimum FPR (for max Fs) | Fs (N·s/kg) |
|---|---|---|---|---|
| 0.1 | 7 | 0.117173 | 7 | 780.142 |
| 0.2 | 7 | 0.113847 | 7 | 736.021 |
| 0.3 | 7 | 0.110779 | 7 | 698.223 |
| 0.4 | 7 | 0.107948 | 7 | 665.347 |
| 0.5 | 7 | 0.105341 | 7 | 636.363 |
| 0.6 | 7 | 0.102943 | 7 | 610.489 |
| 0.7 | 7 | 0.100743 | 7 | 587.123 |
| 0.8 | 7 | 0.098734 | 7 | 565.788 |
| 0.9 | 7 | 0.096910 | 7 | 546.099 |
| 1.0 | 7 | 0.095267 | 7 | 527.741 |
| 1.1 | 6.7 | 0.093786 | 6.6 | 510.568 |
| 1.2 | 6.2 | 0.092413 | 6.2 | 494.652 |
| 1.3 | 5.9 | 0.091134 | 5.8 | 479.854 |
| 1.4 | 5.6 | 0.089941 | 5.5 | 466.048 |
| 1.5 | 5.3 | 0.088825 | 5.2 | 453.126 |
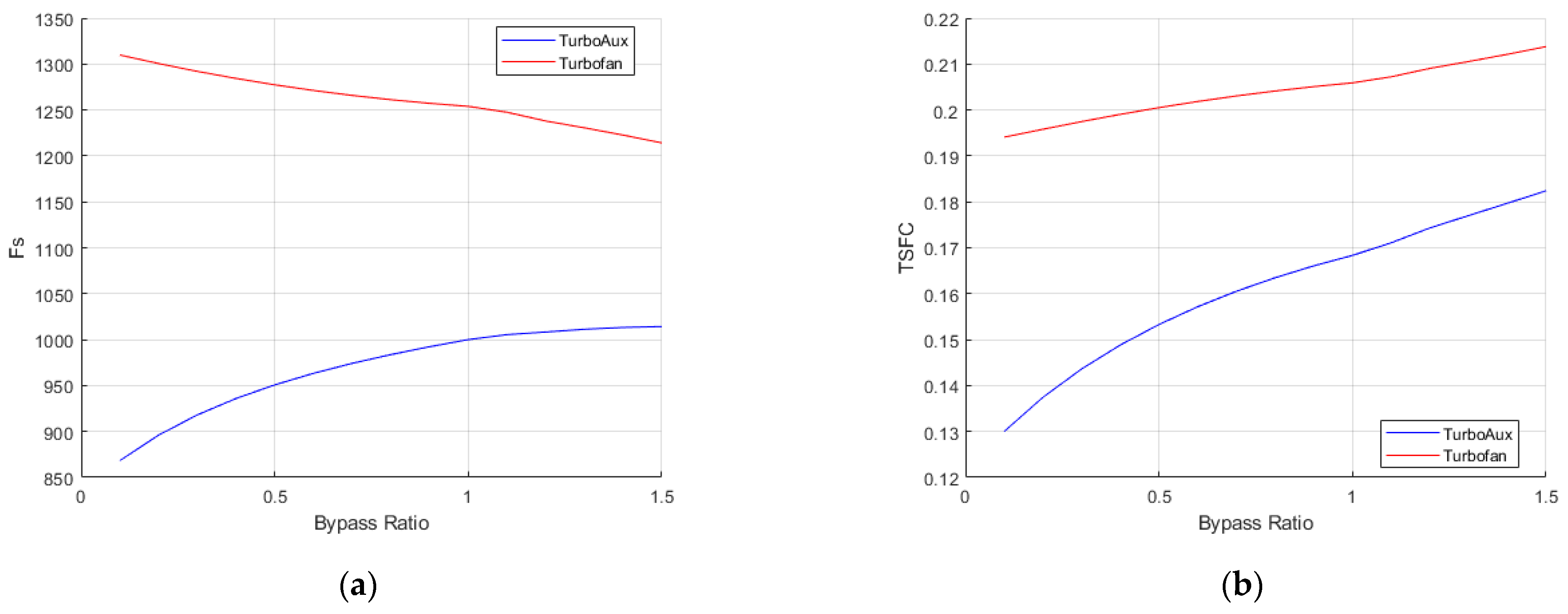
| Engine | Turbojet with Afterburning | Turbofan with Afterburning | TurboAux |
|---|---|---|---|
| FPR | 7 | 7 | 7 |
| Bypass Ratio | N/A | 0.1 | 0.1 |
| Fs | 1319.787153 | 1309.831555 | 868.3308192 |
| TSFC | 0.1923519 | 0.194079469 | 0.12999793 |
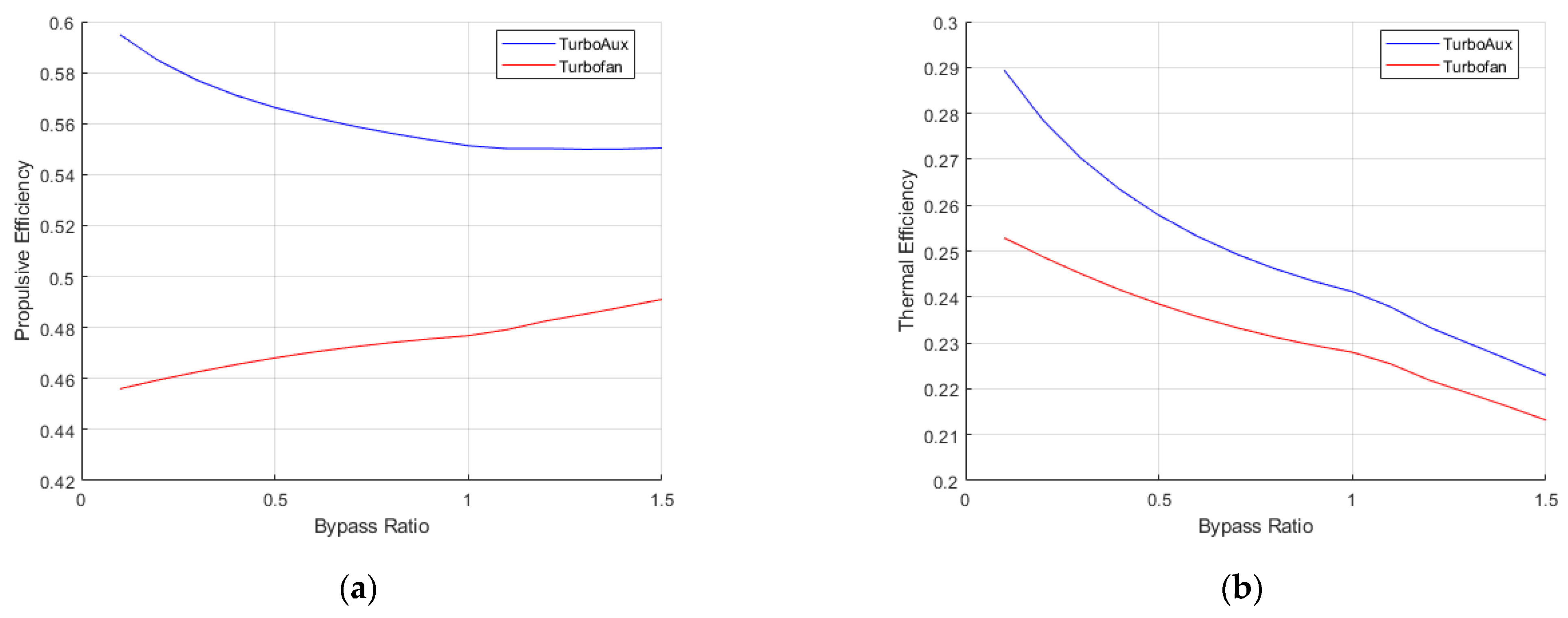
| Propulsive Efficiency | 45.23% | 45.60% | 59.48% |
| Thermal Efficiency | 25.72% | 25.29% | 28.94% |
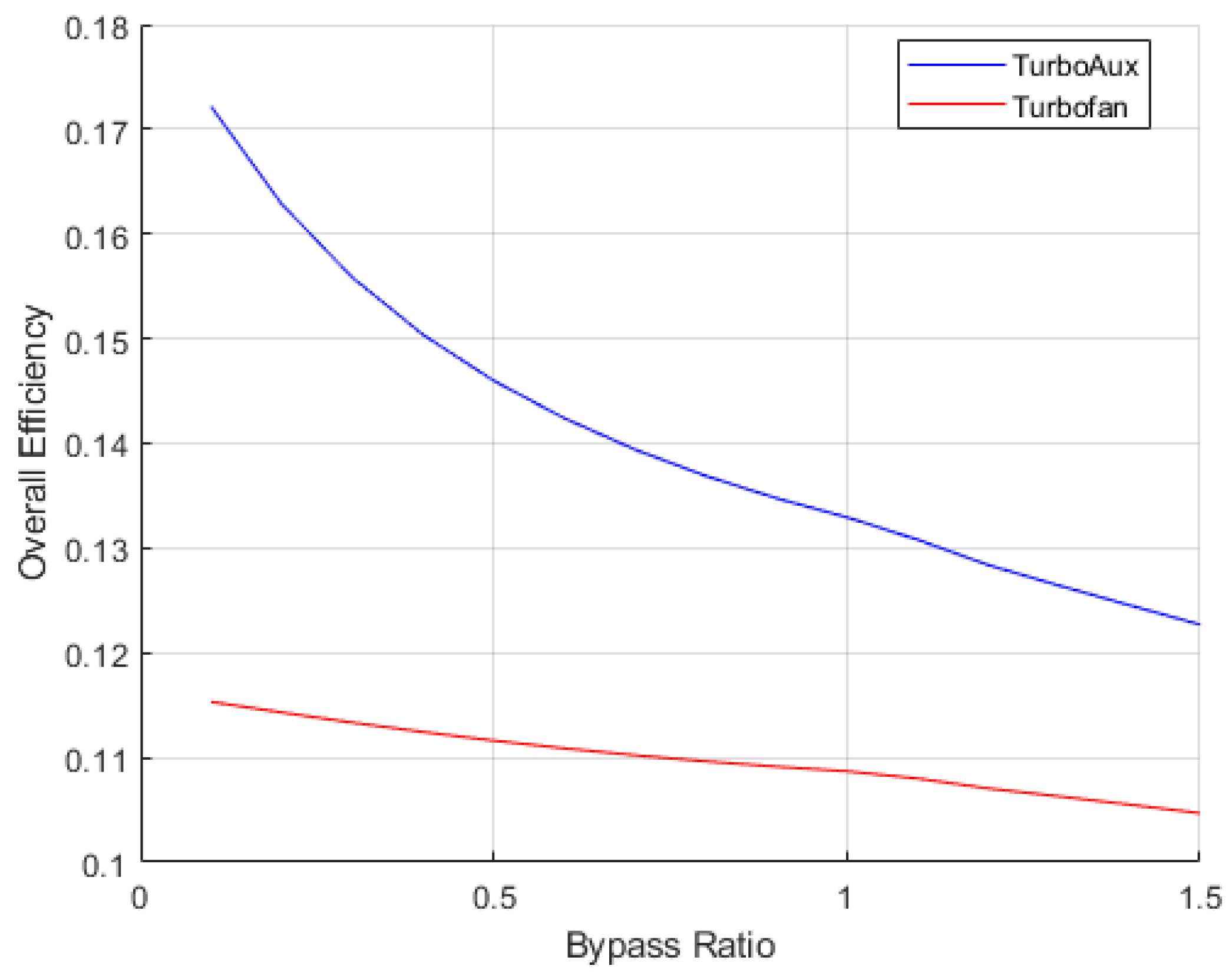
| Overall Efficiency | 11.64% | 11.53% | 17.22% |
| Engine | Turbojet with Afterburning | Turbofan with Afterburning | TurboAux |
|---|---|---|---|
| FPR | 7 | 7 | 7 |
| Bypass Ratio | N/A | 0.8 | 0.8 |
| Fs | 1319.787153 | 1261.226815 | 983.7361302 |
| TSFC | 0.1923519 | 0.204136861 | 0.163484892 |
| Propulsive Efficiency | 45.23% | 47.41% | 55.62% |
| Thermal Efficiency | 25.72% | 23.12% | 24.61% |
| Overall Efficiency | 11.64% | 10.96% | 13.69% |
| Engine | Turbojet with Afterburning | Turbofan with Afterburning | TurboAux |
|---|---|---|---|
| FPR | 5.3 | 5.3 | 5.3 |
| Bypass Ratio | N/A | 1.5 | 1.5 |
| Fs | 1319.826856 | 1214.235908 | 1014.266203 |
| TSFC | 0.192402496 | 0.213796329 | 0.18240472 |
| Propulsive Efficiency | 45.23% | 49.11% | 55.04% |
| Thermal Efficiency | 25.72% | 21.32% | 22.29% |
| Overall Efficiency | 11.63% | 10.47% | 12.27% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Fetahi, K.; Asundi, S.A.; Taylor, A.C. A Comparative Performance Analysis of the Novel TurboAux Engine with a Turbojet Engine, and a Low-Bypass Ratio Turbofan Engine with an Afterburner. Int. J. Turbomach. Propuls. Power 2022, 7, 28. https://doi.org/10.3390/ijtpp7040028
Fetahi K, Asundi SA, Taylor AC. A Comparative Performance Analysis of the Novel TurboAux Engine with a Turbojet Engine, and a Low-Bypass Ratio Turbofan Engine with an Afterburner. International Journal of Turbomachinery, Propulsion and Power. 2022; 7(4):28. https://doi.org/10.3390/ijtpp7040028
Chicago/Turabian StyleFetahi, Kaleab, Sharanabasaweshwara A. Asundi, and Arthur C. Taylor. 2022. "A Comparative Performance Analysis of the Novel TurboAux Engine with a Turbojet Engine, and a Low-Bypass Ratio Turbofan Engine with an Afterburner" International Journal of Turbomachinery, Propulsion and Power 7, no. 4: 28. https://doi.org/10.3390/ijtpp7040028
APA StyleFetahi, K., Asundi, S. A., & Taylor, A. C. (2022). A Comparative Performance Analysis of the Novel TurboAux Engine with a Turbojet Engine, and a Low-Bypass Ratio Turbofan Engine with an Afterburner. International Journal of Turbomachinery, Propulsion and Power, 7(4), 28. https://doi.org/10.3390/ijtpp7040028





